The Partside Packing Company needs a rectangular shipping box. The box must have a length of 1 foot, a width of 8 inches, and a volume of at least 700 cubic inches. What is the least number of inches in height of the box such that the height is a whole number?
The correct Answer is:8
To solve the problem, we need to find the least height of a rectangular shipping box that meets the specified volume requirement. Let's break down the solution step by step.
Step 1: Convert Length to Inches
The length of the box is given as 1 foot. We need to convert this measurement into inches since the width and volume are given in inches.
- Conversion:
Length=1 foot=12 inches
Step 2: Write Down the Known Values
Now that we have the length in inches, we can summarize the known values:
- Length (L) = 12 inches
- Width (W) = 8 inches
- Volume (V) ≥ 700 cubic inches
Step 3: Use the Volume Formula
The volume V of a rectangular box (cuboid) is calculated using the formula:
V=L×W×H
where H is the height of the box.
Step 4: Set Up the Inequality
Since we want the volume to be at least 700 cubic inches, we can set up the following inequality:
12×8×H≥700
Step 5: Calculate the Product of Length and Width
Now, calculate the product of the length and width:
12×8=96
So, we can rewrite the inequality as:
96H≥700
Step 6: Solve for Height (H)
To find H, divide both sides of the inequality by 96:
H≥70096
Calculating the right side:
H≥7.29166667
Step 7: Find the Least Whole Number
Since we need the height H to be a whole number, we take the smallest whole number that is greater than or equal to 7.29166667, which is 8.
Final Answer
Thus, the least height of the box that meets the requirements is:
Height=8 inches
---
The length of the box is given as 1 foot. We need to convert this measurement into inches since the width and volume are given in inches.
Length=1 foot=12 inches
Now that we have the length in inches, we can summarize the known values:
- Length (L) = 12 inches
- Width (W) = 8 inches
- Volume (V) ≥ 700 cubic inches
The volume V of a rectangular box (cuboid) is calculated using the formula:
V=L×W×H
where H is the height of the box.
Since we want the volume to be at least 700 cubic inches, we can set up the following inequality:
12×8×H≥700
Now, calculate the product of the length and width:
12×8=96
So, we can rewrite the inequality as:
96H≥700
To find H, divide both sides of the inequality by 96:
H≥70096
Calculating the right side:
H≥7.29166667
Since we need the height H to be a whole number, we take the smallest whole number that is greater than or equal to 7.29166667, which is 8.
Thus, the least height of the box that meets the requirements is:
Height=8 inches
Topper's Solved these Questions
- BOOK - ENGLISH SATCHAPTER - ADDITIONAL TOPICS IN MATHEXERCISE - EXERCISE45videos
ADDITIONAL TOPICS IN MATH
- BOOK - ENGLISH SATCHAPTER - ADDITIONAL TOPICS IN MATHEXERCISE - Multiple Choice99videos
ADDITIONAL TOPICS IN MATH
- BOOK - ENGLISH SATCHAPTER - ADDITIONAL TOPICS IN MATH EXERCISE - PRACTICE TEST24videos
ADDITIONAL TOPICS IN MATH
- BOOK - ENGLISH SATCHAPTER - COMPLEX NUMBERSEXERCISE - EXERCISE6videos
COMPLEX NUMBERS
Similar Questions
A closed rectangular box is made of wood of 1.5cm thickness. The exterior length and breadth are respectively 78cm and 19cm, and the capacity of the box is 15 cubic decimetres. Calculate the exterior height of the box
View SolutionTwo brands of chocolates are available in packs of 24 and 15 respectively. If I need to buy an equal number of chocolates of both kinds, what is the least number of boxes of each kind I would need to buy?
View Solution
Knowledge Check
- Question 1 - Select One
The volume of a rectangle box is 144 cubic inches. The height of the solid is 8 inches. Which measurements, in inches, could be the dimensions of the base?
A3.3×5.5B2.5×7.2C12.0×8.0D9.0×9.0 - Question 2 - Select One
A 3-inch-tall rectangular box with a square base is constructed to hold a circular pie that has a diameter of 8 inches. Both are shown below. What is the volume, in cubic inches, of the smallest such box that can hold this pie?
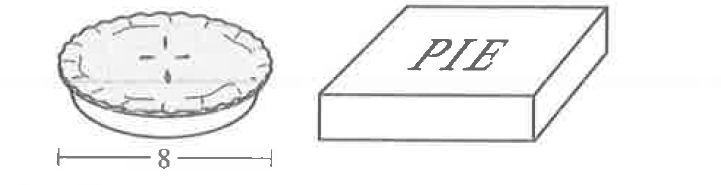 A24B64C72D192
A24B64C72D192 - Question 3 - Select One
A cylinder has a volume of 72π cubic inches and a height of 8 inches. If the height is increased by 4 inches, what will be the new volume of the cylinder in cubic inches?
A76πB108πC328πD576π
A shipping service restricts the dimensions of the boxes it will ship for a certain type of service. The restriction states that for boxes shaped like rectangular prisms, the sum of the perimeter of the base of the box and the height of the box cannot exceed 130 inches. The perimeter of the base is determined using the width and length of the box. If a box has a height of 60 inches and its length is 2.5 times the width, which inequality shows the allowable width x, in inches, of the box?
View SolutionA software company is selling a new game in a standard edition and a collector’s edition. The box for the standard edition has a volume of 20 cubic inches, and the box for the collector’s edition has a volume of 30 cubic inches. The company receives an order for 75 copies of the game, and the total volume of the order to be shipped is 1,870 cubic inches. Which of the following systems of equations can be used to determine the number of standard edition games, s, and collector’s edition games, c, that were ordered?
View SolutionA small, open-top packing box, similar to a shoebox without a lid, is three times as long as it is wide, and half as high as it is long. Each square inch of the bottom of the box costs $0.80 to produce, while each square inch of any side costs $0.03 to produce. If x represents the number of inches in the width of the box,which of the following functions represents the cost, C, of producing the box?
View SolutionTo ship figurines, the figurines are placed in a rectangular box and then small packing pellets are added. The base of the box has an area of 4.4∈2, and the height of the box is 6.5 in. IF the volume of the air in the box after the figures and pellets are added is 8.0∈3 , which of the following is closest to the combined volume of the figurines and pellets in the box?
View SolutionBarb is going to cover a rectangular area 8 feet by 10 feet with rectangular paving blocks that are 4 inches by 8 inches by 2 inches to make a flat patio. What is the minimum number of paving blocks she will need if all the paving blocks will face the same direction?
(Note: Barb will not cut any of the paving blocks.)View Solution

