A small, open-top packing box, similar to a shoebox without a lid, is three times as long as it is wide, and half as high as it is long. Each square inch of the bottom of the box costs $0.80 to produce, while each square inch of any side costs $0.03 to produce. If x represents the number of inches in the width of the box,which of the following functions represents the cost, C, of producing the box?
C(x)=0.42x2
C(x)=0.60x2
C(x)=0.72x2
C(x)=0.96x2
The correct Answer is:B
To solve the problem, we need to derive a cost function C for the packing box based on its dimensions and the costs associated with producing its surfaces. Let's break it down step by step.
Step 1: Define the dimensions of the box
Let:
- x = width of the box (in inches)
- Length l=3x (since the length is three times the width)
- Height h=l2=3x2 (since the height is half the length)
Step 2: Calculate the area of the bottom of the box
The area of the bottom of the box is given by:
Area of the bottom=Length×Width=l×x=(3x)×x=3x2 square inches
Step 3: Calculate the cost of the bottom
The cost to produce the bottom is:
Cost of the bottom=Area of the bottom×Cost per square inch=3x2×0.80=2.4x2 dollars
Step 4: Calculate the area of the sides of the box
The box has four sides:
1. Two sides with dimensions h×x
2. Two sides with dimensions h×l
Calculating the area of the two sides with dimensions h×x:
Area of two sides=2×(h×x)=2×(3x2×x)=3x2 square inches
Calculating the area of the two sides with dimensions h×l:
Area of two sides=2×(h×l)=2×(3x2×3x)=9x2 square inches
Step 5: Total area of the sides
The total area of the sides is:
Total area of sides=3x2+9x2=12x2 square inches
Step 6: Calculate the cost of the sides
The cost to produce the sides is:
Cost of the sides=Total area of sides×Cost per square inch=12x2×0.03=0.36x2 dollars
Step 7: Total cost function
The total cost C of producing the box is the sum of the costs of the bottom and the sides:
C=Cost of the bottom+Cost of the sides=2.4x2+0.36x2=2.76x2 dollars
Final Answer
Thus, the function that represents the cost C of producing the box is:
C(x)=2.76x2
Let:
- x = width of the box (in inches)
- Length l=3x (since the length is three times the width)
- Height h=l2=3x2 (since the height is half the length)
The area of the bottom of the box is given by:
Area of the bottom=Length×Width=l×x=(3x)×x=3x2 square inches
The cost to produce the bottom is:
Cost of the bottom=Area of the bottom×Cost per square inch=3x2×0.80=2.4x2 dollars
The box has four sides:
1. Two sides with dimensions h×x
2. Two sides with dimensions h×l
Area of two sides=2×(h×x)=2×(3x2×x)=3x2 square inches
Area of two sides=2×(h×l)=2×(3x2×3x)=9x2 square inches
The total area of the sides is:
Total area of sides=3x2+9x2=12x2 square inches
The cost to produce the sides is:
Cost of the sides=Total area of sides×Cost per square inch=12x2×0.03=0.36x2 dollars
The total cost C of producing the box is the sum of the costs of the bottom and the sides:
C=Cost of the bottom+Cost of the sides=2.4x2+0.36x2=2.76x2 dollars
Thus, the function that represents the cost C of producing the box is:
C(x)=2.76x2
Topper's Solved these Questions
Similar Questions
A box of constant volume c is to be twice as long as it is wide. The material on the top and four sides cost three times as much per square metre as that in the bottom. What are the most economic dimensions?
View SolutionFind the perimeter of a table 34.5 inches wide and 58.5 inches long.
View Solution
Knowledge Check
- Question 1 - Select One
The length of a rectangular banner is 3 feet 2 inches, and the width is 2 feet 4 inches. Which one of the following equals the area of the banner?
A5 sq. feetB5 1/2 sq. feetC6 1/3 sq. feetD7 7/18 sq. feet - Question 2 - Select One
A circle has a radius that is the same length as the sides of a square , if the square has a perimeter of 64 square inches , what is the area , in square inches , of the circle ?
A16B16πC32πD256π - Question 3 - Select One
A table screen has a 12-inch diagonal. If the length of the screen is √3 times longer than the width, what is the area, in square inches, of the screen ?
A12B12√3C36D36√3
The Partside Packing Company needs a rectangular shipping box. The box must have a length of 1 foot, a width of 8 inches, and a volume of at least 700 cubic inches. What is the least number of inches in height of the box such that the height is a whole number?
View SolutionWhat is the longest diagonal of a rectangular box that is 120 inches long, 90 inches wide, and 80 inches tall?
View Solution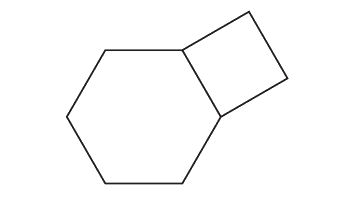
The figure above shows a regular hexagon with sides of length a and a square with sides of length a. If the area of the hexagon is 384 √3 square inches, what is the area, in square inches, of the square?View SolutionThe dimensions of a block of cheese are 12 inches by 3 inches by 3 inches. What is the volume , in cubic inches , of the block of cheese ?
View SolutionIf a sector of a circle has an arc length of 2π inches and an area of 6π square inches, what is the length of the radius of the circle?
View Solution

