The probability distribution of a random variable X is given as under: P(X=x)={kx2forx=1,2,32kxforx=4,5,60otherwise , where k is a constant. Find (i) P(X≥4 ) (ii) E(X) (iii) E(3X2)
To solve the problem step by step, we will follow the instructions provided in the video transcript.
Step 1: Define the Probability Distribution
The probability distribution of the random variable X is given as:
P(X=x)=⎧⎨⎩kx2for x=1,2,32kxfor x=4,5,60otherwise
Step 2: Calculate the Value of k
To find the constant k, we need to ensure that the sum of all probabilities equals 1:
P(X=1)+P(X=2)+P(X=3)+P(X=4)+P(X=5)+P(X=6)=1
Calculating each probability:
- P(X=1)=k⋅12=k
- P(X=2)=k⋅22=4k
- P(X=3)=k⋅32=9k
- P(X=4)=2k⋅4=8k
- P(X=5)=2k⋅5=10k
- P(X=6)=2k⋅6=12k
Now, summing these probabilities:
k+4k+9k+8k+10k+12k=44k
Setting the sum equal to 1:
44k=1⟹k=144
Step 3: Calculate P(X≥4)
Now we need to find P(X≥4):
P(X≥4)=P(X=4)+P(X=5)+P(X=6)
Calculating each:
- P(X=4)=8k=8⋅144=844=211
- P(X=5)=10k=10⋅144=1044=522
- P(X=6)=12k=12⋅144=1244=311
Now summing these probabilities:
P(X≥4)=211+522+311
To add these fractions, we convert them to a common denominator (which is 22):
211=422,311=622
Thus,
P(X≥4)=422+522+622=1522
Step 4: Calculate E(X)
The expected value E(X) is calculated as:
E(X)=∑P(X=x)⋅x
Calculating each term:
E(X)=k⋅1+4k⋅2+9k⋅3+8k⋅4+10k⋅5+12k⋅6
Substituting k=144:
E(X)=144(1+8+27+32+50+72)
Calculating the sum inside the parentheses:
1+8+27+32+50+72=190
Thus,
E(X)=19044=9522
Step 5: Calculate E(3X2)
To find E(3X2):
E(3X2)=3∑P(X=x)⋅x2
Calculating each term:
E(3X2)=3(k⋅12+4k⋅22+9k⋅32+8k⋅42+10k⋅52+12k⋅62)
Substituting k=144:
E(3X2)=3⋅144(1+16+81+128+250+432)
Calculating the sum inside the parentheses:
1+16+81+128+250+432=908
Thus,
E(3X2)=3⋅90844=272444=68111
Final Answers
(i) P(X≥4)=1522
(ii) E(X)=9522
(iii) E(3X2)=68111
The probability distribution of the random variable X is given as:
P(X=x)=⎧⎨⎩kx2for x=1,2,32kxfor x=4,5,60otherwise
To find the constant k, we need to ensure that the sum of all probabilities equals 1:
P(X=1)+P(X=2)+P(X=3)+P(X=4)+P(X=5)+P(X=6)=1
Calculating each probability:
- P(X=1)=k⋅12=k
- P(X=2)=k⋅22=4k
- P(X=3)=k⋅32=9k
- P(X=4)=2k⋅4=8k
- P(X=5)=2k⋅5=10k
- P(X=6)=2k⋅6=12k
k+4k+9k+8k+10k+12k=44k
Setting the sum equal to 1:
44k=1⟹k=144
Now we need to find P(X≥4):
P(X≥4)=P(X=4)+P(X=5)+P(X=6)
Calculating each:
- P(X=4)=8k=8⋅144=844=211
- P(X=5)=10k=10⋅144=1044=522
- P(X=6)=12k=12⋅144=1244=311
P(X≥4)=211+522+311
To add these fractions, we convert them to a common denominator (which is 22):
211=422,311=622
Thus,
P(X≥4)=422+522+622=1522
The expected value E(X) is calculated as:
E(X)=∑P(X=x)⋅x
Calculating each term:
E(X)=k⋅1+4k⋅2+9k⋅3+8k⋅4+10k⋅5+12k⋅6
Substituting k=144:
E(X)=144(1+8+27+32+50+72)
Calculating the sum inside the parentheses:
1+8+27+32+50+72=190
Thus,
E(X)=19044=9522
To find E(3X2):
E(3X2)=3∑P(X=x)⋅x2
Calculating each term:
E(3X2)=3(k⋅12+4k⋅22+9k⋅32+8k⋅42+10k⋅52+12k⋅62)
Substituting k=144:
E(3X2)=3⋅144(1+16+81+128+250+432)
Calculating the sum inside the parentheses:
1+16+81+128+250+432=908
Thus,
E(3X2)=3⋅90844=272444=68111
(i) P(X≥4)=1522
(ii) E(X)=9522
(iii) E(3X2)=68111
Topper's Solved these Questions
Similar Questions
The probability distribution of a random variable x is given as under
P(X=x)=⎧⎪⎨⎪⎩kx2x=1,2,32kxx=4,5,60,otherwise
where, k is a constant. Calculate
(i) E(X) (ii) E(3X2) (iii) P(X≥4)View SolutionIf the probability distribution of a random variable X is given by X=xi: 1 2 3 4 P(X=xi): 2k 4k 3k k Write the value of k .
View Solution
Knowledge Check
- Question 1 - Select One
The probability distribution of a random variable X is given as follows:
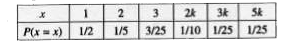
If E(X)=2.3 , then value of k isA1/2B5/4C3D2 - Question 2 - Select One
The probability distribution of a random variable X is given by.
X=x:01234P(X=x):0.40.30.10.10.1
The variance of X, isA1.76B2.45C3.2D4.8 - Question 3 - Select One
If the probability mass function of a discrete random variable X is
P(x)=Cx3,x=1,2,3
=0, otherwise
Then E(X)=A343297B294251C297294D251294
The probability distribution of a discrate random variable X is :
X=x12345P(X=x)k2k3k4k5k
Find P(X≤4).View SolutionThe probability distribution of a discrete random variable x is given as under
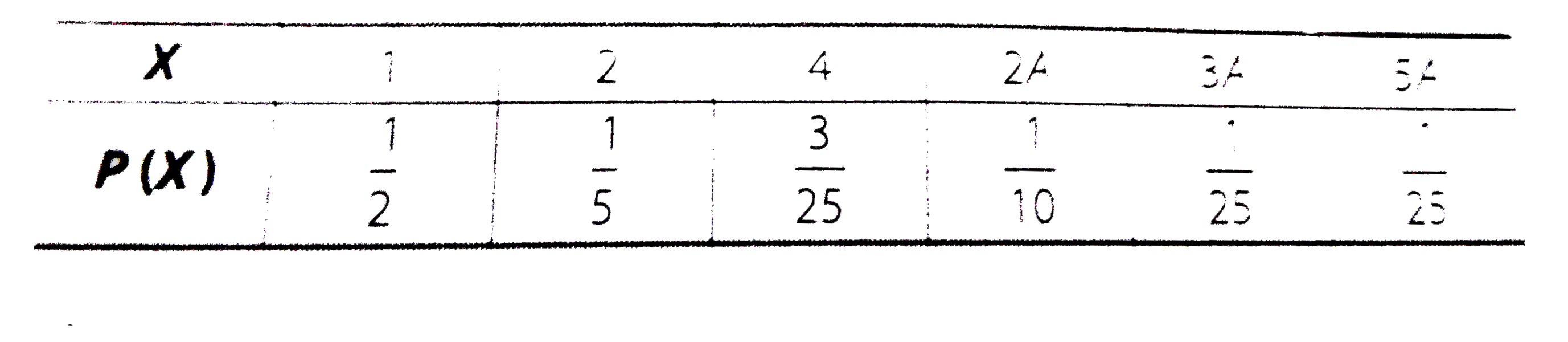
Calculate
(i) the value of A, if E(X)=2.94.
(ii) variance of X.View SolutionThe probability distribution of discrete random variable X is given below
x2345P(x)5K7k9k11k
find the value of K.View SolutionLet X be a discrete random variable whoose probability distribution is defined as follows.
P(X=x)=⎧⎪⎨⎪⎩k(x+1),for x=1,2,3,42kx, for x=5,6,70, otherwise
where,k is a constant. Calculate (i) the value of k. (ii) E (X).
(ii) standard deviation of X.View SolutionThe probability distribution for a random variable X is as follows:
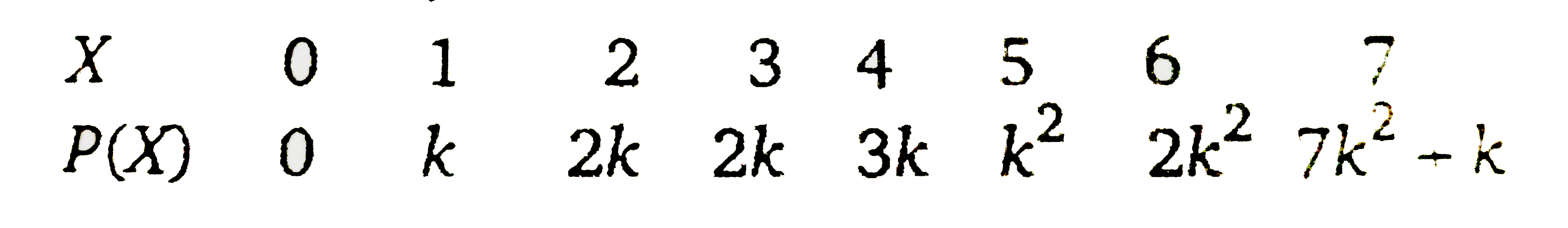
Find:
(i) k (ii) P(X≥3)
(iii) P(X≥6) (iv) P(0<X<3)View Solution
