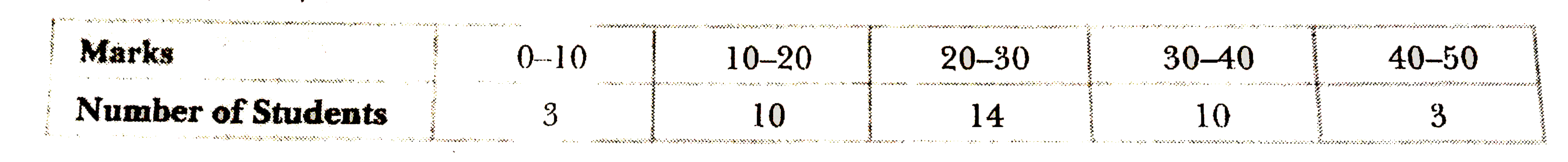There are 60 students in a class. The following is the frequency distribution of marks obtained by the students in a test: Marks:, 0, 1, 2, 3, 4, 5 Frequency , x−2 , x , x2 , (x+1)2 , 2x , x+1. Determine mean and standard deviation of the marks.
To solve the problem step by step, we will first determine the value of x using the given frequency distribution, and then we will calculate the mean and standard deviation of the marks obtained by the students.
Step 1: Determine the value of x
We know that the total frequency of students is 60. Therefore, we can set up the equation:
(x−2)+x+x2+(x+1)2+2x+(x+1)=60
Now, let's simplify this equation:
1. Expand (x+1)2:
(x+1)2=x2+2x+1
2. Substitute back into the equation:
(x−2)+x+x2+(x2+2x+1)+2x+(x+1)=60
3. Combine like terms:
x−2+x+x2+x2+2x+1+2x+x+1=60
2x2+7x+0=60
4. Rearranging gives us:
2x2+7x−60=0
Step 2: Solve the quadratic equation
Now we will solve the quadratic equation 2x2+7x−60=0 using the factorization method or the quadratic formula.
1. Factor the quadratic:
(2x−8)(x+15)=0
2. Set each factor to zero:
2x−8=0⇒x=4
x+15=0⇒x=−15(not valid since x must be positive)
Thus, x=4.
Step 3: Calculate the frequencies
Now we substitute x=4 back into the frequency distribution:
- Marks: 0, 1, 2, 3, 4, 5
- Frequencies:
- x−2=4−2=2
- x=4
- x2=42=16
- (x+1)2=(4+1)2=25
- 2x=2×4=8
- x+1=4+1=5
So the frequency table becomes:
| Marks | Frequency |
|-------|-----------|
| 0 | 2 |
| 1 | 4 |
| 2 | 16 |
| 3 | 25 |
| 4 | 8 |
| 5 | 5 |
Step 4: Calculate the mean
The mean μ is calculated using the formula:
μ=∑(fi⋅xi)∑fi
Calculating fi⋅xi:
- 0⋅2=0
- 1⋅4=4
- 2⋅16=32
- 3⋅25=75
- 4⋅8=32
- 5⋅5=25
Now, summing these products:
0+4+32+75+32+25=168
The total frequency ∑fi=60.
Thus, the mean is:
μ=16860=2.8
Step 5: Calculate the standard deviation
To find the standard deviation, we first need to calculate the variance σ2:
σ2=∑(fi⋅x2i)n−μ2
Calculating fi⋅x2i:
- 02⋅2=0
- 12⋅4=4
- 22⋅16=64
- 32⋅25=225
- 42⋅8=128
- 52⋅5=125
Now, summing these products:
0+4+64+225+128+125=546
Now, substituting into the variance formula:
σ2=54660−(2.8)2
σ2=9.1−7.84=1.26
Finally, the standard deviation σ is:
σ=√1.26≈1.12
Final Answers
- Mean: 2.8
- Standard Deviation: 1.12
(x+1)2=x2+2x+1
(x−2)+x+x2+(x2+2x+1)+2x+(x+1)=60
x−2+x+x2+x2+2x+1+2x+x+1=60
2x2+7x+0=60
2x2+7x−60=0
(2x−8)(x+15)=0
2x−8=0⇒x=4
x+15=0⇒x=−15(not valid since x must be positive)
- Frequencies:
- x−2=4−2=2
- x=4
- x2=42=16
- (x+1)2=(4+1)2=25
- 2x=2×4=8
- x+1=4+1=5
|-------|-----------|
| 0 | 2 |
| 1 | 4 |
| 2 | 16 |
| 3 | 25 |
| 4 | 8 |
| 5 | 5 |
- 1⋅4=4
- 2⋅16=32
- 3⋅25=75
- 4⋅8=32
- 5⋅5=25
- 12⋅4=4
- 22⋅16=64
- 32⋅25=225
- 42⋅8=128
- 52⋅5=125
σ2=9.1−7.84=1.26
- Standard Deviation: 1.12
Topper's Solved these Questions
Similar Questions
Knowledge Check
If for somes x∈R the frequency distribution of marks obtained by 20 students in a test is:

Then the mean of the marks is:A2.5B3.0C2.8D3.2If for some x∈R the frequency distribution of the marks obtained by 20 statement in a test is :
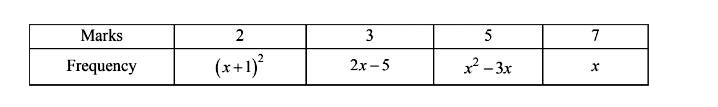
Marks2357Frequency(x+12)(2x−5)(x2−3x)x then the mean of the marks is :A3.2B3C2.5D2.8
There are 60 students in a class. The following is the frequency distribution of the marks obtained by the students in a test:

Where "p" is positive integer. Determine the mean and standard deviation of the marks.
View SolutionIf for somex∈R, the frequency distribution of the marks obtained by 20 students in a test is:
Then the mean of the marks is:
View SolutionThe following frequency distbution table shows marks obtained by 180 students in Mathematics examination

Find the value of x .
View SolutionThe following table shows frequency distribution of marks secured by 100 students in a particular examination. Find the mean of the marks.
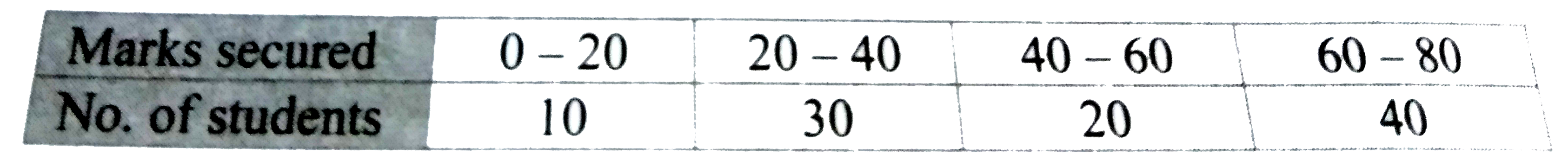
View SolutionPercentage of marks of 35 students is given in the following grouped frequency distribution table.

Which class has highest frequency?
View SolutionThe frequency distribution of the marks obtained by 28 students in a test carrying 40 marks is given below :

If the mean of the above data is 20 , then find the difference between x and y .
View Solution