Two chords AB and CD of lengths 24 cm and 10 cm respectively of a circle are parallel. If the chords lie on the same side of the centre and distance between them is 7 cm, find the length of a diameter of the circle.
The correct Answer is:26cm
To find the diameter of the circle given the lengths of two parallel chords and the distance between them, we can follow these steps:
Step 1: Understand the Problem
We have two chords AB and CD of lengths 24 cm and 10 cm, respectively. The distance between the two chords is 7 cm. We need to find the diameter of the circle.
Step 2: Bisect the Chords
Since the chords are parallel, we can drop perpendiculars from the center of the circle to each chord. Let’s denote the midpoint of AB as M and the midpoint of CD as N.
- Length of AB = 24 cm, so AM = MB = 12 cm (since M bisects AB).
- Length of CD = 10 cm, so CN = ND = 5 cm (since N bisects CD).
Step 3: Set Up the Geometry
Let the distance from the center of the circle to chord AB be h and the distance from the center to chord CD be h+7 (since the distance between the two chords is 7 cm).
Step 4: Apply the Pythagorean Theorem
We can apply the Pythagorean theorem in triangles formed by the radius, the half-length of the chord, and the distance from the center to the chord.
For chord AB:
r2=h2+122
r2=h2+144(1)
For chord CD:
r2=(h+7)2+52
r2=(h+7)2+25(2)
Step 5: Expand Equation (2)
Expanding equation (2):
r2=(h2+14h+49)+25
r2=h2+14h+74(3)
Step 6: Set Equations Equal
Since both equations (1) and (3) equal r2, we can set them equal to each other:
h2+144=h2+14h+74
Step 7: Simplify the Equation
Cancel h2 from both sides:
144=14h+74
Subtract 74 from both sides:
70=14h
Divide by 14:
h=5
Step 8: Find the Radius
Now substitute h=5 back into equation (1) to find the radius:
r2=52+144
r2=25+144
r2=169
Taking the square root:
r=13cm
Step 9: Calculate the Diameter
The diameter D of the circle is given by:
D=2r
D=2×13=26cm
Final Answer
The length of the diameter of the circle is 26 cm.
We have two chords AB and CD of lengths 24 cm and 10 cm, respectively. The distance between the two chords is 7 cm. We need to find the diameter of the circle.
Since the chords are parallel, we can drop perpendiculars from the center of the circle to each chord. Let’s denote the midpoint of AB as M and the midpoint of CD as N.
- Length of CD = 10 cm, so CN = ND = 5 cm (since N bisects CD).
Let the distance from the center of the circle to chord AB be h and the distance from the center to chord CD be h+7 (since the distance between the two chords is 7 cm).
We can apply the Pythagorean theorem in triangles formed by the radius, the half-length of the chord, and the distance from the center to the chord.
r2=h2+122
r2=h2+144(1)
r2=(h+7)2+52
r2=(h+7)2+25(2)
Expanding equation (2):
r2=(h2+14h+49)+25
r2=h2+14h+74(3)
Since both equations (1) and (3) equal r2, we can set them equal to each other:
h2+144=h2+14h+74
Cancel h2 from both sides:
144=14h+74
Subtract 74 from both sides:
70=14h
Divide by 14:
h=5
Now substitute h=5 back into equation (1) to find the radius:
r2=52+144
r2=25+144
r2=169
Taking the square root:
r=13cm
The diameter D of the circle is given by:
D=2r
D=2×13=26cm
The length of the diameter of the circle is 26 cm.
Topper's Solved these Questions
- CHAPTERWISE REVISION (STAGE 1) BOOK - ICSECHAPTER - CHAPTERWISE REVISION (STAGE 1) EXERCISE - Statistics
9 Videos
- CHAPTERWISE REVISION (STAGE 1) BOOK - ICSECHAPTER - CHAPTERWISE REVISION (STAGE 1) EXERCISE - mean and median
12 Videos
- CHAPTERWISE REVISION (STAGE 1) BOOK - ICSECHAPTER - CHAPTERWISE REVISION (STAGE 1) EXERCISE - Area theorems
11 Videos
- CHAPTER REVISION (STAGE 2) BOOK - ICSECHAPTER - CHAPTER REVISION (STAGE 2) EXERCISE - DISTANCE FORMULA
12 Videos
- CHAPTERWISE REVISION (STAGE 3) BOOK - ICSECHAPTER - CHAPTERWISE REVISION (STAGE 3) EXERCISE - DISTANCE FORMULA
11 Videos
Similar Questions
Two chords AB and CD of lengths 5 cm and 11 cm respectively of a circle are parallel to each other and are on opposite sides of its centre. If the distance between AB and CD is 6 cm, find the radius of the circle.
View SolutionTwo chords AB and CD of lengths 5 cm and 11 cm respectively of a circle are parallel to each other and are on opposite sides of its centre. If the distance between AB and CD is 6 cm, find the radius of the circle.
View Solution
Knowledge Check
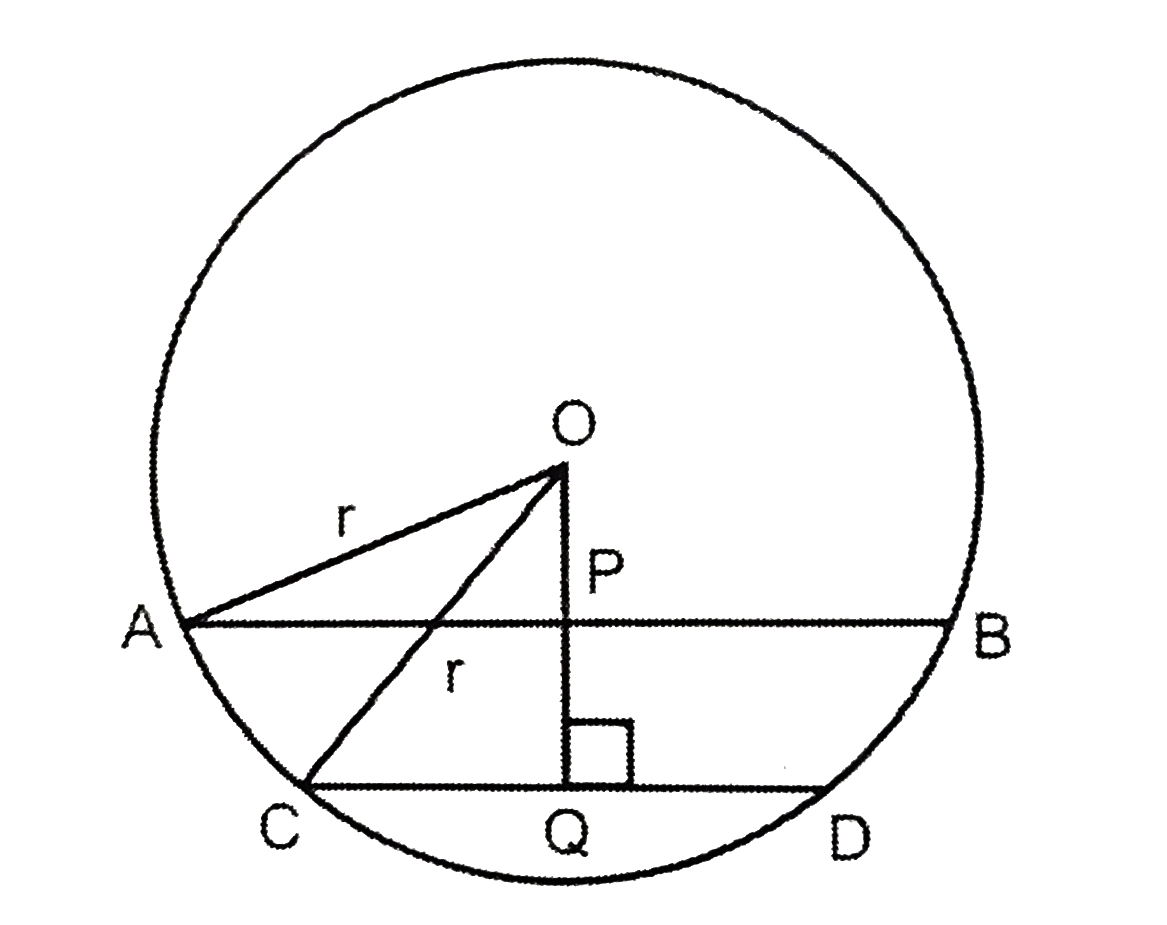
2 parallel chords 24 cm, and 18 cm, are on the same side of the centre of a circle. If the distance between the chords is 3 cm, calculate the radius of the circle.A15 cmB14 cmC13 cmD12 cm
Two chords AB and CD of lengths 5 cm and 11 cm respectively of a circle are parallel to each other and are on opposite sides of its centre. If the distance between AB and CD is 6 cm, find the radius of the circle.
View SolutionTwo parallel chords of a circle , 12 cm and 16 cm long are on the same of the centre. The distance between them is 2 cm. Find the radius of the circle.
View SolutionChords AB and CD of a circle are parallel to each other and lie on opposite sides of the centre of the circle. If AB = 36 cm, CD = 48 cm and the distance between the chords is 42 cm, find the radius of the circle.
View SolutionFind the area of a trapezium whose parallel sides are of lengths 10cm and 12cm and the distance between them is 4cm.
View SolutionAB and CD are two parallel chords of a circle which are on opposite sides of the centre such that AB=10cm, CD=24cm and the distance between AB and CD is 17cm. Find the radius of the circle.
View SolutionAB and CD are two chords such that AB = 10 cm , CD = 24 cm and AB // CD The distance between the chords is 17 cm . Find the radius of the circle.
View SolutionA chord of length 24 cm is at a distance of 5 cm from the centre of the circle. Find the length of the chord of the same circle which is at a distance of 12 cm from the centre.
View Solution
