Two line segment AB and AC include an angle of 60∘, where AB=5 cm and AC= 7 cm. Locate points P and Q on AB and AC, respectively such that AP=34AB and AQ=14AC. Join P and Q and measure the length PQ.
To solve the problem step-by-step, we will follow the instructions given in the question and use the necessary geometric principles.
Step 1: Draw the Diagram
1. Draw two line segments AB and AC such that they form an angle of 60 degrees between them.
2. Label the points: A at the vertex, B at the end of segment AB, and C at the end of segment AC.
Step 2: Mark the Lengths of the Segments
1. Measure and mark the length of segment AB as 5 cm.
2. Measure and mark the length of segment AC as 7 cm.
Step 3: Locate Points P and Q
1. Point P is located on segment AB such that AP=34AB.
- Calculate AP=34×5 cm=154 cm=3.75 cm.
- Therefore, PB=AB−AP=5 cm−3.75 cm=1.25 cm.
2. Point Q is located on segment AC such that AQ=14AC.
- Calculate AQ=14×7 cm=74 cm=1.75 cm.
- Therefore, QC=AC−AQ=7 cm−1.75 cm=5.25 cm.
Step 4: Use the Cosine Rule to Find PQ
1. We need to find the length of segment PQ. We can use the cosine rule in triangle APQ.
- The lengths we have are:
- AP=3.75 cm (let's denote it as X)
- AQ=1.75 cm (let's denote it as Y)
- The angle ∠PAQ=60∘.
2. According to the cosine rule:
PQ2=AP2+AQ2−2⋅AP⋅AQ⋅cos(60∘)
- Substitute the values:
PQ2=(3.75)2+(1.75)2−2⋅(3.75)⋅(1.75)⋅12
- Calculate (3.75)2=14.0625 and (1.75)2=3.0625.
- Calculate 2⋅(3.75)⋅(1.75)⋅12=3.75⋅1.75=6.5625.
- Now substitute these values:
PQ2=14.0625+3.0625−6.5625=10.5625
- Therefore, PQ=√10.5625≈3.25 cm.
Final Answer
The length of segment PQ is approximately 3.25 cm.
---
1. Draw two line segments AB and AC such that they form an angle of 60 degrees between them.
2. Label the points: A at the vertex, B at the end of segment AB, and C at the end of segment AC.
1. Measure and mark the length of segment AB as 5 cm.
2. Measure and mark the length of segment AC as 7 cm.
1. Point P is located on segment AB such that AP=34AB.
- Calculate AP=34×5 cm=154 cm=3.75 cm.
- Therefore, PB=AB−AP=5 cm−3.75 cm=1.25 cm.
2. Point Q is located on segment AC such that AQ=14AC.
- Calculate AQ=14×7 cm=74 cm=1.75 cm.
- Therefore, QC=AC−AQ=7 cm−1.75 cm=5.25 cm.
1. We need to find the length of segment PQ. We can use the cosine rule in triangle APQ.
- The lengths we have are:
- AP=3.75 cm (let's denote it as X)
- AQ=1.75 cm (let's denote it as Y)
- The angle ∠PAQ=60∘.
2. According to the cosine rule:
PQ2=AP2+AQ2−2⋅AP⋅AQ⋅cos(60∘)
- Substitute the values:
PQ2=(3.75)2+(1.75)2−2⋅(3.75)⋅(1.75)⋅12
- Calculate (3.75)2=14.0625 and (1.75)2=3.0625.
- Calculate 2⋅(3.75)⋅(1.75)⋅12=3.75⋅1.75=6.5625.
- Now substitute these values:
PQ2=14.0625+3.0625−6.5625=10.5625
- Therefore, PQ=√10.5625≈3.25 cm.
The length of segment PQ is approximately 3.25 cm.
Topper's Solved these Questions
- BOOK - NCERT EXEMPLAR ENGLISHCHAPTER - CONSTRUCTIONSEXERCISE - Exercise 10.3 short Answer type Questions4videos
CONSTRUCTIONS
- BOOK - NCERT EXEMPLAR ENGLISHCHAPTER - CIRCLESEXERCISE - EXERCISE 9.4 LONG ANSWER TYPE QUESTIONS14videos
CIRCLES
- BOOK - NCERT EXEMPLAR ENGLISHCHAPTER - COORDINATE GEOMETRYEXERCISE - Exercise 7.4 Long Answer Type Questions6videos
COORDINATE GEOMETRY
Similar Questions
Construct a ΔABC in which ∠A=60∘ AB=5cm and AC - BC = 1.5 cm.
View SolutionConstruct a ΔABC in which ∠A=30∘ and AB = 4.5 cm and AC - BC = 1.2 cm.
View SolutionIn the given figure, ∠B=60∘,AB=16 cm and BC=23 cm.
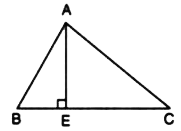
Calculate:
(ii) AC.View SolutionABCD is a rectangle measuring 60 cm by 50 cm P,Q,and R are the points on AB, AD , and BC, respectively, such that AP=40cm,AQ=20cm,andBR=40cm. Find the area of the polygon QPBCD.
View SolutionIn a triangle ABC , let P and Q be points on AB and AC respectively such that PQ∣∣BC . Prove that the median AD bisects PQ.
View SolutionIn ΔABC,∠B=90∘,AB=12cmandAC=15cm. D and E are points on AB and AC respectively such that ∠AED=90∘ and DE = 3 cm. Calculate the area of ΔABC and then the area of ΔADE.
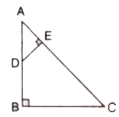 View Solution
View SolutionIn a ΔABC,AB=AC,PandQ are points on AC and AB respectively such that CB=BP=PQ=QA. if ∠AQP=θ, then tan2θ is a root of the equation
View SolutionConstruct a triangle ABC in which AC=5cm, BC= 7 cm and AB= 6cm.
Mark D, the mid-point of ABView SolutionConstruct a ΔABC in which BC = 4 cm, ∠B=60∘ and AB + AC = 6.5 cm.
View SolutionIf P and Q are points on the sides AB and AC respeactively of △ABC, if PQ||BC,l AP= 2 cm , AB = 6 cm and AC= 9 cm find AQ.
View Solution

