Two people standing on the same side of a tower in a straight line with it, measure the angles of elevation of the top of the tower as 25∘and50∘ respectively. If the height of the tower is 70 m, find the distance between the two people
The correct Answer is:91.38m
To solve the problem step by step, we will use trigonometric ratios to find the distances from each person to the base of the tower and then calculate the distance between the two people.
Step 1: Understand the Problem
We have a tower of height 70 m, and two people (A and B) are standing on the same side of the tower. The angles of elevation from person A and person B to the top of the tower are 25° and 50°, respectively. We need to find the distance between the two people.
Step 2: Draw the Diagram
1. Draw a vertical line representing the tower PQ, where PQ = 70 m.
2. Mark point A and point B on the ground, both on the same side of the tower.
3. Draw lines from A and B to the top of the tower P, forming angles of elevation of 25° and 50° respectively.
Step 3: Define Variables
- Let AQ = x (the distance from person A to the base of the tower).
- Let BQ = y (the distance from person B to the base of the tower).
Step 4: Use the Tangent Function for Person B
For person B, we can use the tangent function:
tan(50°)=PQBQ=70y
Rearranging gives:
y=70tan(50°)
Calculating tan(50°):
tan(50°)≈1.1917
Thus:
y=701.1917≈58.73 m
Step 5: Use the Tangent Function for Person A
For person A, we can use the tangent function:
tan(25°)=PQAQ=70x
Rearranging gives:
x=70tan(25°)
Calculating tan(25°):
tan(25°)≈0.4663
Thus:
x=700.4663≈150.11 m
Step 6: Calculate the Distance Between the Two People
The distance between the two people (AB) is given by:
AB=AQ−BQ=x−y
Substituting the values we found:
AB=150.11−58.73≈91.38 m
Final Answer
The distance between the two people is approximately 91.38 m.
---
We have a tower of height 70 m, and two people (A and B) are standing on the same side of the tower. The angles of elevation from person A and person B to the top of the tower are 25° and 50°, respectively. We need to find the distance between the two people.
1. Draw a vertical line representing the tower PQ, where PQ = 70 m.
2. Mark point A and point B on the ground, both on the same side of the tower.
3. Draw lines from A and B to the top of the tower P, forming angles of elevation of 25° and 50° respectively.
- Let AQ = x (the distance from person A to the base of the tower).
- Let BQ = y (the distance from person B to the base of the tower).
For person B, we can use the tangent function:
tan(50°)=PQBQ=70y
Rearranging gives:
y=70tan(50°)
Calculating tan(50°):
tan(50°)≈1.1917
Thus:
y=701.1917≈58.73 m
For person A, we can use the tangent function:
tan(25°)=PQAQ=70x
Rearranging gives:
x=70tan(25°)
Calculating tan(25°):
tan(25°)≈0.4663
Thus:
x=700.4663≈150.11 m
The distance between the two people (AB) is given by:
AB=AQ−BQ=x−y
Substituting the values we found:
AB=150.11−58.73≈91.38 m
The distance between the two people is approximately 91.38 m.
Topper's Solved these Questions
- HEIGHTS AND DISTANCES BOOK - ICSECHAPTER - HEIGHTS AND DISTANCES EXERCISE - Exercise 22 A
14 Videos
- HEIGHTS AND DISTANCES BOOK - ICSECHAPTER - HEIGHTS AND DISTANCES EXERCISE - Exercise 22 B
19 Videos
- GST [GOODS AND SERVICES TAX] BOOK - ICSECHAPTER - GST [GOODS AND SERVICES TAX] EXERCISE - Exercise 1(B)
16 Videos
- LINEAR INEQUATIONSBOOK - ICSECHAPTER - LINEAR INEQUATIONSEXERCISE - Competency Based Questions
15 Videos
Similar Questions
Two men are on the opposite sides of a tower. They measure the angles of elevation of the top of the tower as 45∘ and 30∘ respectively. If the height of the tower is 40 m, then the distance between the men is
View SolutionTwo points A and B are on the same side of a tower and in the same straight line with its base. The angles of depression of these points from the top of the tower are 60∘ and 45∘ respectively. If the height of the tower is 15 m, then find the distance between these points.
View SolutionTwo man are on the opposite sides of a tower. They measure the angles of elevation the top of the tower as 30∘ and 60∘. If the height of the tower is 150 m, find the distance between the two men.
View SolutionThe persons are standing on the opposite sides of a tower. They observe the angles of elevation of the top of the tower to be 30∘and38∘ respectively. Find the distance between them, if the height of the tower is 50 m
View SolutionIn figure, the angle of elevation of the top of a tower AC from a point B on the ground is 60∘. If the height of the tower is 20m , find the distance of the point from the foot of the tower.
View SolutionIn the figure-2, the angle of elevation of the top of tower AC from a point B on the ground is 60∘ . If the height of the tower is 20 m. find the distance of the point from the foot of the tower.
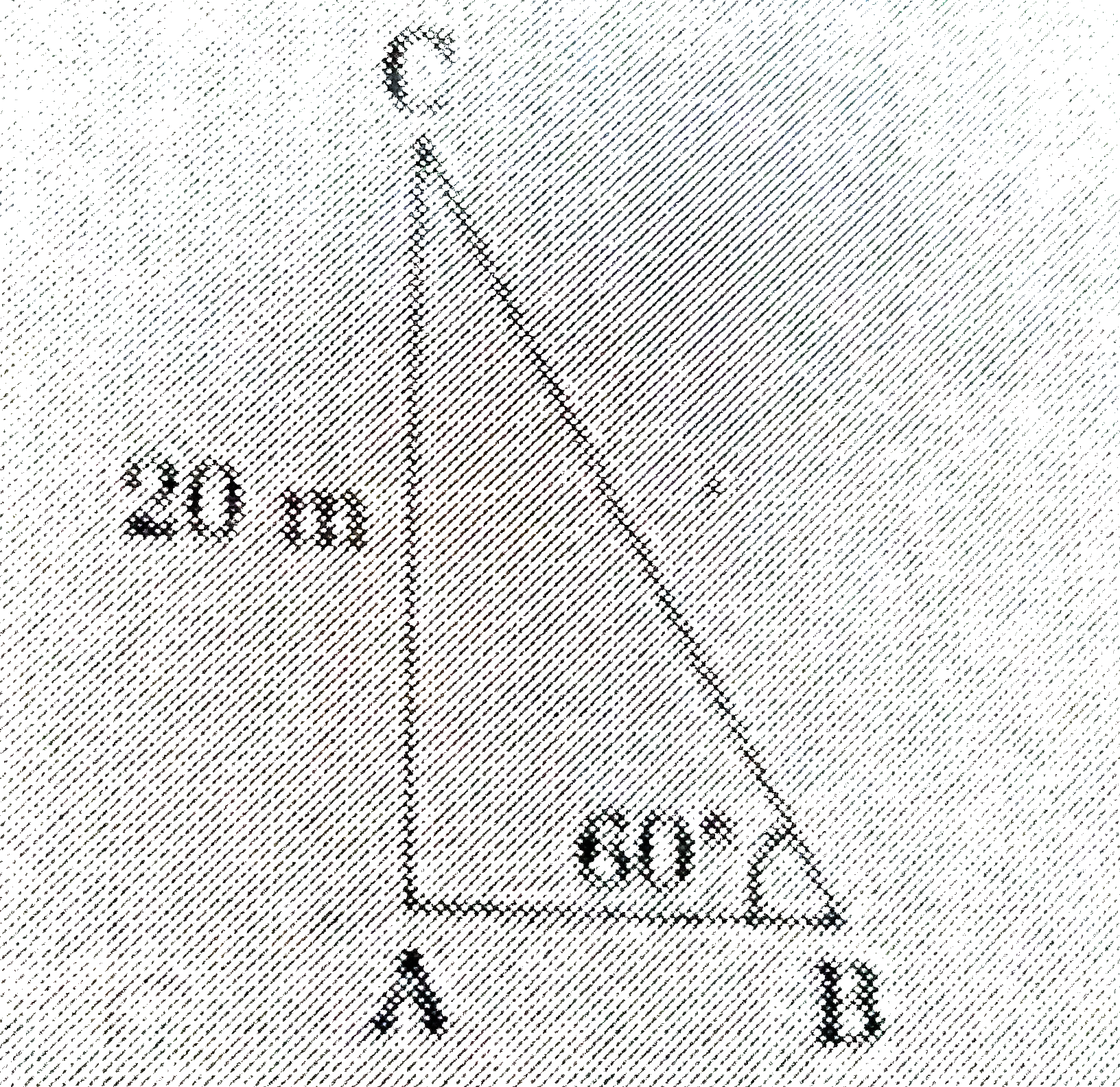
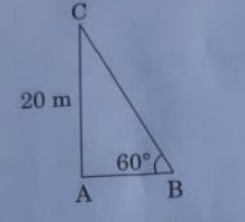
View SolutionIn figure, the angle of elevation of the top of a tower AC from a point B on the ground is 60∘. If the height of the tower is 20m , find the distance of the point from the foot of the tower.
View SolutionOn the same side of a tower, two objects are located. When observed from the top of the tower, their angles of depression are 45o and 60o . If the height of the tower is 150m, find the distance between the objects.
View SolutionAt one side of a road, there os a house and on the other side there is a tower. House and tower are on the same horizontal plane. The angle of depression of the top and foot of the house, from the top of the tower are 30∘ and 45∘ respectively. If the height of the house is 10 m, then find the distance between the house and the tower.
View SolutionPQ is a post of given height a , and AB is a tower at some distance. If α and β are the angles of elevation of B , the top of the tower, at P and Q respectively. Find the height of the tower and its distance from the post.
View Solution
