Question

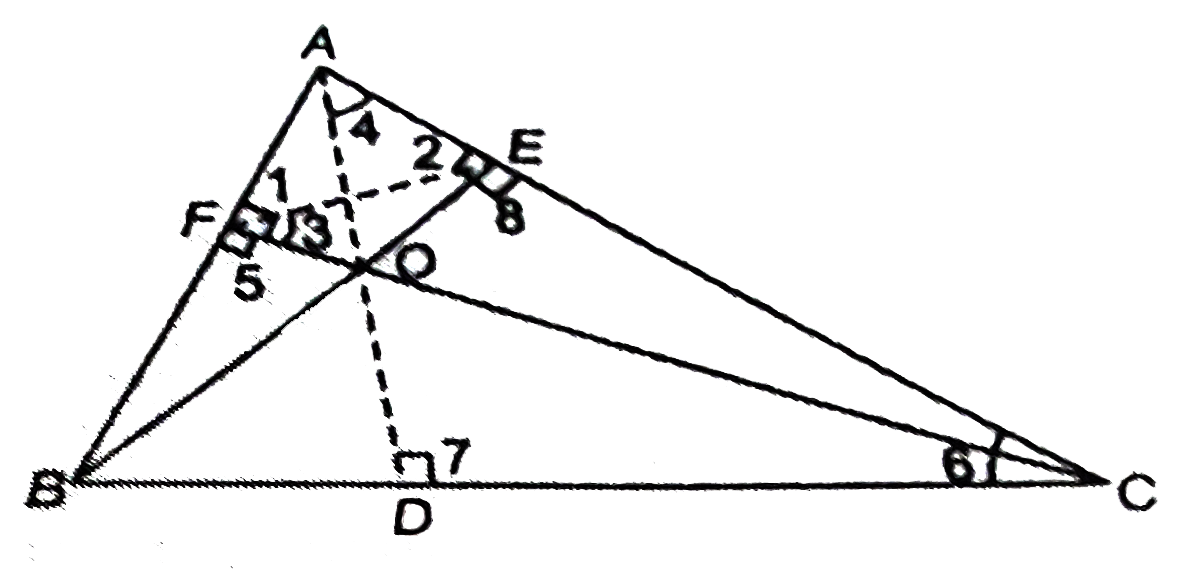
Using vector method, prove that the altitudes of a triangle are concurrent.

Explanation

Given : ABC be a triangle in which BE and CF are perpendiculars drawn from B and C to the opposite sides AC and AB respectively .
To Prove : All the perpendicualar are concurrent i.e., pass through the same point.
Construction : join AO and produce, where it meets BC at D.
Proof : we have taken BE and CF as perpendiculars intersecting each other at O. if we prove that `AOD bot BC`, it means all the three perpendicualars will pass through O.
Now, `angle1=angle2` (each `90^(@)`)
`thereforeangle1+angle2 =90^(@) +90^@=180^@`
`therefore square AEOF` is a cyclic quadrilateral.
`thereforeangle3=angle4` (angles of same segment are equal)....(1)
Now, since `anlge5=angle8` (each `90^@`)
But these are the angles subtended by line segmetn joining two points on the same side of the line.
So, B,F,E,C are concyclic.
i.e., square ` BFEC` is cyclic.
`therefore(angle5+angle3)+angle6=180^@` (sum of opposite angles of a cyclic qluadrilateral)
`rArr 90^@+angle4+angle 6=180^@` [form (1)]
`rArrangle4+angle6 =90^@`
Now, in `Delta ADC`,
`angle7+angle4 +angle6 =180^@`
`rArrangle7+90^@=180^@` (angle sum property)
`therefore angle 7=90^@`
It means line joining AO to D is perpendicular to BC.
Hence, all the perpendicular pass through the same point, i.e., they are concurrent.