Complete Solutions to EXAMPLE of chapter STATISTICS of Class 10 book with complete answers and questions
| Class 10 | MATHS |
| Chapter | STATISTICS |
| BEYOND PUBLICATION | EXAMPLE |
| Number Of Questions | 82 |
| Academic Year | 2023-24 |
The mean value can be calculated from both ungrouped and grouped date . Which one you think is more accurate ? Why ?
View SolutionWhen it is more conveninet to use grouped date for analysis ?
View SolutionIs the result obtained by all the three methods the same when finding the mean ?
View SolutionIf `x_(i)and f_(i)` are sufficiently samll , then which method is an appropriate choice ?
View SolutionIf `x_(i)and f_(i)` are numerically large numbers , then which methods are appropriate to use ?
View SolutionThe marks obtained in mathematics by 30 students of Class X of a certain school are given in table below. Find the mean of the marks obtained by the students.

View SolutionThe distribution below shows the number of wickets taken by bowlers in one day cricket matches. Find the mean number of wickets by choosing a suitable method. What does the mean signify?

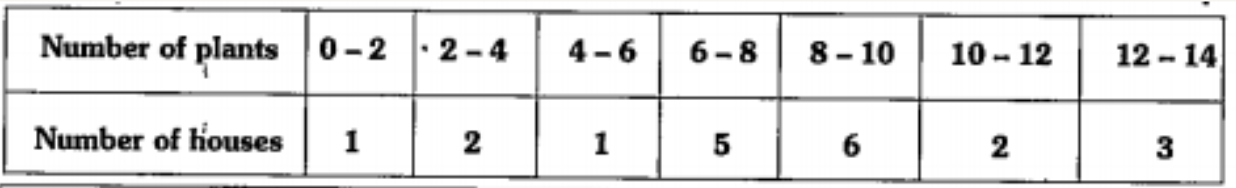
View SolutionA survey was conducted by a group of students as a part of their environmental awareness programme, in which they collected the following data regarding the number of plants In 20 houses in a locality. Find the mean number of plants per house.
View SolutionConsider the following distribution of daily wages of 50 workers of a factory.

find the mean daily wages of the workers of the factory by using an appropriate method.
View SolutionThe table below shows the daily expenditure on food of 25 households in a locality.

Find the mean daily expenditure on food by a suitable method.
View SolutionTo find out the concentratin of `SO_2` in the air (in parts per million, i.e., ppm), the data was collect for 30 localities in a certain city and is presented below.

Find the mean concentration of `SO_2` in the air.
View SolutionA class teacher has the following attendance record of 40 students of a class for the whole term. Find the mean number of days a student was present out of 56 days in the term.

View SolutionThe following table gives the literacy rate(in percentage) of 35 cities. Find the mean literacy rate.

View SolutionFind the mode of the following data. 5, 6, 9, 10, 6, 12, 3, 6, 11, 10, 4, 6, 7.
View SolutionFind the mode of the following data.
20,3,7,13,3,4,6,7,19,15,7,18,3
View SolutionFind the mode of the following data.
2 , 2, 2 , 3, 3, 3, 4, 4, 4 , 5 , 5, 5, 6, 6 , 6,.
View SolutionIs the mode always at the centre of the data ?
View SolutionDoes the mode change , if another observation is added to the data in Example ? Comment .
View SolutionIf the maximum value of an observation in the data in example 4 is changed to 8, would be the mode of the data be affected? Comment.
View SolutionIt depends upon the demand of the situation whether we are intersted in finding the acerage marks obtained by the students or the marks obtained by most of the students .
What do we find in the first situation ?
View SolutionIt depends upon the demand of the situation whether we are intersted in finding the acerage marks obtained by the students or the marks obtained by most of the students .
What do we find in the second situation ?
View SolutionIt depends upon the demand of the situation whether we are intersted in finding the acerage marks obtained by the students or the marks obtained by most of the students .
What do we find in the first situation ?
View SolutionThe wickets taken by a bowler in 10 cricket matches are as follows: 2 , 6 , 4 , 5 , 0 , 2 ,1, 3 ,2 ,3. Find the mode of the date .
View SolutionA survey conducted on 20 households in a locality by a group of students resulted in the following frequency table for the number of family members in a household.

Find the mode of this data.
View SolutionThe marks distribution of 30 students in a mathematics examination are given in the adjacent table. Find the mode of this data. Also, compare and interpret the mode and the mean.

View SolutionThe following table shows the ages of the patients admitted in a hospital during a year:

View SolutionThe following data gives the information on the observed life times (in hours) of 225 electrical components:

Determine the modal life times of the components.
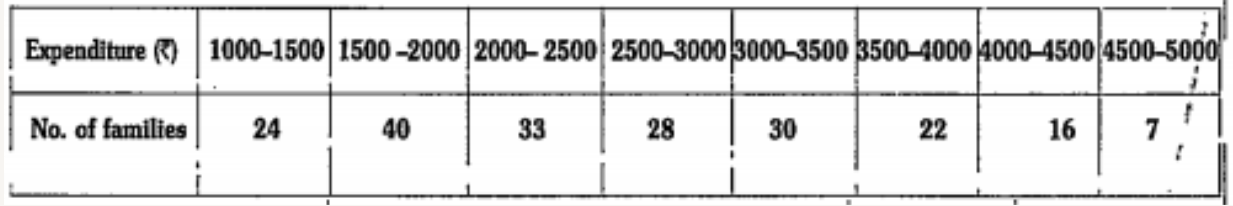
View SolutionThe followinig data gives the distribution of total monthly household expenditure of 200 families of Gummadidala village. Find the modal monthly expenditure of the families. Also fing the mean monthly expenditure.
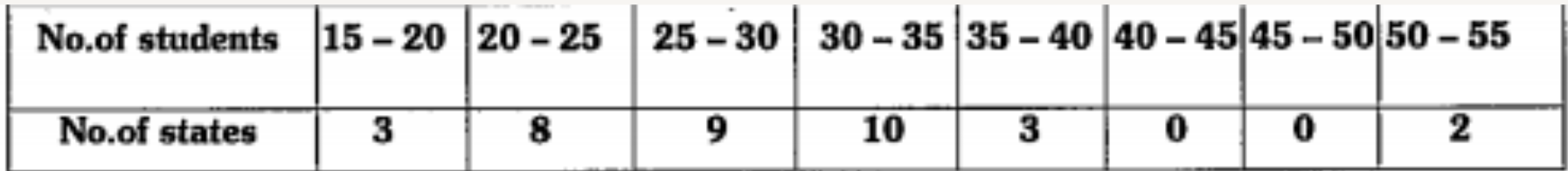
View SolutionThe following distribution gives the state-wise teachers, student ratio in higher secondary schools of India. Find the mode and mean of this data. Interpet the two measures.
View SolutionThe given distribution shows the number of runs scored by some top batsman of the world in one international cricket matches.

View SolutionA student noted the number of cars passing through a spot on a road for 100 periods, each of 3 minutes and summarised this ini the table given below.

View SolutionA survey regarding the heights (in cm) of 51 girls of Class X of a school was conducted and data was obtained as shown in table. Find their median

View SolutionThe median of the following data is 525. find the values of x and y, if the total frequency is 100. Here, Cl stands for class interval and Fr for frequency.

View SolutionIf the median of 60 observationss, give below is 28.5, find the value of x and y.

View SolutionA life insurance agent found the following data about distribution of ages of 100 policy holders. Calculate the median age.(policies are given only to perosns having age 18 years onwards but less than 60 years.)

we find the class intervals and thier corresponding frequencies to calculate the median age.
View SolutionThe lengths of 40 leaves of a plant are measured correct to the nearest millimetre and the data obtained is represented in the following data:

Find the median length of the leaves
The data needs to be converted to continuous classes for findinig the median, since the formula assumes continuous classes. The classes then change to 117.5-126.5, 126.5-135.5,...........171.5-180.5)
View SolutionThe following table gives the distribution of the life time of 400 neon lamps

Find the median life time of a lamp.
View Solution100 surnames were randomly picked up from a local telephone directory and the frequency distribution of the number of letters in the English alphabet in the surnames was obtained as follows:

Determine the median number of letters in the surnames. Find the mean number of letter in the surnames?Also, find the modal size of the surnames.
View SolutionThe distribution below gives the weights of 30 students of a class. Find the median weight of the students.

View SolutionThe Median of the data K/6, K/4, K, K/3, K/2 is 14. Then find the "K"value.
View SolutionIf the Mean and median of a data are 32.5 and 37.62 respectively. Find the mode of the data.
View SolutionFind the mean when the median is 72.8 mode is 65.
View SolutionFind the median of the data given below:
14, 18, 9, 21, 15, 20, 16, 6
View SolutionFind the Median for the following data 30, 17, 12, 21, 33, 22
Ascending order: 12, 17, 21, 22, 30, 33
View SolutionFind the mode of the following data.
20,3,7,13,3,4,6,7,19,15,7,18,3
View SolutionIf the median of 60 observations, given below is 28.5 find the value of x and y.

View SolutionThe mean of x+y observations is x-y find the sum of all the observations. Give mean of x + y observations is x - y.
we know x = `frac(Sigmaf_iu_i)(f_i)`
View SolutionFind the medain and mode of the following observation. 12,5,9,6,14,9 and 8.
View SolutionWrite the formula for calculating 'Arithmetic mean'. In step deivation method and explain each letter in it.
View SolutionFor the following data if the Median of 60 observation is 28.5 find the values of x and y.

View SolutionFind the mean of 5,6,9,10,6,12,3,6,11,10.
View SolutionWrite the formula for the median of a grouped data. Explain symbol with their used meaning.
View SolutionFind the median of 5, 3, 1, `-4`, 6, 7, 0.
View SolutionFind the mode of 5, 6, 9, 6, 12, 3, 6, 11, 6, 7.
View SolutionFind the mean of first ‘n’ natural numbers.
View SolutionConsider the following distribution of daily wages of 50 workers of a factory.

find the mean daily wages of the workers of the factory by using step deviation method.
View SolutionFind the mean daily wages of the workers of the factory for using an appropriate method In Rupees

View SolutionFind the Mode following data
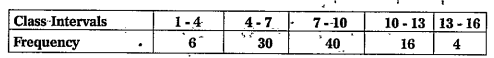
View SolutionFind the Mode of the following data
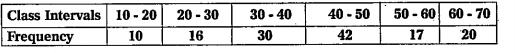
View SolutionFind the Mode of following data
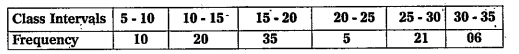
View SolutionFind the Mode of following data

View Solution100 surnames were randomly picked up from a local telephone directory and the frequency distribution of the number of letters in the English alphabet in the surnames was obtained as follows
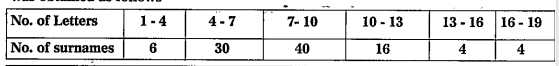
Determine Median number of letters in the surnames
View SolutionThe distribution below gives the weights of 40 students of a class. Find the median weight of the students.

View SolutionFind the median of the following data

View SolutionFind the median of following data

View SolutionFind the median of 5, 3, 1, `-4`, 6, 7, 0.
View SolutionFind the mean of 5,6,9,10,6,12,3,6,11,10.
View SolutionFind the mode of 5,6,5,11,12,12,5,5,1,0,2,3
View SolutionFind the mode of the following data

View SolutionFind the mode of following data
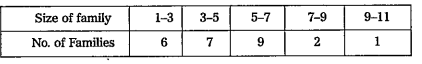
View SolutionFind the mean of following data

View SolutionFind the median of the following data
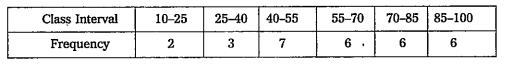
View SolutionFind the mode of the following data
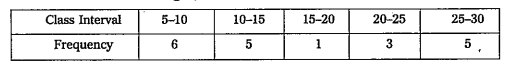
View SolutionFind the mean of the following data
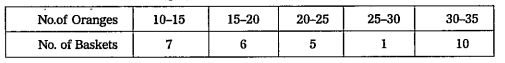
View SolutionFind the mean for the following data

View SolutionCalculate the mean for the following data

View SolutionFind the median of the following data.

View SolutionFind the mode of the following table

View SolutionFind the Median when mean 10.5 and mode 17.
View SolutionFind the mode of the following 20,21,15,18,21,17,21
View SolutionFind the median of the following 30,25,26,101,100,720,1000
View SolutionFind the median of the following 6,10,5,4,1,19
View Solution
EXAMPLE questions and complete solutions for chapter STATISTICS of BEYOND PUBLICATION of Class 10