Complete Solutions to ZEN ADDITIONAL QUESTIONS ( LONG ANSWER [LA] TYPE - QUESTIONS) of chapter CIRCLES of Class 10 book with complete answers and questions
| Class 10 | MATHS |
| Chapter | CIRCLES |
| ZEN PUBLICATION | ZEN ADDITIONAL QUESTIONS ( LONG ANSWER [LA] TYPE - QUESTIONS) |
| Number Of Questions | 8 |
| Academic Year | 2023-24 |
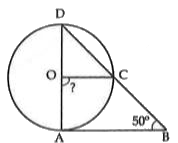
In the given figure, AD is a diameter of a circle with centre O and AB is a tangent at A. C is a point on the circle such that DC produced intersects the tangent at B and `|__ABD=50^(@)`. Find `|__COA`.
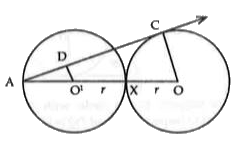
View SolutionIn the given figure, two equal circles with centres O and O' touch each other at X. OO' produced meets the circle with centre O' at A. AC is a tangent to the circle with centre O at the point C. O'D is perependicular to AC. Find the value of `(DO')/(CO)`.
View SolutionIn the given figure, AB is a chord of a circle with centre O such that `AB=16cm` and radius of the circle is 10 cm. Tangent at A and B intersect each other at P. Find PA.
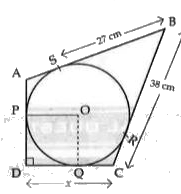
View SolutionIn the adjoining figure, quadrilateral ABCD is circumscribed. If the radius of the incircle with centre O is 10 cm and AD is perpendicular to DC, find x.
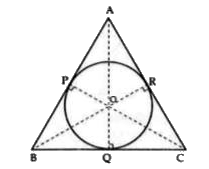
View SolutionIn the figure, the sides AB, BC and CA of triangle ABC touch a circle with centre O and radius r at P, Q , and R respectively. Prove that
(i) `AB+CQ=AC+BQ`
(ii) Area `(DeltaABC)=1/2 ("Perimeter of" DeltaABC)xx r`
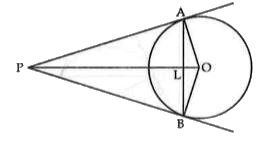
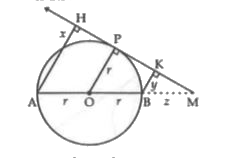
View SolutionAB is a diameter of circle. P is a point on the semicircle APB. AH and BK are perpendiculars from A and B respectively to the tangents at P. Prove that `AH+BK=AB`.
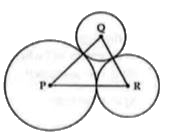
View SolutionPQR is a triangle with `PQ=10cm, QR=8cm` and `PR=11cm`. Three circles are drawn touching with each other such that the vertices as their centres. Find the radii of each circle.
View SolutionIf an isosceles `DeltaABC` in which `AB=AC=6cm` is inscribed in a circle of radius 9 cm, find the area of the triangle.
View Solution
ZEN ADDITIONAL QUESTIONS ( LONG ANSWER [LA] TYPE - QUESTIONS) questions and complete solutions for chapter CIRCLES of ZEN MATHS (KANNADA ENGLISH) of Class 10
All Exercises from ZEN PUBLICATION Class 10 MATHS CIRCLES
- ILLUSTRATIVE EXAMPLE
- TEXTUAL ( Exercise 4.1 )
- TEXTUAL ( Exercise 4.2 )
- ZEN ADDITIONAL QUESTIONS ( HOT [HIGHER ORDER THINKING SKILLS] - QUESTIONS)
- ZEN ADDITIONAL QUESTIONS ( HOT [HIGHER ORDER THINKING SKILLS] - QUESTIONS) (IIT AND IMO)
- ZEN ADDITIONAL QUESTIONS ( LONG ANSWER [LA] TYPE - QUESTIONS)
- ZEN ADDITIONAL QUESTIONS ( SHORT ANSWER [SA] TYPE - 2 - QUESTIONS)
- ZEN ADDITIONAL QUESTIONS ( SHORT ANSWER [SA] TYPE 1 - QUESTIONS)
- ZEN ADDITIONAL QUESTIONS (MCQs)
- ZEN ADDITIONAL QUESTIONS (VERY SHORT ANSWER [VSA] TYPE QUESTIONS)