Complete Solutions to Thermometry And Thermal Exapansion of chapter THERMAL PROPERTIES OF MATTER of Class 11 book with complete answers and questions
| Class 11 | PHYSICS |
| Chapter | THERMAL PROPERTIES OF MATTER |
| A2Z | Thermometry And Thermal Exapansion |
| Number Of Questions | 35 |
| Academic Year | 2023-24 |
When a copper ball is heated, the largest percentage increase will occur in its
View SolutionWhen a bimetallic strip is heated, it
View SolutionAt some temperature T, a bronze pin is a little large to fit into a hole drilled in a steel block. The change in temperature required for an exact fit is minimum when
View SolutionIf the length of a cylinder on heating increases by `2%`, the area of its base will increase by
View SolutionA solids floats submerged in a liquid. When the liquid is heated, which of the following is most likely to happen?
View SolutionA uniform metal rod is used as a bar pendulum. If the room temperature rises by `10^(@)C`, and the coefficient of linear expansion of the metal of the rod is `2 xx 10^(-6) per^(@)C`, the period of the pendulum will have percentage increase of
View SolutionIf on heating liquid through `80^(@)C`, the mass expelled is `(1/100)^(th)`of mass still remaining , the coefficient of apparent expansion of liquid is
View SolutionA glass flask of volume one liter at `0^(@)C` is filled, level full of mercury at this temperature. The flask and mercury are now heated to `100^(@)C`. How much mercury will spill out if coefficient of volume expansion of mercury is `1.82 xx 10^(-4)//^(@)C` and linear expansion of glass is `0.1 xx 10^(-4)//^(@)C` respectively?
View SolutionA steel scale measures the length of a copper wire as `80.0 cm` when both area at `20^(@)C` (the calibration temperature for scale). What would be the scale read for the length of the wire when both are at `40^(@)C`? (Given `alpha_("steel") = 11 xx 10^(-6) per ^(@)C` and `alpha_("copper") = 17 xx 10^(-6) per^(@)C`)
View SolutionA piece of metal weighs 46 g in air and 30 g in lipuid of density `1.24 xx 10^3 kg m^(-3)` kept at `27^0C`. When the temperature of the liquid is raised to `42^0C` the metal piece weights 30.5 g . The density of the liqued at `42^0 C` is `1.20 xx 10^3 kg m^(-3)`. Calculate the coefficient of linear expandsion of the metal.
View SolutionThe coefficient of linear expansion of crystal in one direction is `alpha_(1)` and that in every direction perpendicular to it is `alpha_(2)`. The coefficient of cubical expansion is
View SolutionOn an X temperature scale, water freezes at `-125.0^(@) X` and boils at `375.0^(@) X`. On a Y temperature scale, water freezes at `-70.0^(@)Y` and boils at `-30.0^(@)Y`. The value of temperature on X-scale equal to the temperature of `50.0^(@)Y` on Y-scale is
View SolutionAt what temperature the Fahrenheit and kelvin scales of temperature give the same reading ?
View SolutionIn figure which strip brass or steel have higher coefficient of linear expansion.
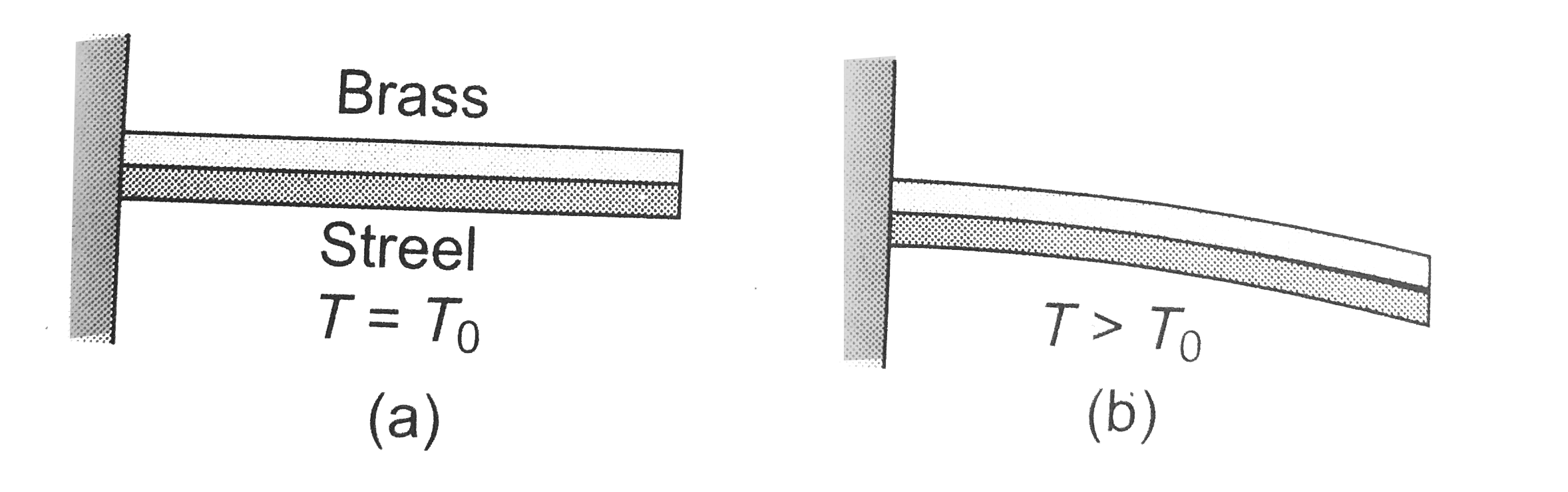
View SolutionThe co-efficient of thermal expansion of a rod is temperature dependent and is given by the formula `alpha = aT`, where `a` is a positive constant at T `"in"^(@)C`. if the length of the rod is l at temperature `0^(@)C`, then the temperature at which the length will be `2l` is
View SolutionThe length of two metallic rods at temperatures `theta` are `L_(A)` and `L_(B)` and their linear coefficient of expansion are `alpha_(A)` and `alpha_(B)` respectively. If the difference in their lengths is to remain constant at any temperature then
View SolutionA perpendicular clock (fitted with a small heavy bob that is connected with a metal rod) is `5` seconds fast each day at a temperature of `15^(@)C` and `10` seconds slow at a temperature of `30^(@)C`. The temperature at which it is designed to give correct time, is
View SolutionA `30.0 cm` long metal rod expands by `0.0650 cm` when its temperature is raised from `0^(@)C` to `100^(@)C`. A second rod of different metal and of the same length expands by `0.0350 cm` for the same rise in temperature. A third composite rod, also `30.0 cm` long, is made up of pieces of each of the above metals placed end to end and expands by `0.0580 cm` when temperature is increased from `0^(@)C` to `100^(@)C`. the length of the longer portion of the composite bar in cm at `0^(@)C` is
View SolutionA steel rod of length `1 m` is heated from `25^@ "to" 75^@ C` keeping its length constant. The longitudinal strain developed in the rod is ( Given, coefficient of linear expansion of steel = `12 xx 10^-6//^@ C`).
View SolutionIn the shown planar frame made of thin uniform rods, the length of section `AB` and `EF` is `l_(1)` and its thermal linear coefficient of expansion is `alpha_(1)`. The length of section `CD` is `l_(2)` and its thermal linear coefficient of expansion is `alpha_(2)`.`CB and DE` are of same length having thermal linear coefficient of expansion `alpha_(2)`. point `A, B, E and F` reside on same line, that is, sections `AB and EF` overlap. then the ratio of `(l_(1))/(l_(2)` for which the distance between end `A` and end `F` remains the same at all temperatures, is

View SolutionA brass rod of length `50 cm` and diameter `3.0 cm` is joined to a steel rod of the same length and diameter. What is the change in length of the combined rod at `250^(@)C`, if the original length are at `40.0^(@)C`?
(Coefficient of linear expansion of brass `=2.0 xx 10^(-5)//^(@)C, steel = 1.2 xx 10^(-5)//^(@)C`
View SolutionAn isosceles triangle is formed with a thin rod of length `l_(1)` and coefficient of linear expansion `alpha_(1)`, as the base and two thin rods each of length `l_(2)` and coefficient of linear expansion `alpha_(2)` as the two sides. The distance between the apex and the midpoint of the base remain unchanged as the temperature is varied. the ratio of lengths `(l_(1))/(l_(2))` is

View SolutionA copper and a tungsten plate having a thickness `d` each are riveted together so that at `0^(@)C` they form a flat bimetallic plate. Find the average radius of the curvature of this plate at temperature T. the coefficient of linear expansion for copper and tungsten are `alpha_(Cu) and alpha_(W)`.
View SolutionA brass wire `1.8 m` long at `27^(@)C` is held taut with little tension between two rigid supports. If the wire cooled to a temperature of `-39^(@)C`, what is the tension developed in the wire, if its diameter is `2.0 mm`? Coefficient of linear expansion of brass `= 2.0 xx 10^(-5)//^(@)C`, Young's modulus of brass `= 0.91 xx 10^(11) Pa`.
View SolutionA steel tape `1 m` long is correctly calibrated for a temperature of `27.0^(@)C`. The length of a steel rod measured by this tape is found to be `63.0 cm` on a hot day when the temperature is `45^(@)C`. Coefficient of linear expansion of steel `= 1.20 xx 10^(-5) //K`. what is the actual length of the steel rod on that day?
View SolutionA steel scale measures the length of a copper wire as `80.0 cm` when both area at `20^(@)C` (the calibration temperature for scale). What would be the scale read for the length of the wire when both are at `40^(@)C`? (Given `alpha_("steel") = 11 xx 10^(-6) per ^(@)C` and `alpha_("copper") = 17 xx 10^(-6) per^(@)C`)
View SolutionThe coefficient of volume expansion of glycerine is `49 xx 10^(-5)//^(@)C`. What is the fractional change in its density (approx.) for `30^(@)C` rise in temperature?
View SolutionA body is float inside liquid. If we increase temperature then what changes occur in buyancy force? (Assume body is always in floating condition)
View SolutionA solid sphere of iron at `2^(@)C` is lying at the bottom of a bucket full of water at `2^(@)C`. If the temperature of the water is increased to `3^(@)C`, the buoyant force on the sphere due to water will
View SolutionA thin rod of negligible mass and a cross-section of `2 xx 10^(-6) m^(2)` suspended vertically from one end, has a length of `0.5 m` at `200^(@)C`. The rod is cooled at `0^(@)C`, but prevented from contracting by attaching a mass at the lower end. The value of this mass is : (Young's modulus `=10^(11) N//m^(2)`, Coefficient of linear expansion `10^(-5) K^(-1) and g = 10 m//s^(2)`):
View SolutionA rod of length `1000 ` `mm` and co-efficient of linear expansion `alpha = 10^(-4)` per degree celsius is placed in horizontal smooth surface symmetrically between fixed walls separated by `1001` ` mm `. The young's modulus of rod is `10^(11)N//m^(2)`. If the temperature is increased by `20^@C`, then the stress developed in the rod is (in ` N//m^(2)`)
View SolutionA steel rod of length `1 m` is heated from `25^@ "to" 75^@ C` keeping its length constant. The longitudinal strain developed in the rod is ( Given, coefficient of linear expansion of steel = `12 xx 10^-6//^@ C`).
View SolutionCalculate the stress developed inside a tooth cavity filled with copper when hot tea at temperature of `57^(@)C` is drunk. You can take body (tooth) temperature to be `37^(@)C` and `alpha_(Cu) = 1.7 xx 10^(-5)//^(@)C` bulk modulus for copper `B_(Cu) = 140 xx 10^(9) N//m^(2)`.
View SolutionThermal coefficient of volume expansion at constant pressure for an ideal gas sample of n moles having pressure `P_(0)`, volume `V_(0)`, and temperature `T_(0)` is
View SolutionA uniform pressure P is exerted by an external agent on all sides of a solid cube at temperature `t^(@)C`. By what amount should the temperature of the cube be raised in order to bring its volume back to its original volume before the pressure was applied if the bulk modulus is B and co-efficient of volumetric expansion is `gamma`?
View Solution
Thermometry And Thermal Exapansion questions and complete solutions for chapter THERMAL PROPERTIES OF MATTER of A2Z PHYSICS (HINGLISH) of Class 11