Complete Solutions to Level 2 of chapter ELASTICITY of Class 11 book with complete answers and questions
| Class 11 | PHYSICS |
| Chapter | ELASTICITY |
| ARIHANT MASTER PROBLEM | Level 2 |
| Number Of Questions | 8 |
| Academic Year | 2023-24 |
A thin ring of radius R is made of a wire of density `rho` and Young’s modulus Y. It is spun in its own plane, about an axis through its centre, with angular velocity w. Determine the amount (assumed small) by which its circumference increases.
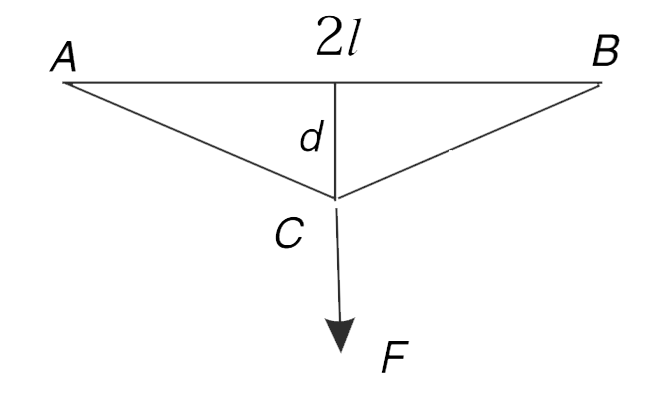
02:54View SolutionA steel wire of radius r is stretched without tension along a straight line with its ends fixed at A and B (figure). The wire is pulled into the shape ACB. Assume that d is very small compared to length of the wire. Young’s modulus of steel is Y.
(a) What is the tension (T) in the wire?
(b) Determine the pulling force F. Is F larger than T?
 04:03View Solution
04:03View SolutionA uniform material rod of length L is rotated in a horizontal plane about a vertical axis through one of its ends. The angular speed of rotation is w. Find increase in length of the rod. It is given that density and Young’s modulus of the rod are r and Y respectively.
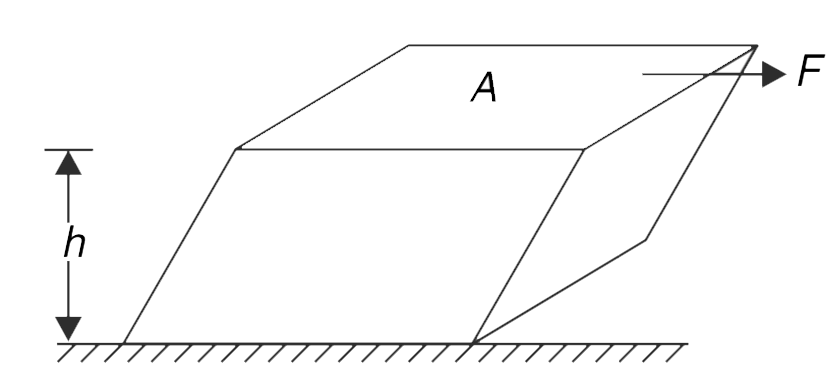
03:47View SolutionA rectangular bar is fixed to a hard floor. Height of the bar is h and its area in contact with the floor is A. A shearing force distorts the bar as shown. Prove that the work done by the shearing force is `W = ((sigma^(2))/(2 eta)) xx` volume of the bar. Here `sigma` is shear modulus of elasticity. Assume the deformation to be small.
 02:15View Solution
02:15View SolutionA thin uniform rod of mass M and length L is free to rotate in vertical plane about a horizontal axis passing through one of its ends. The rod is released from horizontal position shown in the figure. Calculate the shear stress developed at the centre of the rod immediately after it is released. Cross sectional area of the rod is A. [For calculation of moment of inertia you can treat it to very thin]
 02:56View Solution
02:56View SolutionA rigid cylindrical container has inner radius r. A cork having radius `r + Delta r` and length L is to be fitted so as to close the container. Uniform pressure `(Delta P)` is needed on the curved cylindrical surface of the cork. Poisson’s ratio of a cork is almost zero, and its bulk modulus is B.
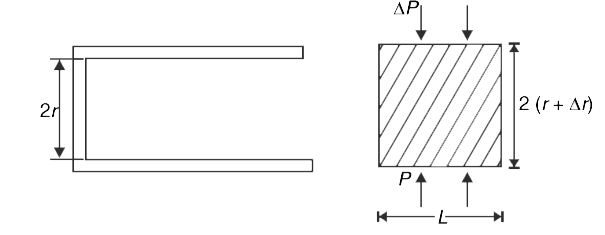
(a) Calculate `Delta P`
(b) After the cork is fitted how much force will be needed to pull it out of the container? Coefficient of friction between the container and the cork is `mu`.03:00View SolutionAssume that the least load which would break a thread when simply suspended from it is M and that this load produces a strain of 1 percent at the moment of breaking. Also assume that Hooke's law applies to the thread right up to breakingpoint. A load of mass m is suspended from a thread of length `lambda`. It is raised to a height and released. Find the least height to which the load must be raised so that it will will break the thread when allowed to fall.
02:36View SolutionAtmospheric pressure is `P_(0)` and density of water at the sea level is `rho_(0)`. If the bulk modulus of water is B, calculate the pressure deep inside the sea at a depth h below the surface.
View Solution
Level 2 questions and complete solutions for chapter ELASTICITY of ARIHANT PHYSICS (HINGLISH) of Class 11
