Complete Solutions to Solved Example of chapter RELATIVE MOTION of Class 11 book with complete answers and questions
| Class 11 | PHYSICS |
| Chapter | RELATIVE MOTION |
| CP SINGH | Solved Example |
| Number Of Questions | 27 |
| Academic Year | 2023-24 |
The rain is falling vertically downward with velocity `6m//s` and a man is moving horizontally with velocity `8m//s`. Find the velocity of rain with respect to the man.
03:39View SolutionFrom a light-house an observer two ships `A` and `B`. Ship `A` proceeding towards north at a speed `20sqrt(2) km//h` and ship `B` proceeding towards north-east at a speed of `20 km//h`. Find in which direction and at what speed the ship `B` would appear to move to an observer standing on the deck of the ship `A`.
06:14View SolutionA man is walking due west with a velocity `3 km//h` and rain appears to be falling vertically with a velocity `4km//h`. Find the velocity of rain with respect to the ground.
03:18View SolutionA person travelling east wards at the rate of ` 4 km h^(-1)` finds that the wind seems to blow directly from the north . On doubling its speed, the wind appears to come from ` 45^@` north of east. Find the actual velocity of the wind.
04:14View SolutionA man running on a horizontal road at `6 km//h` finds the rain falling vertically. He doubles his speed and find that the raindrops make an angle `37^(@)` with the vertical. Find the velocity of rain with respect to the ground.
06:46View SolutionTwo cars `A` and `B` are running with same speed `v`. A along east and `B` towards north. Car `A` is at origin `O` and `B` is at distance `d` as shown. After how much time, the distance between cars is minimum and what is the minimum distance?
05:10View SolutionTwo straight roads meet an angle of `60^(@)`. Initially one man is at a distance of `10 m` on one road from the crossing and the other at a distance of `20 m` on the other road from the crossing. They starts moving at the same instant with the same speed towards the crossing. find the minimum distance between them.
07:07View SolutionTwo particles, 1 and 2, move with constant velocities `v_1` and `v_2` along two mutually perpendicular straight lines toward the intersection point O. At the moment `t=0` the particles were located at the distances `l_1` and `l_2` from the point O. How soon will the distance between the particles become the smallest? What is it equal to?
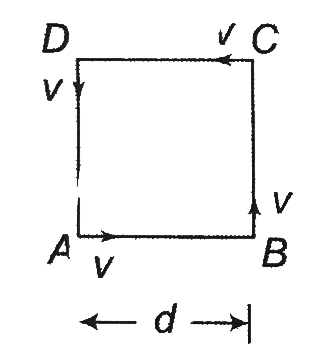
10:37View SolutionThree particles A, B and C are situated at the vertices of an equilateral triangle ABC of side d at time `t=0.` Each of the particles moves with constant speed v. A always has its velocity along AB, B along BC and C along CA. At what time will the particles meet each other?
05:17View SolutionRepeat the previous problem in the following cases:
 View Solution
View SolutionA motorboat coverse a distance between two points in `4h` along the flow and in `8h` opposite to the flow. In how much time, distance can be covered in still water?
03:37View SolutionA motorboat going downstream overcome a raft at a point A, `tau=60min` later it turned back and after some time passed the raft at a distance `l=6.0km` from the point A. Find the flow velocity assuming the duty of the engine to be constant.
06:45View SolutionA man can swim in still water with speed `v`. He wants to cross a river of width `d` that flows with speed `u` and reaches the point directely opposite to his starting point. (a) In which direction should he try to swim (i.e., find the angle the makes with the river flow)? (b) much time will he take to cross the river ?
03:30View SolutionIn the previous problem, the man wants to cross the river in the minimum time. In which directions, he should move? Find the minimum time to cross the river and his displacement (drift) along the flow.
04:19View SolutionIf `v=u/2`, in which direction the man should swim so that his drift along the flow is minimum and find value of the minimum drift.
05:04View SolutionTwo boats, `A` and `B`, move away from a point `P` at the middle of a river along the mutually perpendicular straight lines. Boat `A` moves along the river and boat `B` across the river. Having moved off equal distance from point `P` the boats returned. find the ratio of times of motion of boats `(t_(A))/(t_(B))` if `v=etau(etagt1)`. (`v` : velocity of boat with respect to water. `u`: stream velocity)
05:35View SolutionTwo swimmers leave point `A` on one bank of the river to reach point `B` lying right across on the other bank. One of them crosses the river along the straight line `AB` while the other swims at right angles to the stream and them walks the distance that he has been carried by the stream to get to point `B`. what was the velocity `v_(0)` of his walking if both swimmers reach the destination simultaneously? The stream velocity is `u` and `v` is the velocity of swimmer in still water.
05:24View SolutionA man can swim at a speed of `5km//h` in the still water. He wants to cross a `1600m` wide river flowing at `4 km//h`. He keeps himself at an angle `127^(@)` with the river flow while swimming. (a) Find the time he takes to cross the river. (b) Find the distance traveled by him along the flow.
04:43View SolutionAn aeroplane has to go from a point `O` to another point `A`, at distance `d` due `37^(@)` east of north. A wind is blowing due north at a speed of `20 m//s`. The air speed of the plane is `v`. (a) Find the direction in whihc the pilot should head the plane to reach the point `A`. (b) Find the time taken by the plane to go from `O` to `A`.
05:02View SolutionTwo particles start simulataneously from the same point and moves along two straight lines inclined at angle `alpha`, one with uniform velocity `v_(0)` and other from the rest with uniform acceleration, `a_(0)`. After how much time their relative velocity is minimum and what is it equal to?
06:22View SolutionTwo trains `A` and `B` of length `100m` and `200m`, respectively, are approaching each other on parallel tracks. If they take `15 s` to pass each other and velocity of `A` is three that of `B`, find their velocities.
04:20View SolutionIn how much time trains will pass each other if they are moving in the same direction.
03:18View SolutionA car travelling at `72 km//h` overtakes another car traveling at `54 km//h`. Assuming each car to be `5.0 m` long, find the time taken during overtake and the total road distance used for the overtake.
03:49View SolutionThe driver of a train moving at a speed ` v_(1)` sights another train at a disane ` d`, ahead of him moving in the same direction with a slower speed ` v_(2)`. He applies the brakes and gives a constant teradation ` a` to his train. Show that here will be no collision if ` d gt (v_(1) -v_(2))^(2) //2 a`.
04:15View SolutionTwo trucks are moving in a straight line towards each other at initial velocities `u_(1)` and `u_(2)` and with constant retardations `a_(1)` and `a_(2)`. If the initial separation between them is `d`, find the minimum value of `d` for no collision.
02:43View SolutionA car 2m long and 3m wide is moving at `10m//s` when a bullet hits it in a direction making an angle of `tan^(-1)(3//4)` with the car as seen from the ground. The bullet enters one edge of the car at the corner and passes out at diagonally opposite corner. Neglecting gravity, the time for the bullet to cross the car is
03:44View SolutionA gun is fired from a moving platform and ranges of the shot are observed to be `R_1 and R_2` when the platform is moving forwards and backwards, respectively, with velocity `v_P`. Find the elevation of the gun `prop` in terms of the given quantities.
06:37View Solution
Solved Example questions and complete solutions for chapter RELATIVE MOTION of CP SINGH PHYSICS (HINGLISH) of Class 11
