Complete Solutions to Example of chapter THERMOMETRY,THERMAL EXPANSION & KINETIC THEORY OF GASES of Class 11 book with complete answers and questions
| Class 11 | PHYSICS |
| Chapter | THERMOMETRY,THERMAL EXPANSION & KINETIC THEORY OF GASES |
| DC PANDEY | Example |
| Number Of Questions | 23 |
| Academic Year | 2023-24 |
Express a temperature of `60^@ F` in degrees Celsius and in kelvin.
View SolutionThe temperature of an iron piece is heated from `30^@ C "to" 90^@ C`. What is the change in its temperature on the fahrenheit scale and on the kelvin scale?
View SolutionA steel ruler exactly `20 cm` long is graduated to give correct measurements at `20^@C`.
(a) Will it give readings that are too long or too short at lower temperatures?
(b) What will be that actual length of the ruler when it is used in the desert at a temperature of `40^@ C` ? `alpha_(steel = 1.2 xx 10^-5(.^@ C)^-1` .
View SolutionThe scale on a steel meter stick is calibrated at `15^@ C`. What is the error in the reading of `60 cm` at `27^@C` ? `alpha_(steel) =1.2 xx 10^-5 (.^@ C)^-1`.
View SolutionA second's pendulum clock has a steel wire. The clock is calibrated at `20^@ C`. How much time does the clock lose or gain in one week when the temperature is increased to `30^@ C` ? `alpha_(steel = 1.2 xx 10^-5(^@ C)^-1`.
View SolutionA sphete of deamrter 7.0 cm and mass 266.5 g float in a bath of liquid. As the temperature is raised, the sphere begins to sink at a temperature of `35^@ C`. If the density of liqued is `1.527 g cm^(-3)` at` 0^@C`, find the coeffiecient of cubical expamsion of the liquid. Neglect the expansion of the sphere.
View SolutionA glass beaker holds exactly `1 L "at" 0^@ C`
(a) What is its volume at `50^@ C` ?
(b) If the beaker is filled with mercury at `0^@ C`, what volume of mercury overflows ehen the temperature is `50^@ C` ? `alpha _ g = 8.3 xx 10^-6 per ^@ C` and` gamma_Hg = 1.82 xx 10^-4 per ^@ C`.
View Solution`p - V` diagram of same mass of a gas are drawn at two different temperatures `(T_1)` and `(T_2)`. Explain whether `T_1 gt T_2 or T_2 gt T_1`.
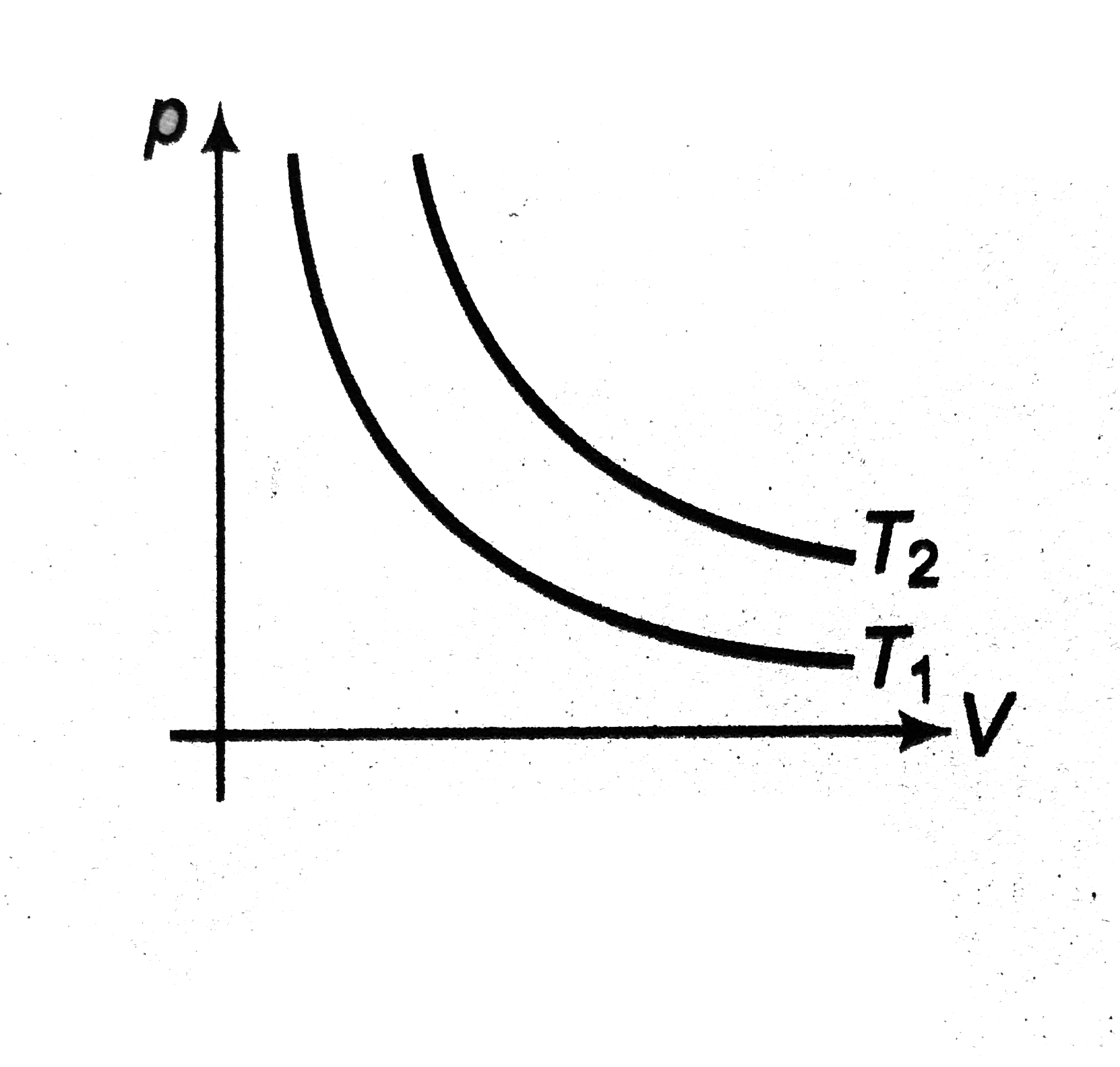 .
.
View SolutionThe `p -V` diagram of two different masses `m_(1)` and `m_(2)` are drawn (as shown) at constant temperature `(T)`. State whether `m_(1) gt m_(2) "or" m_(2) gt m_(2)`.
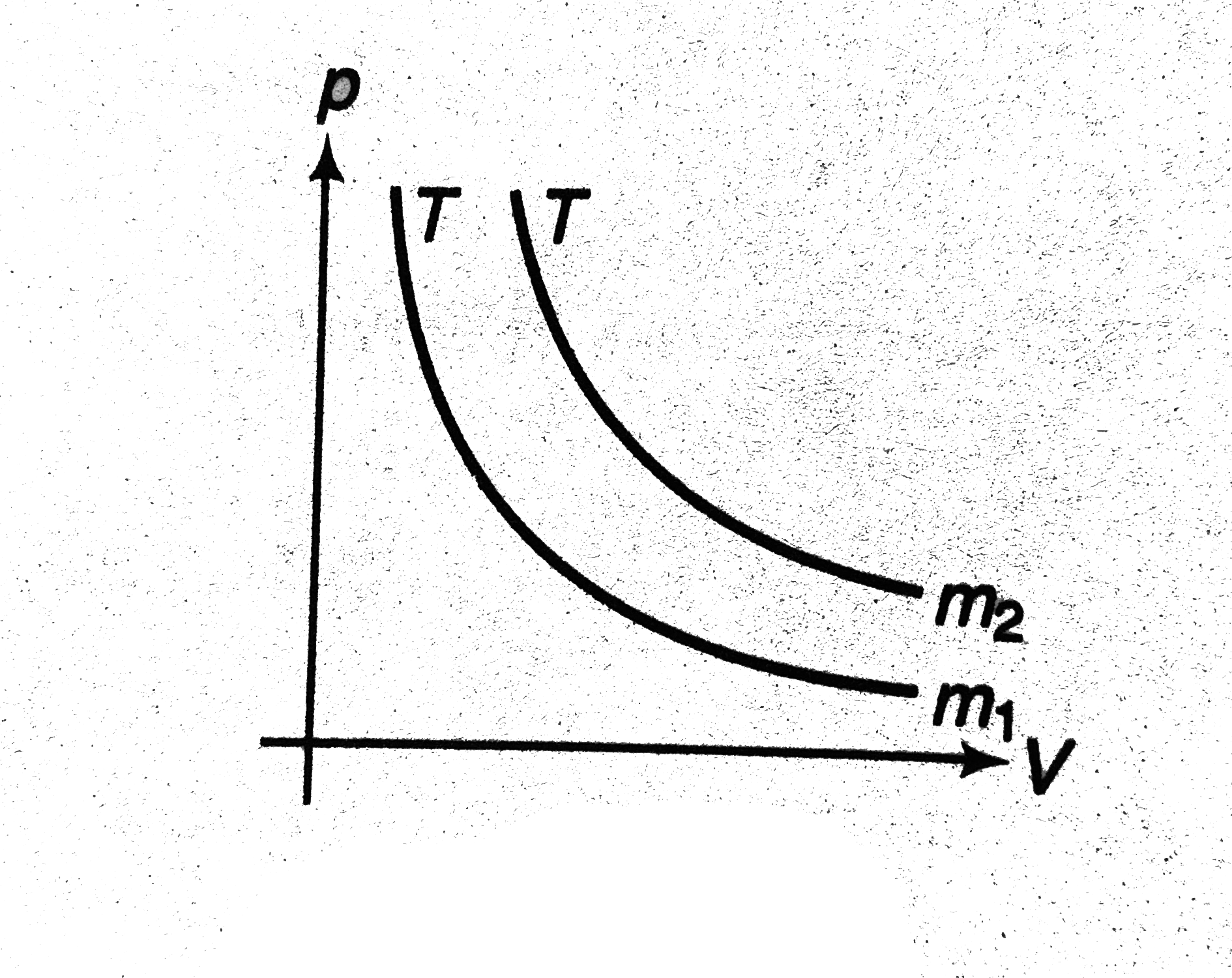 .
.
View SolutionThe p-T graph for the given mass of an ideal gas is shown in figure. What inference can be drawn regarding the change in volume (whether it is constant, increasing or decreasing) ?
How to proceed Definitely, it is not constant. Because when volume of the gas is constant `p-T` graph is a straight line passing through origin. The given line does not pass through origin, hence volume is not constant. .
.
`V = (nR)((T)/P)`
Now, to see volume of the gas we will have to see whether `(T)/(P)` is increasing or decreasing.
View SolutionA gas at `27^@ C` in a cylinder has a volume of 4 litre and pressure `100 Nm^-2`.
(i) Gas is first compressed at constant temperature so that the pressure is `150 Nm^-2` . Calaulate the change in volume.
(ii) It is then heated at constant volume so that temperature becomes `127^@ C`. Calculate the new pressure.
View SolutionA balloon partially filled with helium has a volume of `30 m^3`, at the earth's surface, where pressure is `76 cm` of (Hg) and temperature is `27^@ C` What will be the increase in volume of gas if balloon rises to a height, where pressure is `7.6 cm` of `Hg` and temperature is `-54^@ C` ?
View SolutionFind total internal energy of 3 moles of hydrogen gas at temperature `T`.
View SolutionTen moles of `(O_2)` gas are kept at temperature `T`. At some higher temperature `2T`, fourty percent of molecular oxygen breaks into atomic oxygen. Find change in internal energy of the gas.
View SolutionAt a given temperature internal energy of a monoatomic, diatomic and non - linear gas is `(U_(0)` each. Find their translational and rotiational kinetic energies separately.
View SolutionTwo moles of helium (He) are mixed with four moles of hydrogen `(H_2)`. Find
(a) `(C_(V)` of the mixture
(b) `(C_(P)` of the mixture and
( c) `(gamma) `of the mixture.
View SolutionTemperature of two moles of a monoatomic gas is increased by `300 K` in the process `p prop V`. Find
(a) molar heat capacity of the gas in the given process
(b) heat required in the given process.
View SolutionFind the rms speed of hydrogen molecules at room temperature `( = 300 K)`.
View SolutionA tank used for filling helium balloons has a volume of `0 .3 m^3` and contains (2.0) mol of helium gas at `20 .0^@ C`. Assuming that the helium behaves like an ideal gas.
(a) What is the total translational kinetic energy of the molecules of the gas ?
(b) What is the average kinetic energy per molecule ?
View SolutionConsider an `1100` particels gas system with speeds distribution as follows :
1000 particles each with speed `100 m//s`
2000 particles each wityh speed `200 m//s`
4000 particles each with speed `300 m//s`
3000 particles each with speed `400 m//s` and 1000 particles each with speed `500 m//s`
Find the average speed, and rms speed.
View SolutionCalculate the change in internal energy of `3 .0` mol of helium gas when its temperature is increased by `2. 0 K`.
View SolutionIn a crude model of a rotating diatomic molecule of chlorine `(Cl_2)`, the two `(C l)` atoms are `2.0 xx 10^-10 m` apart and rotate about their centre of mass withb angular speed `omega = 2.0 xx 10^12 "rad" //s`. What is the rotational kinetic energy of one molecule of `C l_(2)` , Which has a molar mass of `70.0 g // mol` ?
 .
.
View SolutionProve that the pressure of an ideal gas is numerically equal to two third of the mean translational kinetic energy per unit volume of the gas.
View Solution
Example questions and complete solutions for chapter THERMOMETRY,THERMAL EXPANSION & KINETIC THEORY OF GASES of DC PANDEY ENGLISH of Class 11