Complete Solutions to Level 1 Subjective of chapter THERMOMETRY,THERMAL EXPANSION & KINETIC THEORY OF GASES of Class 11 book with complete answers and questions
| Class 11 | PHYSICS |
| Chapter | THERMOMETRY,THERMAL EXPANSION & KINETIC THEORY OF GASES |
| DC PANDEY | Level 1 Subjective |
| Number Of Questions | 48 |
| Academic Year | 2023-24 |
Change each of the given temperature to the Celsius and Kelvin scales : `68^@ F, 5^@ F and 176 ^@ F`.
View SolutionChange each of the given temperature to the Fahrenheit and Reaumur scale : `30^@ C, 5^@ C and -20^@ C`.
View SolutionAt what temperature do the Celsius and Fahrenheit readings have the same numerical value ?
View SolutionYou work in a materials testing lab and your boss tells you to increase the temperature of a sample by `40.0^@ C`. The only thermometer you can find at your workbench reads in `^@ C`. If the initial temperature of the sample is `68.2^@ C`. What is its temperature in `^@ C` when the desired temperature increase has been achieved ?
View SolutionThe steam point and the ice point of a Mercury thermometer are marked as `80^0 and 20^0`. What will be the temperature in centigrade mercury scale when this thermometer reads `32^0`?
View SolutionA platinum resistance thermometer reads `0^@ C` when its resistance is `80 Omega and 100^@ C` when its resistance is `90 Omega`. Find the temperature at which the resistance is `86 Omega`.
View SolutionThe steam point and the ice point of a mercury thermometer are marked as `80^@ and 10^@`. At what temperature on centigrade scale the reading of this thermometer will be `59^@` ?
View SolutionFind the temperature at which oxygen molecules would have the same rms speed as of hydrogen molecules at `300 K`.
View SolutionFind the mass (in kilogram) of an ammonia molecule `NH_(3)`.
View SolutionThree moles of an ideal gas having `gamma = 1.67` are mixed with 2 moles of another ideal gas having `gamma = 1.4`. Find the equivalent value of `gamma` for the mixture.
View SolutionHow many degress of freedom have the gas molecules, if under standard conditions the gas density is `rho = 1.3 kg//m^3` and velocity of sound propagation o it is `v = 330m//s` ?
View Solution`4 g` hydrogen is mixed with 11.2 litre of He at (STP) in a container of volume 20 litre. If the final temperature is `300 K`, find the pressure.
View SolutionOne mole of an ideal monoatomic gas is taken at a temperature of `300 K`. Its volume is doubled keeping its pressure constant. Find the change in internal energy.
View SolutionTwo perfect monoatomic gases at absolute temperature `T_(1)` and `T_(2)` are mixed. There is no loss of energy. Find the temperature of the mixture if the number of moles in the gases are` n_(1)` and `n_(2)`.
View SolutionIf the water molecules in `1.0 g` of water were distributed uniformly over the surface of earth, how many such molecules would there be in `1.0 cm^2` of earth's surface ?
View SolutionIf the kinetic energy of the molecules in 5 litre of helium at 2 atm is `(E )`. What is the kinetic energy of molecules in 15 litre of oxygen at 3 atm in terms of `(E )` ?
View SolutionAt what temperature is the "effective" speed of gaseous hydrogen molecules (molecular weight = 2) equal to that of oxygen molecules (molecular weight = 32) at `47^@ C`?
View SolutionAt what temperature is `v_(rms)` of `H_(2)` molecules equal to the escape speed from earth's surface. What is the corresponding temperature for escape of hydrogen from moon's surface ? Given `g_m = 1.6 m//s^(2), R_e = 6367 km "and" R_m = 1750 km`.
View SolutionThe pressure of the gas in a constant volume gas thermometer is 80cm of mercury in melting ice at `1 atm`. When the bulb is placed in a liquid, the pressure becomes `160cm` of mercury. Find the temperature of the liquid.
View SolutionThe resistances of a platinum resistance thermometer at the ice point, the steam point and the boiling point of sulphur are `2.50, 3.50 and 6.50 (Omega)` respectively. Find the boiling point of sulphur on the platinum scale. The ice point and the steam point measure `0^@ and 100^@`, respectively.
View SolutionIn a constant volume gas thermometer, the pressure of the working gas is measured by the differenced in the levels of mercury in the two arms of a U-tube connected to the gas at one end. When the bulb is placed at the room temperature `27.0^0 C`, the mercury column in the arm open to atmosphere stands `5.00 cm` above the level of mercury in the other arm. When the bulb is placed in a hot liquid, the difference of mercury levels becomes `45..0 Cm`. Calculate the temperature of the liquid. (Atmospheric pressure `= 75.0cm` of mercury).
View SolutionA steel wire of `2.0 mm^2` cross- section is held straight(but under no tension) by attaching it firmly to two points a distance `1.50 m` apart at `30^@ C`. If the temperature now decreases to `-10^@ C` and if the two points remain fixed, what will be the tension in the wire ? For steel, `Y = 20.0000 MPa`.
View SolutionA metallic bob weights `50 g` in air. If it is immersed in a liquid at a temperature of `25^@ C`, it weights `45 g` . When the temperature of the liquid is raised to `100^@ C`, it weights `45.1 g`. Calculate the coefficient of cubical expansion of the liquid. Given that coefficient of cubical expansion of the metal is `12 xx 10^(-6) .^@ C^-1`.
View SolutionAn ideal gas exerts a pressure of `1.52 MP`a when its temperature is `298.15 K` and its volume is `10^-2m^3`. (a) How many moles of gas are there ? (b) What is the mass density if the gas is molecular hydrogen ? ( c) What is the mass density if the gas is oxygen ?
View SolutionA compressor pumps `70 L` of air into a `6 L` tank with the tempertaure remaining unchanged. If all the air is originally at `1 atm`. What is the fianl absolute pressure of the air in the tank ?
View SolutionA partially inflated balloon contains `500 m^3` of helium at `27^@ C` and `1 atm` pressure. What is the volume of the helium at an altitude of `18000 ft`, where the pressure is `0.5 atm` and the temperature is `-3^@ C` ?
View SolutionA cylinder whose inside diameter is `4.00 cm` contains air compressed by a piston of mass `m = 13.0 kg` which can slide freely in the cylinder. The entire arrangement is immersed in a water bath temperature can be controlled. The system is initially in equilibrium at temperature `t_i = 20^@ C`. The initial height of the piston above the bottom of the cylinder is `h_i = 4.00 cm`. The temperature of the water bath is gradually increased to afinal temperature `t_f = 100^@ C`. Calculate the final height `h_f` of the piston.
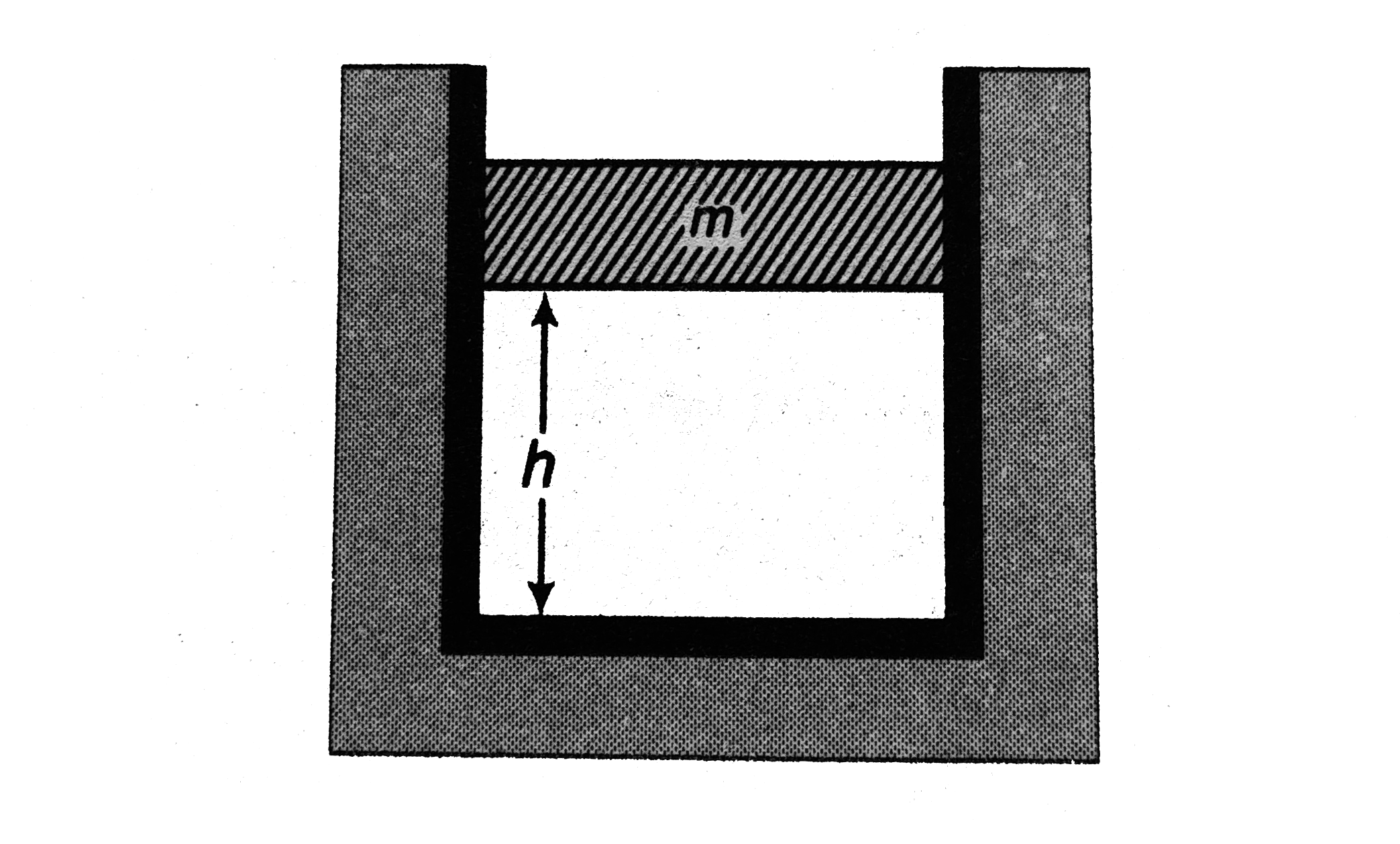 .
.
View SolutionThe closed cylinder shown in figure has a freely moving piston separating chambers 1 and 2. Chamber 1 contains `25 mg` of `N_2` and chamber 2 contains `40 mg` of helium gas. When equilibrium is established what will be the ratio `L_1// L_2` ? What is the ratio of the number of moles of `N_2` to the number of moles of He ?(Molecular weights of `N_2` and He are 28 and 4.
 .
.
View SolutionTwo gases occupy two containers (A) and (B). The gas in (A) of volume `0.11 m^3` experts a pressure of `1.38 Mpa`. The gas in (B) of volume `0.16 m^3` experts a pressure of `0.69 Mpa. Two containers are united by a tube of negligible volume and the gases are allowed to intermingle. What is the final pressure in the container if the temperature remains constant ?
View SolutionA glass bulb of volume `400 cm^(3)` is connected to another bulb of volume `200 cm^(3)` by means of a tube of negligible volume. The bulbs contain dry air and are both at a common temperature and pressure of `20^(@) C` and 1.000 atm, respectively. The larger bulb is immersed in steam at `100^(@) C` and the smaller in melting ice at `0^(@)`. Find the final common pressure.
View SolutionThe condition called standard temperature and pressure (STP) for a gas is defined as temperature of `0^@ C = 273.15 K` and a pressure of `1 atm = 1.013 xx 10^5 Pa`. If you want to keep a mole of an ideal gas in your room at (STP), how big a container do you need ?
View SolutionA large cylindrical tank contains `0.750 m^3` of nitrogen gas at `276@ C and 1.50 xx 10^5 Pa` (absolute pressure). The tank has a tightfitting piston that allows the volume to be changed. What will be the pressure if the volume is decreased to `0.480 m^3` and the temperature is increased to `157^@ C`.
View SolutionA vessel of volume 5 litres contains `1.4 g` of `N_2` and `0.4 g` of He at `1500 K`. If `30 %` of the nitrogen molecules are dissociated into atoms then find the gas pressure.
View SolutionTemperature of diatomic gas is `300 K`. If moment of intertia of its molecules is `8.28 xx 10^-38 g- cm^2`. Calculate their root mean square angular velocity.
View SolutionFind the number of degrees of freedom of molecules in a gas. Whose molar heat capacity
(a) at constant pressure `C_p = 29 J mol^-1 K^-1`
(b) `C = 29 J mol^-1 K^-1` in the process (pT) = constant.
View SolutionIn a certain `(2/5) th` of the energy of molecules is associated with the ratation of molecules and the rest of it is associated with the motion of the centre of mass.
(a) What is the average translational energy of one such molecule when the temperature id `27^@ C` ?
(b) How much energy must be supplied to one mole of thsi gas constant volume to raise the temperature by `1^@ C` ?
View SolutionA mixture contains `1` mole of helium `(C_p = 2.5 R, C_v= 1.5 R. )` and `1` mole of hydrogen `(C_p = 3.5 R, C_v = 2.5 R, )` . Calculate the values of `C_p , C_v and gamma` for the mixture.
View SolutionAn ideal gas `(C_p / C_v = gamma)` is taken through a process in which the pressure and volume vary as `(p = aV^(b))`. Find the value of b for which the specific heat capacity in the process is zero.
View SolutionAn ideal gas is taken through a process in which the pressure and the volume are changed according to the equation `p=kv`. Show that the molar heat capacity of the gas for the process is given by `(C=C_v + ( R)/(2))`.
View SolutionThe pressure of a gas in a `100 mL` container is `200 kPa` and the. Average translational kinetic energy of each gas particle is `6 xx 10^-21 J`. Find the number of gas particles in the container. How many moles are there in the container ?
View SolutionOne gram mole `NO_2 "at" 47^@ C` and 2 atm pressure in kept in a vessel. Assuming the molecules to be moving with (rms) velocity. Find the number of collisions per which the molecules make with one square metre area of the vessel wall.
View SolutionA `2.00 mL` volume container contains `50 mg` of gas at a pressure of `100 kPa`. The mass of each gas particle is `8.0 xx 10^-26 kg`. Find the average translational kinetic energy of each particle
View SolutionCall the (rms) speed of the molecules in an ideal gas `V_(0)` at temperature `T_(0)` and pressure `p_(0)`. Find the speed if (a) the temperature id raised from `T_0 = 293 K "to" 573 K` (b) the pressure is doubled and `T = T_0` ( c) the molecular weight of each of the gas molecules is tripled.
View Solution(a) What is the average translational kinetic energy of a molecule of an ideal gas at temperature of `276@ C` ?
(a) What is the total random translational kinetic energy of the molecules in one mole of this gas ?
( c) What is the rms speed of oxygen molecules at this temperature ?
View SolutionAt `0^@ C and 1.0 atm ( = 1.01 xx 10^5 N//m^2)` pressure the densities of air, oxygen and nitrogen are `1.284 kg//m^3, 1.429 kg//m^3 and 1.251 kg //m^3` respectively. Calculate the percentage of nitrogen in the air from these data, assuming only these two gases to be present.
View SolutionAn air bubble of `20 cm^3` volume is at the bottom of a lake `40 m` deep where the temperature is `4^@ C`. The bubble rises to the surface which is at a temperature of `20 ^@ C`. Take the temperature to be the same as that of the surrounding water and find its volume just before it reaches the surface.
View SolutionFor a certain gas the heat capcity at constant pressure is greater than that at constant volume by `29.1 J//K`.
(a) How many moles of the gas are there ?
(b) if the gas is monatomic, what are heat capacities at constant volume and pressure ?
( c) If the gas molecules are diatomic which rotate but do nit vibrate, what are heat capacities at constant volume and at constant pressure.
View SolutionThe heat capacity at constant volume of a monoatomic gas is `35 j//K`. Find
(a) the number of moles
(b) the internal energy at `0^@ C`.
(c ) the molar heat capacity at constant pressure.
View Solution
Level 1 Subjective questions and complete solutions for chapter THERMOMETRY,THERMAL EXPANSION & KINETIC THEORY OF GASES of DC PANDEY ENGLISH of Class 11