Complete Solutions to Subjective Type of chapter ATOMIC STRUCTURE of Class 11 book with complete answers and questions
| Class 11 | CHEMISTRY |
| Chapter | ATOMIC STRUCTURE |
| GRB PUBLICATION | Subjective Type |
| Number Of Questions | 55 |
| Academic Year | 2023-24 |
Assume that `10^(-17)`J of light energy is needed by the interior of the human eye to see an object . How many photons of green light `(lambda = 495nm)` are needed to generate this minimum energy .
`[h=6.6xx10^(-34) Js]`
View SolutionAverage life time of a hydrogen atom excited to `n=2 ` state is `10^(-8) s.` Find the number of revolutions made by the electron on an average before it jumps to the ground state. If your answer in scientific notation is `x xx 10^(y), ` then find the value of y.
View SolutionCalculate ratio of wavelength for a proton and `alpha-`particle . If their KE are same.
View SolutionWith what velocity should an alpha `(alpha)`- particle travel towards the nucleus of a copper atom arrive at a distance of `10^(-13)m` from the nucleus of the copper atom ?
View SolutionFor a broadcasted electromagnetic wave having frequency of 1200 kHz, calculate number of waves that will be formed in 1 km distance (wave number per km).
View SolutionVisible spectrum contains light of following colours ''Violet - Indigo - Blue - Green - Yellow - Orange - Red'' (VIBGYOR).
Its frequency ranges from violet `(7.5xx10^(14) Hz)` to re `(4xx10^(14) Hz.)` Find out the maximum wavelength `("in" "Å")` in this range.
View SolutionFor a wave frequency is 10 Hz and wavelength is 2.5 m. How much distance (in metres) it will travel in 40 seconds?
View SolutionCalculate minimum value of uncertainity in position of a particle whose de broglie wavelength is `sqrtpi "Å"` and uncertainity in de broglie wavelength is 0.05"Å". Express your asnwer in Angstrom ("Å").
View SolutionIn a sample of H-atoms in ground state electrons make transition from ground state to a particular excited state where path length is 5 times de Broglie wavelength , electrons make back transition to the ground state producing all possible photons. If photon having 2nd highest energy of this sample can be used to excite the electron in a particular excited state of `Li^(2+)` ion then find the final excited state of `Li^(2+)` ion .
View SolutionA particular transition in hydrogen atom from a higher level 'A' to lower level 'B' causes a change in de Broglie wavelength which is 3 times the de Broglie wavelength in first Bohr orbit . Calculate total different wavelength possible if all possible transitions between level 'A' and level 'B' occur .
View SolutionCalculate frequency of revolution of electron in 4th Bohr orbit `Be^(+3)` ion .
Given that `(pi^(2)me^(4)k^(2))/(h^(3)) = 1.62 xx10^(15) sec^(-1),` symbols have usual meaning.
Express your answers in terms of `10^(15) sec^(-1).`
Write the answer upto one significant figure.
View SolutionIf radiation corresponding to first line of ''Balmer series'' of `He^(+)` ion is subjected to a smaple of `Ki^(+2)` ion (containing atoms in different energy states) and it causes ejection of photoelectron with non-zero kinetic energy then calculate least shell number in which the electron must be present in `Li^(+2)`.
View SolutionA sample of hydrogen contains equal number of `H^(1),H^(2) "and"H^(3)` atoms. The ratio of total number of protons and neutrons `((p)/(n))` in the sample is :
View SolutionA `Li^(2+)` ion is in a higher excited state of quantum number 'n' This excited ion can make a transition to the first excited state by successively emitting two photons of energies 10.2 eV and 17.0 eV respectivley . The value of 'n' is :
View SolutionTwo bulbs 'A' and 'B' emit red light and yellow light at 8000 "Å" and 4000 "Å" respectively. The number of photons emitted by both the bulbs per second is the same. If the red bulb is labelled as 100 watts, find the wattage of the yellow bulb.
View SolutionWhich state of triply ionised Beryllium `(Be^(+++))` the same orbital radius as that of the ground state hydrogen ?
View SolutionConsider Bohr's theory for hydrogen atom . The magnitude of orbit angular momentum orbit radius and velocity of the electron in nth energy state in a hydrogen atom are l , r and v respectively. Find out the value of 'x' if product of v, r and l (vrl) is directly proportional to `n^(x)`.
View Solutionwhen an electron falls from (n +2) state to (n) state in a `He^(+)` ion the photon emitted has energy `6.172xx10^(-19)` joules. What is the value of n?
View SolutionAt what atomic number would a transition from `n=2` to `n=1` energy level result in emission of photon of `lambda= 3xx10^(-8)m`?
View SolutionIn a container a mixture is prepared by mixing of three samples of hydrogen helium ion `(He^(+))` and lithium ion `(Li^(2+)).` In sample , all the hydrogen atoms are in 1st excited state and all the `He^(+)` ions are in third excited state and all the `Li^(2+))` ions are in fifth excited state. Find the total number of spectral lines observed in the emission spectrum of such a sample when the electrons return back to the ground state.
View SolutionThe uncertainty in position and velocity of the particle are 0.1 nm and `5.27xx10^(-24) ms^(-1)` respectively then find the approximate integral mass of the particle (in g ) . `(h=6.625xx10^(-34) Js)`
View SolutionWavelenth of the Balmer `H_(alpha)` line is 6565 "Å". Calculate the wavelength (in "Å") of `H_(beta)` line of same hydrogen like atom.
View SolutionElectrons in a sample of H-atoms make transition from state n=x to some lower excited state. The emission spectrum from the sample is found to contain only the line belonging to a particular series. If one of the photons has an energy of 0.6375 eV . Find the value of x.
`["Take" 0.6375 eV = 3/4 xx0.85 eV]`
View SolutionAn electron in `Li^(2+)` ion makes a transition from higher state `n_(2)` to lower state `n_(1)=6.` The emitted photons is used to ionize an electron in H-atom from 2nd excited state. The electron on leaving the H-atom has a de Broglie wavelength `lambda-12.016 "Å"`.Find the value of `n_(2).`
Note : Use `(12.016)^(2)= (150xx144)/(13.6xx11),lambda_("Å")=sqrt((150)/(KE_(eV)))`
View SolutionNitrogen has an atomic number of 7 and oxygen has an atomic number of 8. The total number of electron in the nitrate ion `(NO_(3)^(-))` is :
View Solution`h/pi` is the angular momentum of the electron in the `n= "………………."` Orbit of `He^(+).`
View SolutionElectrons in the H-atoms jumps from some higher level to 3rd energy level . If six spectral lines are possible for the transition find the initial position of electron.
View SolutionA light source of wavelength `lambda` illuminates a metal and ejects photoelectron with `(KE)^(max) = 1 eV.`
Another light source of wave length `(lambda)/(3),` ejects
photoelectrons from same metal with `(KE)^(max)=5 eV.`
Find the value of work function (eV) of metal.
View SolutionWavelength of rediowaves is 124 nm. Total number of photons per second produced by a source that consumes energy at the rate of `16"W is x" xx 10^(19).` the value of x is :
View SolutionThe ionisation potential of a hydrogen like species is 36 volt. What is the value of excitation energy from ground state to 1st excited state (in eV)?
View SolutionThe circumference of the second orbit of an atom or ion having single electron is 4 nm the de Broglie wavelength of electron (in nm) revolving in this orbit si :
View SolutionThe speed fo this dust particle `("mass" =n 10^(-3) g)` is measured with the uncertainty of `(3.313)/(pi) xx10^(-3) m//s.`
The minimum uncertainty in position of the dust particle `("in order of"10^(-26) m)` is :
View SolutionX-rays emitted from a copper target and a molybdenum target are found to contains a line of wavelength `22.85nm` attributed to the `K_(alpha)` line of an impurity element. The `K_(alpha)` lines of a copper `(Z=29)` and molybdenum `(Z=42)` have wavelength `15.42nm` and `7.12nm` respectively. Using Moseley's law, `gamma^(1//2)=a(Z-b)`. Calculate the atomic number of the impurity element.
View Solutionde Broglie wavelength `'lambda'` of an ideal gas molecule at any given temperature is given as `lambda prop m^(-x) xxT^(-y).`
Where `m=` mass of one gas molecule, `T=` temperature (K).
`"Given" x + y=?`
View SolutionWavelength of electron waves in two Bohr orbits is in ratio3:5 the ratio of kinetic energy of electron is 25 : x, hence x is :
View SolutionTotal different spectral lines observed in between 11th excited state and 3rd energy level in H-atom emission spectrum are:
View SolutionOn a metal with work function 2.0 eV light of wavelength 400 nm falls, maximum velocity of ejected photoelectron are `6.22xx10^(x)m//s.`. Hence x is :
View SolutionBy a sample of ground state atomic hydrogen ,UV light of energy `(13.6xx48)/(49) (eV)/("quanta")` is absorbed. How many different wavelengths will be observed in Balmer region of hydrogen spectrum?
View Solution`'alpha` particle' of 3.6 MeV are fired toward nucleus `._(Z)^(A)X,` at point of closest separation distance between `'alpha`particle' and 'X' is `1.6xx10^(-14)m.` Calculate atomic number of 'X'.
`["Given":1/(4piepsilon_(0))=9xx10^(9) "in S.I.units"]`
View SolutionFind the number of spectral lines in Paschen series emitted by atomic H, when electron is excited from ground state to 7th energy level returns back .
View SolutionCalculate the minimum kinetic energy in eV of photoelectron produced in caesium by 400 nm light . The critical (maximum) wavelength for the photoelectric effect in caesium is 660 nm, when the potential difference is 1.78 V.
View SolutionA beam of light has three `lambda, 4144 "Å", 4972 "Å" "and"6216 "Å"` with a total intensity of `3.6 xx10^(-3) Wm^(-2)` equally distributed amongst the three `lambda.` The beam falls normally on an area `1.0 cm^(2)` of a cleam metallic surface of work function 2.3 eV. Assume that there is no loss of light by reflection etc. Calculate the no. of photoelectrons emitted in 2 sec, in scientific notation,`x xx 10^(y)` find the value of y.
View SolutionA particle of charge equal to that of electron and mass 208 times the mass of the electron moves in a circular orbit around a nucleus of charge `+3e.` Assuming that the Bohr model of the atom is applicable to this system find the value of n for which the radius of the orbit is approximately the same as that of the first Bohr orbit of the hydrogen atom.
View SolutionCalculate the wavelength of a rested electron (in "Å") after it absorbs a photon of wavelength 9 nm.
`["Given"h=6xx10^(-34) J-sec,m=9xx10^(-31) kg].`
View SolutionA hydrogen like species with atomic number 'Z' is in higher excited state 'n' and emits photons of energy 25.7 and 8.7 eV when making a transition to 1st and 2nd excited state respectively. Calculate value of 'n'.
View Solution`barr,` average distance also described as expectation value of the distance of the electron from the nucleus is different from `r_(max).` For 1s orbital of H-atom, `r_(max)=a_(0), barr=3/2a_(0).` For 2s orbital of H-atom
`r_(max) =0.77a_(0) "and" 5.23a_(0).` Find `barr"for"r_(max) = 5.23a_(0).`
Also find `barr_(2)` for 1s orbital of `Li^(2+)` ion. Hence find the value of `((barr_(1)xxbarr_(2)))/(a_(0)^(2)).`
View SolutionThe number of lines of Balmer series of H-atom that belong to visible region.
View SolutionIn a sample of three H-atoms , all in the 5th excited state, electrons make a transition to 1st excited state. The maximum number of different spectral lines observed will be :
View SolutionIf a metal is exposed with light of wavelength `lambda,` the maximum kinetic energy produced is found to be 2 eV. When the same metal is exposed to a
wavelength `(lambda)/(5)` the maximum kinetic energy was found to be 14 eV. Find the value of work function (in eV).
View SolutionIf `n_(1)+n_(2) = 4 "and" n_(2)^(2)-n_(1)^(2)= 8,` then calculate maximum value of wavelength emitted in transition form `n_(2) to n_(1) "for"Li^(2+) "in nm[Given"R_(H) = 10^(7) m^(-1)].`
View SolutionSuppose the potential energy between electron and proton at a distance r is given by `(ke^(2))/(3r^(3))`. Use Bohr's theory to obtain energy of such a hypothetical atom.
View SolutionA cylindrical source of light which emits radiation radially (from curved surface) only , placed at the centre of hollow, metallic cylindrical surface, as shown in diagram.
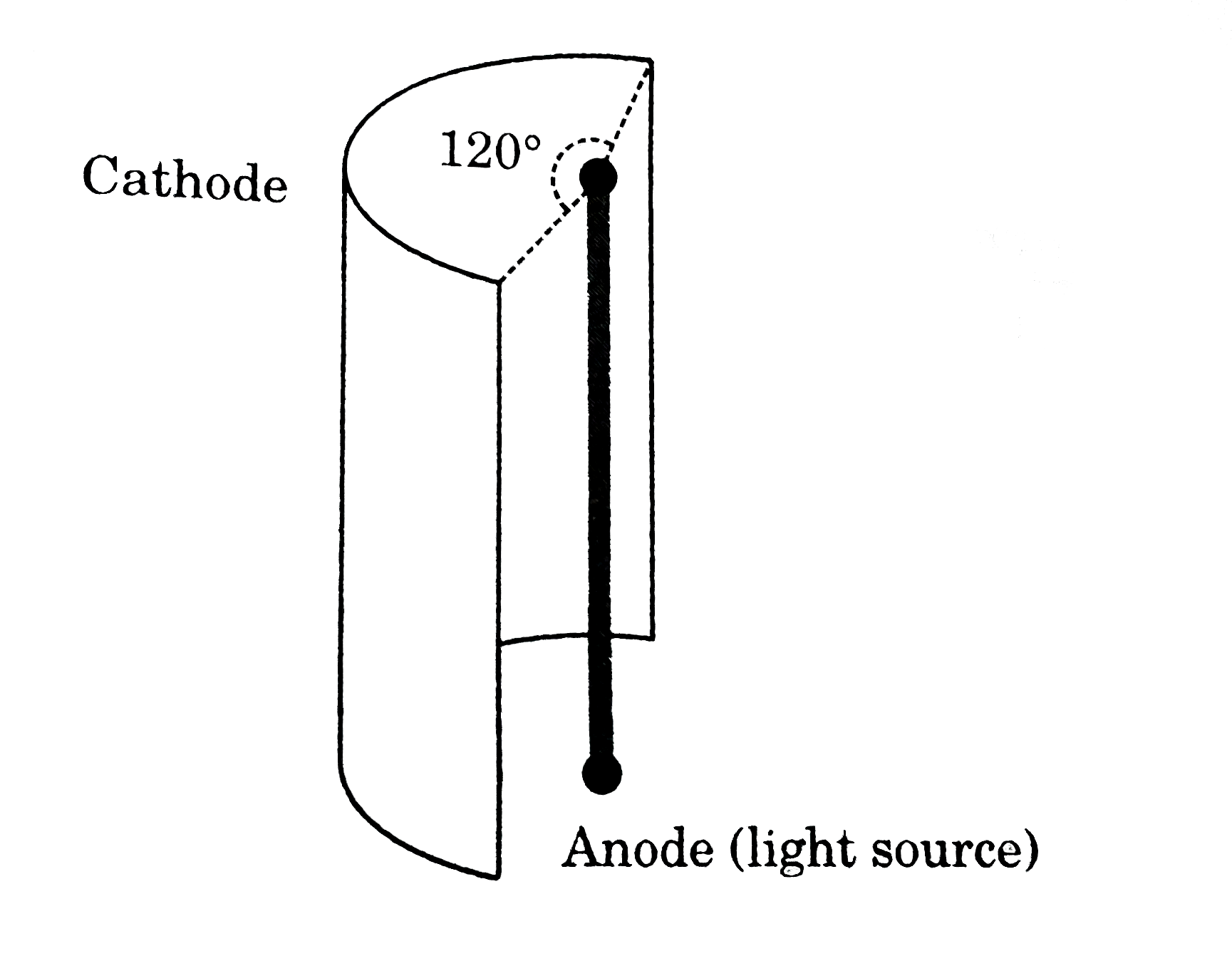
The power of source is 90 watt and it emits light of wavelength `4000 "Å"` only . The emitted photons strike the metallic cylindrical surface which results in ejection of photoelectrons. All ejected photoelectrons reaches to anode (light source). The magnitude of photocurrent (in amp) is :
`["Given": h=6.4xx10^(-34) J//sec]`
View SolutionCalculate the energy (in KJ) required to excite one litre of hydrogen gas at 1 atm and 298 K to the first excited state of atomic hydrogen. The energy for the dissociation of `H-H` id 436 KJ `mol^(-1)`. Give your answer excluding decimal places.
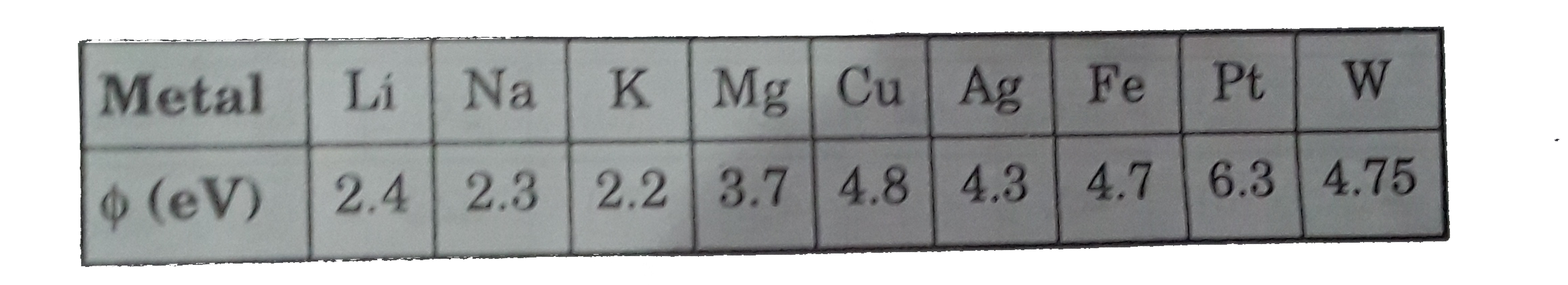
View SolutionThe work function `(phi)` of some metals is listed below . The number of metals which will show photoelectric effect when light of 300 nm wavelength falls on the metal is :
View SolutionThe atomic masses of He and Ne are 4 and 20 amu respectively . The value of the de Broglie wavelength of He gas at`-73.^(@)C` is ''M'' times that of the de Broglie wavelength of Ne at `727.^(@)C.` M is
View Solution
Subjective Type questions and complete solutions for chapter ATOMIC STRUCTURE of GRB CHEMISTRY (HINGLISH) of Class 11