Complete Solutions to Problem of chapter UNITS AND DIMENSIONS of Class 11 book with complete answers and questions
| Class 11 | PHYSICS |
| Chapter | UNITS AND DIMENSIONS |
| GRB PUBLICATION | Problem |
| Number Of Questions | 24 |
| Academic Year | 2023-24 |
In British engineering system the unit of mass is slug while the unit of force is force is pound. How many kg are in a slug?
06:18View SolutionIf a composite physical quantity in terms of moment of inertia I, force F, velocity v, work W and length L is defined as,
`Q = (IFv^(2)//WL^(3))`,
find the dimensions of Q and identify it.03:15View SolutionA calorie is a unit of heat or energy and it equals about `4.2 J, where 1 J = 1 kg m^(2) s^(-2)`. Suppose we employ a system of units in which the unit of mass equals `alpha kg`, the unit of length equals is `beta m` , the unit of time is `gamma s`. Show tthat a calorie has a magnitude `4.2 alpha^(-1) beta^(-1) gamma^(2)` in terms of the new units.
07:45View SolutionA gas bubble, from an exlosion under water, oscillates with a period `T` proportional to p^(a)d^(b)E^( c )`. Where `'P'` is the static pressure, `'d'` is the density of water `'E'` is the total energy of the explosion. Find the values of `a, b and, c`.
02:43View SolutionIf force, time and velocity are treated as fundamental quantities then dimensional formula of energy will be
01:39View SolutionIt is estimated that per minute each `cm^(2)` of earth receives about 2 calorie of heat energy from the sun. This constant is called solar constant S. Express solar constant in SI units.
02:07View SolutionFinding dimensions of resistance R and indcutance L, speculate what physcial quantities `(L//R) and (1)/(2)LI^2` represent, where I is current?
03:58View SolutionIn the formula `X=3YZ^(2)`, X and Z have dimensions of capacitance and magnetic induction respectively. What are the dimensions of Y in MKSQ system?
03:54View SolutionA quantity X is given by `in_(0) L(DeltaV)/(Delta t)`, where `in_(0)` is the permittivity of free space, L is a length, `DeltaV` is a potential difference and `Delta t` is a time interval. The dimensional formula for X is the same as that of -
04:16View SolutionColumn-1 gives three physical quantities. Select the appropriate units for the choices given in column-II. Some of the physical quantities may have more than one choice.
`{:("Column-I",,"Column-II"),("Capacitance",,"ohm-second"),("Inductance",,"Coulomb"^(2)//"Joule"),("Magnetic induction",,"coulomb"//"volt"),(,,"newton"//"ampere metre"),(,,"volt-second"//"ampere"):}`05:03View SolutionThe terms in column X are somehow related to the terms in columns Y and Z, match the following columns correctly:
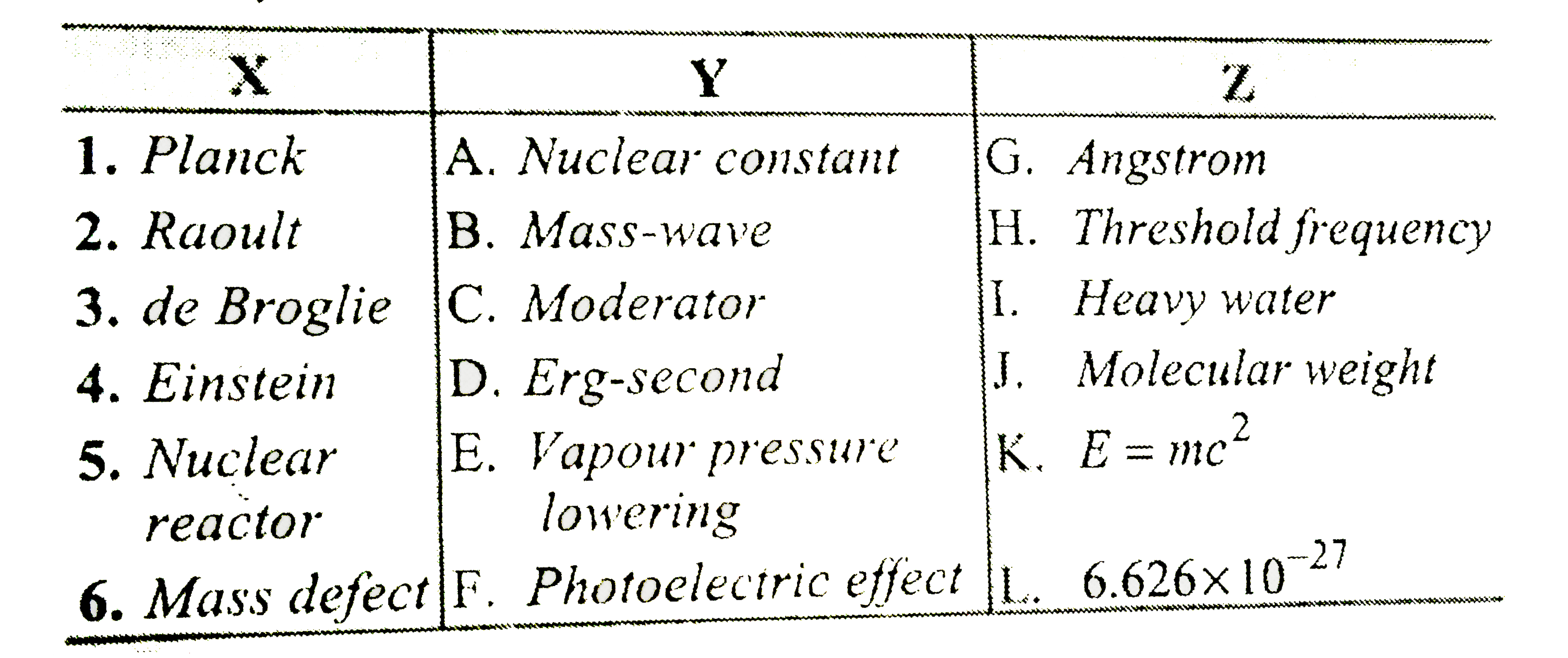 11:10View Solution
11:10View SolutionFour physical quantities are listed in column I. Their values are listed in column II in random order.
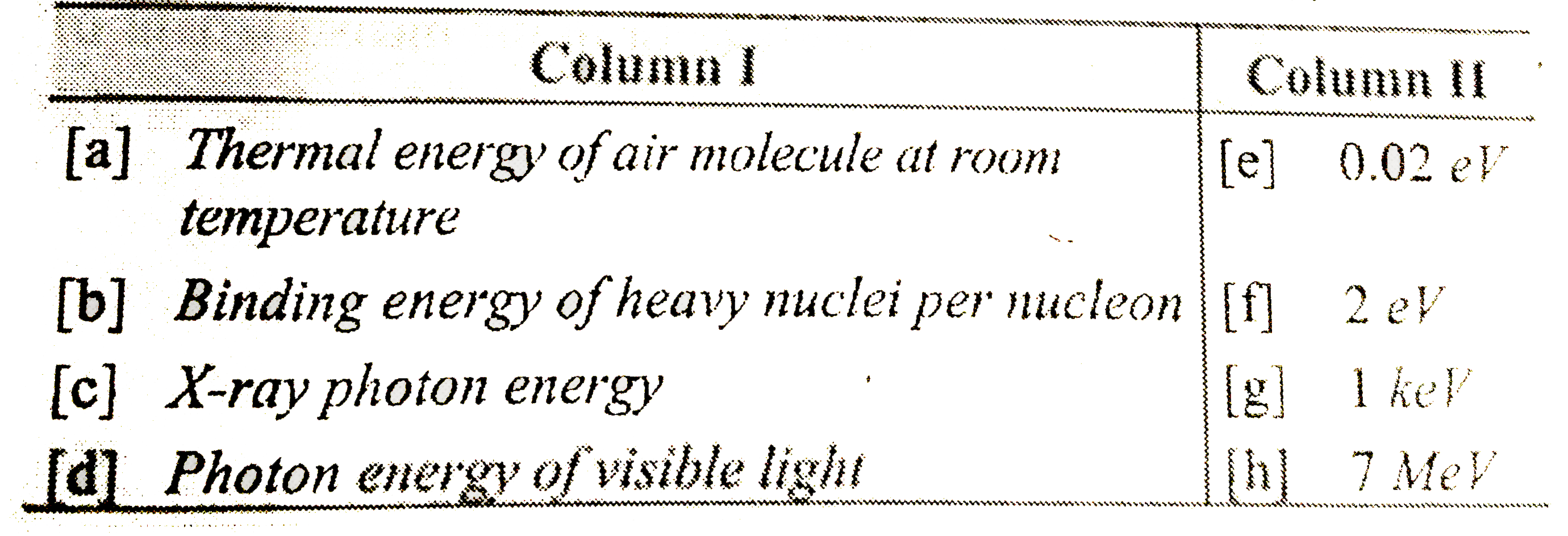
Match the column correctly.06:20View SolutionThe diameter of a ball was measured five times with the aid of a micrometer whose absolute error `(Deltad_("inst"))= pm 0.01 mm`. The results of measuring the diameter of the ball are `d_(1)=5.27 mm, d_(2)=5.30 mm, d_(3)=5.28 mm, d_(4)=5.32 mm` and `d_(5)=5.28 mm`. Find (a) mean value of ball diameter (b) mean absolute error (c) result of measurement (d) relative error (e) persentage error.
09:25View SolutionIn an experiment on determining density of a rectangular block, the dimensions of the block are mesured with vernier callipers `(V.C = 0.01 cm)` and its mass is measured with a beam balance `(L.C. = 0.1 g)`. The measured values are :
Mass`(m) = 39.3` g
Length`(l) = 5.12` cm
Breadth`(b) = 2.56` cm
Thickness`(t)=0.37` cm
Calculate density of the block with permissible limits of error.07:28View SolutionIn determination of value of acceleration due to gravity (g) by simple pendulum, the time period is measured by a topwatch (L.C. = 0.5 second) and length of the thread is measured with metre scale (L.C. 0.1 cm) and diametre of bob is measured with vernier callipers (L.C. = 0.1 cm). The following observations are recorded :
Length of the thread `=105.3 cm`
Diameter of the bob `=2.45 cm`
Time period `=2.07 s`
Number of oscillations `=10`
Calculate the value of g, estimate error and write the result in proper significant figures.12:42View SolutionGiven that mass of sun is `2 xx10^(30)` kg and radius of sun is `7 xx10^(8) m`. In what range do you expect the mass density of the sum to be ? Is it in the range of density of solid or liquid or gas ? Explain.
05:10View SolutionIn searle's experiment, the diameter of the wire, as measured by a screw gauge of least count 0.001 cm is 0.500 cm. The length, measured by a scale of least count 0.1cm is 110.0cm. When a weight of 40N is suspended from the wire, its extension is measured to be 0.125 cm by a micrometer of least count 0.001 cm. Find the Young's modulus of the meterial of the wire from this data.
06:05View SolutionIn an experiment to determine the specific heat of a metal,a `0.20 kg` block of the metal at `150 .^(@) C` is dropped in a copper calorimeter (of water equivalent `0.025 kg`) containing `150 cm^3` of water at `27 .^(@) C`. The final temperature is `40.^(@) C`. The specific heat of the metal is.
05:08View SolutionIn resonance apparatus for determining the velocity of sound in air, a turnning fork of frequency 320 Hz is used. A loud sound is heard when water level is at 24.8 cm and then at 78.5 cm. These lengths are called the first and second resonance lengths of air column in the resonance apparatus. Calculate the velocity of sound in air within the limits of permissiblr error.
06:13View SolutionIn `u-v` method for finding the focal length of a concave mirror. The mirror is fixed at position `A` marked `20.0cm` on an optical bench and an object needle is placed at position `B` marked `45.0cm` on an optical banch. For no parallax between object needle and image needle the image needle at position `C 57.5cm` on optical bench. Then percentage error in the measurement of focal length of the mirror is
05:47View SolutionWhen a body is weighed successively in the two pans of a physical balance with unequal arms, the apparent masses are found to be `M_(1)` and `M_(2)`. Show that the length of the arms are in the ratio `sqrt(M_(1)): sqrt(M_(2))`.
03:56View SolutionIf the lengths of the arms of a physical balance are `l_(1)` and `l_(2)` and a salesman weighs out twice to a customer articles of mass M by putting weughts first in one pan and then in the other, shown that the salesman is a loser by `M[((l_(1)-l_(2))^(2))/(l_(1)l_(2))]`.
03:56View SolutionThe pitch of a screw gauge is `1 mm` and three are `100` divisions on its circular scale. When nothing is put in between its jaws, the zero of the circular scale lies `6` divisions below the reference line. When a wire a placed between the jaws, `2` linear scale divisions are clearly visible while ` 62` divisions on circular scale coincide with the reference line. Determine the diameter of the wire.
02:18View SolutionLeast count of a vernier callipers is `0.01 cm` When the two jaws of the instrument touch each other the `5th` division of the vernier scale coincide with a main scale division and the zero of the vernier scale lies to the left of the zero of the main scale. Furthermore while measuring the diameter of a sphere, the zero markof the vernier scale lies between `2.4 cm` and `2.5 cm` and the `6th` vernier division coincides with a main scale division. Calculate the diameter of the sphere.
03:06View Solution
Problem questions and complete solutions for chapter UNITS AND DIMENSIONS of GR BATHLA & SONS PHYSICS (HINGLISH) of Class 11
