Complete Solutions to PROBLEMS FOR PARCTICE of chapter VECTORS of Class 11 book with complete answers and questions
| Class 11 | PHYSICS |
| Chapter | VECTORS |
| GRB PUBLICATION | PROBLEMS FOR PARCTICE |
| Number Of Questions | 23 |
| Academic Year | 2023-24 |
Given an example of a physical quantity which :
(a) has neither unit nor direction
(b) has direction but not a vector
(c) can be either a scalar no vector
(d) is nether a scalar nor a vector.01:09View SolutionWhat are the properties of two vectors `vecA` and `vecB` such that:
(a) `vecA + vecB = C and A + B =C `
(b) `vecA + vecB =vecC and A^(2) + B^(2) = C^(2)`02:11View SolutionCan the resultant be zero in case of :
(a) two unequal vectros
(b) three coplanar vectors
(c) three non- complanar vectors01:48View SolutionUnder what consition :
(a) resulant of tow vectors will be zero
(b) sum of the two vectors is equal to their differenc
(c) the magnitude of sum of two vectros is equal to the magnetude of diffrence between them .04:41View SolutionState whether the folowing statements are trune or false giving reason in brief :
(a) As adiidtion of vector is commutive so subtraction must also be
(b) Component of a vector is equal to their difference to itself is zero
(c) Angle between two vectors can be greater than m`180^(@)`
(d) A vector cannot be divided by a vector.04:53View SolutionA room has dimensions `3m xx 4m xx 5m`. A fly starting at one corner ends up at the diametrocally opposite corner .
(a). What is the magnetic of its displacement ?
(b) Could the length of its path be less than this distance ?
(c) Choosing a situtable cooridinate system find the position vector .
(d) If the fly does not fly but walks , what is the lenght of the shortest path it can take ?02:20View SolutionA 5 kg object with speed of `30 m//s` strikes a steel plants at an angle `45^(@)` and rebound at the same speed and same angle (Fig. 3.43). Calculate.
(a) the magntiude of the change is momentum of the object and
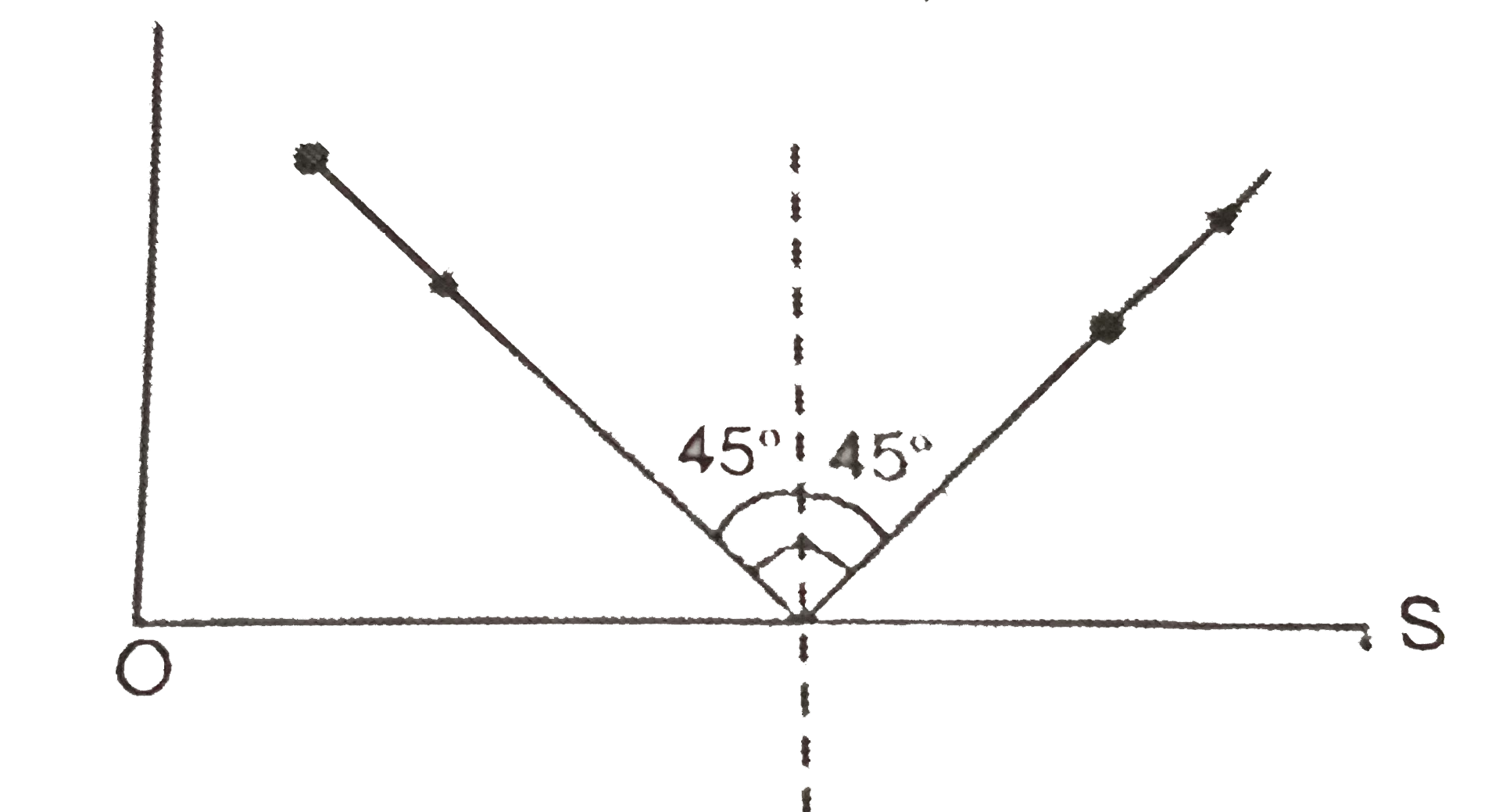
(b) the change in the magnitude of the momentum of the object.04:55View SolutionA body is moving uniformly on a circle with speed v. Find the magnitude of change in its velcotiy whem, it has turend an angle `theta`.
03:22View SolutionWhy do use express the laws of physics in vector form ? Explain.
01:11View SolutionIf `vecA=3hati+4hatj` and `vecB=7hati+24hatj`, find a vector having the same magnitude as `vecB` and parallel and same direction as `vecA`.
02:23View SolutionWhat is the condition that two non-zero vectors are (a) orhtogonal and (b) collinera?
02:03View SolutionIf `vecA = 2hati + hatj -3hatk, vecB = hati- 2hatj + hatk` and `vecC = - hati + hatj - 4hatk`, Calculate (i) `vecA.(vecB xx vecC), (ii)vecC (vecAxx vecB), (iii) vecA xx (vecB xx vecC)`
02:25View SolutionTwo constat forece `vecF_(1) = (2hati + 3hatj + 3hatk)` newton and `vecF_(2) = (5hati - 6hatj - 2hatk)` newton act toghter on a particle during its displacement from the position `(20hati + 15hatj)`m to `8vecKm`. Calculate the work done.
01:07View SolutionFind the moment of force `vecF = hati + hatj + hatk` acting at point `(-2, 3, 4)` about the point `(1, 2, 3)`.
02:24View SolutionCompute the force (in vetcor notation ) on an electron moving with velocity `vecV = 2.5 xx 10^(6)hati m//s` in a magnetic field `vecB =(10hati- 6 hatk) xx 10 ^(2) Wb//m^(2)`, if charge on an electron `e =1.6 xx 10^(-19)` coulomb.
01:11View SolutionThe particle of mass m is projected at `t=0` from a point O on the ground with speed `v_(0)` at an angle `45^(@)` to the horizontal as shown in Figure 3.44. Compute the magnitude and direction of the angular mometum of the particle about the point O at position :

When the velocity of the paticle is , `vecV= (0,7v_(0))hati-(0.3v_(0))hatj`View SolutionA river flows at 3 m/s and is 300 m wide . A man swing across the rivers with a velocity of 2 m/s directed always perpendicular to the flow of current . (a) How long does it take the man to cross the river ? (b) In what direction does he actually moves to cross the relative to the shore ? (c) How far down the fiver(from the starting point ) does he reach the opposite bank?
01:25View SolutionA river is flowing from west to east at a speed of `5m//s`. A man on the south bank of the river capable of swimming at `10m//s` in a still water wants to swim, across the river in a shortest time. He should swim in a direction
03:23View SolutionThe width of a rivers is 25m and in it water is flowing with a velocity of `4m//min` . A boatman is standing on the bank of the river . He want to sail the boat to a point at the other bank which is directly opposite to him . In what time will he cross the river , if he can sail the boat at `8m//min`. relative to the water ?
02:09View SolutionGiven that `vecA = 3hati + 4hatj +5hatk`. Find `vecB` such that `vecB.vecA= 38` and `vecB xx A = - hati + 2 hatj - hatk`.
01:05View SolutionA particle moves such that `x = 2t^(3) + t+ 8, y=t^(2) + t+ 3` and `z=3 sin pit` where x, y, z are in meter and t in seconds.
Calculate the acceleration of the particle at ` t = 3` second.03:16View SolutionA particle stats from rest at the origin with a constant acceleration
Calculate the position of the particle at `t=5 ` second.01:54View SolutionA particle moves along ellipatical path gives by
Calculate (i) radial component of acceleration `(veca_(r))`
(ii) trasverse component of acceleration `(veca_(theta))`.01:00View Solution
PROBLEMS FOR PARCTICE questions and complete solutions for chapter VECTORS of GR BATHLA & SONS PHYSICS (HINGLISH) of Class 11
