Complete Solutions to MODULE 1 (FROM CENTRIPETAL FORCE) of chapter CIRCULAR MOTION of Class 11 book with complete answers and questions
| Class 11 | PHYSICS |
| Chapter | CIRCULAR MOTION |
| S CHAND | MODULE 1 (FROM CENTRIPETAL FORCE) |
| Number Of Questions | 31 |
| Academic Year | 2023-24 |
A stone is tied at the end of a string lm long and whirled round by holding the other end. The stone makes 120 revolution is 12 seconds. Calculate the angular velocity and linear velocity.
View SolutionAn aircraft executes a hrorizontal loop of radius 1 km with a steady speed of `900 km h^(-1)` Compare its centripetal acceleration with the acceleration due to gravity ?
View SolutionA stone tied to the end of the string 100 cm long is whirled in a horizontal circle with a constant speed. If the stone makes 30 revolution in 15 s. Calculate the magnitude and direction of acceleration of the stone.
View SolutionAn artificial satellite is orbiting around the earth with the speed of `4 km s^(-1)` at a distance of `10^4` km from the earth. Calculate its centripetal acceleration.
View SolutionA body of mass 0.5 kg is whirled with a velocity of `2 ms^(-1)` using 0.5 m length string which can be withstand a tension of 15 N, Neglecting the force of gravity on the body predict whether or not the string will break.
View SolutionA stone of mass 100 g tied to the end of a string 80 cm long is whirled in a horizontal circle with a constant speed.If the stone makes 14 revolutions in 25 s, calculate the centripetal force acting on the stone.
View SolutionA string 1 m long can just support a weight of 16 kg. Amass of 1 kg is attached to one of its ends. The body is revolved in a horizontal circle about the other fixed end of the string. Find the greatest number of revolutions made by the mass per second ? (Take g = `10 ms^(-2)`)
View SolutionA car is moving with a velocity V. The car driver sees a truck at rest at a distance s. To avoid collision which is better, to apply brake or to turn sharply ?
View SolutionA long playing record revolves with a speed of `33 (1/4)` rev/min. and has a radius of 15 cm. Two coins are placed at 4 cm and 14 cm away from the centre of the record. If the coefficients of friction between the coin and the record is 0.15, which of the two coins will revolve with record ?
View SolutionA 70 kg man stands in contact against the wall of a cylindrical drum of radius 3 m rotating about its vertical axis with 200 rev/min. The coefficient of friction between the wall and his clothing is 0.15. What is the minimum rotational speed of the cylinder to enable the man to remain stuck to the wall (without falling) when the floor is suddenly removed ?
View SolutionYou may have seen in a circus a motorcyclist driving in a vertical loop inside a 'death well' chamber with holes, so the spectators can watch from outside. Explain clearly why the motorcyclist does not drop down when he is at the uppermost point, with no support from below. What is the minimum speed required to perform a vertical loop if the radius of the chamber is 25 m ?
View SolutionA stone of mass 0.3 kg tied to the end of a string in a horizontal plane in whirled round in a circle of radius 1 m withp. speed of 40 rev/min. What is the tension in the string ? What is the maximum speed with which the stone can be whirled around if the string can withstand a maximum tension of 200 N ?
View SolutionWhat is the minimum velocity with which a body of mass m must enter a vertical loop of radius R so that it can complete the loop ?
View SolutionA small mass m starts from rest and slides down the surface of a frictionless solid sphere of radius R. Calculate the angle at which the mass flies off the sphere. If there is friction between the mass and the sphere, does the mass of fly off at a greater or lesser angle than the previous one ?
View SolutionA spring of force constant `1200 Nm^(-1)` is mounted on a horizontal table and a mass of 3.0 kg of attached to the free and of the spring. The mass is made to rotate with an angular velocity of 10 rad/s. What is the elongation produced in the spring if its unstretched length is 60 cm ?
View SolutionA particle describes a horizontal circle on the smooth surface of an inverted cone, the height of the plane of the circle above the vertex is 9.8 cm. Find the speed of the particle (g= `9.8 m//s^2`) [See Fig.] (a) given in the answer section]
View SolutionA stone tied to the end of a string 80 cm long is whirled in a horizontal circle with a constant speed. If the centripetal acceleration of the stone is `9.91 ms^(-2)` how many revolutions does the stone make in 20 seconds.
View SolutionA mass is tied to a, string 1 m long and rotated in a horizontal circle at the rate of 600 rpm. If the tension inthe string is 3943.8 N, calculate the mass.
View SolutionA sphere of mass 20 g is whirled around in a horizontal circular path on the end of a string, the other end of which is stationary. If the tension in the string is 12.62 N, calculate the length of the string.
View SolutionA bridge over a canal is in the form of an arc of a circle of radius 20 m. Calculate the maximum speed at which a motor cycle can pass over the bridge without leaving the ground at the highest point ?.
View SolutionAn earth satellite revolves in a circular orbit at a height of 300 km above the earth's surface with a period of 90 min. What is its speed ? Calculate the radial acceleration of the satellite ? Radius of the earth is `6.38 xx 10^6`m.
View SolutionIn a children's park, using the park ride the visitors can be made to rotate in a vertical circle. At the top of the circle a rider has an effective weight of magnitude 1000 N. If his actual weight is 500 N
(i) What is his effective weight at the bottom of the cricle ?
(ii) What is the effective weight when the rider is halfway to the centre of the circle ?
View SolutionA ball of mass 100 g is made to loop-the-loop in a vertical circle as shown in Fig. 7.1.29. The radius of the circle is 0.5 m (i)-What is the critical velocity at the highest point of the track ? (ii) If the velocity of the ball at the highest point of the track is twice the critical velocity what is the force exerted by the ball against track?
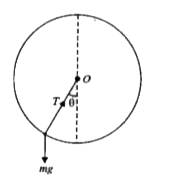
View SolutionWhat is the tension Tin the cord (see Fig. 7.1.30) connected to the ball of mass 0.1 kg rotating in a vertical'circle of radius 1m, if the speed of the ball is 0.2 m/s and `theta = 30^(@)` .
View SolutionThe speed of the electron in the hydrogen atom is approximately `2.2 xx 10^(6) m//s`. What is the centripetal force acting on the electron ifthe radius of the circular orbit is `0.53 xx 10^(-10) m`?Mass of the electron is `9.1 xx 10^(-31) kg`
View SolutionWhat is the centripetal acceleration of a space vehicle moving round the earth, assuming its orbit to be circular ? What is the apparent weight of an astronaut ?
View SolutionA small stone of mass 100 g in rotated I a verticlal circle of radius 40 cm. What is the minimum speed needed at the lowest point for looping the loop? Also find the tension in the string at this point `(g=10ms^(-2)).`
View SolutionSuppose that the rotation of the earth was increased such that the centripetal acceleration was equal to the gravitational acceleration at the equator (i) Find the speed of a person standing at the equator and (ii) How long would a day be ?
View SolutionThe kinetic energy K of a particle moving along a circle of radius R depends on the distance covered s as `K=as^(2)`. The force acting on the particle is
View SolutionA car is moving in a circular horizontal track of radius 10 m with a constant speed of `10ms^(-1)`. A plumb bob is suspended from the roof of the car by a light rigid rod of length 1 m. The angle made by the rod with track is
View SolutionIf a body moves on the circumference of a circle with a speed equal to that which it would acquire by falling freely through half the radius of the circle, prove that its centripetal acceleration is equal to the acceleration of the free fall.
View Solution
MODULE 1 (FROM CENTRIPETAL FORCE) questions and complete solutions for chapter CIRCULAR MOTION of S CHAND PHYSICS (ENGLISH) of Class 11
All Exercises from S CHAND Class 11 PHYSICS CIRCULAR MOTION
- MODULE 1 (CONCEPTUAL SHORT ANSWERS QUESTIONS WITH ANSWERS )
- MODULE 1 (FROM ANGULAR VELOCITY)
- MODULE 1 (FROM BANKING OR RAILS AND ROADS)
- MODULE 1 (FROM CENTRIPETAL FORCE)
- MODULE 1 (LONG ANSWER QUESTIONS)
- MODULE 1 (SHORT ANSWER QUESTIONS)
- MODULE 1 (VERY SHORT ANSWER QUESTIONS)
- MODULE 2 (CONCEPTUAL SHORT ANSWERS QUESTIONS WITH ANSWERS)
- MODULE 2 (FROM ANGULAR MOMENTUM - CONSERVATION)
- MODULE 2 (FROM CENTRE OF MASS)
- MODULE 2 (FROM EQUATIONS OF ROTATIONAL MOTION)
- MODULE 2 (FROM MOMENT OF INERTIA, TORQUE)
- MODULE 2 (FROM ROTATIONAL KINETIC ENERGY , WORK ,POWER)
- MODULE 2 (LONG ANSWER QUESTIONS)
- MODULE 2 (SHORT ANSWER QUESTIONS)
- MODULE 2 (VERY SHORT ANSWER QUESTIONS)
- SOLVED EXAMPLES