A 70 kg man stands in contact against the wall of a cylindrical drum of radius 3 m rotating about its vertical axis with 200 rev/min. The coefficient of friction between the wall and his clothing is 0.15. What is the minimum rotational speed of the cylinder to enable the man to remain stuck to the wall (without falling) when the floor is suddenly removed ?
The correct Answer is:4.67 rad s−1
To solve the problem, we need to determine the minimum rotational speed of the cylindrical drum that will allow the man to remain stuck to the wall when the floor is suddenly removed.
1. Identify the Forces Acting on the Man:
When the floor is removed, the only force that can prevent the man from falling is the frictional force between his clothing and the wall of the drum. The gravitational force acting on him is Fg=mg, where m is the mass of the man and g is the acceleration due to gravity.
2. Frictional Force:
The frictional force can be expressed as:
Ff=μN
where μ is the coefficient of friction and N is the normal force acting on the man.
3. Normal Force in Circular Motion:
In circular motion, the normal force N is provided by the centripetal force, which is given by:
N=mω2r
where ω is the angular velocity in radians per second and r is the radius of the drum.
4. Setting Up the Equation:
To prevent the man from falling, the frictional force must be equal to or greater than the gravitational force:
Ff≥Fg⟹μN≥mg
Substituting for N:
μ(mω2r)≥mg
5. Canceling Mass:
Since m appears on both sides of the equation, we can cancel it out (assuming m≠0):
μω2r≥g
6. Solving for Angular Velocity ω:
Rearranging the inequality gives:
ω2≥gμr
Taking the square root:
ω≥√gμr
7. Substituting Values:
Given:
- m=70kg (not needed for calculation)
- r=3m
- μ=0.15
- g=10m/s2
We substitute these values into the equation:
ω≥√100.15×3
8. Calculating the Right Side:
ω≥√100.45=√100045=√22.22≈4.72rad/s
Final Answer:
The minimum rotational speed of the cylinder to enable the man to remain stuck to the wall is approximately 4.72rad/s.
---
When the floor is removed, the only force that can prevent the man from falling is the frictional force between his clothing and the wall of the drum. The gravitational force acting on him is Fg=mg, where m is the mass of the man and g is the acceleration due to gravity.
The frictional force can be expressed as:
Ff=μN
where μ is the coefficient of friction and N is the normal force acting on the man.
In circular motion, the normal force N is provided by the centripetal force, which is given by:
N=mω2r
where ω is the angular velocity in radians per second and r is the radius of the drum.
To prevent the man from falling, the frictional force must be equal to or greater than the gravitational force:
Ff≥Fg⟹μN≥mg
Substituting for N:
μ(mω2r)≥mg
Since m appears on both sides of the equation, we can cancel it out (assuming m≠0):
μω2r≥g
Rearranging the inequality gives:
ω2≥gμr
Taking the square root:
ω≥√gμr
Given:
- m=70kg (not needed for calculation)
- r=3m
- μ=0.15
- g=10m/s2
ω≥√100.15×3
ω≥√100.45=√100045=√22.22≈4.72rad/s
The minimum rotational speed of the cylinder to enable the man to remain stuck to the wall is approximately 4.72rad/s.
Topper's Solved these Questions
- CIRCULAR MOTION BOOK - ICSECHAPTER - CIRCULAR MOTION EXERCISE - MODULE 1 (FROM BANKING OR RAILS AND ROADS)
13 Videos
- CIRCULAR MOTION BOOK - ICSECHAPTER - CIRCULAR MOTION EXERCISE - MODULE 2 (CONCEPTUAL SHORT ANSWERS QUESTIONS WITH ANSWERS)
14 Videos
- CIRCULAR MOTION BOOK - ICSECHAPTER - CIRCULAR MOTION EXERCISE - MODULE 1 (FROM ANGULAR VELOCITY)
4 Videos
- COMPETITION CARE UNITBOOK - ICSECHAPTER - COMPETITION CARE UNITEXERCISE - OBJECTIVE QUESTIONS FROM PREVIOUS IAS EXAMINATIONS
50 Videos
Similar Questions
A 70kg man stands in contact against the inner wall of a hollow cylindrical drum of radius 3m rotating about its vertical axis. The coefficient of friction between the wall and his clothing is 0.15. What is the minimum rotational speed of the cylinder to enable the man to remain stuck to the wall (without falling) when the floor is suddenly removed?
View SolutionA 70kg man stands in contact against the inner wall of a hollw cylindrical drum of radius 3m rotating about its verticle axis. The coefficient of friction between the wall and his clothing nis 0.15 . What is the minimum rotational speed of the cylinder to enable the man to remain stuck to the wall (without falling) when the floor is suddenly removed?
View SolutionA 70kg man stands in contact against the inner wall of a hollow cylindrical drum of radius 3m rotating about its verticle axis. The coefficient of friction between the wall and his clothing is 0.15 . What is the minimum rotational speed of the cylinder to enable the man to remain stuck to the wall (without falling) when the floor is suddenly removed?
View SolutionA 70 kg man stands in contact against the inner wall of a hollow cylindrical drum of radius 3 m rotating about its vertical axis with 200 rev/min. The coefficient of friction between the wall and his clothing is 0.15 m What is the minimum rotational speed of the cylinder to enable the man to remain stuck to the wall (without falling) when the floor is suddenly removed ?
View SolutionA person stands in contact against the inner wall of a rotor of radius r. The coefficient of friction between the wall and the clothing is μ and the rotor is rotating about vertical axis. The minimum angular speed of the rotor so that the person does not slip downward is
View SolutionA block of mass 10 kg in contact against the inner wall of a hollow cylindrical drum of radius 1m. The coefficient of friction between the block and the inner wall of the cylinder is 0.1. The minimum angular velocity needed for the cylinder to keep the block stationary when the cylinder is vertical and rotating about its axis, will be (g=10m/s2)
View SolutionA block is mass m moves on as horizontal circle against the wall of a cylindrical room of radius R. The floor of the room on which the block moves is smooth but the friction coefficient between the wall and the block is μ. The block is given an initial speed v0. As a function of the speed v write a. the normal force by the wall on the block. b. the frictional force by the wall and c. the tangential acceleration of the block. d. Integrate the tangential acceleration (dvdt=vdvds) to obtain the speed of the block after one revoluton.
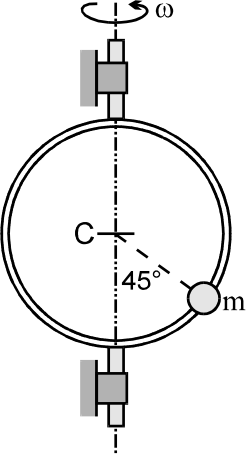
View SolutionA small bead of mass m = 1 kg is free to move on a circular hoop. The circular hoop has centre at C and radius r = 1 m and it rotates about a fixed vertical axis. The coefficient of friction between bead and hoop is μ=0.5. The maximum angular speed of the hoop for which the bead does not have relative motion with respect to hoop, at the position shown in figure is : (Take g=10m/s2)
View SolutionTwo bodies A and B of masses 5 kg and 10 kg in contact with each other rest on a table against a rigid wall as shown in fig. The coefficient of friction between the bodies and the table is 0.15. A force 200 N is applied horizontally to A. What are
(a) the reaction of the partition
(b) the action-reaction forces between A and B ?
(c) What happens when the wall is removed? Does the answer to (b) change, when the bodies are in motion? Ignore the difference between μs and μk
View SolutionA uniform ladder of length 3.25 m and weight 250 N is placed against a smooth vertical wall with its lower end 1.25 m from the wall. If coefficient of friction between the ladder and the floor is 0.3, then, find the frictional force acting on the ladder at the point of contact between ladder and floor.
View Solution
