Complete Solutions to Exercise of chapter THERMODYNAMICS of Class 11 book with complete answers and questions
| Class 11 | CHEMISTRY |
| Chapter | THERMODYNAMICS |
| P BAHADUR | Exercise |
| Number Of Questions | 100 |
| Academic Year | 2023-24 |
During `200J` work done on the system, `140J` of heat is given out. Calculate the change in internal energy.
View SolutionA system does `40J` work on surrounding as well as gives out `20J` energy. Calculate the change in internal energy.
View SolutionA system does `100J` work on surroundings gy absorbing `150J` of heat. Calculate the change in internal energy.
View SolutionA gas absorbs `200J` of heat and expands against the external pressure of `1.5 atm` from a volume of `0.5` litre to `1.0` litre. Calculate the change in internal energy.
View SolutionCalculate the work done during the process, when one mole of gas is allowed to expand freely into vacuum.
View SolutionTwo liters of `N_(2)` at `0^(@)C` and `5` atm pressure is expanded isothermally against a constant external pressure of `1` atm untill the pressure of gas reaches `1` atm. Assuming gas to be ideal, claculate the work of expansion.
View SolutionThe internal energy change in the conversion of `1.0` mole of the calcite form of `CaCO_3` to the aragonite form is `+0.21 KJ`. Calculate the enthalpy change when the pressure is `1.0 `bar, given the densities of the solids are `2.71 gcm^(-3)` and `2.93 gcm^(-3)` respectively.
View SolutionCalculate the work done in an open vessel at `300K,` when `112g` ion reacts with dil. `HCl`.
View SolutionA sample of `0.20` mole of a gas at `44^@C` and `1.5 atm` pressure is cooled to `27^@C` and compressed to `3.0atm`. Calculate `DeltaV`. Suppose the original sample of gas was heated at constant volume until its pressure was `3.0atm` and then cooled at `27^@C`, what would have been `DeltaV`?
View SolutionConsider the reaction `N_2 + 3H_2 to 2NH_3`, taking place at `300K` temperature and constant pressure. For the reaction, `DeltaH = -22.0 kcal`. Calculate the amount of heat released if the reaction is carried out at `400K` and same constant pressure. Given that: `C_(p(N_(2)))=C_(p(H_(2)))=3.5 R, C_(p(NH_(3)))=4R`
View SolutionA sample of argon gas at `1atm` pressure and `27^@C` expands reversibly and adiabatically from `1.25dm^3` to `2.5dm^3`. Calculate the enthalpy change in the process. Given that `C_(v(m))` for `Ar` is `12.45JK^(-1)mol^(-1)` and antilog `(0.199)=1.58`.
View SolutionOne mole of an ideal gas is heated at constant pressure from `0^(@)C` to `100^(@)C`.
a. Calculate the work done.
b. If the gas were expanded isothermally and reversibly at `0^(@)C` from `1 atm` to some othe pressure `P`, what must be the final pressure if the maixmum work is equal to the work in `(a)`?
View SolutionOne mole of an ideal mono-atomic gas is taken round cyclic process `ABCD` as shown in figure below. Calculate
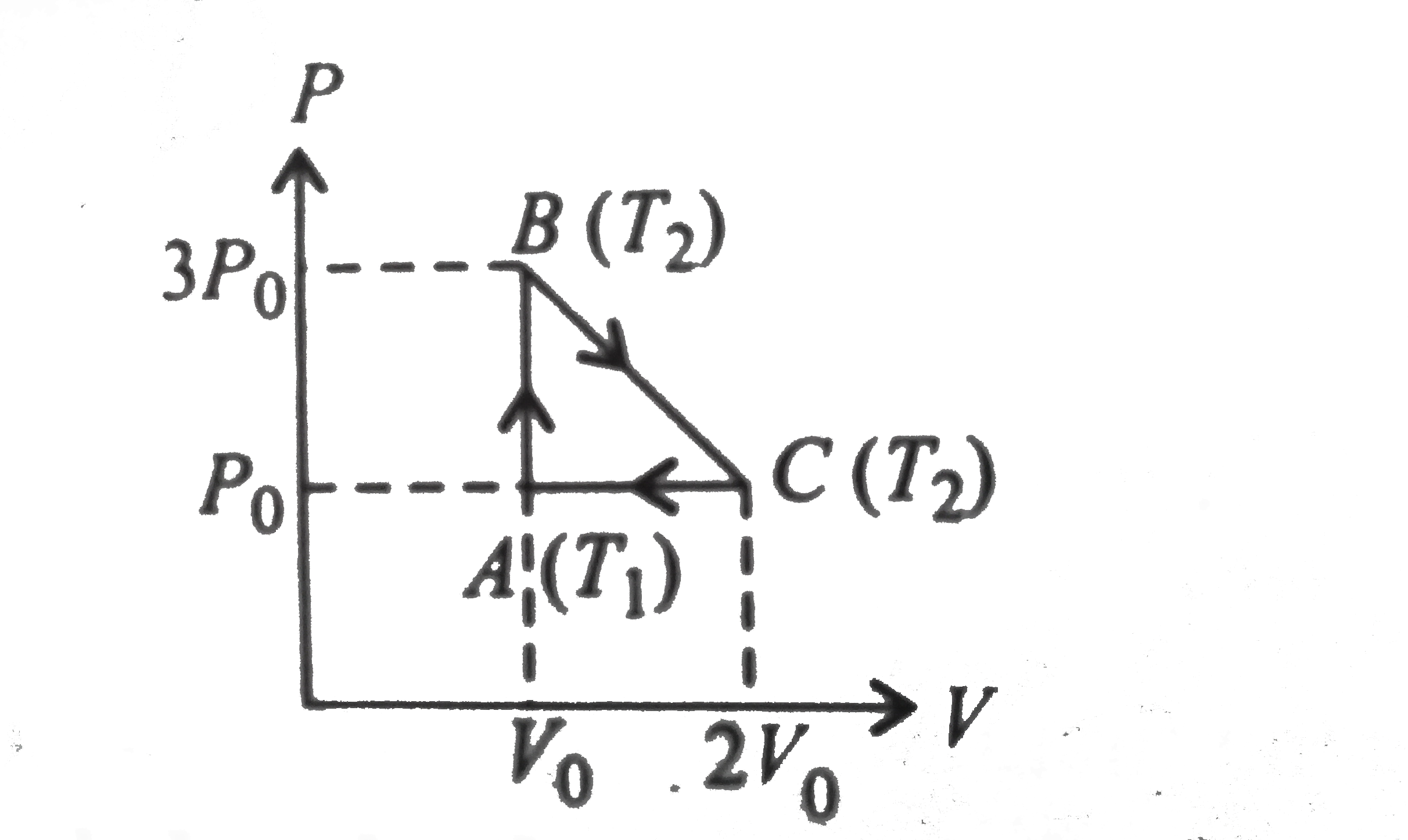
View SolutionDerive a relation showing reversible work of expansion from volume `V_1` to `V_2` by 'n' moles of a real gas obeying van der waal's equation at temperature `T` where volume occupied by molecules may be taken as negligible in comparison to total volume of gas.
View SolutionWhat work is to be done on `2` mole of a perfect gas at `27^@C` if it is compressed reversibly and isothermally from a pressure of `1.01 xx 10^5 Nm^(-2)` to `5.05 xx 10^6 Nm^(-2)`? Also calculate the free energy change.
View SolutionCalculate the maximum work done in expanding `16g` of oxygen at `300K` occupying volume of `5dm^(3)` and isothermally untill the volume become `25dm^(3)`?
View SolutionCalculate`q, W, DeltaU` and `DeltaH` for the isothermal reversible expansion of one mole of an ideal gas from an initial pressure of `1.0` bar to a final pressure of `0.1` bar at a constant temperature of `273K.`
View SolutionCalculate the change of entropy, `Delta_(r)S^(Theta)` at `298K` for the reaction in which urea is formed from `NH_(3)` and `CO_(2)`.
`2NH_(3)(g)+CO_(2)(g) rarr NH_(2)CONH_(2)(aq)+H_(2)O(l)`
The standard entropies `(J K^(-1)mol^(-1))` are:
`NH_(2)CONH_(2)(aq) =174.0, H_(2)O(l) = 69.9`
`NH_(3)(g) = 192.3, CO_(2)(g) = 213.7`
View SolutionThe enthalpy of vaporisation of liquid diethly ether `-(C_2H_5)_2O,` is `26.0kJmol(-1)` at its boiling point `(35.0^@C)`. Calculate `DeltaS` for conversion of : (a) liquid to vapour, and (b) vapour to liquid at `35^@C`.
View SolutionEthanol boils at `78.4^@C` and the enthalpy of vaporisation of ethanol is `42.4kJ mol^(-1)`. Calculate the entropy of vaporisation of ethanol.
View SolutionCalculate the entropy change for the conservation of following: (a) `1g` ice to water at `273K , DeltaH_f` for `ice=6.025kJmol^(-1)`. (b) `36g` water to vapour at `373K, DeltaH_v`for `H_2O=40.63kJmol(-1)`
View SolutionGiven `S^@` for `C_("Graphite"), H_(2(g))` and `CH_(4(g))` are `5.70, 130.7` and `186.3 JK^(-1) mol^(-1)`. Also standard heat of formation of `CH_4` is `-74.81kJmol^(-1).` Calculate the standard Gibbs energy change for the formation of methane at `298K.`
`C_("Graphite")+2H_(2(g))toCH_(4(g))`
View SolutionIn a fuel cell, methanol if used as fuel and oxygen gas is used as an oxidiser. The reaction is
`CH_(3)OH(l) +(3)/(2)O_(2)(g)rarrCO_(2)(g)+2H_(2)O(l)`
Calculated standard Gibbs free enegry change for the reaction that can be converted into electircal work. If standard enthalpy of combustion for methanol is `-702 kJ mol^(-1)`, calculate the efficiency of converstion of Gibbs energy into useful work.
`Delta_(f)G^(Theta)` for `CO_(2),H_(2)O, CH_(3)OH,O_(2)` is `-394.00, -237.00,-166.00`and `0 kJ mol^(-1)` respectively.
View SolutionFor the water gas reaction:
`C(s) +H_(2)O(g) hArr CO(g) +H_(2)(g)`
the standard Gibbs enegry for the reaction at `1000K` is `-8.1 kJ mol^(-1)`. Calculate its equilibrium constant.
View SolutionThe standard Gibbs free energies for the reaction at `1773K` are given below:
`C(s) +O_(2)(g) rarr CO_(2)(g), DeltaG^(Theta) =- 380 kJ mol^(-1)`
`2C(s) +O_(2)(g) hArr 2CO(g),DeltaG^(Theta) =- 500 kJ mol^(-1)`
Discuss the possibility of reducing `AI_(2)O_(3)` and `PbO` with carbon at this temperature,
`4AI +3O_(2)(g) rarr 2AI_(2)O_(3)(s),DeltaG^(Theta) =- 22500 kJ mol^(-1)`
`2Pb +O_(2)(g) rarr 2PbO(s),DeltaG^(Theta) =- 120 kJ mol^(-1)`
View SolutionPredict whether it is possible or not to reduce magnesium oxide using carbon at `298K` according to the reaction.
`MgO(s) +C(s) rarr Mg(s) +CO(g)`
`Delta_(r)H^(Theta) = +491.18 kJ mol^(-1)` and `Delta_(r)S^(Theta) = 197.67 J K^(-1) mol^(-1)`
If not at what temperature, the reaction becomes spontaneous.
View SolutionCalculate `DeltaG^(Theta)` for the conversion of oxygen to ozone, `((3)/(2)) O_(2)(g) hArr O_(3)(g) at 298 K`, of `K_(p)` for this conversion is `2.47 xx 10^(-29)`.
View SolutionCalculate the equilibrium constant `K_p` for the reaction given below if `DeltaG^@ =-10.632` kJ at `300K.`
`CO_(2(g))+H_(2(g))hArrCO_((g))+H_(2)O_((g))`
View SolutionCalculate the value of `DeltaG` at `700K` for the reaction, `nX to mB`. Given that value of `DeltaH=-113kJ mol^(-1)` and `DeltaS=-145JK^(-1)mol^(-1).`
View SolutionCalculated the Gibbs energy change on dissolving one mole of sodium chloride at `25^(@)C`.
Lattice `=+ 777.0 kJ mol^(-1)`
Hydration of `NaCI =- 774.0 kJ mol^(-1)`
`DeltaS` at `25^(@)C = 40 J K^(-1) mol^(-1)`
View SolutionCalculate the standard free energy change for the reaction,
`N_(2(g))+3H_(2(g))to2NH_(3(g)) at 298 K`.
Given, `DeltaH^@ =-92.4kJ` and `DeltaS^@ = -198.3 JK^(-1)`. Also, comment on the result.
View SolutionFor reaction, `N_(2(g))+3H_(2(g))to2NH_(3(g))`, `DeltaH=-95.4kJ` and `DeltaS=-198.3JK^(-1)`. Calculate the maximum temperature at which the reaction will proceed in forward direction.
View SolutionThe equilibrium constant for the reaction given below is `2.0xx10^(-7)` at `300K.` Calculate the standard free energy change for the reaction,
`PCl_(5(g))hArrPCl_(3(g))+Cl_(2(g))`.
Also, calculate the standard entropy change if `DeltaH^@=28.40kJmol^(-1)`.
View SolutionCalculate the Gibbs energy change for the reaction given below:
`Zn_((s))+Cu_((s))^(2+)toCu_((s))+Zn_((aq.))^(2+)`
Given that standard Gibbs energy (`G^@`) for `Zn_((aq.))^(2+),Cu_((aq.))^(2+)` are `-147.2 kJ mol^(-1)` and `65.0 kJ mol^(-1)` respectively.
View SolutionCalculate the boiling point of water at `24` torr pressure. The average `DeltaH_(vap.)` over the temperature range is `10.12 kcal mol^(-1)`. Will all the water form gaseous state if placed in a closed container, if not then, how wer can convert all the water into vapour state?
View SolutionCalculate `q, W, DeltaU` and `DeltaH` for the isothermal reversible expansion of one mole of an ideal gas from an initial pressure of `1.0bar` to a final pressure of `0.1bar` at a constant temperature of `273K`.
View Solution`10g` of argon gas is compressed isothermally and reversibly at a temperature of `27^(@)C` from `10L` to `5L`. Calculate `q, w, DeltaU`, and `DeltaH` for this process. `R = 2.0 cal K^(-1) "mol"^(-1), log_(10)2 = 0.30`. Atomic weight of `Ar = 40`.
View SolutionA sample of argon gas at `1atm` pressure and `27^(@)C` expands reversibly and adiabatically from `1.25 dm^(3)` to `2.50 dm^(3)`. Calculate the enthalpy change in this process. `C_(vm)` for orgon is `12.48J K^(-1) mol^(-1)`.
View Solution`1mol` of an ideal gas undergoes reversible isothermal expansion form an initial volume `V_(1)` to a final volume `10V_(1)` and does `10kJ` of work. The initial pressure was `1xx 10^(7) Pa`.
a. Calculate `V_(2)`.
b. If there were `2mol` of gas, what must its temperature have been?
View SolutionTwo moles of a perfect gas undergo the following process: (a) A reversible isobaric expansion from (`1.0` atm, `20.0` litre) to (`1.0` atm,`40.0`litre). (b) A reversible isochoric change of state from (`1.0` atm, `40.0` litre) to (`1.0` atm, `40.0` litre). (b) A reversible isochoric change of state from (`1.0` atm, `40.0` litre) to (`0.5` atm, `40.0` litre). (c) A reversible isothermal compression from (`0.5`atm, `40.0` litre) to (`1.0` atm, `20.0` litre). (i) Sketch with labels each of the process on the same `P-V` diagram. (ii) Calculate the total work `(W)` and the total heat change `(q)` involved in the above process. (iii) What will be the value of `DeltaU, DeltaH` and `DeltaS` for the overall process?
View SolutionA gas expands from `3 dm^(3)` to `5dm^(3)` anainst a constant pressure of `3atm`. The work done during the expansion if used to heat `10mol` of water at temperature `290K`. Find the final temperature of water, if the specific heat of water `= 4.18g^(-1)K^(-1)`.
View SolutionShow that the reaction
`CO(g) +(1//2)O_(2)(g) rarr CO_(2)(g)`
at `300K` is spontaneous and exothermic, when the standard entropy change is `-0.094 k J mol^(-1) K^(-1)`. The standard Gibbs free energies of formation for `CO_(2)`and `CO` are `-394.4`and `-137.2kJ mol^(-1)`, respectively.
View Solution`100mL` of a liquid is contained in an insulated container at a pressure of 1bar. The pressure is steeply increased to `100`bar. The volume of the liquid is decreased by `1mL` at this constant pressure. Find `DeltaH` and `DeltaU`.
View SolutionIn which of the following cases entropy decreases?
View SolutionIn a reaction `DeltaG` and `DeltaH` both are positive. The reaction would not be spontaneous if :
View SolutionTemperature of `1mol` of a gas is increased by `1^(@)` at constant pressure. The work done is
View SolutionWhen a gas is subjected to adiabatic expansion, it gets cooled due to :
View SolutionDuring isothermal expansion of an ideal gas, its:
View SolutionChange in entropy is negative for
View SolutionThe `DeltaG` in the process of melting of ice at `-15^@C` is :
View SolutionA boiled egg show `a//an`…… in entropy.
View SolutionFor the adiabatic expansion of an ideal gas:
View SolutionThe occurrence of a reaction of impossible if
View SolutionWhich statements is correct?
View SolutionAn ideal gas undergoing expansion in vacuum shows:
View SolutionHuman body is an example of:
View Solution`1` mole of gas occupying `3` litre volume is expanded against a constant external pressure of `1` atm to a volume of `15` litre. The work done by the system is:
View SolutionThe latent heat of vapourisation of a liquid at `500K` and `1atm` pressure is `10kcal mol^(-1)`. What will be change in internal energy of `3mol` of liquid at same temperature?
View SolutionOne mole of ice is converted into water at `273K`. The entropies of `H_2O(s)` and `H_2O(l)` are `38.20` and `60.01Jmol^(-1)K^(-1)` respectively. The enthalpy change for the conversion is:
View SolutionA carnot engine operates between temperatures `T` and `400K(Tgt400K)`. If efficiency of engine is `25%`, the temperature `T` is :
View SolutionThe molar heat capacity for a gas at constant `T` and `P` is
View SolutionThe work done in an open vessel at `300K`, when `112g` iron reacts with dil. `HCL` is:
View Solution`16g` oxygen gas expands at `STP` to occupy double of its oxygen volume. The work done during the process is:
View SolutionHeat changes measured in a bomb calorimeter are reported in terms of :
View SolutionOne mole of a gas in the state `A(P_1, V_1, T_1)` is subjected to adiabatic expansion to attain state `B(P_2,V_2,T_2)`. The work done by the gas is:
View SolutionDuring an adiabatic process, the pressure of a gas is found to be proportional to cube of its absolute temperature. The poision's ratio of gas is:
View SolutionFor a gas having molar mass `M`, specific heat at constant pressure can be given as:
View SolutionIf `20kJ` work is done by the water fall per unit volume of water and `30kJ` heat was lost per unit volume, the energy change per unit volume of water is:
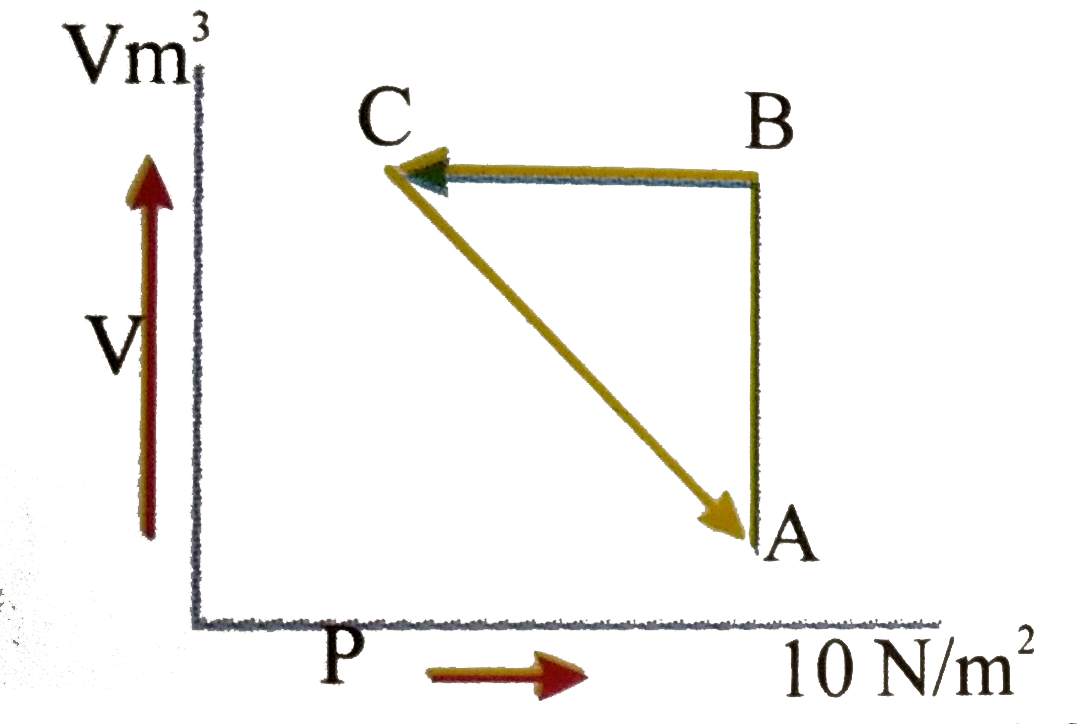
View SolutionAn ideal gas is taken through the cycle `A rarr B rarr C rarr A` As shown in figure. If net heat supplied to the gas in the cycle is 5j. Find the work done by the gas in the process `C rarr A` in Joule (taken mole value)
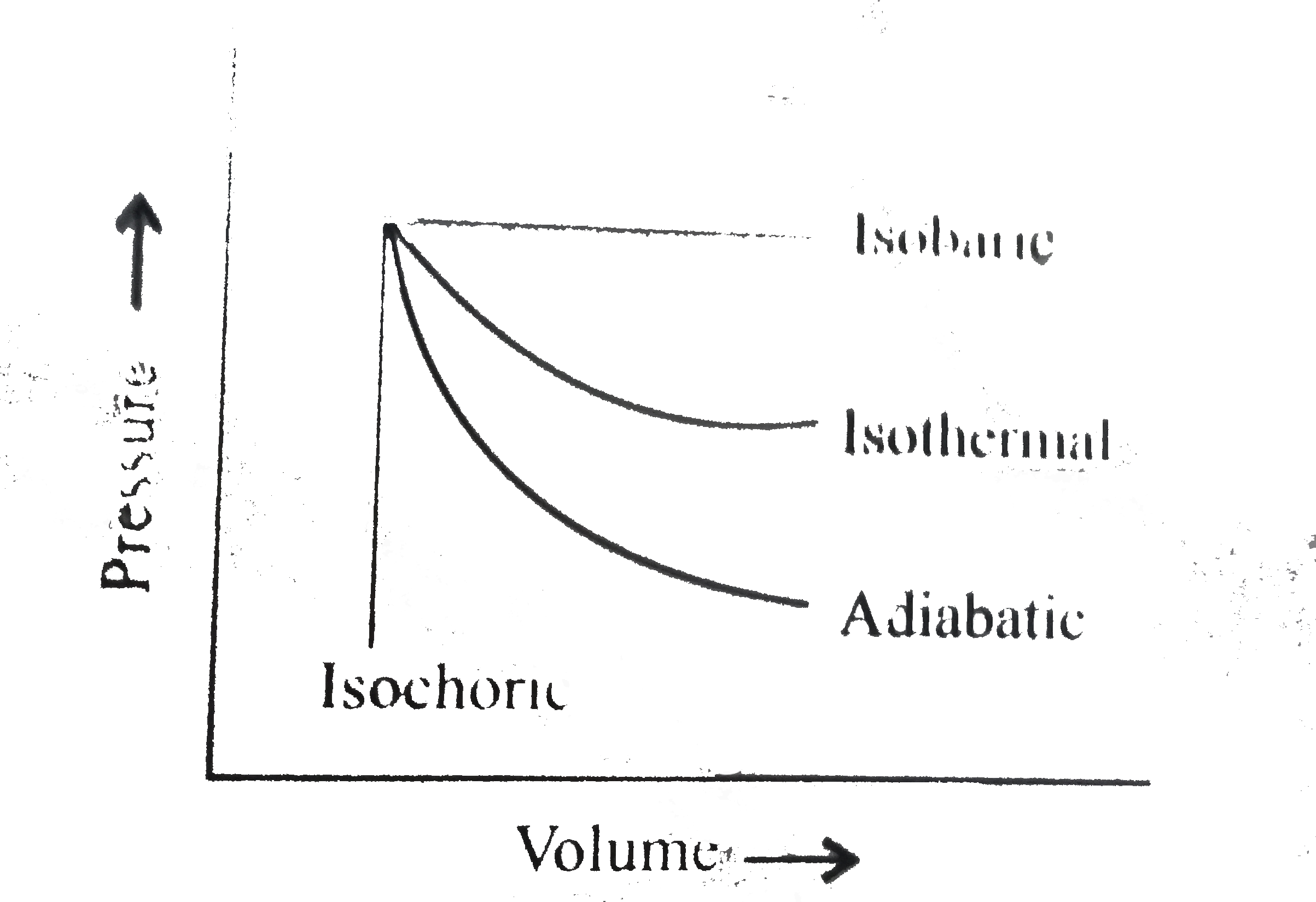
View SolutionThe pressure-volume of various thermodynamic process is shown in graphs:
Work is the mole of transference of energy. It has been observed that reversible work done by the system is the maximum obtainable work.
`w_(rev) gt w_(irr)`
The works of isothermal and adiabatic processes are different from each other.
`w_("isothermal reversible") = 2.303 nRT log_(10) ((V_(2))/(V_(1)))`
`= 2.303 nRT log_(10)((P_(2))/(P_(1)))`
`w_("adiabatic reversible") = C_(V) (T_(1)-T_(2))`
If `w_(1),w_(2),w_(3)` and `w_(4)` are work done in isothermal, adiabatic, isobaric, and isochoric reversible processes, respectively then the correct sequence (for expansion) would be
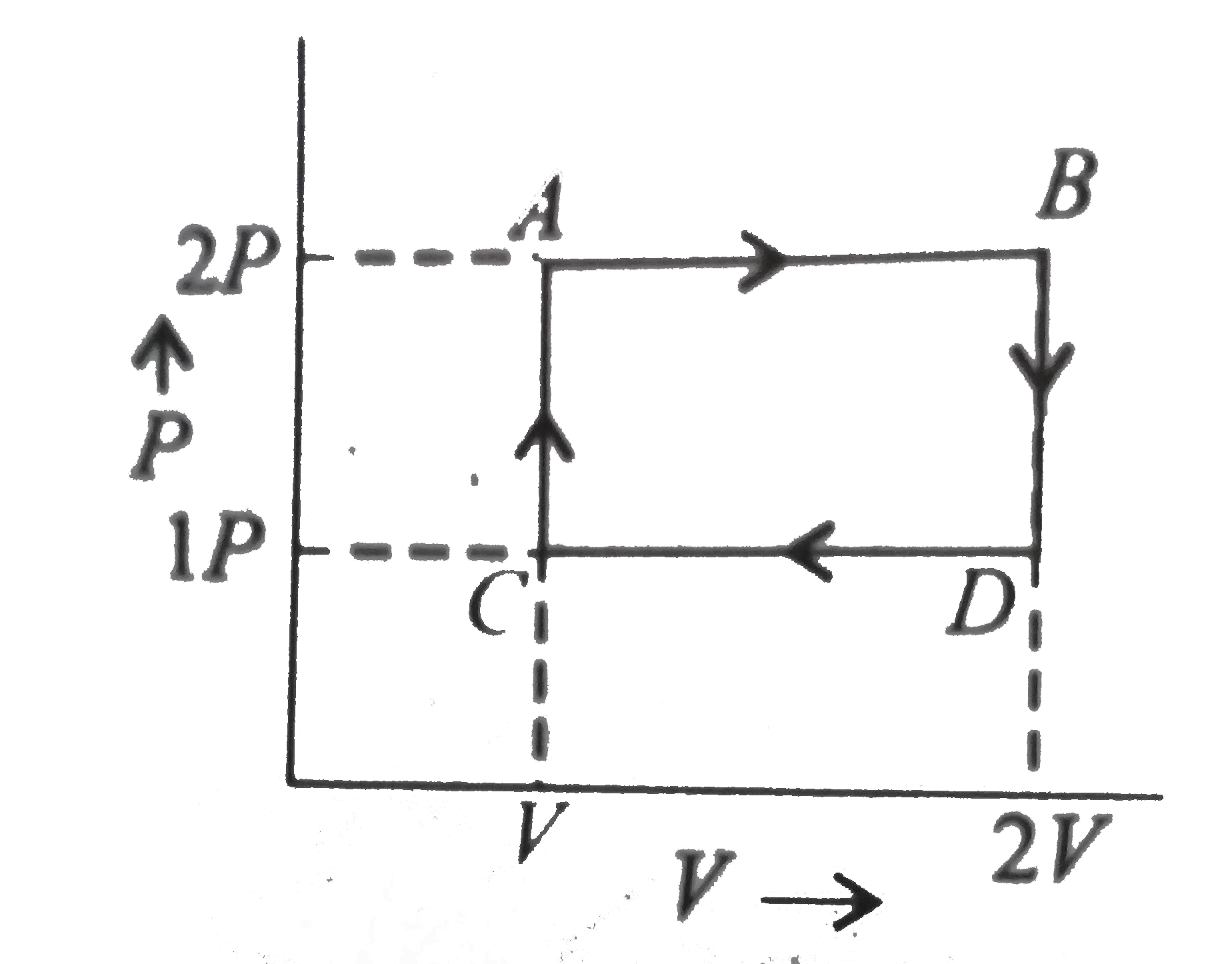
View SolutionAn ideal mono-atomic gas follows the path `ABDC`. The work done during the complete cycle is
View SolutionIn a given process on an ideal gas, `dW=0` and `dQ` is negative, then for the gas:
View SolutionWhich of the following pairs of a chemical reaction is certain to result in a spontaneous reaction ?
View SolutionOne mole of a substance is cooled at the rate of `0.4 kJ min^(-1)` as shown in the graph. Curve `AB`, points `B` and `C` and curve `CD` represent respectively, the cooling of the liquid, start of freezing, completion of freezing and cooling of the solid based on this data. The entropy of fusion in `J molK^(-1)` is :
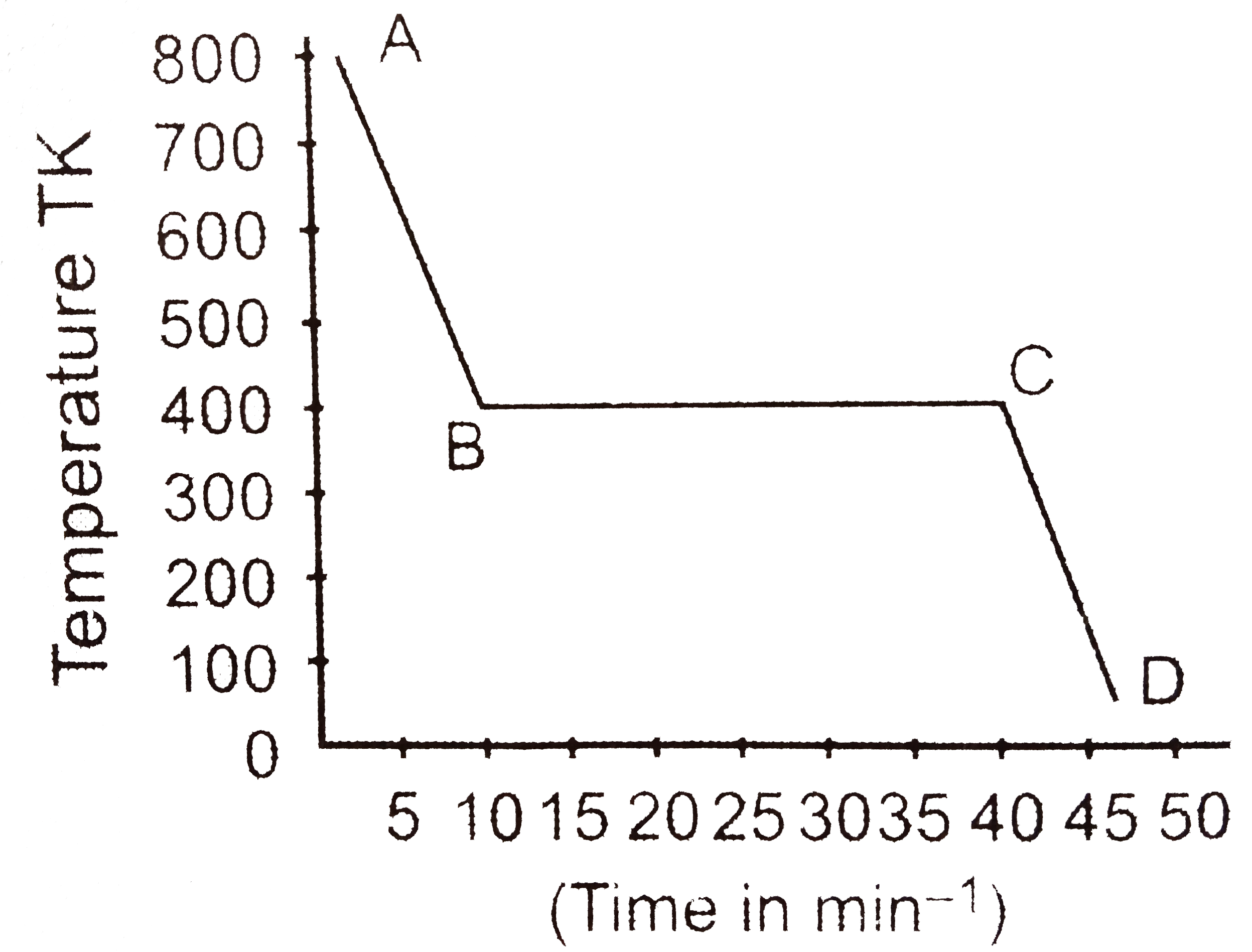
View SolutionIf a certain mass of gas is made to undergo separately adiabatic and isothermal expansions to the same pressure, starting form the same initial conditions of temperature and pressure, then, as compared to that of isothermal expansion, in the case of adiabatic expansion, the final
View SolutionIf separate samples of argon, methane, nitrogen and ammonia, all at the same initial temperature and pressure and expanded adiabatically to double their original volumes, then which one of these gases will require the greatest quantity of heat to restore the original temperature?
View SolutionThe maximum entropy of mixing occurs when hexane and heptane are mixed respectively in the proportion:
View SolutionSelect the correct statements about the following reaction:
`Fe + HCL to FeCl_2 + H_2`
1. The reaction if carried out in electrochemical cell produces non expansion work
2. The reaction if carried out in a cylinder having piston produces expansion work
3. The current flows from cathode to anode and `DeltaG^(@)` for the reaction is `-ve`
4. The current flows from anode to cathode and `DeltaG^(@)` for the reaction is -ve
View SolutionSelect the correct statements for ideal gases:
`1. ((delH)/(delT))_(P)-((delU)/(delT))_(V)=R`
`2. ((delH)/(delT))_(P)-((delU)/(delT))_(P)-((delU)/(delT))_(V)`=work done
`3. ((delU)/(delV))_(T)=+ve`
`4. ((delT)/(delP))_(H)=+ve`
View Solution`1`mole each of `CaCl_2, Al_4C_3, Mg_2C_3` reacts with excess water in separate open flasks work done during the dissolution shows the order:
View SolutionWhen a bottle of perfume is opened, odorous molecules mix with air and slowly diffuse throughout the entire room. Which is not correct for this process?
View SolutionThe Gibbs energy change and standard Gibbs energy change for a reaction are same if the reaction quotient `Q` has value equal to :
View SolutionThe standard change in Gibbs energy for the reaction: `H_2O hArr H^+ +OH^-` at `25^@C` is :
View SolutionIdentify the correct statement for change of Gibbs energy for a system `(DeltaG_(system))` at constant temperature and pressure:
View SolutionA monoatomic gas is suddenly compressed to `1//8` of its original volume adiabatically. The pressure of gas will change to :
View SolutionThe enthalpy and entropy change for the reaction,
`Br_(2)(l)+Cl_(2)(g)rarr2BrCl(g)`
are `30KJmol^(-1)` and `105JK^(-1)mol^(-1)` respectively. The temperature at which the raction will be in equilibrium is:
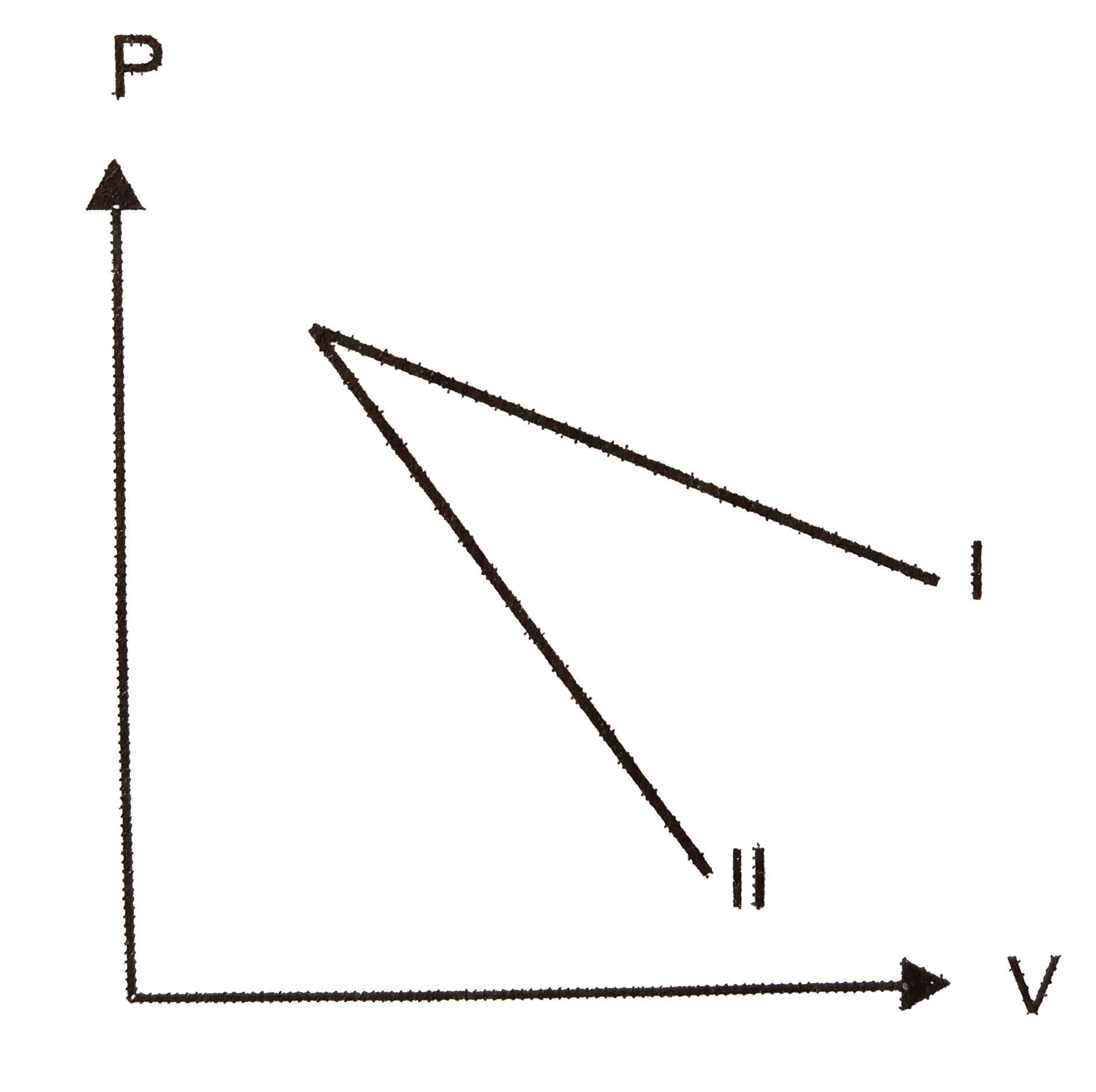
View Solution`P-V` plots for two gases during adiabatic expansion are shown in figure. Plot `I` and `II` should correspond respectively to :
View SolutionFor an adiabatic expansion of a perfect gas `dP//P` is equal to :
View SolutionThe poisson's ratio for `O_(2)` is `1.4`. Which of the following are correct for `O_(2)`?
View SolutionWhich species possesses negative value of specific heat?
View SolutionIn which process net work done is zero ?
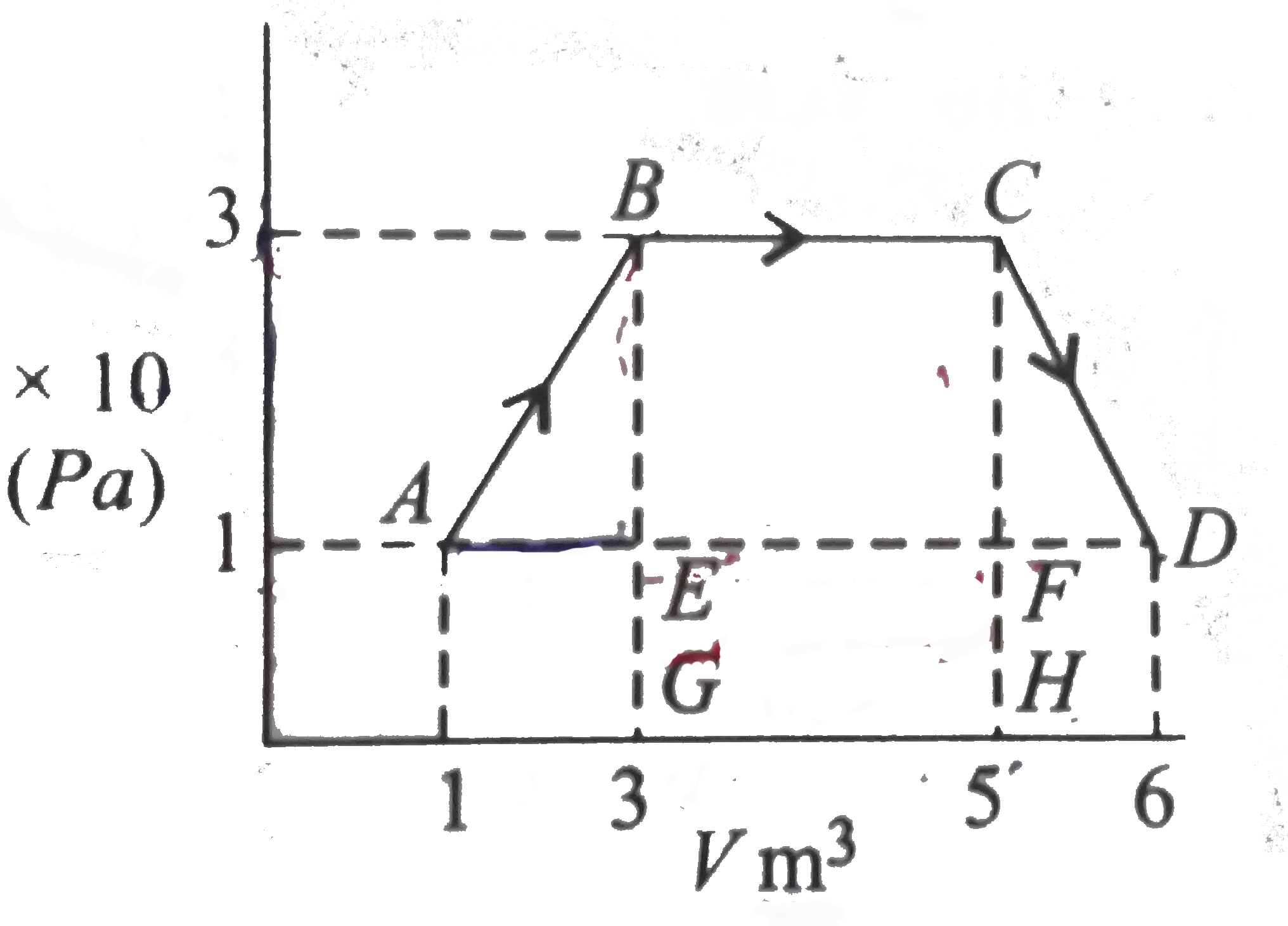
View SolutionThe net work done through a series of changes reported in figure for an ideal gas is
View SolutionSpecific heat of a substance at `m.pt.` or `b.pt.` for isothermal process and specific heat of a substance during adiabatic change respectively are:
View SolutionIf for the reaction,
`2Mg_((s)) + O_(2(g)) to 2MgO_((s)), ` at `27^@C` `Delta r H = -1202kJmol^(-1)` and `Delta r S = -217kJ^(-1) mol^(-1)`. The total entropy change `(DeltaS)_T` and Gibb's energy change during the course of reaction `(Delta r G)` are respectively:
View SolutionThe ratio of slopes of `P-V` plots for reversible adiabatic process and reversible isothermal process of an ideal gas is equal to :
View Solution`300K` a gas `(gamma = 5//3)` is compressed suddenly so that its pressure becomes `1//8` of the original pressure. The final temperature of the gas is :
View SolutionSelect the incorrect statements for the equillibrium under standard condition:
`H_(2)O_((s))hArrH_(2)O_((l)), DeltaS_(1)^(@)`
`H_(2)O_((l))hArrH_(2)O_((v)), DeltaS_(2)^(@)`
`H_(2)O_((l))hArrH_(2)O_((v)), DeltaS_(3)^(@)`
View SolutionHeat of neutralisation of strong acid and strong base under `1 atm` and `25^@C` is `-13.7 kcal`. If standard Gibbs energy change for dissociation of water to `H^+` and `OH^-` is `-19.14 kcal`, the change in standard entropy for dissociation of water is:
View SolutionThe relation between the volume and temperature of a sample of water in the range `0^(@)C` to `100^(@)C` is best represented as
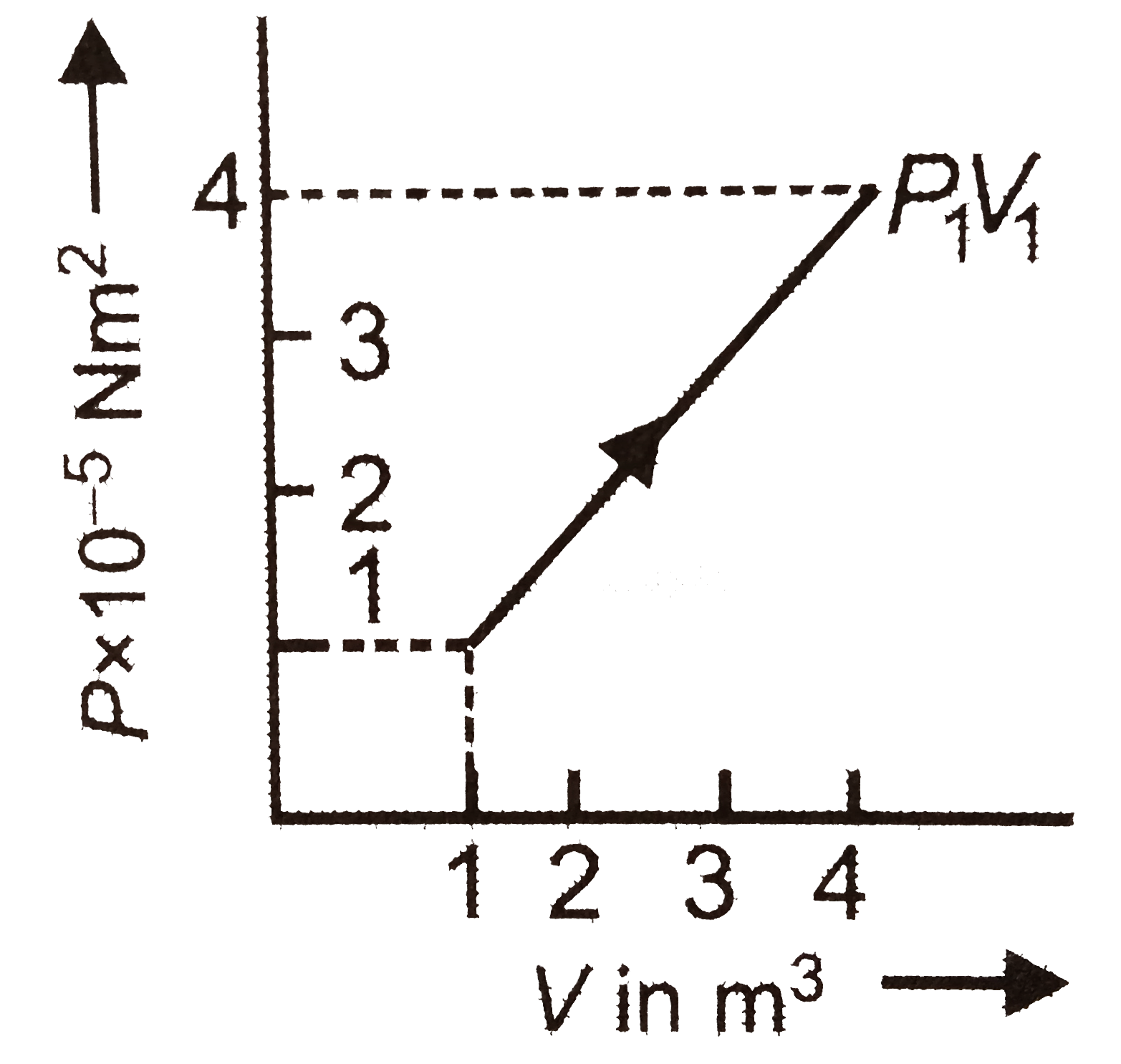
View SolutionThe work done in changing the state from `(P_0, V_0)` to `(P_1, V_1)` in the following system is :
View Solution
Exercise questions and complete solutions for chapter THERMODYNAMICS of P BAHADUR CHEMISTRY (HINGLISH) of Class 11