Complete Solutions to Practive Exercise of chapter LINEAR MOMENTUM & ITS CONSERVATION of Class 11 book with complete answers and questions
| Class 11 | PHYSICS |
| Chapter | LINEAR MOMENTUM & ITS CONSERVATION |
| PHYSICS GALAXY | Practive Exercise |
| Number Of Questions | 28 |
| Academic Year | 2023-24 |
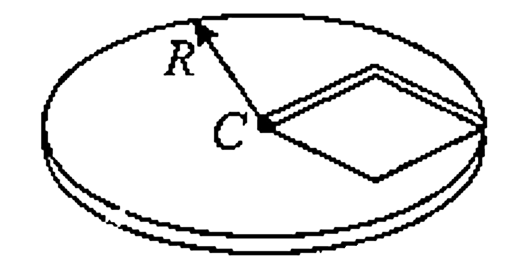
Find the centre of mass of the disc shown in figure. . A square is removed from the disc as shown
View SolutionFrom a gun whose barrel is inclined at an angle `theta` to horizontal fires 3 small balls of mass 2 kg, 5 kg and 4 kg which move in different directions as shown in figure. It is given that the centre of mass of the three shells moes in the direction of barrel of gun find `theta`.
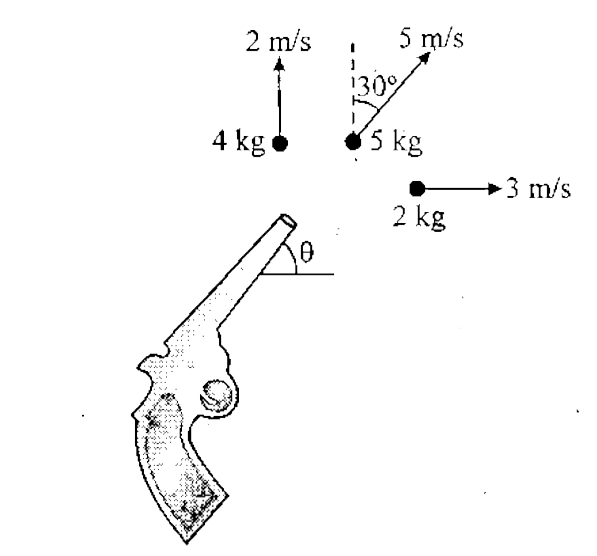
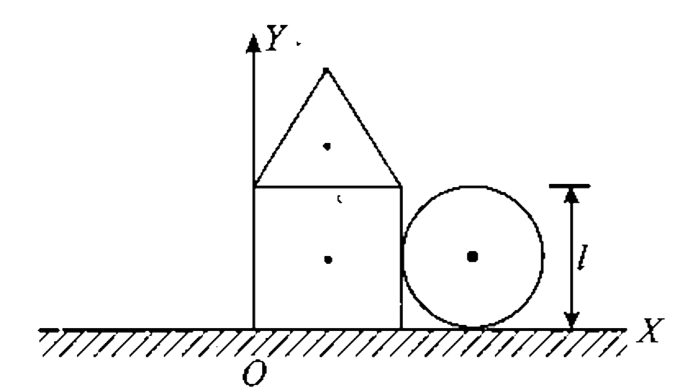
View SolutionThree laminar bojects of uniform density a square, a disc and an equilateral triagle are placed as shown fig. find the coordinates of centre of mass of the three bodies.
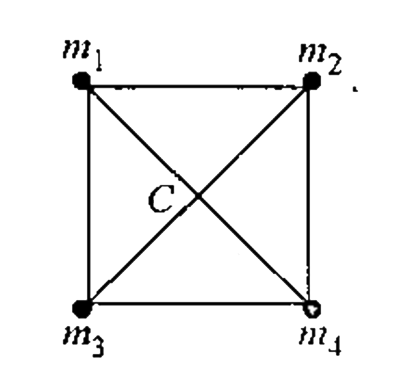
View SolutionFour particle of masses `m_(1)=2kg,m_2=4kg,m_(3)=1kg` and `m_(4)` are placed at four corners of a square as shown in figure. Can mass of `m_(4)` be adjusted I such a way that the centre of mass of system will be at the centre of the square C.
View SolutionFind x and y coordinats of the centre of mass of the plate shown in figure from which a square of side 2 m is cut out.
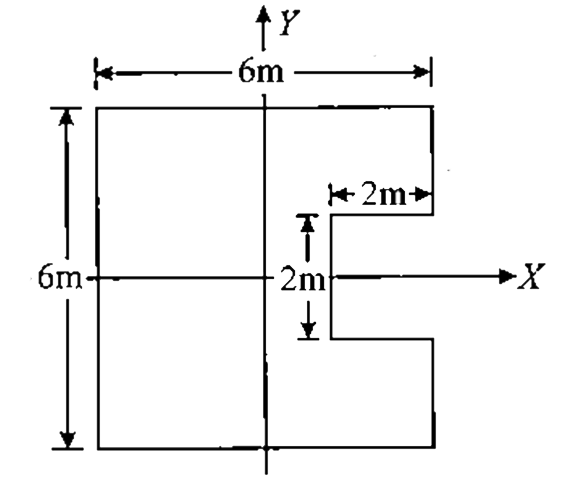
View SolutionFind centre of mass distance from point O of the uniiform circualr arc shown in figure.
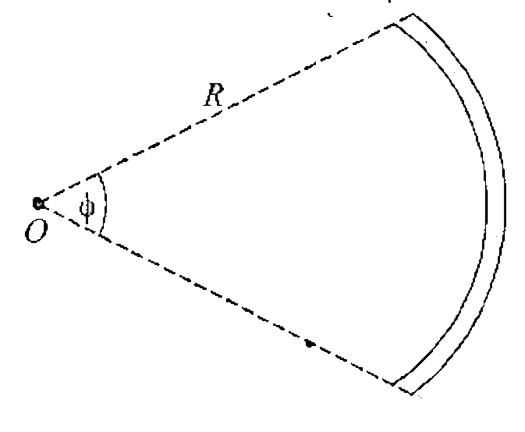
View SolutionMass Center of a sector of a uniform circular plate
Find location of mass center of a sector of a thin uniform plate.
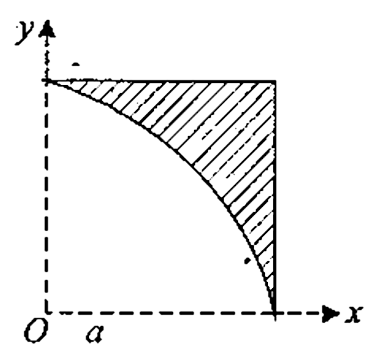
View SolutionFron a sqaure plate of side a,a quarter circular disc of radius a is removed as shown in figure. Find the coordinates of centre of mass of the remaining part.
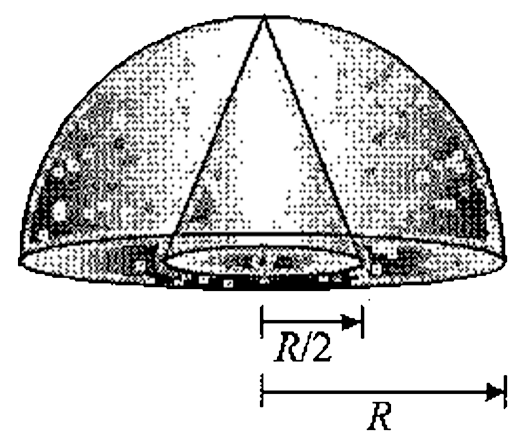
View SolutionFrom a hemisphere of radius R a cone of base radius R/w and height R is cut as shown in figure. Find the height of centre of mass of the remaining object.
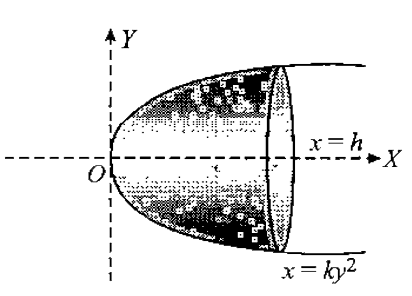
View SolutionFind centre of mass of an object (Paraboloid) which is formed by rotating a parabola `x=ky^(2)` about x-axis and height of object is h as shown in figure. Assume the object is of uniform density.
View SolutionIn a boat of mass 4 M and length l on a frictionless water surface. Two men A (mass=M0 and B(mass 2 M) are standing on the two opposite ends. Now A travels a dsitance l/4 relative to boat towards its centre and B moves a distance 3l/4 relative to boat and meet A. find the distance travelled by the boat on water till A and B meet.
View SolutionA shell of mass m is fired from a gun of mass km which can reecoil freely on a horizontal plane, the elevation of the gun is `45^(@)`. Find the ratio of te energy of the shell to that of the gun.
View SolutionA gun in mounted on a railroad car. Themass of the car with all of its components is 80 m mass of each shell to be fired is 5m. The muzzle velocity of the shells is 100m/s horizontally, what is the recoil speed of the car after seconds shot? Consider car to be at rest initially.
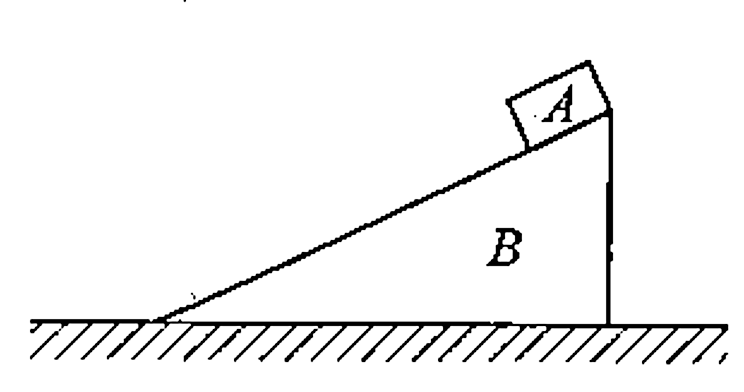
View SolutionA block A (mass 4M) is placed on the top of a wedge block B of base length l (mass=20M) as shown in figure. When the system is released from rest. Find the distance moved by the block B till the block A reaches ground Assume all surfaces are frictionless.
View SolutionA space shuttle of mass M, moving at 4000 kph relative to earth ejects a capsule backward of mass M/5. if speed of ejection of capsule is 120 kph relative to state of shuttle before ejection, find the final velocity of the shuttle.
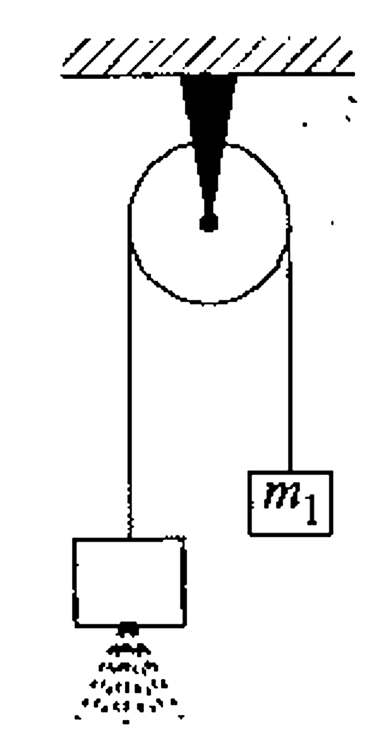
View SolutionA mass `m_(1)` is connected by a weghtless cable of water, whose mass is `m_(0)` at `t=0`. If the container efects water in downward directiona t a constant rate bkg/s. with a velocity `v_(0)` relative to the container, determine the acceleration of `m_(1)` as a function of time.
View SolutionA rocket with initial mass M is launched by emitting, gas wil velocity `v_(0)` (relative to the rocket body) downwards. The mass of the gas emitted per seconds is k. (k is constant and obeys `kv_(0) lt Mg`). Find time when rocket start to lift.
View SolutionA double stage rocket has an initial mass `M_(i)`, gas is exhausted front he rocket at a constant rate of dm/dt and with an exhaust velocity u relative to the rocket. When the mass of the rocket reaches the value `mu`, the first stage of mass m of
Which fuel is exhausted is disengaged from the rocket and then the rocket continues to the second stage at teh same rate and exhaust velocity as int eh first stage, until it reaches a mass `M_(f)`
(a). Calculate the rocket velocity at the end of the first stage, given that it is started at rest.
(b). Calculat ethe rocket velocity at the end of the second stage. ltbr. (c). What is the final velocity of a one stage rocket of the same initial `M_(i)` mass and the same amount of fuel ? it is greater or less than final velocity of the double stage rocket?
View SolutionA particle of mass M is initially at rest starts moving under the action of a constant force `Fhati`. It encounters the resistance of a stream of fine dust movingwith velocity `-v_(0)hati`, which deposits matter on it at a constant rate `rho`, show that its mass will be m when it has travelled a distance-
View SolutionA balloon having mass `m` is filled with gas and is held in hands of a boy. Then suddenly it gets released and gas starts coming out of it with a constant rate. The velocity of the ejected gas is `2m//s` with respect to the balloon. Find out the velocity of the balloon when the mass of gas is reduced to half :-
View SolutionA railroad car of length L aned mass `m_(0)` when empty is moving freely on a horizontal track while being loaded with sand from a stationary chute at a rate dm/dt=q. knowing that the car was approaching the chute a speed `v_(0)`, determine
(a). The mass of the car and its load after the car has cleared the chute.
(b). The speed of the car at that time.

View SolutionA piece of wood of mass 0.03 kg is dropped from the top of a building 100 m high. At the same time, a bullet of mass 0.02 kg is fired vertically upward with a velocity of 100 m/s from the ground the bullet gets embedded in the wooded piece after striking. find the height to which the combination rises above the building before it starts falling take `g=10m//s^(2)`
View SolutionTwo balls A and B having different but unknown masses, collide elastically. A is initially at rest when B has a speed v. After collisio B has a speed v/2 and moves at right angles to its original motion. (a) find the direction in which ball A moves after collision (b) Determine the speed of A.
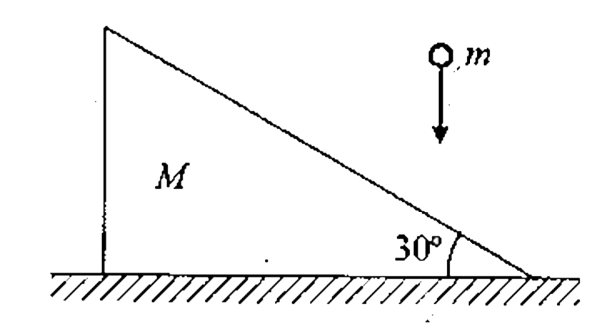
View SolutionA ball of mass m=1kg falling vertically with a velocity `v_(0)=2m//s` strikes a wedge of mass M=2kg kept o a smooth, horizontal surface as shown in figure. The coefficient of resitution between the ball and the wege is `e=1//2`. Find the velocity of the wedge is e=1/2. find the velocity of the wedge and the ball immediately after collison.
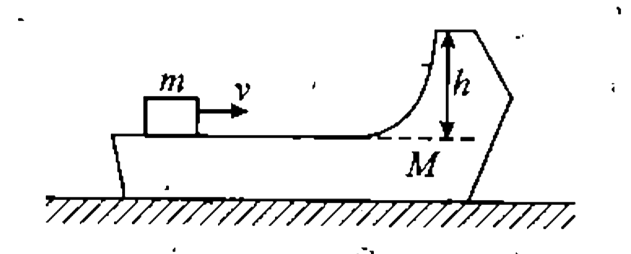
View SolutionA body of mass M with a small box of mass m placed on it rests on a smooth horizontal surface. The box is set in motion in the horizontal direction with a velocity u as shown in figure. To what height relative to the initial level will the box rise after breaking off from the body M? Assume all surfaces are frictionless.
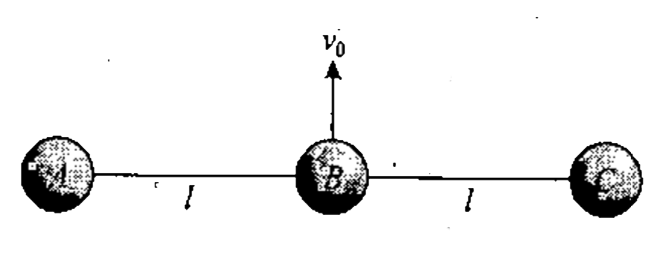
View SolutionThree identical balls eah of mass m=0.5 kg are connected with each other as shown in figure and rest over a smooth horizontal table At moment t=0, ball B is imparted a horizontal velocity `v_(0)=9ms^(-1)` calculate velocity of A just before it collides with ball C.
View SolutionTwo particle, each of mass m, are connected by a light inextensible string of length 2l. Initially they lie on a smooth horizontal table at points A ad B distant l apart the particle at A is projectd across the table with velocity u. find th speed with which the second particle begins to move if the direction. of u is :
(a). along BA
(b). at an angle of `120^(@)` with AB
(c) perpendicular to AB
In each case also calculate (in terms of ma nd u) the impulsive tension in the string.
View SolutionA sphere of mass `m_(1)` in motion hits directly another sphere of mass `m_(2)` at rest and sticks to it, the total kinetic energy after collision is `2//3` of their total K.E. before collision. Find the ratio of `m_(1) : m_(2)`.
View Solution
Practive Exercise questions and complete solutions for chapter LINEAR MOMENTUM & ITS CONSERVATION of GK PUBLICATIONS PHYSICS (HINGLISH) of Class 11