Complete Solutions to Numerical MCQ s of chapter LINEAR MOMENTUM & ITS CONSERVATION of Class 11 book with complete answers and questions
| Class 11 | PHYSICS |
| Chapter | LINEAR MOMENTUM & ITS CONSERVATION |
| PHYSICS GALAXY | Numerical MCQ s |
| Number Of Questions | 75 |
| Academic Year | 2023-24 |
Three particles of the same mass lie in the x-y plane. The (x,y) coordinates of their positions are (1,1),(2,2) and (3,3) respectively. The (x-y) coordinates of the centre of mass are:
View SolutionIn the `HCI` molecule, the separation between the nuclei of the two atoms is about `1.27Å (1Å = 10^(-10)m)`. Find the approximate location of the c.m of the molecule, given that a chlorine atom is about `35.5` times as massive as a hydrogen atom and nearly all the mass of an atom is concentrated in its nucleus ?
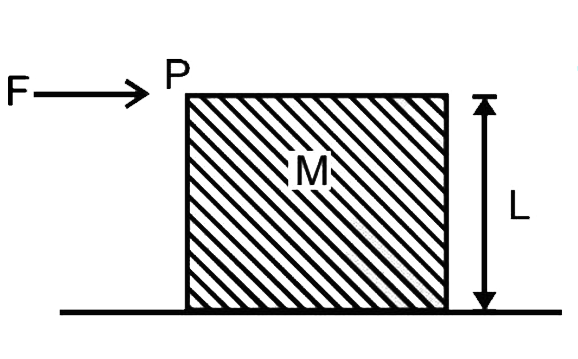
View SolutionA cubical block of side L rests on a rough horizonta surface with coefficient of friction `mu`. A horizontal force F is applied on the block as shown. If the coefficient of friction is sufficiently high so that the block does not slide before toppling, the minimum force required to topple the block is
View SolutionA boy of mass m stands on one end of a wooden plank of length L and mass M. The plank is floating on water. If the boy walks from one end of the plank to the other end at a constant speed, the resulting displacement of the plank is given by :
View SolutionA neutron moving at a speed v undergoes a head-on elastic collision with a nucleus of mass number A at rest. The ratio of the kinetic energies of the neutron after and before collision is :
View SolutionA wooden block of mass `0.9 kg` is suspended from the ceiling of a room by thin wires. A bullet of mass `0.1 kg` moving horizontally with a speed of `10 ms^(-1)` strikes the block and sticks to it. What is the height to which the block rises ? Take `g = 10 ms^(-2)`:
View SolutionIn what is the loss in kinetic energy of the system due to impact ?
View SolutionA shell of mass 2m fired with a speed u at an angle `theta` to the horizontal explodes at the highest point of its trajectory into two fragments of mass m each. If one fragment falls vertically, the distance at which the other fragment falls from the gun is given by :
View SolutionA rubber ball is dropped from a height of `5m `on a plane, where the acceleration due to gravity is not shown. On bouncing it rises to `1.8 m.` The ball loses its velocity on bouncing by a factor of
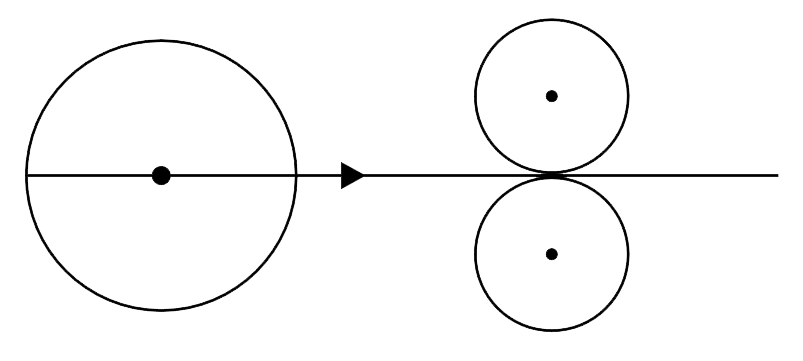
View SolutionTwo identical discs are initially at rest in contact on a horizontal table. A third disc of same mass but of double radius strikes them symmetrically and comes to rest after the impact.
(a) Find the coefficient of restitution for the impact.
(b) Find the minimun kinetic energy of the system (as a percentage of original kinetic energy before collision) during the process of collision.
Treat the collision to be instantaneous
View SolutionA neutron having a mass of `1.67 xx 10^(-27) kg` and moving at `10^(8) m//s`collides with a deuteron at rest and sticks to it. If the mass of the deuteron is `3.33 xx 10^(-27) kg` then the speed of the combination is
View SolutionIn , if the collision were perfectly elastic, what would be the speed of deuteron after the collision ?
View SolutionA loaded spring gun of mass M fires a bullet of mass m with a velocity v at an angle of elevation `theta`. The gun is initially at rest on a horizontal smooth surface. After firing, the centre of mass of the gun and bullet system
View SolutionA shell of mass m is at rest initially. It explodes into three fragments having masses in the ratio `2:2: 1.` The fragments having equal masses fly off along mutually perpendicular directions with speed v. What will be the speed of the third (lighter) fragment ?
View SolutionA ball P of mass `2kg` undergoes an elastic collision with another ball Q at rest. After collision, ball P continues to move in its original direction with a speed one-fourth of its original speed. What is the mass of ball Q ?
View Solution(a) A rocket set for vertical firing weighs `50kg` and contains `450kg` of fuel. It can have a maximum exhaust velocity of `2km//s`. What should be its minimum rate of fuel consumption
(i) to just lift off the launching pad?
(ii) to give it an initial acceleration of `20m//s^2`?
(b) What will be the speed of the rocket when the rate of consumption of fuel is `10kg//s` after whole of the fuel is consumed? (Take `g=9.8m//s^2`)
View Solution(a) A rocket set for vertical firing weighs `50kg` and contains `450kg` of fuel. It can have a maximum exhaust velocity of `2km//s`. What should be its minimum rate of fuel consumption
(i) to just lift off the launching pad?
(ii) to give it an initial acceleration of `20m//s^2`?
(b) What will be the speed of the rocket when the rate of consumption of fuel is `10kg//s` after whole of the fuel is consumed? (Take `g=9.8m//s^2`)
View SolutionA bullet of mass `50 kg` is fired by a gun of mass `5 kg` .If the muzzle speed of the bullet is`200 ms^(-1)`, what is the recoil speed of the gun?
View SolutionA particle is projected with a velocity `200 m//s` at an angle of `60^(@).` At the highest point it explodes into three particle of equal masses. One goes vertically upward with a veloctiy `100m//s` the second particle goes vertically downwards at same speed. What is the velocity of the third particle?
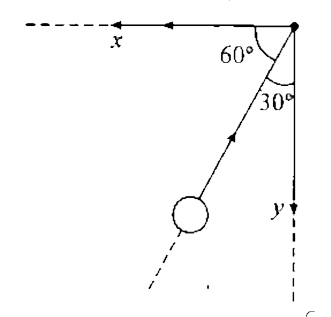
View SolutionA hockey player receives a corner shot at a speed of `15m//s` at an angle of `30^(@)` with the y-axis and then shoots the ball along the x-axis with a speed of `30m//s` If the mass of the ball is `100gm` and it remains in contact with the hockey stick for `0.01s`, the force imparted to the ball in the x-direction is :
View SolutionA shell is fired from a cannot with a speed of `100ms^(-1)` at an anlge `60^(@)` with the horizontal (x-direction). At the highest point of its trajectory the shell explodes into two equal fragments. One of the fragments moves along the negative x-direction with a speed of `50 ms^(-1).` What is the speed of the other fragment at the time of explosion ?
View SolutionFour particles of masses `1kg ,2kg, 3kg` and `4kg` are placed at the corners of a square of side 2m in the x-y plane If the origin of the co-ordinate system is taken at the mass of `1kg,` the (x,y) co-ordinates of the enter of mass expressed in metre are :
View SolutionA child is standing at one end of a long trolley moving with a speed v on a smooth horizontal track. If the child starts running towards the other end of the trolley with a speed u, the centre of mass of the system (trolley + child) will move with a speed :
View SolutionA blacksmith carries a hammer on his shoulder and holds in at the other end of its light handle in his hand. If he changes the point of support of the handle and x is the distance between the point of support and his hand , then the pressure on his hand varies with x as :
View SolutionA ball of mass m moving with a velocity v undergoes an oblique elastic collision with another ball of the same mass m but at rest. After the collision if the two balls move with the same speeds , the angle between their directions of motion will be:
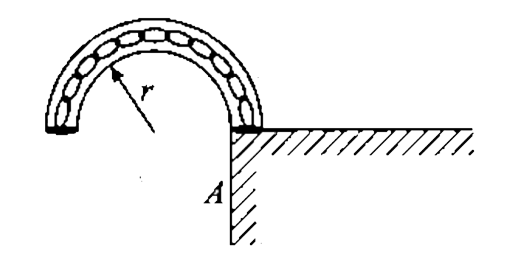
View SolutionA smooth semicircular tube AB of radius r is fixed in a vertical plane and contains a heavy flexible chain of length `pir` and weight `Wpir` as shown. Assuming a slight disturbance to start the chain in motion, the velocity v with it will emerge from the open end B of the tube is:
View SolutionA ball of mass m moving horizontally at a speed v collides with the bob of a simple pendulum at rest. The mass of the bob is also m. If the collision is perfectly inelastic and both balls sticks, the height to which the two balls rise after the collision will be given by:
View SolutionIf a man of ,mass M jumps to the ground from a height h and his centre of mass noves a distance x in the time taken by him to 'hit' the ground the average force acting on him (assuming his retardation to be constant during his impact with the ground) is :
View SolutionA radioactive nucleus of mass number A, initially at rest, emits an `alpha-` particle with a speed v. What will be the recoil speed of the daughter nucleus ?
View SolutionA cart of mass `M` is tied to one end of a massless rope of length `10 m`. The other end of the rope is in the hands of a man of mass `M`. The entire system is on a smooth horizontal surface. The man is at `x=0` and the cart at `x=10 m`. If the man pulls the cart by the rope, the man and the cart will meet at the point
View SolutionA bomb at rest explodes into three fragments of equal massses Two fragments fly off at right angles to each other with velocities of `9m//s` and `12//s` Calculate the speed of the third fragment .
View SolutionFour particles of masses m,m 2 m and 2m are placed at the four corner of a squre of side `"a"` as shown in figure -4. 107. The (x,y) coordinates of the centre of mass are:
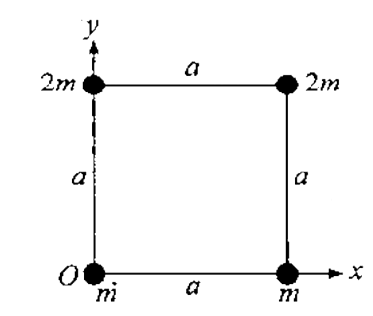
View SolutionA small coin is placed at a distance r from the centre of a gramophone record.The rotational speed of the record is gradually increased. If the coefficient of friction between the coin and the record is `mu,` the minimum angular frequency of the record for which the coin will fly off is given by :
View SolutionSphere A of mass 'm' moving with a constant velocity u hits another stationary sphere B of the same mass. If e is the co-efficient of restitution, then ratio of velocities of the two spheres `v_(A):v_(B)` after collision will be :
View SolutionA smooth sphere is moving on a horizontal surface with velocity vector `3hati+hatj` immediately before it hits a vertical wall. The wall is parallel to the vector `hatj` and the coefficient of restitution between the wall and the sphere is `1//3`. The velocity vector of the sphere after it hits the wall is :
View SolutionFrom a point on smooth floor of a room a toy ball is shot to hit a wall. The ball then returns back to the point of projection. If the time taken by ball in returining is twice the time taken in reaching the wall find the coefficient of restitution:
View SolutionIn the figure shown, the heacvy ball of mass 2m rests on the horizontal surface and the lighter balls of mass m is dropped from a height `hgt2l.` At the instant the string gets taut, the upward velocity of the heavy ball will be :
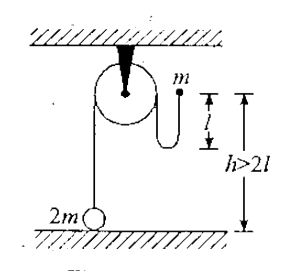
View SolutionA shell is fired from a connot with a velocity V at an angle 0 with the horizontal direction .At the highest point in its path it explodes into two pieces of equal masses. One of the pieces retraces its path to the cannon.The speed of the other piece immediately after the explosion is :
View SolutionA bullet moving with a velcity u passes through a plank which is free to move. The two are of equal mass. After pssing through the plank, the velocity of the bullet becomes `fu`. Its velocity relative to the plank now is
View SolutionA particle of mass `m_(1)` makes an elastic one dimensional collision with a stationary particle of mass `m_(2)`.What fraction of the kinetic energy of `m_(1)` is carried away by `m_(2)`?
View SolutionTwo balls with masses in the ratio of 1:2 moving in opposite direction have a head-on elastic collision. If their velocities before impact were in the ratio of 3:1, then velocities after impact will have the ratio :
View SolutionTwo identical balls of equal masses A and B , are lying on a smooth surface as shown in figure Ball A hits the ball B (which is at rest) with a velocity v=16`m//s` What should be the minimum value of coefficient of restitution e beteeen A and B so that B just reaches the highest point of inclined plane:
`("Take" g=10 m//s^(2))`
View SolutionTwo blocks of mass `m_(1)"and"m_(2)` are connected by light inextensible string passing over a smooth fixed pulleuy of negligible mass. The acceleration of the centre of mass of the system when blocks move under gravity is :
View SolutionA girl throws a ball with an initial velocity v at an angle `45^@`. The ball strikes a smooth vertical wall at a horizontal distance d from the girl and after rebound returns to her hands. What is the coefficient of restitution between wall and ball ? `(v^2 gt dg)`
View SolutionA small block of mass m is pushed towards a movable wedge of mass `etam` and height h with initial velocity u.All surface are smooth. The minimum value of u for which the block will reach the top of the wedge:
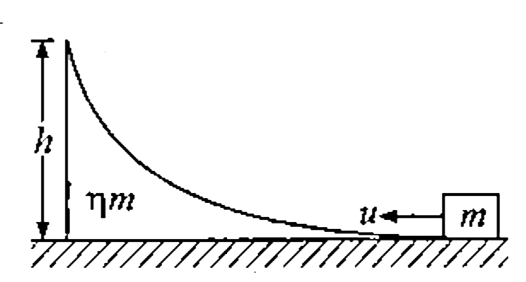
View SolutionA heavy ring fo mass m is clamped on the periphery of a light circular disc.A small particle having equal mass is clamped at the centre of the disc. The system is rotated in such a way that the centre of mass moves in a circle of radius r with a uniform speed v. We conclude that an external forec :
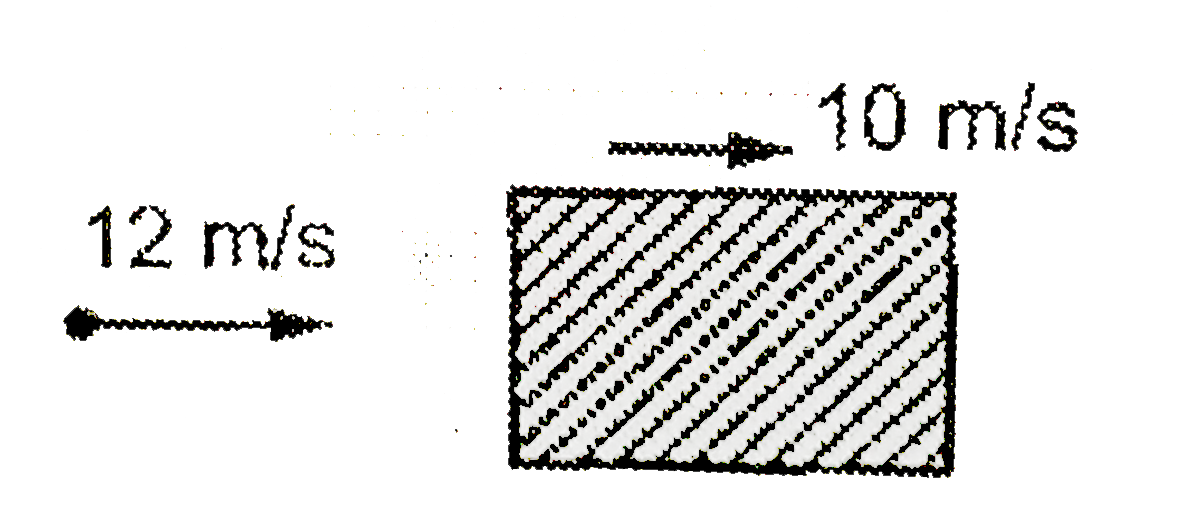
View SolutionA light particle moving horizontally with a speed of `12 m//s` strikes a very heavy block moving in the same direction at `10 m//s`. The collision is one-dimensional and elastic. After the collision, the particle will
View SolutionTwo particles of masses `m_(1)` and `m_(2)` in projectile motion have velocities `vec(v)_(1)` and `vec(v)_(2)` , respectively , at time `t = 0`. They collide at time `t_(0)`. Their velocities become `vec(v')_(1)` and `vec(v')_(2)` at time ` 2 t_(0)` while still moving in air. The value of `|(m_(1) vec(v')_(1) + m_(2) vec(v')_(2)) - (m_(1) vec(v)_(1) + m_(2) vec(v)_(2))|`
View SolutionA chain of length l is placed on a smooth spherical surface of radius R with one of its ends fixed at the top of the sphere. What will be the acceleration w of each element of the chain when its upper end is released? It is assumed that the length of the chain `llt1/2piR`.
View SolutionA ball released from a height ho above a horizontal surface rebounds to a height `h_(1)`, after one bounce. The graph that relates `h_(0)` to `h_(1)` is shown Fig. If the ball (of the mass `m`) was dropped from an initial height `h` and made three bounces, the kinetic energy of the ball immediately after the third impact with the surface was

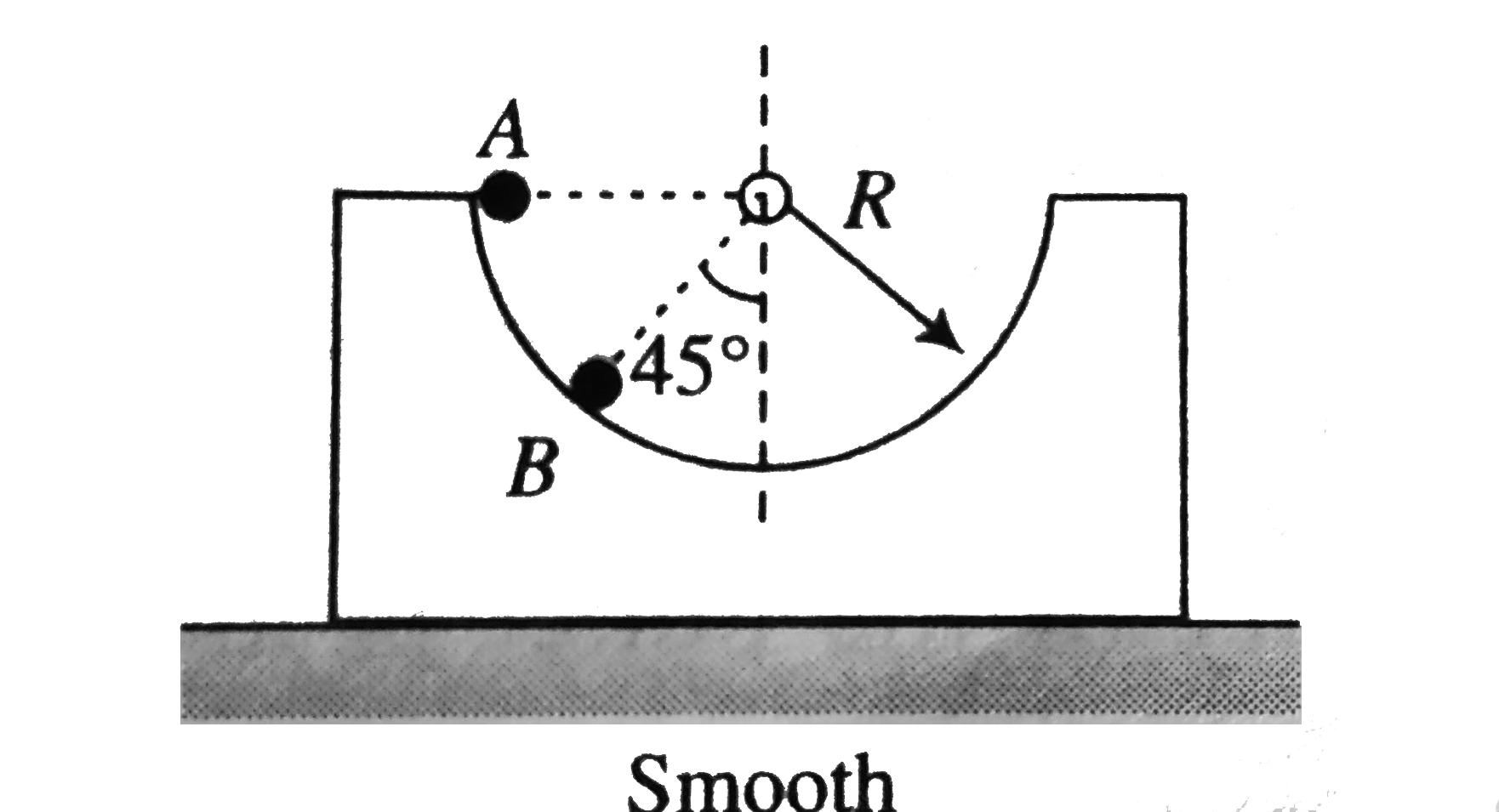
View SolutionA ball of mass `m` is released from A inside a smooth wedge of mass `m` as shown in figure. What is the speed of the wedge when the ball reaches point B?
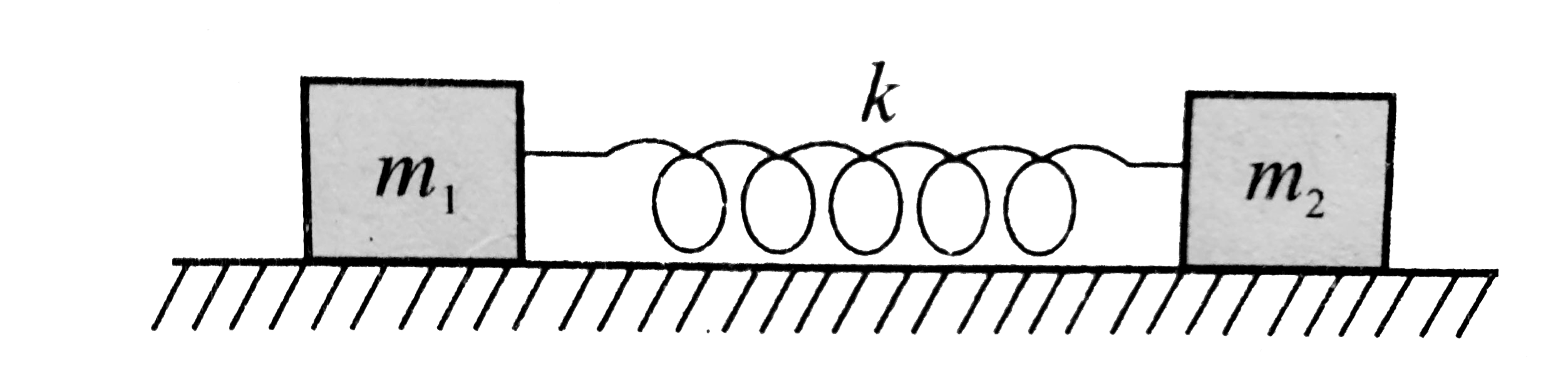
View SolutionTwo blocks `m_(1)` and `m_(2)` are pulled on a smooth horizontal surface, and are joined together with a spring of stiffness `k` as shown in Fig. Suddenly, block `m_(2)` receives a horizontal velocity `v_(0)`, then the maximum extension `x_(m)` in the spring is
View SolutionThe centre of mass of a, non uniform rod of length L whose mass per unit length p varies as p =`(kx^(2))/(L)`where k: is a constant and x is the distance of any point from one end, is (from the same end):
View SolutionTwo objects move in the same direction in a straight line. One moves with a constant velocity `V_(1)`.The other starts at rest and has constant acceleration a. They collide when the second object has velocity `2V_(1).`The distance between the two objects when the second one starts moving is :
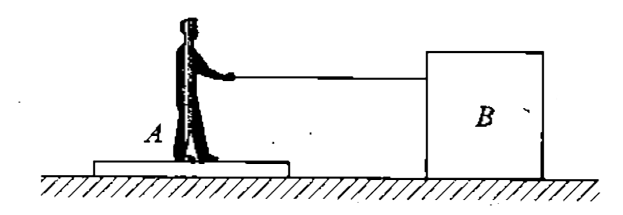
View SolutionFigure shows a boy on a horizontal platform A on a smooth horizontal surface, holding a rope attached to a box B. Boy pulls the rope with a constant force of 50 N. The combined mass of platform A and boy is `250kg` and that' of box B is `500kg.` The velocity of A relative to the box B 5 s after the boy on A begins to pull ,the rope,will be:
View SolutionA wind - powered generator convets and energy into electrical energy . Assume that the generator convents a fixed fraction of the wind energy intercepited by to blades into electrical energy for wind speed `V` , the electrical power output will be propertional to
View SolutionA ball falls vertically onto a floor with momentum `p`, and then bounces repeatedly. If the coefficient of restitution is `e`, then the total momentum imparted by the ball on the floor till the ball comes to rest is
View SolutionA projectile of mass `m` is fired with a velocity `v` from point P at an angle `45^@`. Neglecting air resistance, the magnitude of the change in momentum leaving the point P and arriving at Q is

View SolutionTwo balls of equal mass have a head-on collision with speed `6 m//s`. If the coefficient of restitution is `(1)/(3)`, find the speed of each ball after impact in `m//s`
View SolutionAn alpha particle collides elastically with a stationary nucleus and continues on at an angleof `60^(@)` with respect to the original direction of motion. The nucleus recoils at an angle of `30^(@)` with respect to this direction. Mass number of nucleus is
View SolutionA uniform solid right circular cone of base radius R is joined to a uniform solid hemisphere of radius R and of the same density, so as to have a common face. The centre of mass of the composite solid lies on the common face. The height of the cone is:
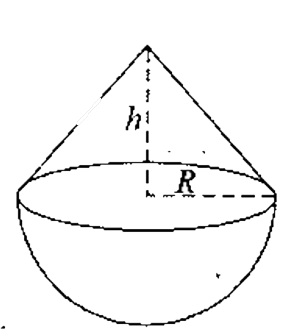
View SolutionA Sphere moving with velocity v strikes a wall moving towards the sphere with a velocity u. If the mass of the wall is infinitely large the work done by the wall during collision will be:
View SolutionA projectile of mass 20 kg is fired with a velocity of `400m//s`at an angle of `45^(@)` with the horizontal .At the highest point of the trajectocy the projectile explodes into two fragments of equal mass,one of which falls vertically downward with zero initial speed, The distance of the point where the other fragment falls from the point offiring is:
View SolutionA ball falls vertically for 2 seconds and hits a plane inclined at `30^(@)` to horizon. If the coefficient of restitution is `3/4,` find the time that elapses before it again hits the plane :
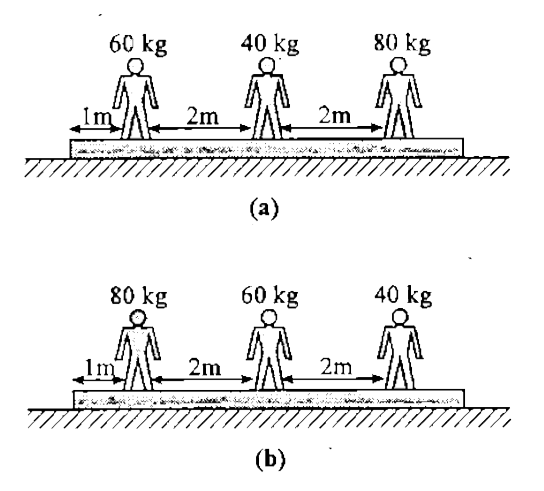
View SolutionThree boys are standing on a horizontal platform of mass 170kg as shown in figure. The exchange their position as shown inthe figure. Distance moved by the platform is:
View SolutionA platform of infinite mass is moving upward with velocity `5 m//s.` At time t = 0, a ball which is at height 100 m above the platform starts falling freely. The velocity of ball just after the collision will be (Assume elastic collision) `(g=10 m//s^(2)):`
View SolutionA force exerts an impulse Ion a particle changing its speed from initial velocity `u` to final velocity `2u`. The applied force and the initial velocity are oppositely oriented along the same line. The work done by the force is
View SolutionWhat is the maximum off set that one can obtained by piling up three identical bricks of length l :
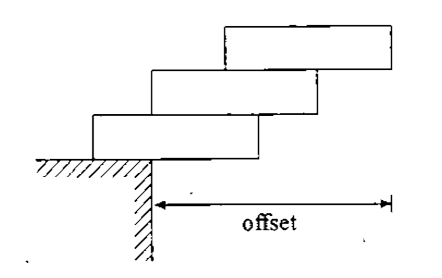
View SolutionWhen the KE of a particle is increased by 300%, the momentum of the body si increased by :
View SolutionTwo astronauts, each of mass `75kg` are floating next to each other in space, outside the space shuttle one of them pushes the other through a distance of 1m (an arms length) with a force of `300N.` What is the final relative velocity of the two ?
View SolutionA stream of water droplets, each of mass m = 0.001kg are fired horizontally at a velocity of `10m//s` towards a vertical steel plate where they collide. The droplets one spaced equidistant with a spacing of `1cm.`What is approximate average force exerted on the plate by the water droplets. (Assuming that they do not rebound after collision.)
View SolutionA bowler tbrows a ball horizontally along east direction with speed of `144"km"//"hr".` The batsman hits the ball such that it deviates from its initial direction of motion by `74^(@)` north of east direction, without changing its speed. If mass of the ball is `1/3 kg` and time of contact between bat and ball is 0.02 s. Average force applied by batsman on ball is:
View SolutionA small ball rolls off the top landing of a staircase. It strikes the mid point of the first step and then mid point of the second step. The steps are smooth & identical in height & width. The coefficient of restitution between the ball & the first step is :
View SolutionAB and CD are two smooth parallel walls. A child rolls a ball along ground from A towards point P find PD so that ball reaches point B after striking the wall CD. Given coefficient of restitution e = 0.5 :
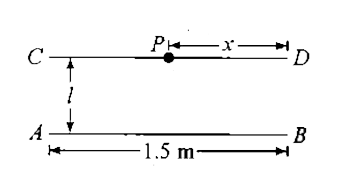
View SolutionA sphere of mass `m_(1)` in motion hits directly another sphere of mass `m_(2)` at rest and sticks to it, the total kinetic energy after collision is `2//3` of their total K.E. before collision. Find the ratio of `m_(1) : m_(2)`.
View Solution
Numerical MCQ s questions and complete solutions for chapter LINEAR MOMENTUM & ITS CONSERVATION of GK PUBLICATIONS PHYSICS (HINGLISH) of Class 11