Complete Solutions to Huygen'S Wave Principle of chapter WAVE OPTICS of Class 12 book with complete answers and questions
| Class 12 | PHYSICS |
| Chapter | WAVE OPTICS |
| A2Z | Huygen'S Wave Principle |
| Number Of Questions | 50 |
| Academic Year | 2023-24 |
The similarity between the sound waves and light waves is
View SolutionFigures shows a wave front P passing through two systems A and B, and emerging as Q and then as R. The systems A and B could, respectively, be

View SolutionLight travel through a glass place of thickness t and refractive index 'n'. If c is the velocity of light in vacuum, then the time taken by light to travel the thickness of the plate will be
View SolutionMonochromatic light of wavelength `lambda_1` travelling in medium of refractive index `n_1` enters a denser medium of refractive index `n_2`. The wavelength in the second medium is
View SolutionA light ray of frequency v and wavelength `lambda` enters a liquid of refractive index `3//2`. The ray travels in the liquid with
View SolutionA beam of light propagating in medium A with index of refraction `n(A)` passes across an interface into medium B with index of refraction `n(B)`. If `v(A)` and `v(B)` are the speeds of light in A and B respectively. Then which of the following is true?
View SolutionA monochromatic beam of light passes from a denser medium into a rarer medium. As a result
View SolutionIf `epsilon_0` and `mu_0` are respectively the electric permittivity and the magnetic permeability of free space and `epsilon` and `mu` the corresponding quantities in a medium, the refractive index of the medium is
View SolutionWave nature of light follows because
View SolutionWavefront means
View SolutionWavefront of a wave has direction with wave motion
View SolutionWhich one of the following phenomena is not explained by Huygens construction of wave front?
View SolutionInterference was observed in interference chamber when air was present, now the chamber is evacuated and if the same light is used, a careful observer will see
View SolutionTwo sources of waves are called coherent if
View SolutionTwo light sources are said to be coherent if they are obtained from
View SolutionFor the sustained interference of light, the necessary condition is that the two sources should
View SolutionHuygens wave theory allows us to know
View SolutionThe wave theory of light was given by
View SolutionThe phase difference between incident wave and reflected wave is `180^@` when light ray
View SolutionWhich of the following phenomena can explain quantum nature of light?
View SolutionWhich of the following is not a property of light?
View SolutionThe phenomenon of interference is shown by
View SolutionTwo identical light sources `S_1` and `S_2` emit light of same wavelength `lambda`. These light rays will exhibit interference if
View SolutionWhich of the following statements indicates that light waves are transverse?
View SolutionRays diverge from a point source of a wavefront that is
View SolutionIf two waves represented by `y_1=4sin omega t` and `y_2=3sin (omegat+pi/3)` interfere at a point, the amplitude of the resulting wave will be about
View SolutionThe two waves represented by `y_1=a sin (omegat)` and `y_2=b cos (omegat)` have a phase difference of
View SolutionTwo waves are represented by the equations `y_1=a sin omega t` and `y_2=a cos omegat`. The first wave
View SolutionIn a wave, the path difference corresponding to a phase difference of `phi` is
View SolutionThe ratio of intensities of two waves are given by `4:1`. The ratio of the amplitudes of the two waves is
View SolutionRatio of amplitude of interfering waves is `3:4`. Now ratio of their intensities will be
View SolutionTwo coherent sources of intensities `I_1` and `I_2` produce an interference pattern. The maximum intensity in the interference pattern will be
View SolutionIntensities of the two waves of light are I and `4I`. The maximum intensity of the resultant wave after superposition is
View SolutionTwo waves of intensity I undergo interference. The maximum intensity obtained is
View SolutionTwo coherent monochromatic light beams of intensities I and `4I` are superposed. The maximum and minimum possible intensities in the resulting beam are
View SolutionIf two light waves having same frequency have intensity ratio `4:1` and they interfere, the ratio of maximum to minimum intensity in the pattern will be
View SolutionIf the ratio of amplitude of two waves is `4:3`, then the ratio of maximum and minimum intensity is
View SolutionTwo coherent sources have intensity in the ratio of `(100)/(1)`. Ratio of (intensity) max/(intensity) min is
View SolutionLight waves producing interference have their amplitudes in the ratio `3:2`. The intensity ratio of maximum and minimum interference fringes is
View SolutionTwo coherent sources of different intensities send waves which interfere. The ratio of maximum intensity to the minimum intensity is 25. The intensities of the sources are in the ratio
View SolutionIf an interference pattern have maximum and minimum intensities in `36:1` ratio, then what will be the ratio of amplitudes?
View SolutionIn the interference pattern, energy is
View SolutionFour light waves are represented by
(i) `y=a_1sin omegat`
(ii) `y=a_2 sin (omega t+varphi)`
(iii) `y=a_1sin 2 Omega t`
(iv) `y=a_2sin 2(omegat+varphi)`
Interference fringes may be observed due to superposition of
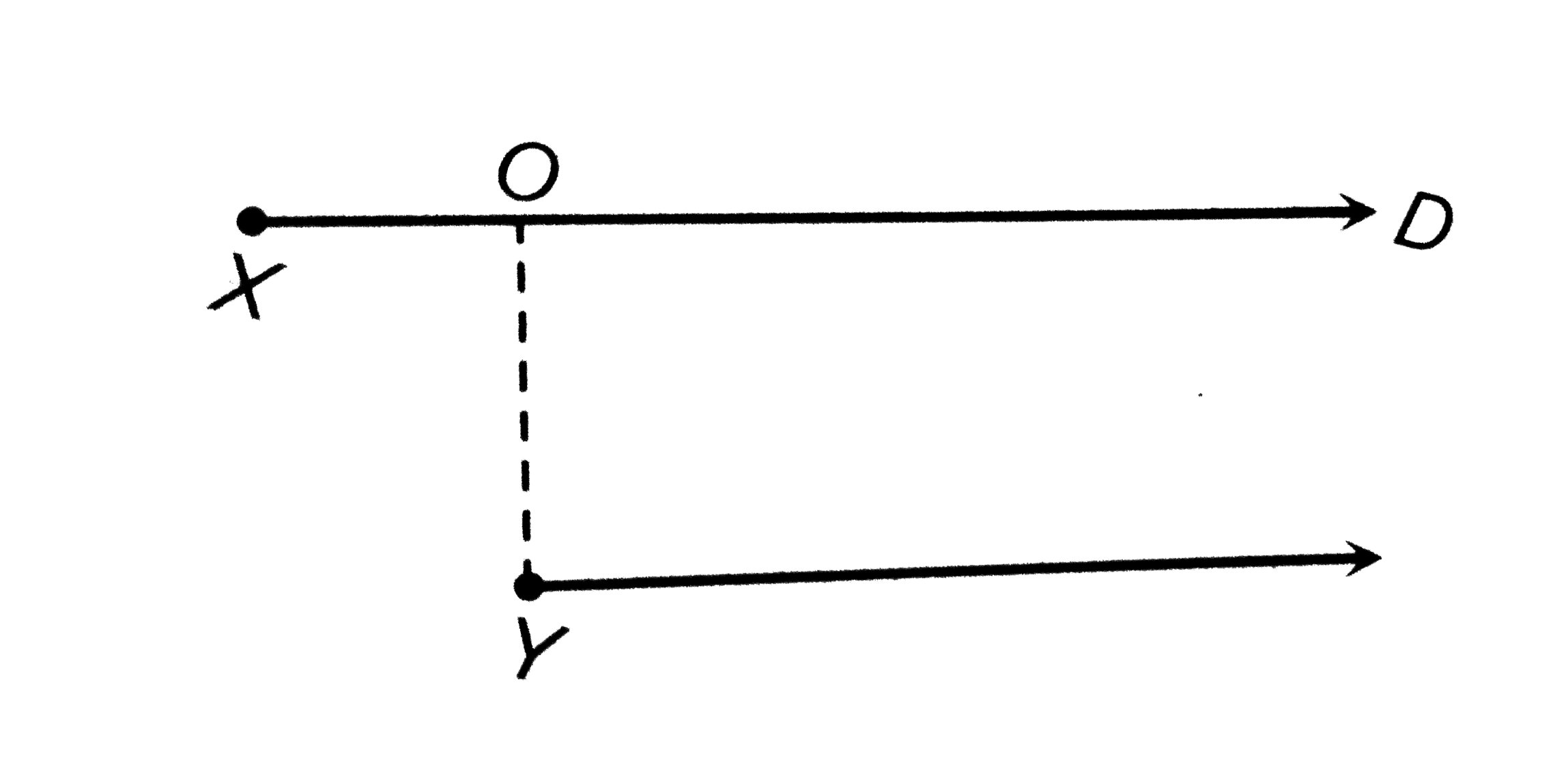
View SolutionTwo point sources X and Y emit waves of same frequency and speed but Y lags in phase behind X by `2pi//radian`. If there is a maximum in direction D the distance XO using n as an integer is given by
View SolutionAmong the two interfering monochromatic sources A and B, A is ahead of B in phase by `66^@`. If the observation be taken from point P, such that `PB-PA=lambda//4`. Then the phase difference between the waves from A and B reaching P is
View SolutionThe intensity ratio of two coherent sources of light is p. They are interfering in some region and produce interference patten. Then the fringe visibility is
View SolutionThree waves of equal frequency having amplitudes `10mum`, `4mum` and `7mum` arrive at a given point with successive phase difference of `pi//2`, the amplitude of the resulting wave in `mum` is given by
View SolutionFig, here shows P and Q as two equally intense coherent sources emitting radiations of wavelength 20m. The separation PQ si 5m, and phase of P is ahead of the phase Q by `90^(@)`. A, B and C are three distant points of observation equidistant from the mid - point of PQ. The intensity of radiations of A, B, C will be in the ratio

View SolutionTwo coherent sources separated by distance `d` are radiating in phase having wavelength `lambda`. A detector moves in a big circle around the two sources in the plane of the two sources. The angular position of `n=4` interference maxima is given as

View SolutionTwo coherent sources `S_1` and `S_2` area separated by a distance four times the wavelength `lambda` of the source. The sources lie along y axis whereas a detector moves along +x axis. Leaving the origin and far off points the number of points where maxima are observed is
View Solution
Huygen'S Wave Principle questions and complete solutions for chapter WAVE OPTICS of A2Z PHYSICS (HINGLISH) of Class 12