Complete Solutions to ASSIGNMENT (SECTION -B) (Objective Type Questions) of chapter THERMODYNAMICS of Class 12 book with complete answers and questions
| Class 12 | PHYSICS |
| Chapter | THERMODYNAMICS |
| AAKASH INSTITUTE | ASSIGNMENT (SECTION -B) (Objective Type Questions) |
| Number Of Questions | 30 |
| Academic Year | 2023-24 |
A container is filled with 20 moles of an ideal diatomic gas at absolute temperature T. When heat is supplied to gas temperature remains constant but 8 moles dissociate into atoms. Heat energy given to gas is
View SolutionLiquid oxygen at 50K is heated at 300K at constant pressure of 1at. The rate of heating is constant. Which one of the following graphs represents the variation of temperature with time?
View SolutionIn an isobaric process, the ratio of heat supplied to the system `(dQ)` and work done by the system `(dW)` for diatomic gas is
View Solution3 moles of an ideal gas are contained within a cylinder by a frictionless piston and are initially at temperature T . The pressure of the gas remains constant while it is heated and its volume doubles . If R is molar gas constant , the work done by the gas in increasing its volume is
View SolutionTwo moles of a gas at temperature T and volume V are heated to twice its volume at constant pressure. If `(C _(p))/(C _(v)) = gamma` then increase in internal energy of the gas is-
View SolutionA triatomic, diatomic and monoatomis gas is supplied same amount of heat at constant pressure then
View Solution105 calories of heat is required to raise the temperature of 3 moles of an ideaol gas at constant pressure from` 30^(@)C` to `35^(@)C.` The amount of heat required in calories to raise the temperature of the gas though the range `(60 ^(@)C` to `65^(@)C)` at constant volume is `(gamma = (C_(p))/( C_(v)) = 1.4)`
View SolutionTo an ideal triatomic gas 800 cal heat energy is given at constant pressure . If vibrational mode is neglected , then energy used by gas in work done against surroundings is
View SolutionN moles of a diatomic gas in a cylinder are at a temperature T. Heat is supplied to the cylinder such that the temperature remains constant but n moles of the diatomic gas get converted into monoatomic gas. What is the change in the total kinetic energy of the gas?
View SolutionFigure shows the isotherms of a fixed mass of an ideal gas at three temperatures `T_(A) , T_(B)` and `T_(C)` , then
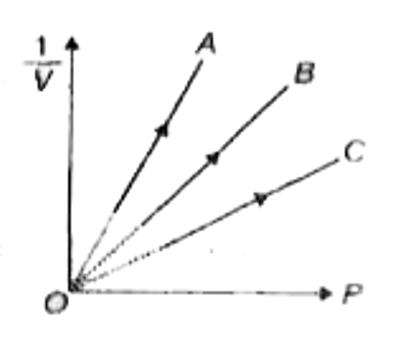
View SolutionAn ideal monatomic gas at 300 K expands adiabatically to 8 times its volume . What is the final temperature ?
View SolutionSlope of isotherm for a gas (having `gamma = (5)/(3)`) is `3 xx 10^(5) N//m^(2)` . If the same gas is undergoing adiabatic change then adiabatic elasticity at that instant is
View SolutionA gas may expand either abiabatically or isothermally .A number of P-V curves are drawn for the two processes over different ranges of pressure and volume . It will be found that :
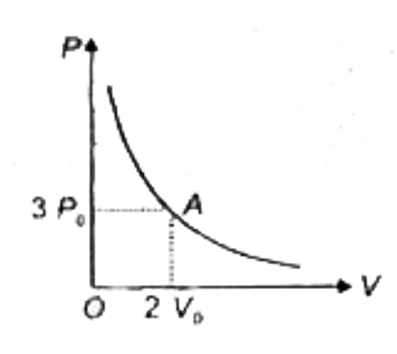
View SolutionThe variation of pressure P with volume V for an ideal monatomic gas during an adiabatic process is shown in figure . At point A the magnitude of rate of change of pressure with volume is
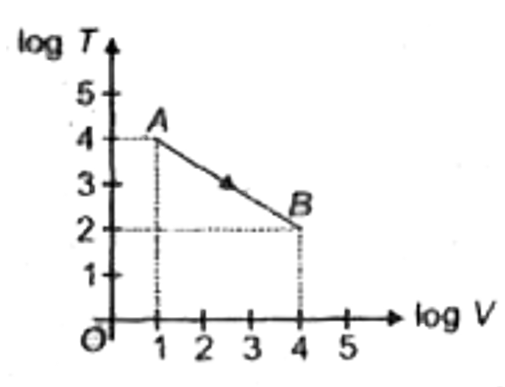
View SolutionFigure shows , the adiabatic curve on log T and log V scale performed on ideal gas . The gas is
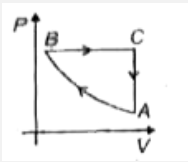
View SolutionA cyclic process on an ideal monatomic gas is shown in figure . The correct statement is
View SolutionA diatomic gas undergoes a process represented by `PV^(1.3)`= constant . Choose the incorrect statement
View SolutionIf a gas is taken from A to C through B then heat absorbed by the gas 8 J . Heat absorbed by the gas in taking it from A to C directly is
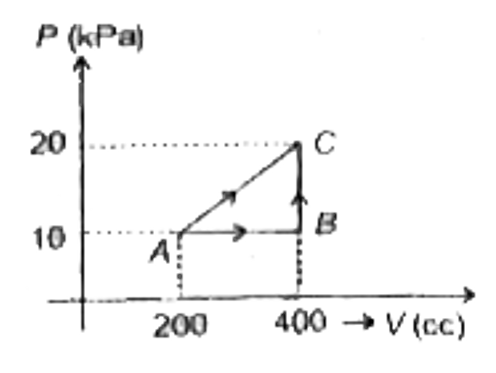
View SolutionThe process CD is shown in the diagram . As system is taken from C to D , what happens to the temperature of the system ?
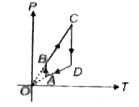
View SolutionA. P . T graph is shown for a cyclic process . Select correct statement regarding the
View SolutionA hydrogen cylinder is designed to withstand an internal pressure of 100 atm . At `27^(@) C` , hydrogen is pumped into the cylinder which exerts a pressure of 20 atm . At which temperature does the danger of explosion first sets in ?
View SolutionAn ideal gas of volume V and pressure P expands isothermally to volume 16 V and then compressed adiabatically to volume V . The final pressure of gas is [`gamma = 1.5]`
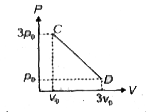
View SolutionThe pressure P of an ideal diatomic gas varies with its absolute temperature T as shown in figure . The molar heat capacity of gas during this process is [R is gas constant]
View SolutionAn ideal gas expands according to the law `P^(2) V ` = constant . The internal energy of the gas
View SolutionThe variation of pressure P with volume V for an ideal diatomic gas is parabolic as shown in the figure . The molar species heat of the gas during this process is
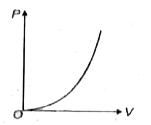
View SolutionNeon gas of a given mass expands isothermally to double volume . What should be the further fractional decreases in pressure , so that the gas when adiabatically compressed from that state , reaches the original state ?
View SolutionWhen `1 kg` of ice at `0^(@)C` melts to water at `0^(@)C`, the resulting change in its entropy, taking latent heat of ice to be `80 cal//.^(@)C`, is
View SolutionCarrot cycle is plotted in P-V graph . Which portion represents an isothermal expansion ?
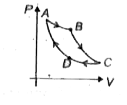
View SolutionEfficiency of a heat engine working between a given source and sink is 0.5 . Coefficient of performance of the refrigerator working between the same source and the sink will be
View SolutionA heat engine rejects 600 cal to the sink at `27^(@)C` . Amount of work done by the engine will be
(Temperature of source is `227^(@) C` & J = 4.2 J/cal )
View Solution
ASSIGNMENT (SECTION -B) (Objective Type Questions) questions and complete solutions for chapter THERMODYNAMICS of AAKASH INSTITUTE ENGLISH of Class 12
All Exercises from AAKASH INSTITUTE Class 12 PHYSICS THERMODYNAMICS
- ASSIGNMENT (SECTION -A) (Objective Type Questions)
- ASSIGNMENT (SECTION -B) (Objective Type Questions)
- ASSIGNMENT (SECTION -C) (Previous Year Questions)
- ASSIGNMENT (SECTION -D) (Assertion - Reason Type Questions)
- Assignment (Section-A) Objective Type Questions (one option is correct)
- Assignment (Section-B) Objective Type Questions (one option is correct)
- Assignment (Section-C) Objective Type Questions (More than one option are correct)
- Assignment (Section-D) Linked comprehension Type questions
- Assignment (Section-E) Assertion-Reason Type Questions
- Assignment (Section-F) Matrix-Match type Question
- Assignment (Section-G) Integer Answer Type Questions
- Assignment (Section-H) Multiple True-False Type Question
- Assignment (Section-I) Subjective Type
- Assignment (Section-J) Aakash Challengers Questions
- Example
- EXERCISE
- TRY YOURSELF
- Try Youself