Complete Solutions to Single correct Answer of chapter THEORY OF EQUATIONS of Class 12 book with complete answers and questions
| Class 12 | MATHS |
| Chapter | THEORY OF EQUATIONS |
| CENGAGE NEW DPP | Single correct Answer |
| Number Of Questions | 69 |
| Academic Year | 2023-24 |
Number of real solutions of `sqrt(2x-4)-sqrt(x+5)=1` is (a) 0 (b) 1 (c) 2 (d) infinite
View SolutionNumber of real solutions of `sqrt(x)+sqrt(x-sqrt(1-x))=1` is (a) 0 (b) 1 (c) 2 (d) infinite
View SolutionThe set of real values of `a` for which the equation `(2a^(2)+x^(2))/(a^(3)-x^(3))-(2x)/(ax+a^(2)+x^(2))+(1)/(x-a)=0` has a unique solution is (a) (−∞,1) (b) (−1,∞) (c) (-1,1) (d) R−{0}
View SolutionNumber of distinct real solutions of the equation `x^(2)+((x)/(x-1))^(2)=8` is (a) 1 (b) 2 (c)3 (d)4
View SolutionIf `m`, `n` are positive integers and `m+nsqrt(2)=sqrt(41+24sqrt(2))`, then `(m+n)` is equal to (a) 5 (b) 6 (c) 7 (d) 8
View SolutionThe equation `(x+3-4(x-1)^(1//2))^(1//2)+(x+8-6(x-1)^(1//2))^(1//2)=1` has (A) no solution (B) only `1` solution (C) only `2` solutions (D) more than `2` solutions
View SolutionThe number of solutions of `sqrt(3x^(2)+x+5)=x-3` is (A) `0` (B) `1` (C) `2` (D) `4`
View SolutionThe number of real or complex solutions of `x^(2)-6|x|+8=0` is (a) 6 (b) 7 (c) 8 (d) 9
View SolutionIf `alpha, beta` ar the roots of the quadratic equation `x ^(2) -(3+ 2 ^(sqrt(log _(2)3))-3 ^(sqrt(log _(3)2)))x-2 (3 ^(log _(3)2)-2^(log _(z)3))=0, ` then the value of `alpha ^(2) + alpha beta +beta^2` is equal to :
View SolutionWhich of the following is not true for equation `x^(2)log8-xlog5=2(log2)-x` (A) equation has one integral root (B) equation has no irrational roots (C) equation has rational roots (D) none of these
View SolutionLet `f(x)` be a quadratic expression such that `f(-1)+f(2)=0`. If one root of `f(x)=0` is `3`, then the other root of `f(x)=0` lies in (A) `(-oo,-3)` (B) `(-3,oo)` (C) `(0,5)` (D) `(5,oo)`
View SolutionIf `f(x)=(x^(2)+3x+2)(x^(2)-7x+a)` and `g(x)=(x^(2)-x-12)(x^(2)+5x+b)`, then the value of `a` and `b`, if `(x+1)(x-4)` is H.C.F. of `f(x)` and `g(x)` is (a) a=10 : b=6` (b) `a=4 : b=12` (c)`a=12 : b=4` (d)`a=6 : b=10`
View SolutionThe remainder obtained when the polynomial `x+x^(3)+x^(9)+x^(27)+x^(81)+x^(243)` is divided by `x^(2)-1` is (a)6x+1 (b)5x+1 (c)4x (d)6x
View SolutionLet `f(x)=x^(2)-ax+b`, `'a'` is odd positive integar and the roots of the equation `f(x)=0` are two distinct prime numbers. If `a+b=35`, then the value of `f(10)=`
View SolutionIf `0 lt alpha lt beta lt gamma lt pi//2`, then the equation
`(x-sinbeta)(x-singamma)+(x-sinalpha)(x-singamma)+(x-sinalpha)(x-sinbeta)=0` has
View SolutionIf the system of equation `r^(2)+s^(2)=t` and `r+s+t=(k-3)/(2)` has exactly one real solution, then the value of `k` is
View SolutionIf `a,` `b `, `c in R` and `3b^(2)-8ac lt 0`, then the equation `ax^(4)+bx^(3)+cx^(2)+5x-7=0` has
View SolutionFor real solution of equation `3sqrt(x+3p+1)-3sqrt(x)=1`, we have
View SolutionFor `a`, `b`,`c` non-zero, real distinct, the equation, `(a^(2)+b^(2))x^(2)-2b(a+c)x+b^(2)+c^(2)=0` has non-zero real roots. One of these roots is also the root of the equation :
View SolutionThe equation `x^(2)+bx+c=0` has distinct roots. If `2` is subtracted from each root the result are the reciprocal of the original roots, then `b^(2)+c^(2)` is
View SolutionThe equation `(x^(2)+3x+4)^(2)+3(x^(2)+3x+4)+4=x` has
View SolutionIf one root of the equation `(x-1)(7-x)=m` is three times the other, then `m` is equal to
View SolutionIf the roots of the equation `ax^(2)-4x+a^(2)=0` are imaginery and the sum of the roots is equal to their product then `a` is
View SolutionIf the sum of squares of roots of equation `x^(2)-(sin alpha-2)x-(1+sin alpha)=0` is the least, then `alpha` is equal to
View SolutionIf `alpha` and `beta` are the roots of the equation `ax^(2)+bc+c=0` then the sum of the roots of the equation `a^(2)x^(2)+(b^(2)-2ac)x+b^(2)-4ac=0` is
View SolutionIf the roots of the quadratic equation `ax^(2)+bx-b=0`, where `a`, `b in R` such that `a*b gt 0`, are `alpha` and `beta`, then the value of `log_(|(beta-1)|)|(alpha-1)|` is
View SolutionIf `cos^4 alpha +k and sin^4 alpha+k` are the roots of `x^2+lambda(2 x+1)=0 and sin^2 alpha+1 and cos^2 alpha +1` are the roots of `x^2 +8 x+4=0,` then the sum of the possible values of `lambda` is
View SolutionLet `f(x)=ax^(2)+bx+c`, `g(x)=ax^(2)+qx+r`, where `a`, `b`, `c`,`q`, `r in R` and `a lt 0`. If `alpha`, `beta` are the roots of `f(x)=0` and `alpha+delta`, `beta+delta` are the roots of `g(x)=0`, then
View SolutionIf `a`, `b`, `c` are in geometric progresion and the roots of the equations `ax^(2)+2bx+c=0` are `alpha` and `beta` and those of `cx^(2)+2bx+a=0` are `gamma` and `delta` then
View SolutionIf `alpha`, `beta` are the roots of the equation `ax^(2)+bx+c=0` and `S_(n)=alpha^(n)+beta^(n)`, then `aS_(n+1)+bS_(n)+cS_(n-1)=(n ge 2)`
View SolutionLet `f(x)=ax^(2)+bx+c`, `g(x)=ax^(2)+px+q`, where `a`, `b`, `c`, `q`, `p in R` and `b ne p`. If their discriminants are equal and `f(x)=g(x)` has a root `alpha`, then
View SolutionIf `alpha` and `beta` be the roots of equation `x^(2) + 3x + 1 = 0` then the value of `((alpha)/(1 + beta))^(2) + ((beta)/(1 + alpha))^(2)` is equal to
View SolutionThe roots of the equation `a(b-2c)x^(2)+b(c-2a)x+c(a-2b)=0` are, when `ab+bc+ca=0`
View SolutionIf the equations `2x^(2)-7x+1=0` and `ax^(2)+bx+2=0` have a common root, then
View SolutionIf `alpha` and `beta`, `alpha` and `gamma`, `alpha` and `delta` are the roots of the equations `ax^(2)+2bx+c=0`, `2bx^(2)+cx+a=0` and `cx^(2)+ax+2b=0` respectively where `a`, `b`, `c` are positive real numbers, then `alpha+alpha^(2)` is equal to
View SolutionThe product of uncommon real roots of the polynomials ` p(x)=x^4+2x^3-8x^2-6x+15` and ` q(x) ` `= x^3+4x^2-x-10 ` is :
View SolutionNumber of values of `x` satisfying the pair of quadratic equations `x^(2)-px+20=0` and `x^(2)-20x+p=0` for some `p in R` is
View SolutionIf the equation `4x^2-x-1=0 and 3x^2+(lambda+mu)x+lambda-mu=0` have a root common, then the irrational values of `lambda and mu` are (a) `lambda=(-3)/4` b. `lambda=0` c. `mu=3/4` b.`mu=0`
View SolutionIf the equations `x^(2)+2lambdax+lambda^(2)+1=0`, `lambda in R` and `ax^(2)+bx+c=0` , where `a`, `b`, `c` are lengths of sides of triangle have a common root, then the possible range of values of `lambda` is
View SolutionIf bot the roots of `lamda(6x^(2)+3)rx+2x^(2)-1=0` and `6 lamda(2x^(2)+1)+px+4x^(2)-2=0` are common, then `2r-p` is equal to
View Solution`x^(3)+5x^(2)+px+q=0` and `x^(3)+7x^(2)+px+r=0` have two roos in common. If their third roots are `gamma_(1)` and `gamma_(2)` , respectively, then `|gamma_(1)+gamma_(2)|=`
View SolutionLet `a,b in N, a != b` and the two quadratic equations `(a-1)x^2-(a^2+2)x+a^2+2a=0 and (b-1)x^2-(b^2+2)x+(b^2+2b)=0` have a common root. The value of `ab` is
View SolutionA quadratic equations `p(x)=0` having coefficient `x^(2)` unity is such that `p(x)=0` and `p(p(p(x)))=0` have a common root, then
View SolutionIf `ax^2+bx+c=0 and cx^2 + bx+a=0(a,b,c in R)` have a common non-real roots, then which of the following is not true ?
View SolutionConsdier the equaiton `2 + |x^(2) + 4x + 3_= m , m in R`
Set of all real values of m so that given equation have four distinct solutions, is
View SolutionIf the equation `|x^2-5x + 6|-lambda x+7 lambda=0` has exactly 3 distinct solutions then `lambda` is equal to
View SolutionLet `alpha`, `beta (a lt b)` be the roots of the equation `ax^(2)+bx+c=0`. If `lim_(xtom) (|ax^(2)+bx+c|)/(ax^(2)+bx+c)=1` then
View SolutionIf the quadratic polynomials defined on real coefficient
`P(x)=a_(1)x^(2)+2b_(1)x+c_(1)` and `Q(x)=a_(2)x^(2)+2b_(2)x+c_(2)` take positive values `AA x in R`, what can we say for the trinomial `g(x)=a_(1)a_(2)x^(2)+b_(1)b_(2)x+c_(1)c_(2)` ?
View SolutionFor which of the following graphs the quadratic expression `y=ax^(2)+bx+c` the product `abc` is negative ?
View SolutionThe difference of maximum and minimum value of `(x^(2)+4x+9)/(x^(2)+9)` is
View SolutionIf `a gt 1` , then the roots of the equation `(1-a)x^(2)+3ax-1=0` are
View SolutionThe values of `'a'` for which the quadraic expression `ax^(2)+(a-2)x-2` is negative for exactly two integral values of `x`, belongs to
View SolutionIf the roots of equation `(a + 1)x^2-3ax + 4a = 0` (a is not equals to -1) are greater than unity, then
View SolutionThe equation `ax^(4)-2x^(2)-(a-1)=0` will have real and unequal roots if
View SolutionIf `ax^(2)+bx+c=0`, `a ne 0`, `a`, `b`, `c in R` has distinct real roots in `(1,2)`, then `a` and `5a+2b+c` have (a) same sign (b) opposite sign (c) not determined (d) none of these
View SolutionIf `c lt a lt b lt d`, then roots of the equation `bx^(2)+(1-b(c+d)x+bcd-a=0`
View SolutionIf `2a`, `b`, `2c` are in `A.P.` where `a`, `b`, `c` are `R^(+)`, then the expression `f(x)=(ax^(2)-bx+c)` has
View SolutionIf `a`, `b`, `c` are positive numbers such that `a gt b gt c` and the equation `(a+b-2c)x^(2)+(b+c-2a)x+(c+a-2b)=0` has a root in the interval `(-1,0)`, then
View SolutionIf the quadratic equation `x^(2)-36x+lambda=0` has roots `alpha` and `beta` such that `alpha`, `beta in N` and `(lambda)/(5) cancel in Z` and `lambda` assumes minimum possible value then `(sqrt(alpha+2)sqrt(beta+2))/(|alpha-beta|)` is equal to (a) `(3)/(8)` (b) `(3)/(16)` (c) `(sqrt(111))/(34)` (d) `(sqrt(111))/(17)`
View SolutionIf the equation `2^(2x)+a*2^(x+1)+a+1=0` has roots of opposite sign, then the exhaustive set of real values of `a` is (a)(−∞,0) (b)(−1,−2/3) (c)(−∞,−2/3) (d)(−1,∞)
View SolutionLet `a`, `b`, `c` ne three distinct non-zero real numbers satisfying the system of equation `(1)/(a)+(1)/(a-1)+(1)/(a-2)=1` , `1/b+(1)/(b-1)+(1)/(b-2)=1` , `(1)/(c )+(1)/(c-1)+(1)/(c-2)=1`. Then `abc=` (a) 1 (b)2 (c)3 (d)4
View SolutionIn the given figure graph of `y=p(x)=x^(4)+ax^(3)+bx^(2)+cx+d` is given
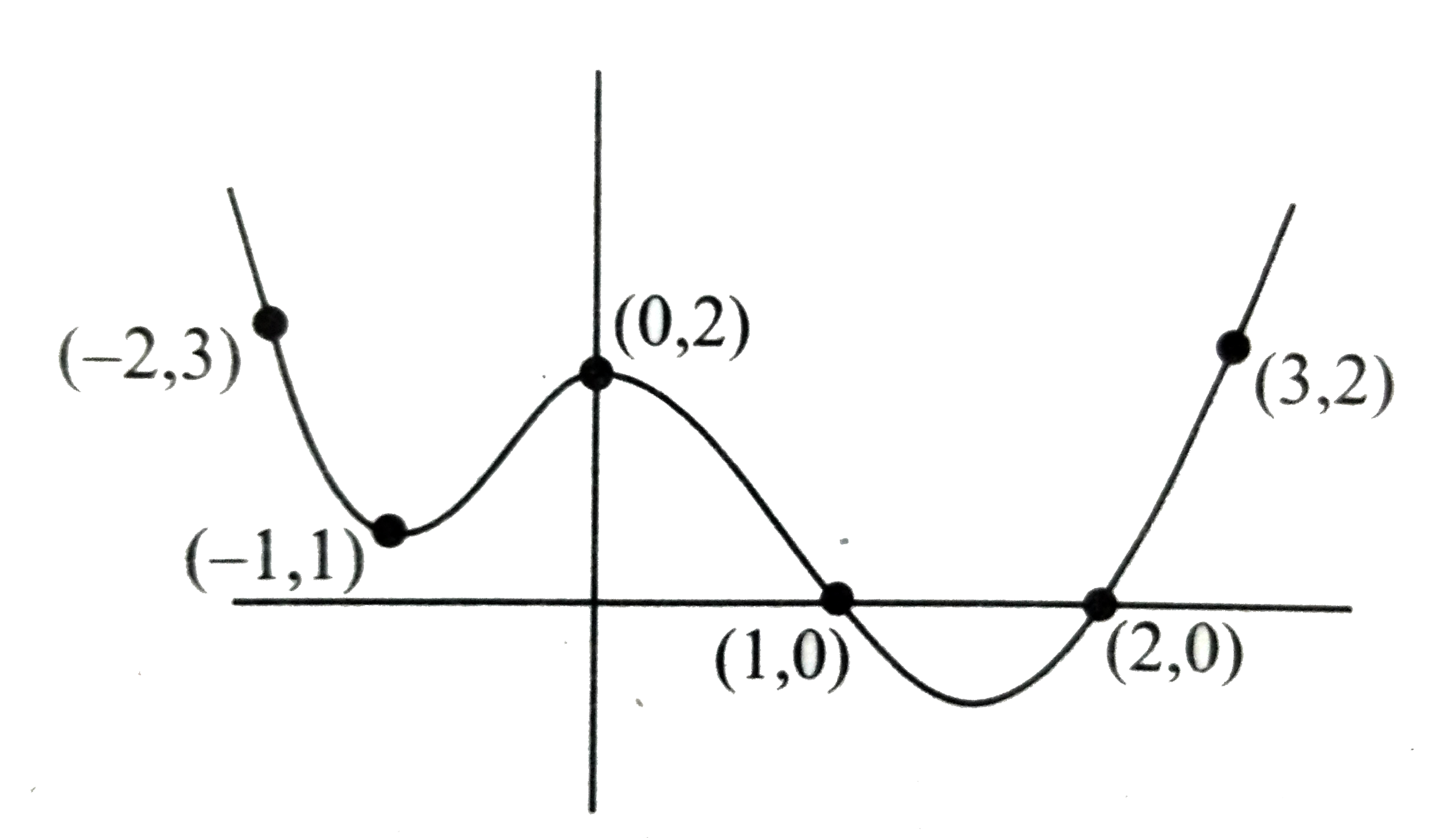
The product of all imaginary roots of `p(x)=0` is (a) 1 (b) 2 (c) `1/3` (d) `1/4`
View SolutionIf `a^(3)-3a^(2)+5a-17=0` and `b^(3)-3b^(2)+5b+11=0` are such that `a+b` is a real number, then the value of `a+b` is
View SolutionLet` f(x) = x^4 + ax^3 + bx^2 + cx + d` be a polynomial with real coefficients and real roots. If |f(i)|=1where `i=sqrt(-1)`, then the value of a +b+c+d is
View SolutionIf f(x) is a polynomial of degree four with leading coefficient one satisfying `f(1)=1, f(2)=2,f(3)=3`.then `[(f(-1)+f(5))/(f(0)+f(4))]`
View SolutionLet `P (x)=x ^(6) -x ^(5) -x ^(3) -x ^(2) -x and alpha, beta, gamma, delta` are the roots of the equation `x ^(4) -x ^(3)-x ^(2) -1=0,` then `P (alpha ) + P (beta) +P (gamma) + P(delta)=`
View SolutionThe line `y=mx+1` touches the curves `y=-x^(4)+2x^(2)+x` at two points `P(x_(1),y_(1))` and `Q(x_(2),y_(2))`. The value of `x_(1)^(2)+x_(2)^(2)+y_(1)^(2)+y_(2)^(2)` is
View SolutionIf `a+2b+3c=4,` then find the least value of `a^2+b^2+c^2dot`
View SolutionIf the roots of `x^(4)+qx^(2)+kx+225=0` are in arthmetic progression, then the value of `q`, is
View Solution
Single correct Answer questions and complete solutions for chapter THEORY OF EQUATIONS of CENGAGE MATHS (ENGLISH) of Class 12
All Exercises from CENGAGE NEW DPP Class 12 MATHS THEORY OF EQUATIONS
- Archives JEE MAIN (single correct Answer Type )
- Comprehension
- CONCEPT APPLICATION EXERCISE 2.1
- CONCEPT APPLICATION EXERCISE 2.10
- CONCEPT APPLICATION EXERCISE 2.11
- CONCEPT APPLICATION EXERCISE 2.12
- CONCEPT APPLICATION EXERCISE 2.13
- CONCEPT APPLICATION EXERCISE 2.2
- CONCEPT APPLICATION EXERCISE 2.3
- CONCEPT APPLICATION EXERCISE 2.4
- CONCEPT APPLICATION EXERCISE 2.5
- CONCEPT APPLICATION EXERCISE 2.6
- CONCEPT APPLICATION EXERCISE 2.7
- CONCEPT APPLICATION EXERCISE 2.8
- CONCEPT APPLICATION EXERCISE 2.9
- ILLUSTRATION
- JEE ADVANCED (Multiple Correct Answer Type )
- JEE ADVANCED (Numerical Value Type )
- JEE ADVANCED (Multiple Correct Answer Type )
- JEE ADVANCED (Single Correct Type )
- Linked Comprechension Type
- MATRIX MATCH TYPE
- Multiple Correct Answer Type
- Multiple Correct Answer
- NUMERICAL VALUE TYPE
- Single correct Answer
- Single Correct Answer Type : Exercise
- SOLVED EXAMPLES