Complete Solutions to Exercise 3.1 of chapter ELECTRIC POTENTIAL of Class 12 book with complete answers and questions
| Class 12 | PHYSICS |
| Chapter | ELECTRIC POTENTIAL |
| CENGAGE PHYSICS | Exercise 3.1 |
| Number Of Questions | 23 |
| Academic Year | 2023-24 |
The electric field inside a hollow charged conductor is zero. Is this true or false ?
View SolutionHow can you check whether the electric potential in a given region of space has constant value ?
View SolutionAre we free to call the potential of earth (+ 100 V) instead of zero ? What effect would such an assumption have on measured values of
A potentials
B potential difference ?
View SolutionIf you know ( E) at a given point, can you calculate V at that point ? If not, what further information do you need ?
View SolutionCan there be a potential difference between two adjacent conductors that carry same amount of positive charge ?
View SolutionAn electron moves from the positive to the negative terminal of a battery (9 V). How much potential energy did it gain or lose ?
View SolutionWe want to produce a proton with kinetic energy `4.3 xx 10^-15 J`. Through what difference of potential should we accelerate the proton to obtain this kinetic energy assuming that it starts from rest and no other forces are present ?
View SolutionThe potential produced by a point charge is `V = kQ// r`. Use this information to determine the shape of equipotential surface ?
View Solutiona. A circle is drawn with center as a charge +q. What is the work done in moving a charge +q from B to C along the circumferebce of the circle ?
B In the above question, if the charge +q is first taken from B to A and then from A to C, on which path is the magnitude of work greater?
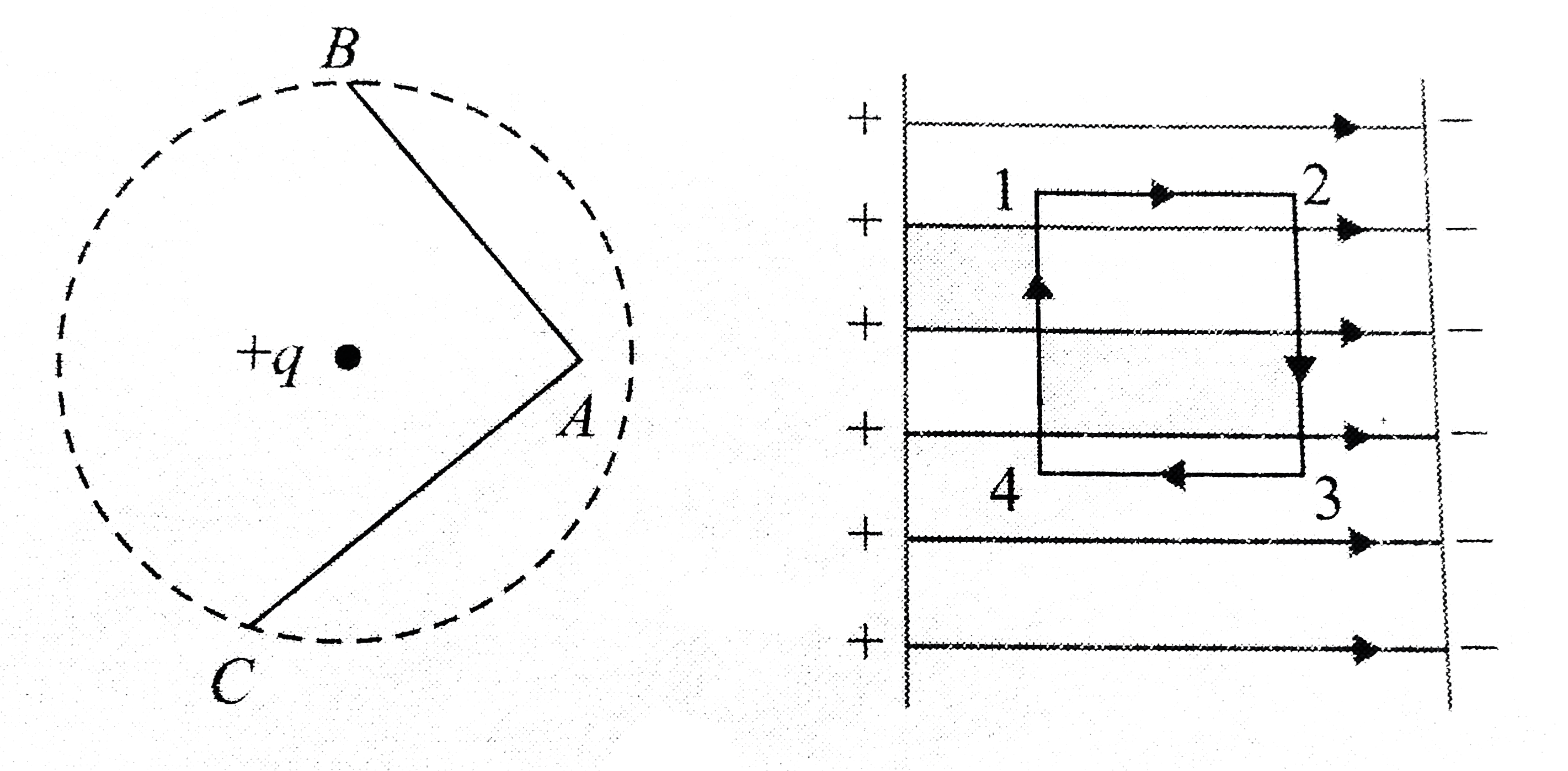
View SolutionA uniform electric field E exists between two oppositely charged plates (Fig. 3.38). What will be the work done in moving a charge q along a closed rectangular path ?
 .
.
View SolutionShow that if at some part of a field the lines of force have the from of concentric circles whose centres are at point O (as given in Fig. 3.39), the field intensity at each point in this part of the field should be inversely proportional to the distance from the point O.
 .
.
View SolutionIf you carry out the integral of the electric field `int vec E. vec d l` for a closed path like that as shown in (Fig. 3.40), the integral will always be equal tom zero, independent of the shape of the path and independent of where charges may be located relative to the path. Explain why.
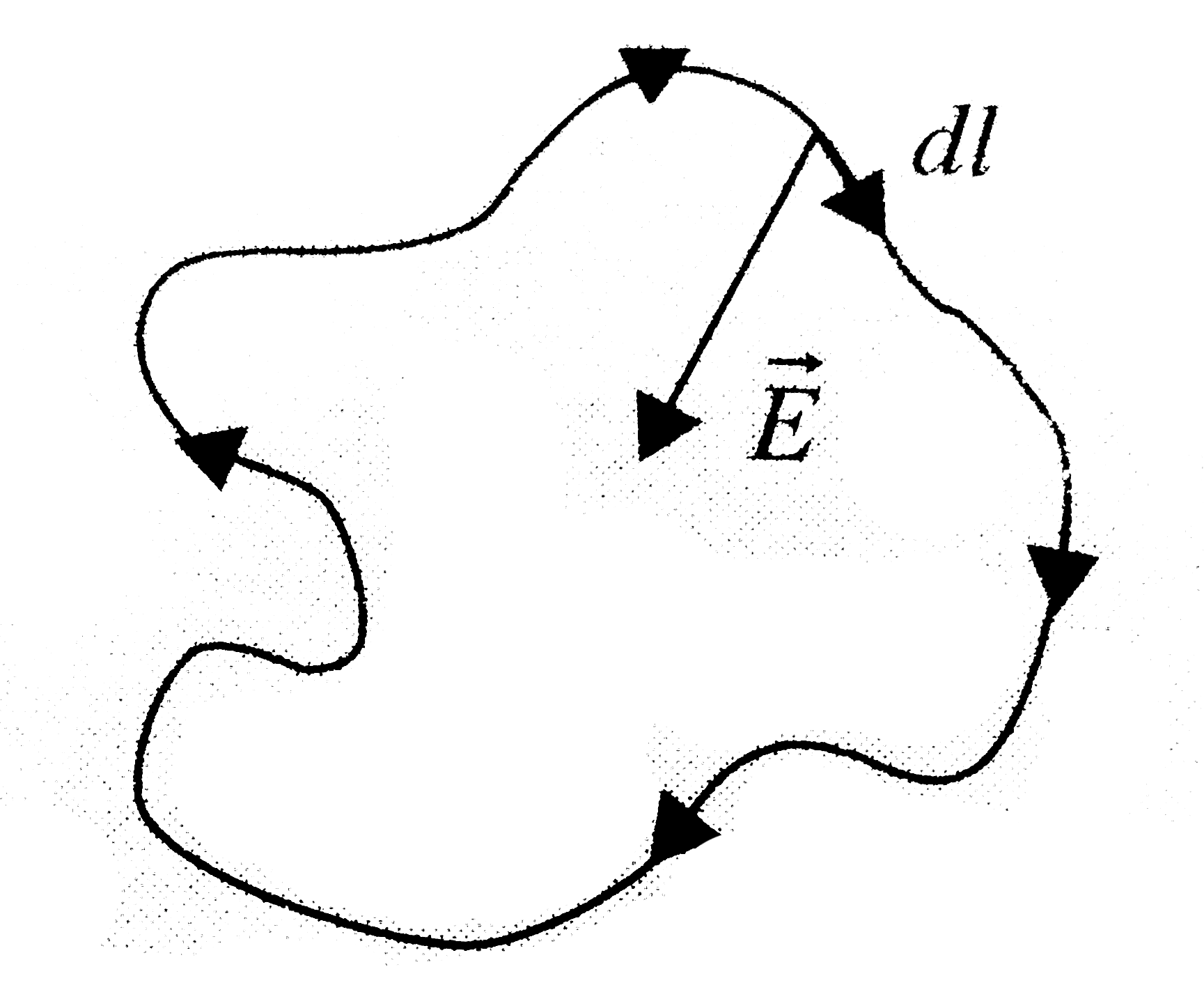 .
.
View Solution(Figure 3.41) shows the lines of constant potential in region in which an electric field is present. The values of potentials are written in brackets. At which points A, B or C, the electric field is greatest.
 .
.
View SolutionA charge `2 muC` is taken from inifinity point in an electric field, without changing its velocity. If work done against eletrostatic forces is `-40 mu J`, then find the potential at that point.
View SolutionWhen charge `10 mu C` is shifted from inifinity to a point in an electric field, it is found that work done by eletrotatic forces is `10 muJ`. If the charge is doubled and taken again from inifinity to the same point without accelerating it, then find the amount of work done by electric field and against electric field.
View SolutionA charge `3 mu C` is released at rest from a point P where electric potential is (20 V). Find its kinetic energy when it reaches inifinity.
View SolutionFind out the following
a. `V_A - V_B`
b. `V_B - V_C`
c. `V_C - V_A`
d. `V_D - V_C`
e. `V_A - V_D`
f. Arrange the order of potential for points (A, B,C and D).
 .
.
View SolutionFour balls, each with mass m, are connected by four nonconducting strings to from a square with side with A, as shown in (Fig. 3.43.) The assembly is placed on a horizontal nonconducting frictionless surface. Balls 1 and 2 each have charge q, and balls 3 and 4 are uncharged. Find the maximum speed of balls 1 and 2 after the string connecting them is cut.
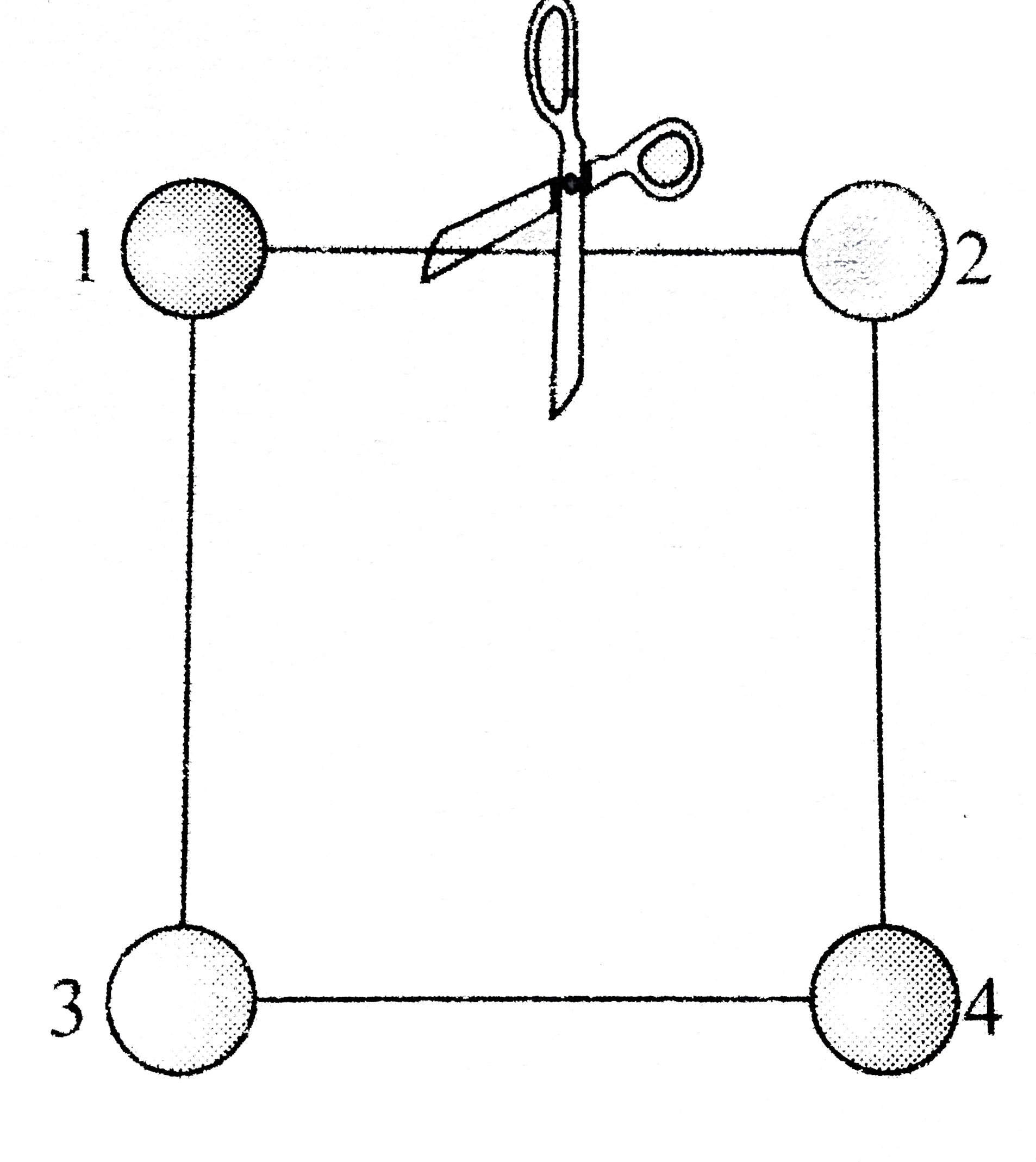 .
.
View SolutionConsider the configuration of a system of four charges each of value (+q). Find the work done by external agent in changing the configuration of the system from (Fig. 3.44 A) to (Fig. 3.4B).
a. ,
,
View SolutionThe electric potential in a region is represented as
`V=2x+3y-z`
obtain expression for electric field strength.
View SolutionFind `V_(ab)` in an electric field
`E = (2 hat(i) +3 hat(j)+4 hat(k))(N)/(C)`
where, `r_(a)=(hat(i)-2 hat(j)+hat(k))m`
and `r_(b)=(2 hat(i)+hat(j)-2 hat(k))m`.
View SolutionA uniform electric field exists in x y plane as shown in Fig. 3.45. Find the potential difference. Between origin O and `A(d,d,0)`.
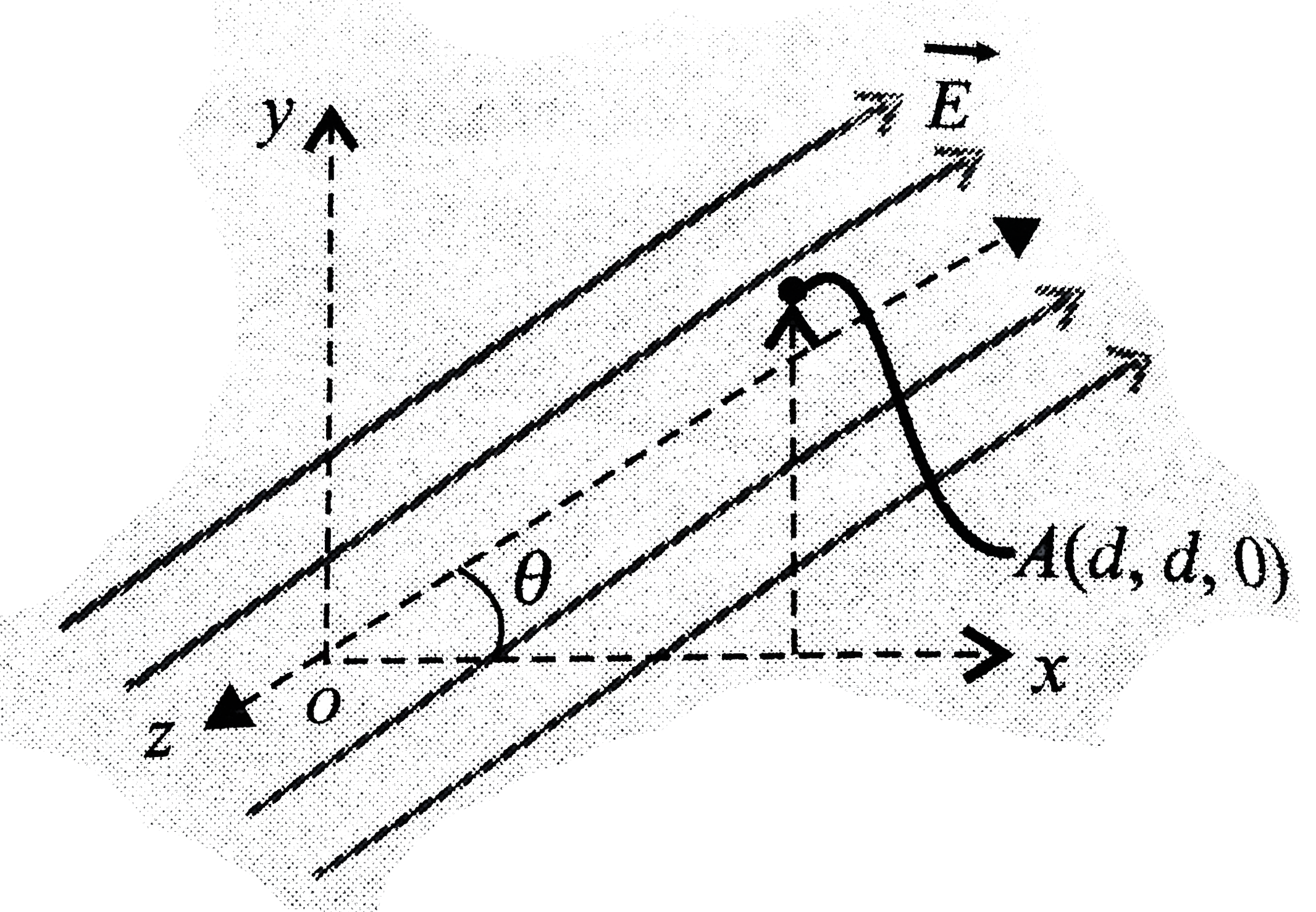 .
.
View SolutionThe electric field in a region is given by `E = (4 axy sqrt(z))hat i + (2 ax^2 sqrt(z)) hat j + (ax^2 y// sqrt(z)) hat k`
where A is a positive constant. The equation of an equipotential surface will be of the form.
View Solution
Exercise 3.1 questions and complete solutions for chapter ELECTRIC POTENTIAL of CENGAGE PHYSICS (ENGLISH) of Class 12