Complete Solutions to DPP 3.4 of chapter ELECTRIC POTENTIAL of Class 12 book with complete answers and questions
| Class 12 | PHYSICS |
| Chapter | ELECTRIC POTENTIAL |
| CENGAGE PHYSICS DPP | DPP 3.4 |
| Number Of Questions | 15 |
| Academic Year | 2023-24 |
Two charges `-q` each are separated by distance `2d`. A third charge `+q` is kept at mid-point O. Find potential energy of `+q` as a function of small distance `x` from O due to `-q` charges. Prove that the charge at O is in an unstable equilibrium.
View SolutionA positively charged particle is released from rest in an uniform electric field. The electric potential energy of the charge.
View SolutionIf identical charges `(-q)` are placed at each corner of a cube of side `b`, then electric potential energy of charge `(+q)` which is placed at centre of the cube will be
View SolutionTwo charges `q_(1)` and `q_(2)` are placed 30 cm apart, as shown in figure. A third charge `q_(3)` is moved along the arc of a circle of radius 40 cm from C to D. The change in the potential energy of the system is `q_(3)/(4pi epsi_(0))K,` where K is

View SolutionA charged particle of charge Q is held fixed another charged particle of mass `m` and charge the same sign is released from a distance `r` impulse of the force exerted by the external agent the fixed charge by the time when distance between Q and q becomes `2r` is
View SolutionA very large sphere having charge `Q` uniformly distributed on the surface s compressed uniformly till its radius reduces to `R`. The work done by the electric force in this process is :
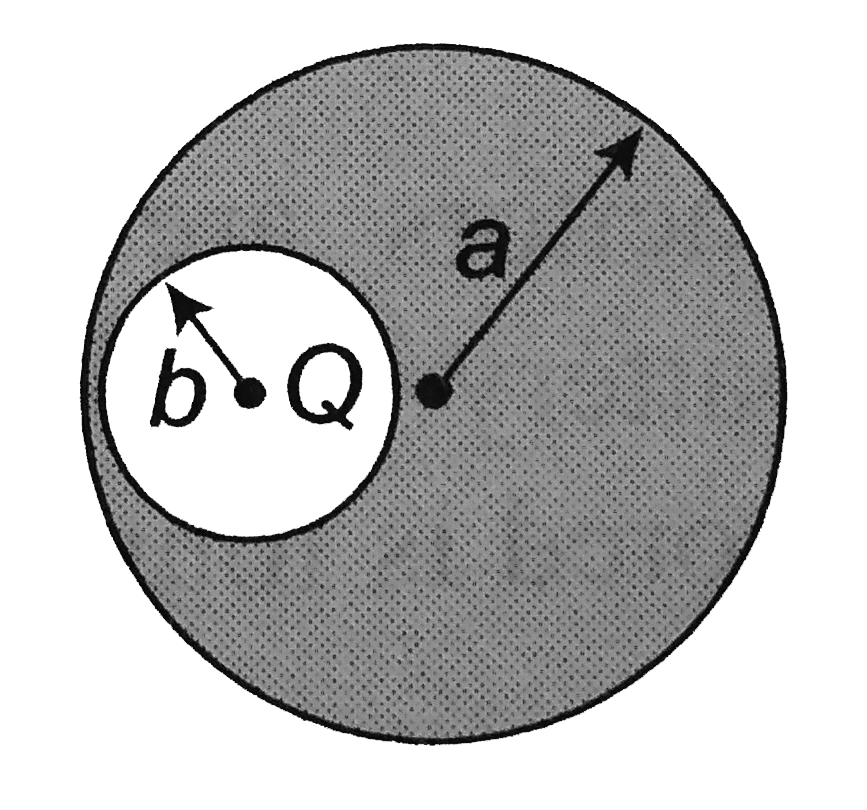
View SolutionIn the figure shown the elctric potential energy of the system is : `(q` is at the centre of the conducting neutral spherical shell of inner radius a and outer radius `b`)

View SolutionA point charge 'Q' is placed at the centre of a spherical cavity of radius 'b' carved inside a solid conducting sphere of radius 'a'. Then total energy of the system is:
`[k = (1)/(4pi in_(0))]`
View SolutionFour charges are rigidly fixed along the Y-axis as shown. A positive charge approches the system along the `X`axis with initial speed just enough to cross the origin. Then its total energy at the origin is

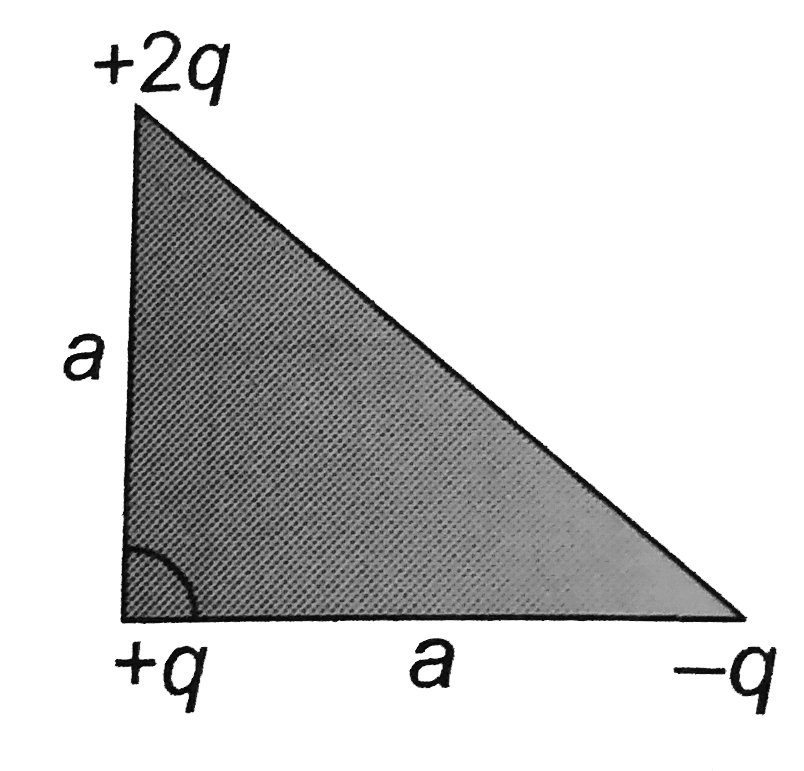
View SolutionThree charge `+q, -q`, and `+2q` are placed at the vertices of a right angled triangle (isosceles triangle)as shown. The net electrostatic energy of the configuration is:
View SolutionThree equal charges `Q` are placed at the three vertices of an equilateral triangle. What should be the va,ue of a charge, that when placed at the centroid, reduces the interaction energy of the system to zero ?
View SolutionYou are moving a negative charge `q lt 0` at a small constant speed away from a uniformly charged non-conducting spherical shell on which resides a negative charge `Q lt 0`. The electrostatic, field of `Q` is `E`. Let `U` be the total energy of the system, `W_(a)` the work done by the force `F_(a)` you exert on `q, W_(E)` the work done by the electrostaitc force `F_(E)` on `q`. Then, as `q` is being moved :
View SolutionA negative charge `Q` is distributed uniformly in volume of a sphere is radius R and a point charge particle (may be negative or positive) is present on the surface of this sphere then variation of escape velocity `(v_(s))` of charge 'q' as a function of 'q' will be [negect gravitational interaction].
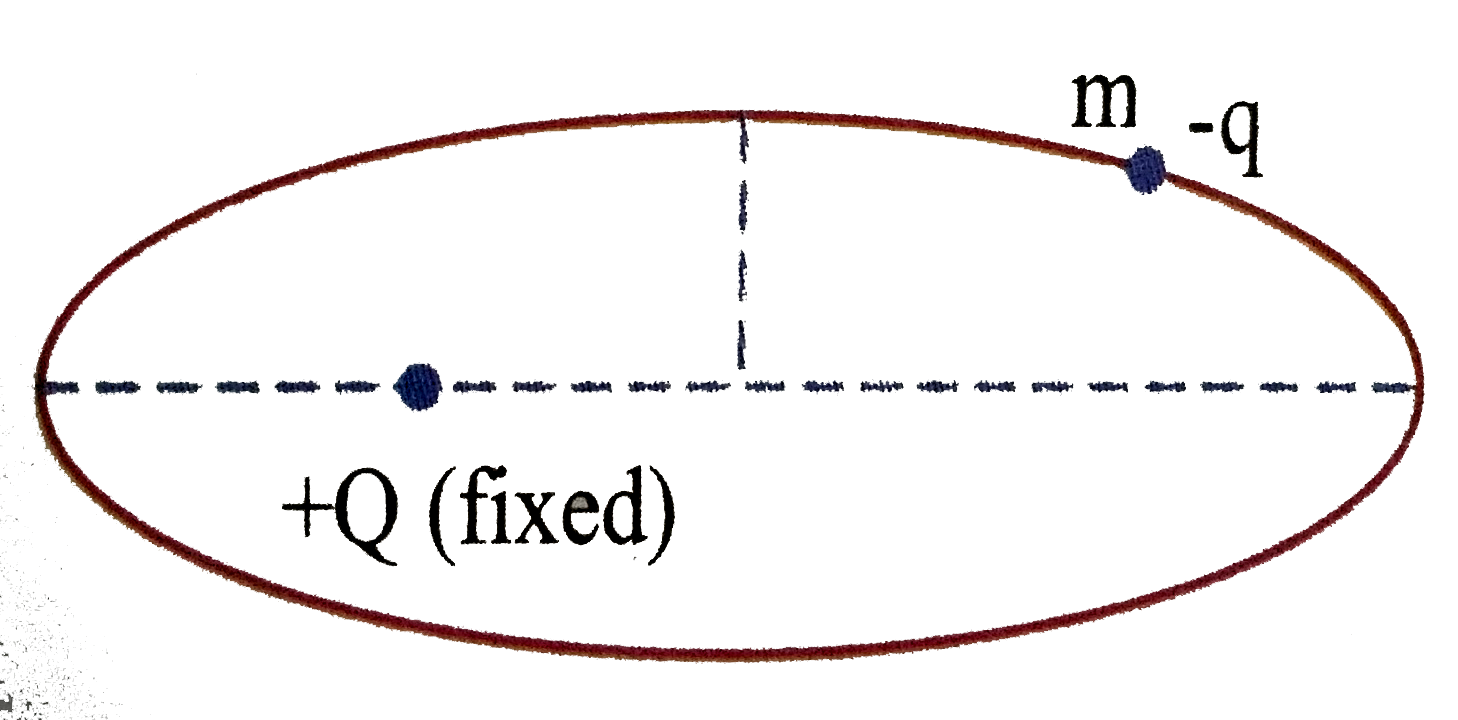
View SolutionA positive point charge `+Q` is fixed in space .A negative point charge `-q` of mass `m` revolves around a fixed charge in elliptical orbits .The fixed charge `+Q `is at one focus of the ellipse.The only force acting on negative charge is the electrostatic force due to positive charge is the electrostatic force due to positive charge.Then which of the following statement is true
View SolutionSmall identical balls with equal charges are fixed at the vertices of a regular polygon of N sides, each of length d. At a certain instant, one of the ball is released. After long time interval, the adjacent ball to the previous one is released. The difference in kinetic energies of the two released balls is K at a sufficiently long distance from the polygon.
View Solution
DPP 3.4 questions and complete solutions for chapter ELECTRIC POTENTIAL of CENGAGE PHYSICS (ENGLISH) of Class 12