Complete Solutions to DPP 3.2 of chapter ELECTRIC POTENTIAL of Class 12 book with complete answers and questions
| Class 12 | PHYSICS |
| Chapter | ELECTRIC POTENTIAL |
| CENGAGE PHYSICS DPP | DPP 3.2 |
| Number Of Questions | 15 |
| Academic Year | 2023-24 |
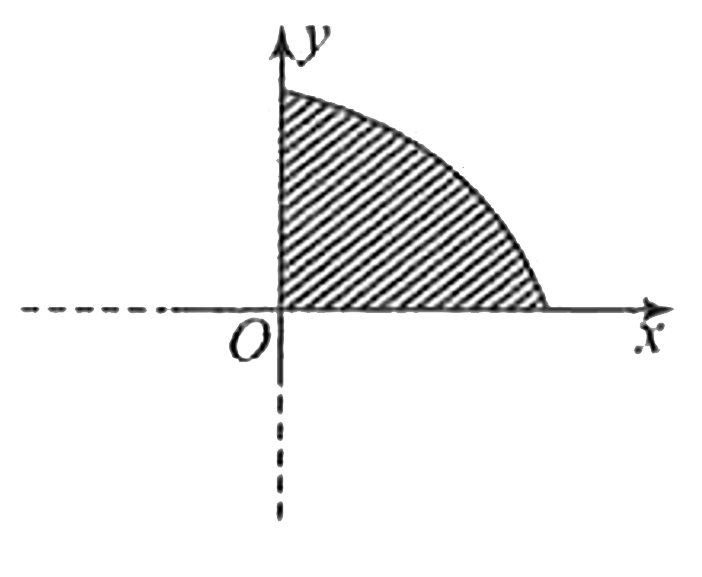
A uniform surface charge of density `sigma` is given to a quarter of a disc extending up to infinity in the first quadrant of `x-y` plane. The centre of the disc is at the origin `O`. Find the z-component of the electric field at the point `(0,0,z)` and the potential difference between the point `(0,0,d)` and `(0,0,2d)`
View SolutionThe electric potential V at any point x,y,z (all in metre) in space is given by `V=4x^2` volt. The electric field at the point `(1m, 0, 2m)` is ……………`V/m`.
View SolutionElectric potential is given by
`V = 6x - 8xy^(2) - 8y + 6yz - 4z^(2)`
Then electric force acting on `2C` point charge placed on origin will be
View SolutionConsider two points `1 and 2` in a region outside a charged sphere. Two points are not very far away from the sphere. If `E and V` respresent the electric fileld vector and the electric potential. Which of the following is not possible ?
View SolutionElectric potential at any point is `V=-5x +3y+sqrt(15)z`, then the magnitude of the electric field is
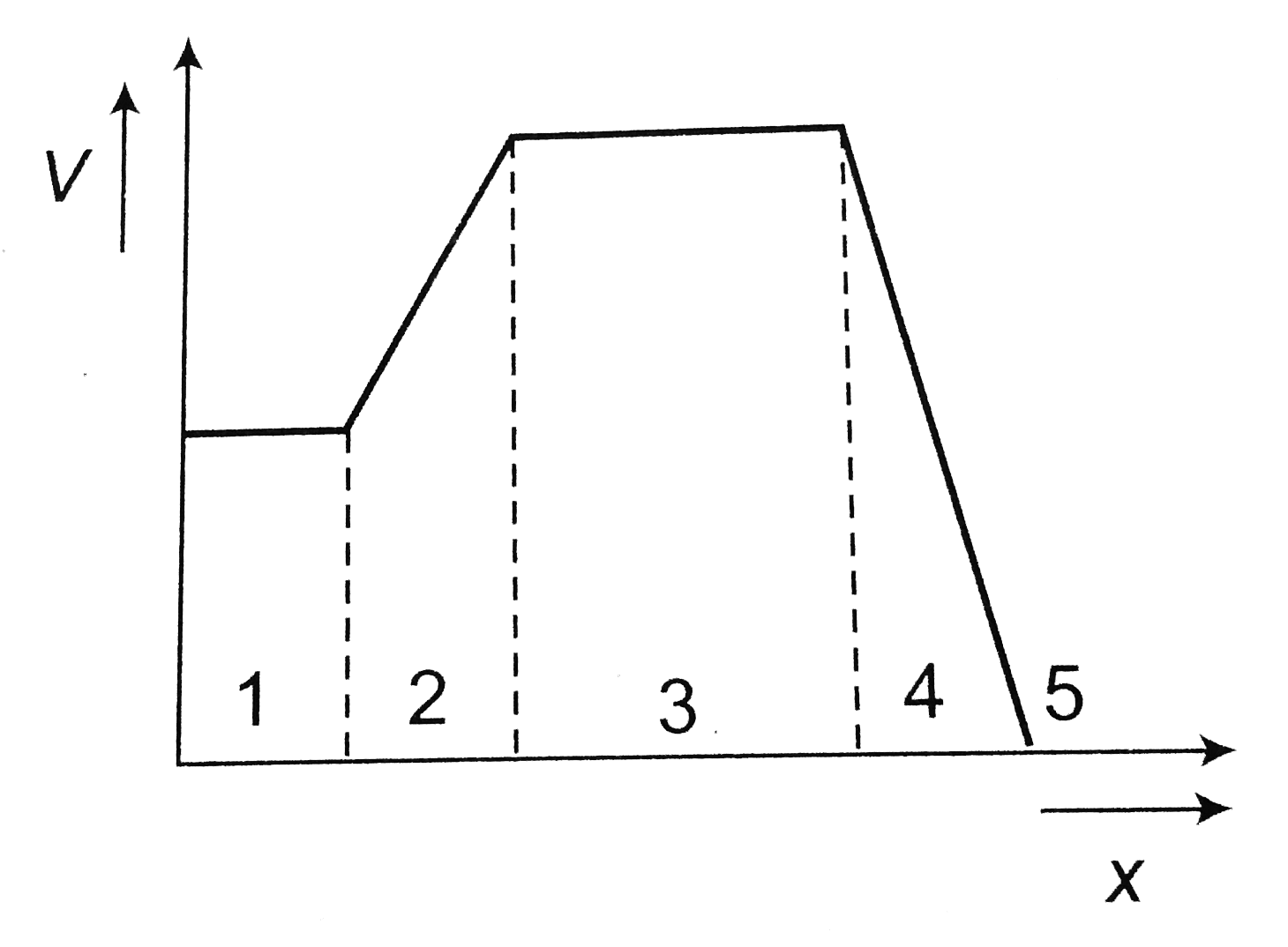
View SolutionThe figure gives the electric potential `V` as a function of distance through five regions on x-axis. Which of the following is true for the electric `E` in these regions ?
View SolutionFigure shown a charged conductor resting on an insulating stand. If at the point `P` the charge density is `sigma`, the potential is `V` and the electric field strength is `E`, what are the values of these quantities at point `Q` ?

View SolutionThe electrostatic potential on the surface of a charged conducting sphere is 100 V. Two statements are made in this regard
`S_(1):` At any point inside the sphere, electric intensity is zero.
`S_(2):` At any point inside the sphere, the electrostatic potential is 100 V.
Which of the following is a correct statement ?
View SolutionIf unifrom electric filed `vecE = E_(0)hati + 2E_(0) hatj`, where `E_(0)` is a constant, exists in a region of space and at `(0, 0)` the electric potential `V` is zero, then the potential at `(x_(0),0)` will be.
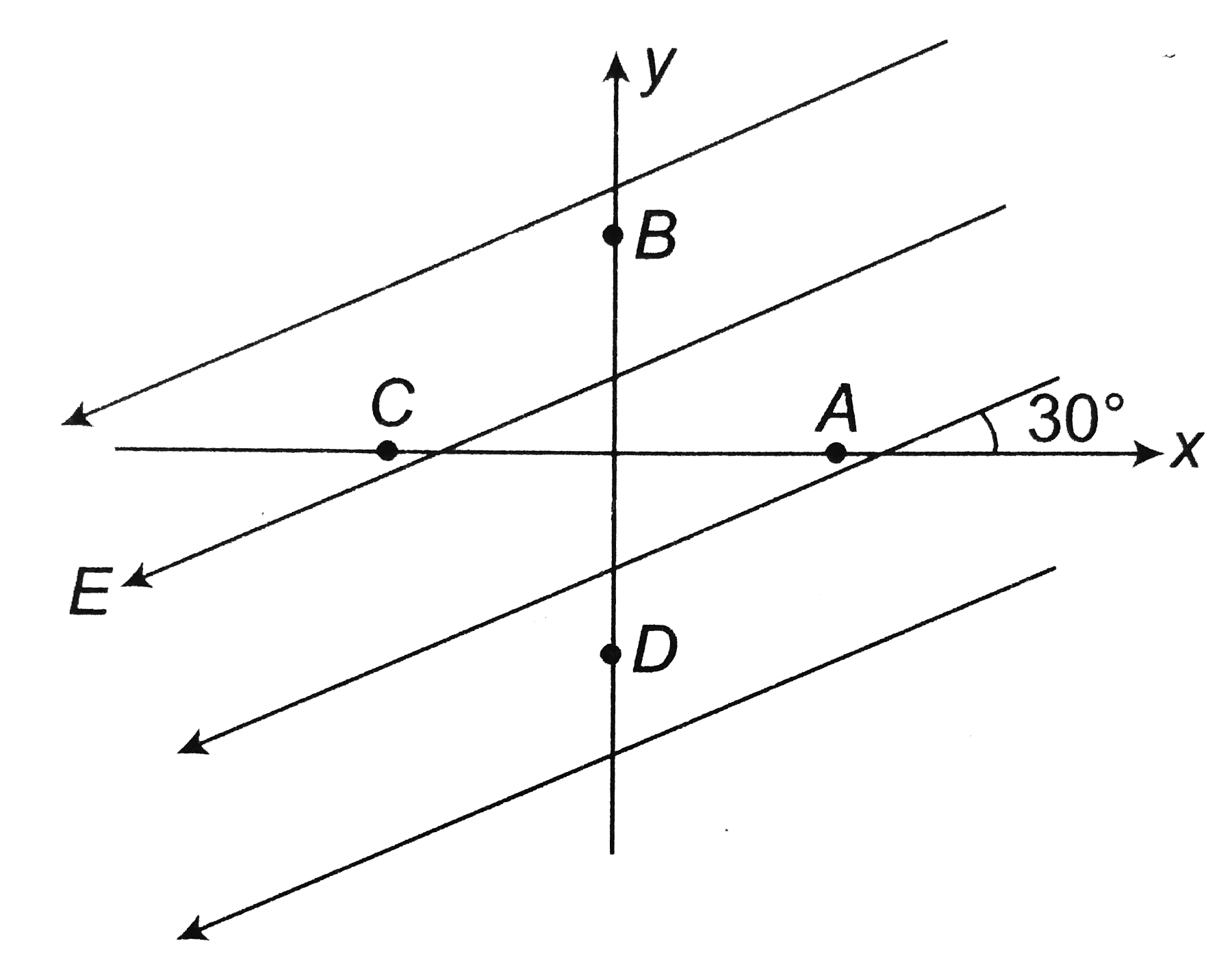
View SolutionThere exists a uniform electric filed in the space as shown. Four points `A, B, C` and `D` are marked which are equildistant from the origin. If and `V_(D)` are their potentials respectively, then
View SolutionThe potential of an electric field `vec(E) = (y hat(i)+x hat(j))` is
View SolutionA uniform electric field exists in `x-y` plane. The potential of points `A (-2m, 2m), B(-2m, 2m)` and `C(2m, 4m)` are `4V, 16 V` and 1`2 V` respectively. The electric field is
View SolutionFour similar charges each of charge `+q` are placed at four corners of a square of side a. Find the value of integral `-int_(infty)^(a//sqrt(2)) vec(E).vec(d)r.` (in volts) if value of integral `-int_(a//sqrt(2))^(0) vec(E).vec(dr)=(sqrt(2)Kq)/(a)("where" K=(1)/(4 pi epsilon_(0)))`
View SolutionTwo infinite, parallel, nonconducting sheets carry equal positive charges density `sigma`. One is placed in the yz plane and the other at distance `x = a`. Take potential `V = 0` at `x = 0`. Then.
View SolutionAbout an electric field which of the following statements are not true ?
View Solution
DPP 3.2 questions and complete solutions for chapter ELECTRIC POTENTIAL of CENGAGE PHYSICS (ENGLISH) of Class 12