Complete Solutions to EXERCISE-1 : CONCEPT BUILDER of chapter WAVE OPTICS of Class 12 book with complete answers and questions
| Class 12 | PHYSICS |
| Chapter | WAVE OPTICS |
| DISHA PUBLICATION | EXERCISE-1 : CONCEPT BUILDER |
| Number Of Questions | 65 |
| Academic Year | 2023-24 |
According to Huygen's construction which of the following wavefront does not exists?
View SolutionNewton postulated his corpuscular theory on the basis of
View SolutionFigure shows wavefront P Passing through two systems A and B, and emerging as Q and then as R. Then systems A and B could, respectively, be
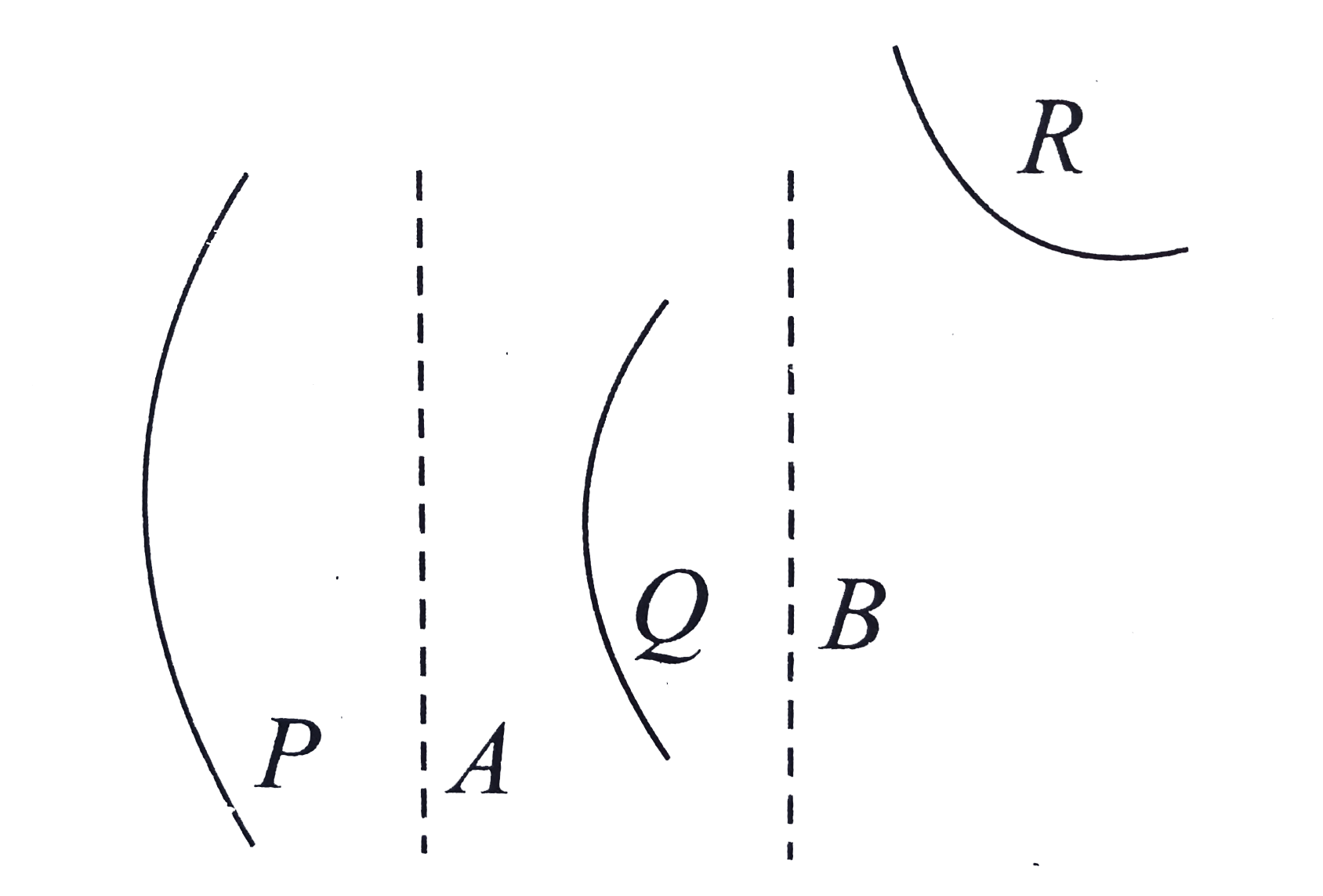
View SolutionSpherical wavefronts, emanating from a point source, strike a plane reflecting surface. What will happen to these wave fronts, immediately after reflection?
View SolutionOcean waves moving at a speed of 4.0 m/s are approaching a beach at an angle of `30^(@)` to the normal, as shown in figure. Suppose the water depth changes abruptly at a certain distance from the beach and the wave speed there drops to 3.0 m/s. Close to the angle `theta` is :

View SolutionAccording to Huygen's construction , tangential envelope which touches all the secondary spheres is the position of
View SolutionA wave front AB passing through a system C emerges as DE. The system C could be
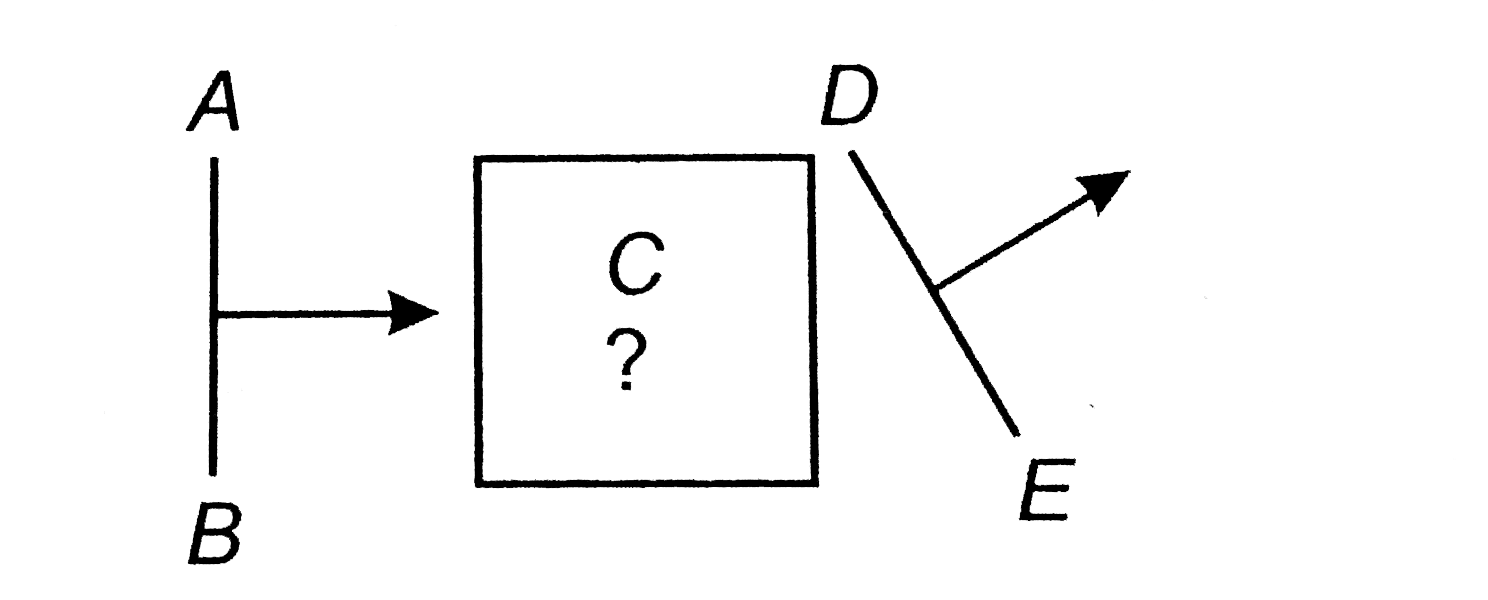
View SolutionThe wavefronts of a light wave travellilng in vacuum are given by `x+y+z=c`. The angle made by the direction of propagation of light with the X-axis is
View SolutionFind the minimum thcknessof a film which will strongly reflect the light of wavelength 589 nm. The refractive index of the material of the film is 1.25.
View SolutionA parallel beam of white light is reflected from a thin wedge shaped film. The colour of the fringe at the edge of the wedge will be
View SolutionThe path difference between two wavefronts emitted by coherent sources of wavelength 5460 Å is 2.1 micron . The phase difference between the wavefronts at that point is –
View SolutionTo deminstrate the phenimenon of interference, we require two sources which emit radiation
View SolutionWhen a thin transparent plate of thickness t and refractive index `mu` is placed in the path of one the two interfering waves of light, then the path difference changes by
View SolutionThe intensity ratio of two waves is `9:1`. If they produce interference, the ratio of maximum to minimum intensity will be
View SolutionInterference was observed in interference chamber when air was present, now the chamber is evacuated and if the same light is used, a careful observer will see
View SolutionWhite light falls normally on a film of soap water whose thickness is `5xx10^(-5)cm` and refractive index is 1.40. The wavelengths in the visible region that are reflected the most strongly are :
View SolutionTwo coherent light sources, each of wavelength `lambda`, are separated by a distance `3 lambda`, The maximum number of minima formed on line AB, which funs from `-oo` to `+oo`, is

View SolutionTwo coherent sources separated by distance d are radiating in phase having wavelength `lambda`. A detector moves in a big circle around the two sources in the plane of the two sources. The angular position of `n=4` interference maxima is given as

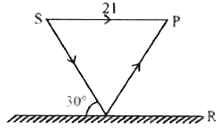
View SolutionInterference pattern is observed at 'P' due to superimposition of two rays coming out from a source 'S' as shown in the figure. The value of '1' for which maxima is obtained at 'P' is : (R is perfect reflecting surface)
View SolutionTwo coherent monochromatic light beams of intensities I and `4I` are superposed. The maximum and minimum possible intensities in the resulting beam are
View SolutionThe path difference between two interfering waves at a point on screen is 171.5 times the wavelength if the path difference is 0.01029 cm find the wavelength.
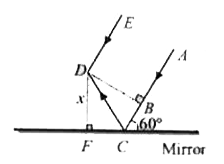
View SolutionFor the two parallel rays AB and DE shown here, BD is the wavefront. For what value of wavelength of rays destructive interference takes place between ray DE and reflected ray CD?
View SolutionIn the adjacent diagram, CP represents a wavefront and AO & BP, the corresponding two rays. Find the condition on `theta` for constructive interference at P between the ray BP and reflected ray OP.
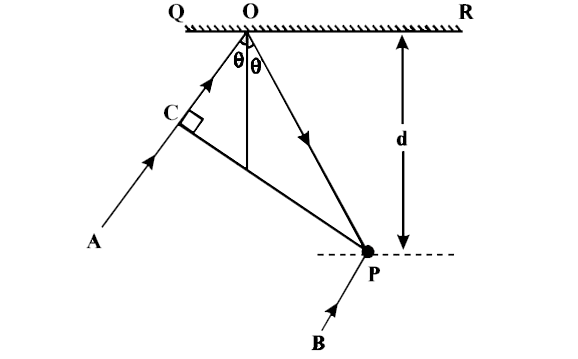
View SolutionTwo beam of light having intensities I and 4I interfere to produce a fringe pattern on a screen. The phase difference between the beams is `(pi)/(2)` at point A and `pi` at point B. Then the difference between resultant intensities at A and B is : `(2001 , 2M)`
View SolutionLight from two coherent sources of the same amplitude A and wavelength `lambda` illuminates the screen. The intensity of the central maximum is `I_(0)`. If the sources were incoherent, the intensity at the same point will be
View SolutionA point p is situated `90.50cm and 90.58` cm away from two coherent sources. The nature of illumination of the point p of the wavelength of light is 400Å is,
View SolutionFor observing interference in thin films with a light of wave length `lamda` the thickness of the film:
View SolutionSodium light `(lambda= 6xx 10^(-7)m)` is used to produce interference pattern. The observed fringe width is 0.12 mm. The angle between two interfering wave trains, is
View SolutionIn which of the following is the interference due to the division of wave front
View SolutionIn Young's double slit experiment, one slit is covered with red filter and another slit is covered by green filter, then interference pattern will be
View SolutionIn Young's double slit experiment, a minimum is obtained when the phase difference of super imposing waves is
View SolutionInstead of using two slits as in Young's experiment, if we use two separate but identical sodium lamps, which of the following will occur?
a) uniform illuminations is observed
b) widely separate interference
c) very bright maximum
d) very minimum
View SolutionThe maximum intensity of fringes in Young's experiment is I. If one of the slit is closed, then the intensity at that place becomes `I_o`. Which of the following relation is true?
View SolutionWhen we close one slit in the Young's double slit experiment then
View SolutionS is the size of the slit, d is the separation between the slits and D is the distance of slits from a plane where Young's double slit interference pattern is being observed . If `lamda` be the wavelength of light, then for sharp fringes, the essential conditional is
View SolutionDistance between screen and source is decreased by 25%. Then the percentage change in fringe width is
View SolutionIn a Young’s double slit experiment, the separation of the two slits is doubled. To keep the same spacing of fringes, the distance D of the screen from the slits should be made
View SolutionIn YDSE, how many maximas can be obtained on a screen including central maxima in both sides of the central fringe if `lamda=3000Å,d=5000Å`
View SolutionThe Young's double slit experiment is performed with blue and with green light of wavelengths `4360 A` and `5460 A` respectively. If `X` is the distance of `4th` maximum from the central one, then :
View SolutionIn Young's double slit experiment, the slits are 3 mm apart. The wavelength of light used is 5000 Å and the distance between the slits and the screen is 90 cm. The fringe width in mm is
View SolutionIn Young's experiment intensity at a point on the scrren is 75% of the maximum value. Minimum phase difference between the waves arriving at this point from the two slits will be
View SolutionIn Young's double slit experiment, `lamda=500nm`, d = 1mm, D = 1m. Minimum distance from the central maximum for which intensity is half of the maximum intensity is
View Solution_E01_080_Q01.png)
The figure shows the interfernece pattern obtained in double slit experiment using light of wavelength 600 nm.
Q. The third order bright fringe is
View SolutionIn YSDE, both slits are covered by transparent slab. Upper slit is covered by slab of R.I. 1.5 and thickness t and lower is
covered by R.I. `4/ 3` and thickness 2t, then central maxima
` (##DPP_PHY_CP24_E01_008_Q01.png" width="80%">
View SolutionIn a Young's experiment, two coherent sources are placed `0.90mm` apart and the fringes are observed one metre away. If is produces the second dark fringe at a distance of `1mm` from the central fringe, the wavelength of monochromatic light used would be
View SolutionA YDSE is conducted in water `(mu_(1))` as shown in figure. A glass plate of thickness t and refractive index `mu_(2)` is placed in the path of `S_(2)`. The optical path difference at O is
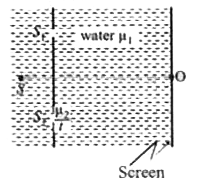
View SolutionIn YDSE, bichromatic light of wavelengths 400 nm and 560 nm
are used. The distance between the slits is 0.1 mm and the distance between the
plane of the slits and the screen is 1m. The minimum distance between two
successive regions of complete darkness is
View SolutionIn Young's double slit experiment intensity at a point is `((1)/(4))` of the maximum intersity. Angular position of this point is
View SolutionIn a Young's double-slit experiment the fringe width is `0.2mm`. If the wavelength of light used is increased by 10% and the separation between the slits if also increased by 10%, the fringe width will be
View SolutionIn Young's double slit experiment, distance between two sources is 0.1mm. The distance of screen from the sources is 20cm. Wavelength of light used is `5460 Å`. Then, angular position of first dark fringe is approximately
View SolutionA single slit diffraction pattern is obtained using a beam of red light. If the red light is replaced by the blue light, then the diffraction pattern
View SolutionFrom Brewster's law of polarisation, it follows that the angle of polarisation depends upon
View SolutionThe first diffraction minima due to a single slit diffraction is at `theta=30^(@)` for a light of wavelength `5000Å` The width of the slit is
View SolutionWhen an unpolarized light of intensity `I_(0)` is incident on a polarizing sheet, the intensity of the light which does not get transmitted is
View SolutionUnpolarised light is incident on a dielectric of refractive indexspt`sqrt 3`. What is the angle of incidence if the reflected beam is completely polarised?
View SolutionWhich of the following diagrams represent the veriation of electric field vector with time for a circularly polarised light
View SolutionA beam of light is incident on a glass slab `(mu = 1.54)` in a direction as shown in the figure. The reflected light is analysed by a polaroid prism. On rotating the polaroid, `(tan 57^(@) = 1.54)`
` (##DPP_PHY_CP24_E01_002_Q01.png" width="80%">
View SolutionUnpolarized light is incident on a plane sheet on water surface. The angle of incidence for which the reflected and refracted rays are perpendicular to each other is (`mu` of water = `4/3`)
View SolutionThe fraunhofer diffraction pattern of a single slit is formed at the focal plane of a lens of focal length 1m. The width of the slit is 0.3 mm. if the third minimum is formed at a distance of 5 mm from the central maximum then calculate the wavelength of light.
View SolutionUnpolarised light of intensity `32 Wm^(-2)` passes through three polarizer such that the transmission axis of the last polarizer is crossed with that of the first. The intensity of final emerging light is `3Wm^(-2)`. The intensity of light transmitted by first polarizer will be
View SolutionA parallel beam of light of wavelength l is incident normally on a narrow slit. A diffraction pattern is formed on a screen placed perpendicular to the direction of the incident beam. At the second minimum of the diffraction pattern, the phase difference between the rays coming from the two edges of slit is
View SolutionA single slit Fraunhofer diffraction pattern is formed with white light. For what wavelength of light the third secondary maximum in the diffraction pattern coincides with the secondary maximum in the pattern for red light of wavelength 6500 Å ?
View SolutionTwo polaroids are placed in the path of unpolarized beam of intensity `I_(0)` such that no light is emitted from the second polarid. If a third polaroid whose polarization axis makes an angle `theta` with the polarization axis of first polaroid, is placed between these two polariods then the intensity of light emerging from the last polaroid will be
View SolutionA beam of unpolarised light of intensity `I_0` is passed through a polaroidA and then through another polaroid B which is oriented so that its principal plane makes an angle of `45^circ` relative to that of A. The intensity of the emergent light is
View SolutionA beam of light of `lambda` = 600 nm from a distant source falls on a single slit 1 mm wide and the resulting diffraction pattern is observed on a screen 2 m away. The distance between first dark fringes on either side of the central bright fringe is
View Solution
EXERCISE-1 : CONCEPT BUILDER questions and complete solutions for chapter WAVE OPTICS of DISHA PUBLICATION PHYSICS (HINGLISH) of Class 12