Complete Solutions to Exercises of chapter GEOMETRICAL OPTICS of Class 12 book with complete answers and questions
| Class 12 | PHYSICS |
| Chapter | GEOMETRICAL OPTICS |
| HC VERMA | Exercises |
| Number Of Questions | 79 |
| Academic Year | 2023-24 |
A concave mirror having a radius of curvature 40 cm is placed in front of an illuminated point source at a distance of 30 cm from it. Find the location of the image.
View SolutionA concave mirror forms an image of 20 cm high object on a screen placed 5.0 m away from the mirror. The height of the image is 50 cm. Find the focal length of the mirror and the distance between the mirror and the object.
View SolutionA concave mirror has a focal length of 20 cm. Find the position or positions of an object for which the image-size is double of the object-size.
View SolutionA 1 cm object is placed perpendicular to the principal axis of a convex mirror of focal length 7.5 cm. Find its distance from the mirror if the image formed is 0.6 cm in size.
View SolutionA candle flame 1.6 cm high is imaged in a ball bearing of diameter 0.4 cm. If the ball bearing is 20 cm away from the flame, find the location and the height of the image.
View SolutionA 3 cm tall object is placed at a distance of 7.5 cm from a convex mirror of focal length 6 cm. Find the location, size and nature of the image.
View SolutionA U-shaped wire is placed before a concave mirror having radius of curvature 20cm as shown in figure,. Find the total length of the image.

View SolutionA man uses a concave mirror for shaving. He keeps his face at a distance of 25 cm from the mirror and gets an image which is 1.4 times enlarged. Find the focal lengt.1 of the mirror
View SolutionFind the diameter of the image of the moon formed by a spherical concave mirror of focal length 7.6 m. The diameter of the moon is 3450 km and the distance of the earth and the moon is `3.8 xx 10^5 km.`
View SolutionA particle goes in a circle of radius 2.0 cm. A concave mirror of focal length 20 cm is placed with its principal axis passing through the centre of the circle and perpendicular to its plane. The distance between the pole of the mirror and the centre of the circle is 30 cm. Calculate the radius of the circle formed by the image.
View SolutionA concave mirror of radius R is kept on a horizontal table. Water (refractive index =mu.) is poured into it up to a height h. Where should an object be placed so that its image is formed on itself ?

View SolutionA point source S is placed midway between two converging mirrors having equal focal length f as shown in figure. Find the values of d for which only one image is formed.

View SolutionA converging mirror `M_1` a point source S and a diverging mirror `M_2` are arranged as shown in figure. The source is placed at a distance of 30 cm from `M_1`. The focal length of each of the mirrors is 20 cm. Consider only the images formed by a maximum of two reflections. It is found that one image is formed on the source itself. (a) Find the distance between the two mirrors. (b) Find the location of the image formed by the single reflection from `M_2

View SolutionA light ray falling at an angle of` 45^@` with the surface of a clean slab of ice of thickness 1.00 m is refracted into it at an angle of 30°. Calculate the time taken by the light rays to cross the slab. Speed of light in vacuum `= 3 xx 10^8ms^-1`
View SolutionA pole of length 1.00 m stands half dipped in a swimming pool with water level 50.0 cm higher than the bed. The refractive index of water is 1.33 and sunlight is coming at an angle of 45° with the vertical. Find the length of the shadow of the pole on the bed.
View SolutionA small piece of wood is floating on the surface of a 2.5 m deep lake. Where does the shadow form on the bottom when the sun is just setting? Refractive index of water = 4/3
View SolutionAn object P is focussed by a microscope M. A glass slab of thickness 2'1 cm is introduced between P and M. If the refractive index of the slab is P5, by what distance should the microscope be shifted to focus the object again ?
View SolutionA vessel contains water up to a height of 20 cm and above it an oil up to another 20 cm. The refractive indices of the water and the oil are 1.33 and F30 respectively. Find the apparent depth of the vessel when viewed from above.
View SolutionLocate the image of the point P as seen hy the eye in the figure

View Solutionk transparent slabs are arranged one over another. The refractive indices of the slabs are `mu_1, mu_2, mu_3, ............. mu_k` and the thicknesses are `t_1, t_2, t_3............., t_k` . An object is seen through this combination with nearly perpendicular light. Find the equivalent refractive index of the system which will allow the image to be formed at the same place.
View SolutionA cylindrical vessel of diameter 12 cm contains 800n cm 3 of water. A cylindrical glass piece of diameter 8.0 cm and height 8.0 cm is placed in the vessel. If the bottom of the vessel under the glass piece is seen by the paraxial rays (see figure 18-E6), locate its image. The index of refraction of glass is 1.50 and that of water is 1.33.

View SolutionConsider the situation in figure. The bottom of the pot is a reflecting plane mirror, S is a small fish and T is a human eye. Refractive index of water is mu. (a) At what distance(s) from itself will the fish see the image(s) of the eye ? (b) At what distance(s) from itself will the eye see the image(s) of the fish. (11-T

View SolutionA small object is placed at the centre of the bottom of a cylindrical vessel of radius 3 cm and height 4 cm filled completely with water. Consider the ray leaving the vessel through a corner. Suppose this ray and the ray along the axis of the vessel are used to trace the image. Find the apparent depth of the image and the ratio of real depth to the apparent depth under the assumptions taken. Refractive index -of water = 1-33.
View SolutionA cylindrical vessel, whose diameter and height both are equal to 30 cm, is placed on a horizontal surface and a small particle P is placed in it at a distance of 5.0 cm from the centre. An eye is placed at a position such that the edge of the bottom is just visible (see figure 18-E8). The particle P is in the plane of drawing. Up to what minimum height should water be poured in the vessel to make the particle P visible ?

View SolutionA light ray is incident at an angle of `45^@` with the normal to a `sqrt2` cm thick plate` (mu2.0)`. Find the shift in the path of the light as it emerges out from the plate.
View SolutionAn optical fibre (mu = 1.72) is surrounded by a glass coating (mu= 1.50). Find the critical angle for total internal reflection at the fibre-glass interface.
View SolutionA light ray is incident normally on the face AB of a right-angled prism `ABC (mu==1.50)` as shown in figure. What is the largest angle `phi` for which the light ray is totally reflected at the surface AC ?

View SolutionFind the maximumsngle of refraction when a gat ray is refracted from glass `(mu= 1.50)` to air.
View SolutionLight is incident from glass `(mu=1.5)` to air. Sketch thevariation of the angle of deviation `delta` with angle of incident i for `0ltilt90^@`
View SolutionLight is incident from glass `(mu = 1.50)` to water `(mu = 1.33)` find the range of the angle of deviation for which there are two angles of incidence.
View SolutionLight falls from glass `(mu=1.5)` to air. Find the angle of incidence for which the angle of deviation is `90^@`.
View SolutionA point source is placed at a depth h below the surface of water (refractive index = mu). (a) Show that light escapes through a circular area on the water surface with its centre directly above the point source. (b) Find the angle subtended by a radius of the area on the source.
View SolutionA container contains water up to a height of 20 cm and there is a point source at the centre of the bottom of the container. A rubber ring of radius r floats centrally on the water. The ceiling of the room is 2.0 m above the water surface. (a) Find the radius of the shadow of the ring formed on the ceiling if r = 15 cm. (b) Find the maximum value of r for which the shadow of the ring is formed on the ceiling. Refractive index of water =4/3.
View SolutionFind the angle of minimum deviation for an equilateral prism made of a material of refractive index 1-732. What is the angle of incidence for this deviation ?
View SolutionFind the angle of deviation suffered by the light ray shown in figure. The refractive index mu = for the prism material.
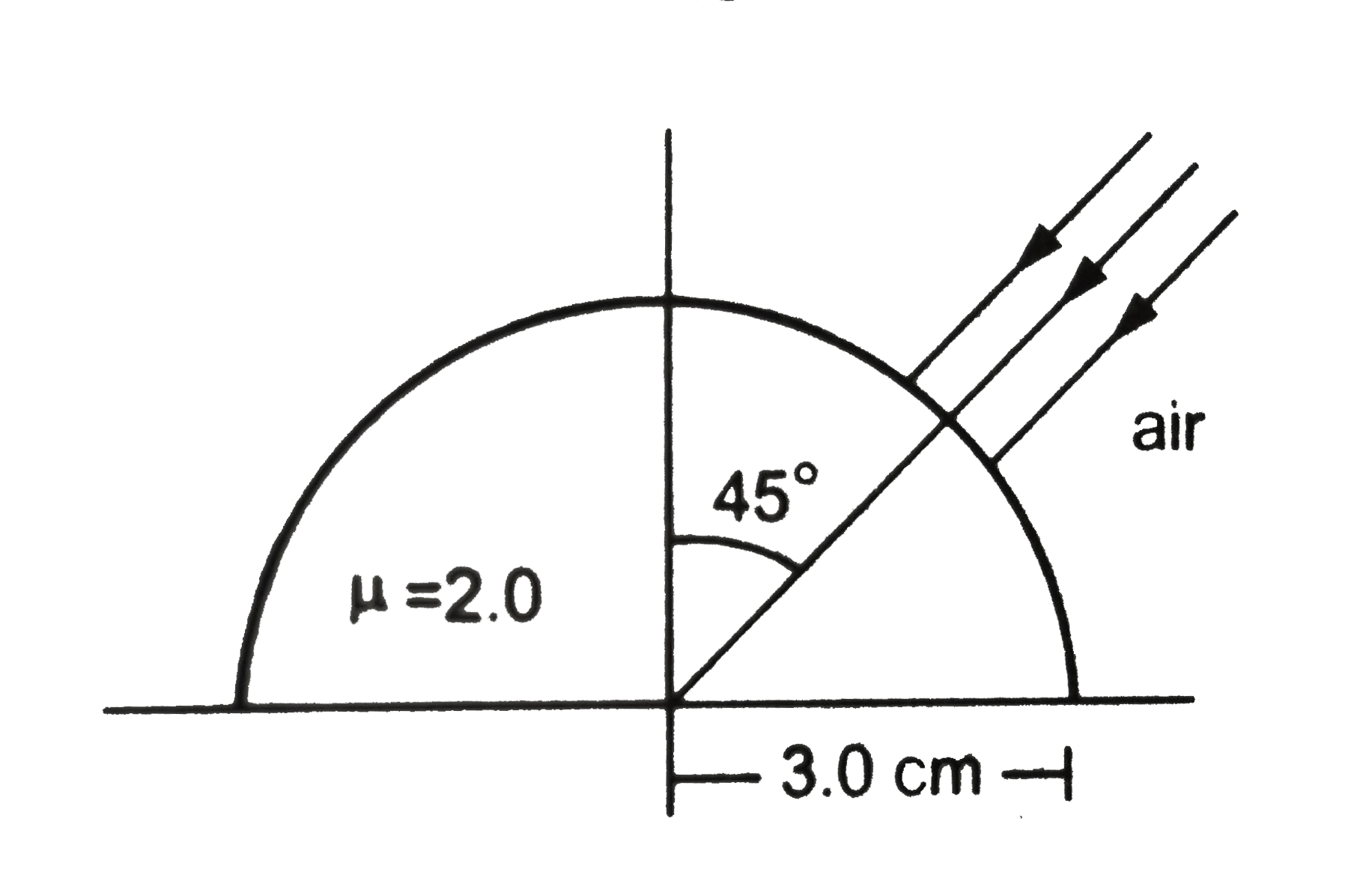
View SolutionA light ray, going through a prism with the angle of prism `60^@`, is found to deviate by ` 30^@`. What limit on the refractive index can be put from these data ?
View SolutionLocate the image formed by refraction in the situation shown in figure
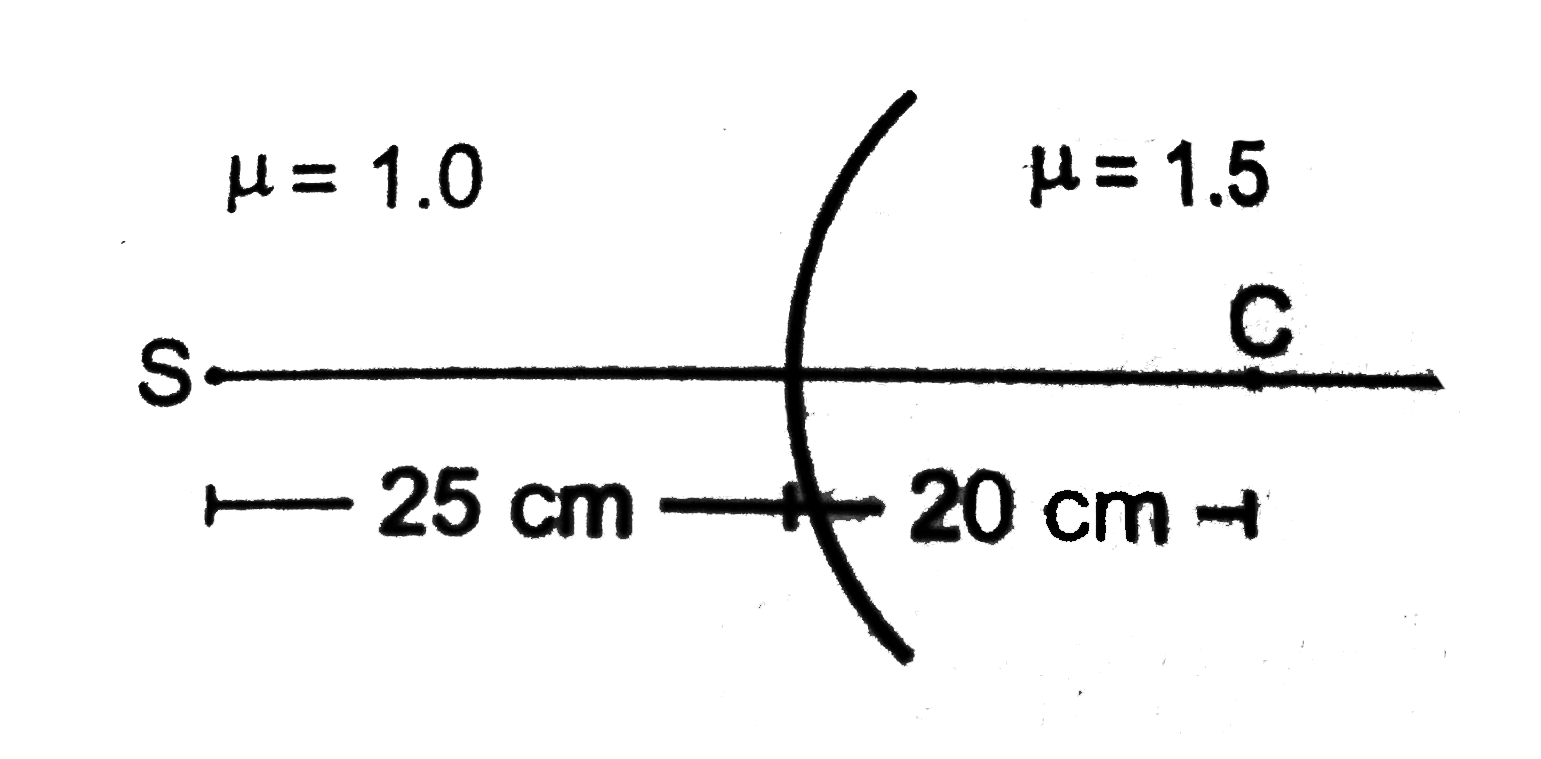
View SolutionA spherical surface of radius 30 cm separates two transparent media A and B with refractive indices 1'33 and 1.48 respectively. The medium A is on the convex side of the surface. Where should a point object be placed in medium A so that the paraxial rays become parallel after refraction at the surface ?
View SolutionFigure shows a transparent hemisphere of radius 3'0 cm made of a material of refractive index 2.0. (a) A narrow beam of parallel rays is incident on the hemisphere as shown in the figure. Are the rays totally reflected at the plane surface ? (b) Find the image formed by the refraction at the first surface. (c) Find the image formed by the reflection or by the refraction at the plane surface. (d) Trace qualitatively the final rays as they come out of the hemisphere.

View SolutionA small object is embedded in a glass sphere (mu =1.5) of radius 5.0 cm at a distance 1.5 cm left to the centre. Locate the image of the object as seen by an observer standing (a) to the left of the sphere and (b) to the right of the sphere.
View SolutionA biconvex thick lens is constructed with glass (mu = 1.50). Each of the surfaces has a radius of 10 cm and the thickness at the middle is 5 cm. Locate the image of an object placed far away from the lens.
View SolutionA narrow pencil of parallel light is incident normally on a solid transparent sphere of radius r. What should be the refractive index if the pencil is to be focused (a) at the surface of the sphere, (b) at the centre of the sphere.
View SolutionOne end of a cylindrical glass rod (mu = 1.5) of radius 1.0 cm is rounded in the shape of a hemisphere. The rod is immersed in water (mu= 4/3) and an object is placed in the water along the axis of the rod at a distance of 8.0 cm from the rounded edge. Locate the image of the object.
View SolutionA paperweight in the form of a hemisphere of radius 3.0 cm is used to hold down a printed page. An observer looks at the page vertically through the paperweight. At what height above the page will the printed letters near the centre appear to the observer ?
View SolutionSolve the previous problem if the paperweight is inverted at its place so that the spherical surface touches the paper
View SolutionA hemispherical portion of the surface of a solid glass sphere (mu = 1.5) of radius r is silvered to make the inner side reflecting. An object is placed on the axis of the hemisphere at a distance 3r from the centre of the sphere. The light from the object is refracted at the unsilvered part, then reflected from the silvered part and again refracted at the unsilvered part. Locate the final image formed.
View SolutionThe convex surface of a thin concave-convex lens of glass of refractive index 1.5 has a radius of curvature 20 cm. The concave surface has a radius of curvature 60 cm. The convex side is silvered and placed on a horizontal surface as shown in figure. (a) Where should a pin be placed on the axis so that its image is formed at the same place ? (b) If the concave part is filled with water (mu = 4/3), find the distance through which the pin should be moved so that the image of the pin again coincides with the pin.

View SolutionA double convex lens has focal length 25 cm. The radius of curvature of one of the surfaces is double of the other. Find the radii, if the refractive index of the material of the lens is 1.5.
View SolutionThe radii of curvature of a lens are + 20 cm and + 30 cm. The material of the lens has a refracting index 1-6. Find the focal length of the lens (a) if it is placed in air, and (b) if it is placed in water (mu = 1.33).
View SolutionLenses are constructed by a material of refractive indeic 1'50. The magnitude of the radii of curvature are 20 cm and 30 cm. Find the focal lengths of the possible lenses with the above specifications.
View SolutionA thin lens made of a material of refractive index`mu_2` has a medium of refractive index `mu_1` on one side and a medium of refractive index on the other side. The lens is biconvex and the two radii of curvature have equal magnitude R. A beam of light travelling parallel to the principal axis is incident on the lens. Where will the image be formed if the beam is incident from (a) the medium p, and (b) from the medium `mu_3`?
View SolutionA convex lens has a focal length of 10 cm. Find the location and nature of the image if a point object is placed on the principal axis at a distance of (a) 9.8 cm, (b) 10.2 cm from the lens.
View SolutionA slide projector has to project a 35 mm slide `(35 mm xx 23 mm)` on a 2 m xx 2m screen at a distance of 10 m from the lens. What should be the focal length of the lens in the projector ?
View SolutionA particle executes a simple harmonic motion of amplitude 1.0 cm along the principal axis of a convex lens of focal length 12 cm. The mean position of oscillation is at 20 cm from the lens. Find the amplitude of oscillation of the image of the particle.
View SolutionAn extended object is placed at a distance of 5.0 cm from a convex lens of focal length 8.0 cm. (a) Draw the ray diagram (to the scale) to locate the image and from this, measure the distance of the image from the lens, (b) Find the position of the image from the lens formula and see how close the drawing is to the correct result.
View SolutionA pin of length 2.00 cm is placed perpendicular to the principal axis of a converging lens. An inverted image of size 1.00 cm is formed at a distance of 40.0 cm from the pin. Find the focal length of the lens and its distance from the pin.
View SolutionA convex lens produces a double size real image when an object is placed at a distance of 18 cm from it. Where should the object be placed to produce a triple size real image ?
View SolutionA pin of length 2.0 cm lies along the principal axis of a converging lens, the centre being at a distance of 11 cm from the lens. The focal length of the lens is 6 cm. Find the size of the image.
View SolutionThe diameter of the sun is `1.4 x 10^9m` and its distance from the earth is `1.5 x 10^11 m`. Find the radius of the image of the sun formed by a lens of focal length 20 cm.
View SolutionA 5.0 diopter lens forms a virtual image which is 4 times the object placed perpendicularly on the principal axis of the lens. Find the distance of the object from the lens.
View SolutionA diverging lens of focal length 12 cm and a converging mirror of focal length 10 cm are placed coaxially at a separation of 5 cm. Where should an object be placed so that a real image is formed at the object itself ?
View SolutionA converging lens of focal length 12 cm and a diverging mirror of focal length 7.5 cm are placed 5.0 cm apart with their principal axes coinciding. Where should an object be placed so that its image falls on itself ?
View SolutionA converging lens and a diverging mirror are placed at a separation of 15 cm. The focal length of the lens is 25 cm and that of the mirror is 40 cm. Where should a point source be placed between the lens and the mirror so that the light, after getting reflected by the mirror and then getting transmitted by the lens, comes out parallel to the principal axis ?
View SolutionA converging lens of focal length 15 cm and a converging mirror of focal length 10 cm are placed 50 cm apart with common principal axis. A point source is placed in between the lens and the mirror at a distance of 40 cm from the lens. Find the locations of the two images formed.
View SolutionConsider the situation described in the previous problem. Where should a point source be placed on the principal axis so that the two images form at the same place ?
View SolutionA converging lens of focal length 15 cm and a converging mirror of focal length 10 cm are placed 50 cm apart. If a pin of length 2.0 cm is placed 30 cm from the lens farther away from the mirror, where will the final image form and what will be the size of the final image ?
View SolutionA point object is placed on the principal axis of a convex lens (f = 15 cm) at a distance of 30 cm from it. A glass plate `(mu= 1.50)` of thickness 1 cm is placed on the other side of the lens perpendicular to the axis. Locate the image of the point object.
View SolutionA convex lens of focal length 20 cm and a concave lens of focal length 10 cm are placed 10 cm apart with their principal axes coinciding. A beam of light travelling parallel to the principal axis and having a beam diameter 5.0 mm, is incident on the combination. Show that the emergent beam is parallel to the incident one. Find the beam diameter of the emergent beam.
View SolutionA diverging lens of focal length 20 cm and a converging lens of focal length 30 cm are placed 15 cm apart with their principal axes coinciding. Where should an object be placed on the principal axis so that its image formed at infinity ?
View SolutionA 5 mm high pin is placed at a distance of 15 cm from a convex lens of focal length 10 cm. A second lens of focal length 5 cm is placed 40 cm from the first lens and 55 cm from the pin. Find (a) the position of the final image, (b) its nature and (c) its size.
View SolutionA point object is placed at a distance of 15 cm from a convex lens. The image is formed on the other side at a distance of 30 cm from the lens. When a concave lens is placed in contact with the convex lens, the image shifts away further by 30 cm. Calculate the focal lengths of the two lenses.
View SolutionTwo convex lenses each of focal length 10 cm, are placed at a saparation of 15 cm with their principal axes coinciding. (a) Show that a light beam coming parallel to the principal axis diverges as it comes out of the lens system. (b) Find the location of the virtual image formed by the lens system of an object placed far away. (c ) Find the focal lenth of the equivalent lens.
View SolutionA ball is kept at a height h above the surface of a heavy transparent sphere made of a material of refractive index The radius of the sphere is R. At t = 0, the ball is dropped to fall normally on the sphere. Find the speed of the image formed as a function of time for `tlt sqrt((2h)/g)`. Consider only the image by a single refraction.
View SolutionA particle is moving at a constant speed V from a large distance towards a concave mirror of radius R along its principal axis. Find the speed of the image formed by the mirror as a function of the distance x of the particle from the mirror.
View SolutionA small block of mass m and a concave mirror of radius R fitted with a stand lie on a smooth horizontal table with a separation d between them. The mirror together with its stand has a mass m. The block is pushed at t = 0 towards the mirror so that it starts moving towards the mirror at a constant speed V and collides with it. The collision is perfectly elastic. Find the velocity of the image (a) at a time `t lt d/V,` (b) at a time `t gt d/V`.
View SolutionA gun of mass M fires a bullet of mass m with a horizontal speed V. The gun is fitted with a concave mirror of focal length f facing towards the receding bullet. Find the speed of separation of the bullet and the image just after the gun was fired.
View SolutionA mass m = 50 g is dropped on a vertical spring of spring constant 500 N nfl from a height h= 10 cm as shown in figure (18-E14). The mass sticks to the spring and executes simple harmonic oscillations after that. A concave mirror of focal length 12 cm facing the mass is fixed with its principasl axis coinciding with the lilne of motion of the mss, its pole being at a distance of 30 cm from the free end of the spring. Find the length in which the image of the mass oscillates

View SolutionTwo concave mirrorsof equal radi of curvature R are fixed on a stand facing opposite directions. The whole system has a mass m nd is kept onn a frictionlesss horiztonal table figure

Two blocks A and B, each of mass m, are placed on the two side of the stnd. At t=0, teh separation between A and the mirrors is 2R and also the separation between B and the mirrors is 2R. The block B moves towards the mirror at a speed v. All collisions which take place are erastic. Taking the original position of the mirrors stand system to be x=0 and X -axis along AB, find the position of the image of A and B at t=
`a. R/(upsilon)`, b. `(3R)/(upsilon)` c. `(5R)/(upsilon)`
View SolutionConsider the stituation shown in figure. The elevator is going up with an acceleration of 2.00 ms^-2 and the focal length of the mirror is 12.0 cm. All the surfaces are smooth and the pulley is light. The mass-pulley system is released from rest (with respect to the elevator) at t=0 when the distance of B from the mirror is 42.0 cm. Find the distance between the image of the block B and the mirror at t=0.200 s. Take `g=10 ms^-2`

View Solution
Exercises questions and complete solutions for chapter GEOMETRICAL OPTICS of HC VERMA PHYSICS (HINGLISH) of Class 12