Complete Solutions to Dispersion And Absorption Of Light of chapter OPTICS of Class 12 book with complete answers and questions
| Class 12 | PHYSICS |
| Chapter | OPTICS |
| IE IRODOV | Dispersion And Absorption Of Light |
| Number Of Questions | 24 |
| Academic Year | 2023-24 |
A free electron is located in the field of a monochromatic light wave. The intensity of light is `I = 150 W//m^(2)`, its frequency is `omega = 3.4 . 10^(15) s^(-1)`. Find:
(a) the electron's oscillation amplitude and its velocity amplitude,
(b) the ratio `F_(m)//F_(e)`, where `F_(m)` and `F_(e)` are the amplitudes of forces with which the magnetic and electric components of the ligth wave field act on the electron, demonstrate that the ratio is equal to `(1)/(2)v//c`, where `v` is the electron's velocity amplitude and `c` is the velocity of light.
Instruction. The action of the magnetic field component can be disregarded in the equation of motion of the electron since the calculations shown it to be negligible.View SolutionAn electromagnetic wave of frequency `omega` propagates in dilute plasma. The free electron concentration in plasma is equal to `n_(0)`. Neglecting the interaction of the wave and plasma ions, find:
(a) the frequency dependence of plasma permittivity,
(b) how the phase velocity of the electromagnetic wave depends on its wavelength `lambda in plasma.05:48View SolutionFind the free electron concentration in ionsphere if its refractive index is equal to `n = 0.90` for radiowaves of frequency `v = 100 MHz`.
03:24View SolutionAssuming electrons of substance to be free when subjected to hard `X`-rays, determine by what magnitude the refractive index of graphite differs from unity in the case of `X-`rays whose wavelength in vaccume is equal to `lambda = 50 pm`.
04:55View SolutionAn electron experiences a quasi-elastic force `kx` and `a` ''friction force'' `yx` in the field of electromagnetic radiation. The `E`component of the field varies as `E = E_(0) cos omegat`. Neglecting the action of the magnetic component of the field, find,
(a) the motion equation of the electron,
(b) the mean power absored by the electron, the frequency at which that power is maximum and the expression for the maximum mean power.View SolutionIn some cases permittivity of substance turns out to be a complex or a negative quantity, and refractive index, respectively, a complex `(n' = n+ ix)` or an imaginary `(n' = ix)` quantity. Write the equation of a plane wave for both of these cases and find out the physical meaning of such refractive indices.
View SolutionA sounding of dilute plasma by radiowaves of various frequencies reveals that rediowaves with wavelength exceeding `lambda_(0) = 0.75m` experience total internal reflection. Find the free electron concentration in that plasma.
03:50View SolutionUsing the definition of the group velocity `u`, derive Rayleigh's fromula `(5.5d)`. Demonstrate that in the vicinity of `lambda = lambda'` the velocity `u` is equal to the segment `upsilon'` cut by the tangent of the curve `upsilon(lambda)` at the point `lambda`' (Fig.)
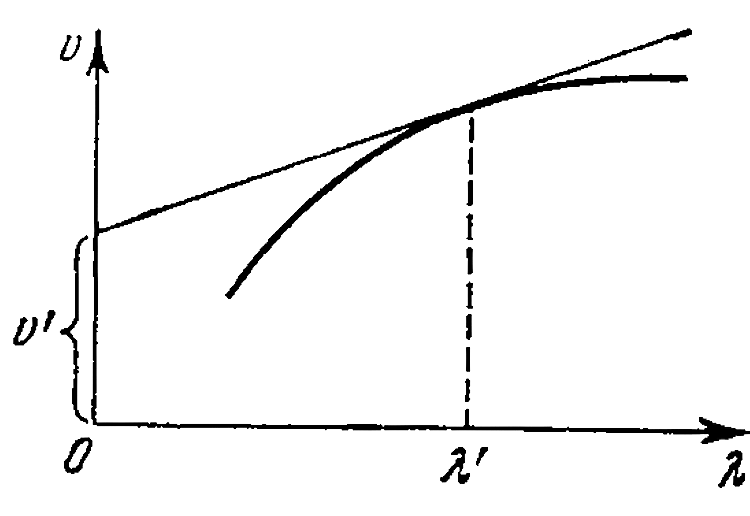 View Solution
View SolutionFind the relation between the group velocity `u` and phase velocity `upsilon` for the following disperison laws:
(a) `upsilon prop 1//sqrt(lambda_(1))`,
(b) `upsilon prop k`,
(c ) `upsilon prop 1// omega^(2)`,
Here `lambda, k`, and `omega` are the wavelength, wave number, and angular frequancy.04:53View SolutionIn a certain medium the relationship between the group and phase velocities of an electromagnetic wave has the form `uv = c^(2)`, where `c` is the velocity of light in vaccume. Find the dependence of permittivity of that medium on wave frequency, `epsilon(omega)`.
01:38View SolutionThe refractive index of carbon dioxide at the wavelengths `509, 534` and `589 nm` is equal to `1.647, 1.640`, and `1.630` respectively. Calculate the phase and group velocities of light in the vicinity of `lambda = 534 nm`.
View SolutionA train of plane light waves propagates in the medium where the phase velocity `v` is a linear function of wavelength: `v = 1 + blambda`, where `a` and `b` are some positive constants. Demonstrate that in such a medium the shape of an arbitary train of light waves is restroed after the time interval `tau = 1//b`.
04:08View SolutionA beam of natural light of intensity `I_(0)` falls on a system of two crossed Nicol prisms between which a tube filled with certain solution is placed in a longitudinal magnetic field of strength `H`. The length of the tube is `l`, the coefficient of linear absorption of solution is `x`, and the verder constant is `V`. Find the intensity of light transmitted through that system.
03:22View SolutionA plane monochromatic light wave of intensity `I_(0)`falls normally on a plane-parallel plate both of whose surfaces have a reflection coeffiecient `rho`. Taking into account multiple reflections, find the intensity of the transmitted light if
(a) the plate is perfectly transparent, i.e the absorption is absent,
(b) teh coefficient of linear absorption is equal to `x`, and the plate thickness is `d`.View SolutionTwo plates, one of thickness `d_(1) = 3.8 mm` and the other oof thickness `d_(2) = 9.0 mm`, are manufactured from a certain substance. When placed alternately in the way of monichromatic light, the first trasmits `tau_(1) = 0.84` fraction of luminous flux and that substance. Light falls at right angles to the plates. The secondary reflections are to be neglected.
03:39View SolutionA beam of monochromatic light passes through a pile of `N = 5` identical plane-parallel glass plates each of thickness `l = 0.50 cm`. The coefficient of reflection at each surface of the plates is `rho = 0.050`. The ratio of the intensity of light transmitted through the pile of plates to the intensity of incident light is `tau = 0.55` Neglecting the secondary reflections of light, find the absorption coefficient of the given glass.
03:55View SolutionA beam of monichromatic light falls normally on the surfcae of a plane-parallel plate of thickness `l`. The absorption coefficient of the substance the plate is made of varies linearly along the normal to its surfcae from `x_(1)` to `x_(2)`. The coefficient of reflection at each surface of the plate is equal to `rho`. Neglecting the secondary reflections, find the transmission coefficient of such a plate.
View SolutionA beam of light of intensity `I_(0)` falls normally on a transparent plane-parallel plate of thickness `l`. The beam contains all the wavelengths in the interval from `lambda_(1)` to `lambda_(2)` of equal spectral intensity. Find the intensity of the transmitted beam if in this wavelength interval the absorption coefficient is a linear function of `lambda`, with exterme values `x_(1)` and `x_(2)`. The coefficient of reflection at each surfcae is equal to `rho`. The secondary reflections are to be neglected.
05:46View SolutionA light filter is a plate of thickness `d` whose absorption coefficient depends on wavelength `lambda` as
`x(lambda) = alpha (1-lambda//lambda_(0))^(2) cm^(-1)`,
where `alpha` and `lambda_(0)` are constants. Find the passband `Delta lambda` of this light filter, that is the band at whose edge the attenuation of light is `eta` times that at the wavelength `lambda_(0)`. The coefficient of reflection from teh surfaces of the light filter is assumed to be the same at all wavelengths.03:24View SolutionA point source of monochromatic light emitting a lumninous flux `Phi` is positioned at the cnetre of a spherical layer of substance. The inside radius of the layer is `a`, the outside one is `b`. The coefficient of linear absorption of the substance is equal to `x`, the reflection coefficient of the surfcaes is equal to `rho`. Neglecting the secondary reflections, find the intensity of light taht passes through that layer.
View SolutionHow many times will the intensity of a narrow `X`-ray beam of wavelength `20pm` decrease after passing through a lead plate of thickness `d = 1.0 mm` if the mass absorption coefficient for the given radiation wavelength is equal to `mu//rho = 3.6 cm^(2)//g`?
02:36View SolutionA narrow beam of `X-`ray radiation of wavelength `62pm` penetrates an aluminium screen `2.6 cm` thick. How thick must a lead screen be to attenuate the beam just as much? The mass absorption coefficients of aluminium and lead for this radiations are equal to `3.48` and `72.0 cm^(2)//g` respectively.
04:30View SolutionFind the thickness of aluminium layer which reduces by half the intensity of a narrow monochromatic `X`-ray beam if the corresponding mass absorption coefficient is `mu//rho = 0.32 cm^(2)//g`.
02:26View SolutionHow many `50%` absorption layers are there in the plate reducing the intensity of a narroe `X`-ray beam `eta = 50` times?
02:41View Solution
Dispersion And Absorption Of Light questions and complete solutions for chapter OPTICS of IE IRODOV PHYSICS (HINGLISH) of Class 12
