Complete Solutions to EXAMPLES of chapter PROBABILITY of Class 12 book with complete answers and questions
| Class 12 | MATHS |
| Chapter | PROBABILITY |
| MODERN PUBLICATION | EXAMPLES |
| Number Of Questions | 77 |
| Academic Year | 2023-24 |
A four digit number is formed using the digits 1, 2, 3, 5 with no repetitions. Write the probability that the number is divisible by 5.
View SolutionIf a leap year is selected at random, what is the chance that it will contain 53 wednesday ?
View SolutionIf `P(A)=(6)/(11),P(B)=(5)/(11)` and `P(A uu B)=(7)/(11)`, find `P(A//B)`.
View SolutionIf `P(A//B)=0.8,P(B//A)=0.6` and `P(A^( C )uu B^( C ))=0.7`, then find the value of `P(A uu B)`.
View SolutionA black and a red die are rolled together. Find the conditional brobability of obtaining the sum 8, given that the red die resulted a number less than 4.
View SolutionTen cards numbered1 through 10 are placed in a box, mixed up thoroughly and then one card is drawn randomly. If it is known that the number on the drawn card is more than 3, what is the probability that it is an even number.
View SolutionConsider the experiment of tossing a coin. If the coin shows head, toss it again but if it shows tail then throw a die. Find the conditional probability of the event that the die shows a number greater than 4 given that there is at least one tail
View SolutionA fair coin and an unbiased die are tossed. Let A be the event head appears on the coin and B be the event 3 on the die. Check whether A and B are independent events or not.
View SolutionA die marked 1, 2, 3 in red and 4, 5, 6 in green is tossed. Let A be the event, the number is even, and B be the event, the number is red. Are A and B independent?
View SolutionIf `P(A)=3/5" P(B)"=(5)/(7)`, where A and B are independent events, then find `P(A uu B)` and `P(A//B)`.
View SolutionIf A and B are independent events such that `P(A)=(3)/(10),P(B)=(2)/(5)`, then find :
(i) P(A and B) and (ii) P(A or B).
View SolutionOne card is drawn from a pack of 52 cards so that each card is equally likely to be selected. Prove that the following cases are independent :
(a) A : "The card drawn is a spade"
B : "The card drawn is an ace."
(b) A : "The card drawn is black"
B : "The card drawn is a king."
View SolutionAn unbiased die is thrown twice. Let the event A be "odd number on the first throw" and B the event "odd number on the second throw". Check the independence of the events A and B.
View SolutionThree coins are tossed simultaneously. Consider the events :
E : 'three heads or three tails'
F : 'at least two heads'
G : 'at most two heads'.
Of the pairs (E, F), (E, G) and (F, G), which are independent, which are dependent ?
View SolutionA die marked 1, 2, 3 in red and 4, 5, 6 in green is tossed. Let A be the event, the number is even, and B be the event, the number is red. Are A and B independent?
View SolutionA husband and his wife appear for an interview for two posts. The probability of husband's selection is `(1)/(7)` and that of wife's selection is `(1)/(5)`. What is the probability that only one of them is selected ?
View SolutionIn a hockey match, both teams A and B scored same number of goals upto the end of the game, so to decide the winner, the referee asked both the captains to throw a die alternately and decided that the team whose captain gets a six first, will be declared the winner. If the captain of team A was asked to start, then find their respective probabilities of winning the match and state whether the decision of the referee was fair or not.
View SolutionA speaks truth in 60% of cases, while B in 90% of the cases. In what percent of cases are they likely to contradict each other in stating the same fact?
View SolutionThe probabilities of two students A and B coming to the school in time are `3/7"\ "` and `5/7` respectively. Assuming that the events, A coming in time and B coming in time are independent, find the probability of only one of them coming to the school in time. Write at least one advantage of coming to school in time.
View SolutionA and B throw a pair of dice alternately. A wins the game if he gets a total of 7 and B wins the game if he gets a total of 10. If A starts the game, then find the probability that B wins.
View SolutionIf A and B are two independent events such that :
`P(bar(A)nn B)=(2)/(15)` and `P(A nn bar(B))=(1)/(6)`, then find P(A) and P(B).
View SolutionHow many times must a man toss a fair coin, so that the probability of having at least one head is more than 80%?
View SolutionA and B appeared for an interview. The probability of their selection is `(1)/(2)` and `(1)/(3)` respectively. Find the probability that :
(i) both selected
(ii) at least one of them selected.
View SolutionThe probability of solving a specific problem independently by A and B are `(1)/(3)` and `(1)/(5)` respectively. If both try to solve the problem independently, find the probability that the problem is solved.
View SolutionA problem in Mathematics is given to three students whose chances of solving it are `(1)/(2),(1)/(3),(1)/(4)`.
What is the probability in the following cases ?
(i) that the problem is solved
(ii) only one (exactly one) of them solves it correctly
(iii) at least one of them may solve it.
View SolutionIn a set of 10 coins, 2 coins are with heads on both the sides. A coin is selected at random from this set and tossed five times. If all the five times, the result was heads, find the probability that the selected coin had heads on both the sides.
View SolutionA person has undertaken a construction job. The probabilities are 0.65 that there will be strike. 0.80 that the construction job will be completed on time if there is no strike, and 0.32 that the construction job will be completed on time if ther
View SolutionThere are two bags I and II. Bag I contains 4 white and 3 red balls while another Bagt II contains 3 white and 7 red balls. One ball is drawn at random from one of the bags and it is found to be white. Find the probability that it was drawn from bag I.
View SolutionA bag X contains 4 white balls and 2 black balls, while another bag Y contains 3 white balls and 3 black balls. Two balls are drawn (without replacement) at random from one of the bags and were found to be one white and one black. Find the probability that the balls were drawn from bag Y.
View SolutionAssume that the chances of a patient having a heart attack is 40%. Assuming that a meditation and yoga course reduces the risk of heart attack by 30% and prescription of certain drug reduces its chance by 25%. At a time a patient can choose any one of the two options with equal probabilities. It is given that after going through one of the two options, the patient selected at random suffers a heart attack. Find the probability that the patient followed a course of meditation and yoga. Interpret the result and state which of the above stated methods is more beneficial for the patient.
View SolutionAn insurance company insured 2000 scooter drivers, 4000 car drivers and 6000 truck drivers. The probability of an accident involving a scooter, a car and a truck are 0.01, 0.03 and 0.15 respectively. One of the insured persons meets with an accident. What is the probability that he is a scooter driver.
View SolutionA card from a pack of 52 playing cards is lost. From the remaining cards of the pack three cards are drawn at random (without replacement) and are found to be all spades. Find the probability of the lost card being a spade.
View SolutionThree persons A, B and C apply for a job of Manager in a Private Company. Chances of their selection (A, B and C) are in the ratio 1 : 2 : 4. The probabilities that A, B and C can introduce changes to improve profits of the company are 0.8, 0.5 and 0.3 respectively. If the change does not take place, find the probability that it is due to the appointment of C.
View SolutionOften it is taken that a truthful person commands more respect in the society. A man is known to speak the truth 4 out of 5 times. He throws a die and reports that it is a six. Find the probability that it is actually a six.
View SolutionIn a bolt factory, machines A, B and C manufacture respectively 25%, 35% and 40% of the total bolts. Of their output 5, 4 and 2 percent are respectively defective bolts. A bolt is drawn at random from the product. What is the probability that the bolt drawn is defective?
View SolutionA manufacturer has three machine operators A, B and C. The first operator A produces 1% defective items, where as the other two operators B and C produce 5% and 7% defective items respectively. A is on the job for 50% of the tune, B is on the job
View SolutionSuppose a girl throws a die. If she gets 1 or 2 , she tosses a coin three times and notes the number of tails. If she gets 3,4,5 or 6, she tosses the coin once and notes whether 'head' or 'tail' is obtained. If she obtained exactly one 'tail', what is the probability that she threw 3,4,5,or 6 with the die
View SolutionSuppose a girl throws a die. If she gets a 5 or 6, she tosses a coin three times and notes the number of heads. If she gets 1, 2, 3 or 4, she tosses a coin once and notes whether a head or tail is obtained. If she obtained exactly one head, what
View SolutionBag I contains 3 red and 4 black balls and Bag II contains 4 red and black balls. Two balls are transferred at random from Bag I to Bag II and then a ball is drawn from Bag II. The ball so drawn is found to be red in colour. Find the probability that the transferred balls were both black.
View SolutionThere are three coins. One is a coin having tails on both faces, another is a biased coin that comes up tails on both faces, anoter is a biased coin that comes up tails 70% of the time and the third is an unbiased coin. One of the coins is chosen at random and tossed, it shows tail. Find the probability that it was a coin with tail on both the faces.
View SolutionSuppose that the reliability of a HIV test is specified as follows: Of people having HIV. 90% of the test detects the disease but 10% go undetected. Of people free of HIV, 99% of the test are judged HTV-ive but 1% are diagnosed as showing H3V+ive
View SolutionOf the students in a school, it is known that 30% have 100% attendance and 70% students are irregular. Previous year results report that 70% of all students who have 100% attendance attain A grade and 10% irregular students attain A grade in their annual examination. At the end of the year, one student is chosen at random from the school and he was found to have an A grade. What is the probability that the student has 100% attendance ? Is regularity required only in school ? Justify your answer.
View SolutionThe following is a probability distribution function of a random variable :
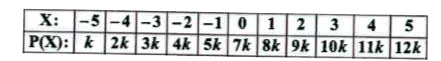
(i) Find k (ii) Find `P(X gt 3)` (iii) Find `P(-3 lt X lt 4)`
(iv) Find `P(X lt -3)`.
View SolutionA bag contains 2 white and 1 red balls. One ball is drawn at random and then put back in the box after noting its colour. The process is repeated again. If X denotes the number of red balls recorded in the two draws, describe X.
View SolutionA person plays a game of tossing a coin thrice. For each head, he is given Rs 2 by the organiser of the game and for each tail, he has to give Rs 1.50 to the organiser. Let X denotes the amount gained or lost by the person. Show that X is a rando
View SolutionA coin is biased so that the head is 3 times as likely to occur as tail. If the coin is tossed three times, find the probability distribution of number of tails.
View SolutionFind the probability distribution of `X`; the number of heads in two tosses of a coin ( or a simultaneous toss of two coins).
View SolutionFour defective oranges are accidently mixed with sixteen good ones and by looking at them it is not possible to differentiate between them. Three oranges are drawn at random from the lot. Find the probability distribution of X, the number of defective oranges.
View SolutionThe random variable `X` can the values 0, 1, 2, 3, Give P(X = 0) = P(X = 1)= p and P(X = 2) = P(X = 3) such that `sum p_i x_i^2=2sum p_i x_i` then find the value of `p`
View SolutionLet a pair of dice be thrown and the random variable X be the sum of the numbers that appear on the two dice. Find the mean or expectation of X.
View SolutionFind the mean and variance of the numbers obtained on a throw of an unbiased die.
View SolutionFind the mean and variance of the number of heads in the two tosses of a coin.
View SolutionTwo cards are drawn (without replacement) from a well shuffled deck of 52 cards. Find the probability of both being king of red colour.
View SolutionTwo numbers are selected at random(without replacement) from the first five positive integers. Let X denote the larger of the two numbers obtained. Find the mean and variance of X
View SolutionTwo cards are drawn simultaneously (without replacement) from a well-shuffled pack of 52 cards. Find the mean and variance of the number of red cards.
View SolutionThere are 4 cards numbered 1, 3, 5 and 7, one number on one card. Two cards are drawn at random without replacement. Let 'X' denote the sum of the numbers on the two drawn cards. Find the mean and variance of X.
View SolutionFrom a lot of 10 items containing 3 defective items a sample of 4 items is drawn at random. Let the random variable 'X' denote the number of defective items in the sample. If the sample is drawn without replacement, find :
(i) The Probability Distribution of X
(ii) Mean of X (iii) Variance of X.
View Solutionthree numbers are selected at random (without replacement) from first six positive integers. Let X denote the largest of the three numbers obtained. the probability distribution of X. Also, find the mean
View SolutionSix balls are drawn successively from an um containing 7 red and 9 black balls. Tell whether or not the trials of drawing balls are Bernoulli trials when after each draw the ball drawn is (i) replaced (ii) not replaced in the urn.
View SolutionAn experiment succeeds thrice as often as it fails. Find the probability that in the next five trials, there will be atleast 3 successes.
View SolutionAn unbiased coin is tossed 6 times .Find using binomial distribution , the probability of getting at least 5 heads .
View SolutionA pair of dice is thrown 4 times. If getting a doublet is considered as a success,
(i) find the probability of getting a doublet
(ii) hence, find the probability of two succeses.
View SolutionThe probability that a student entering university will graduate is 0.4. Find the probability that out of 3 students of the university: (i) none will graduate, (ii) only one will graduate, (iii) all will graduate.
View SolutionTen eggs are drawn successively with replacement from a lot containing 10% defective eggs. Find the probability that there is at least one defective egg.
View SolutionThe probability of a shooter hitting a target is `3/4`. How many minimum number of times must he/she fire so that the probability of hitting the target at least once is more than 0.99?
View SolutionFive dice are thrown 729 times. How many times do you expect that at least four dice to show five or six ?
View SolutionIn a backward state, there are 729 families having six children each. If probability of survival of a girl is `(1)/(3)` and that of a boy is `(2)/(3)`, find the number of families having 2 girls and 4 boys.
View SolutionA die is thrown again and again until three sixes are obtained. Find the probability of obtaining the third six in the sixth thrown of the die.
View SolutionIn an examination, 10 questions of true-false type are asked. A student tosses a fair coin to determinehis answer to each question. If the coin falls heads, he answers "true" and if it falls Lails, he answers "false "Show that the probability that he answers at most correctly 7 questions is `121/128`.
View SolutionFind the mean of the Binomial distribution `B(4,1/3)`.
View SolutionThe sum of mean and variance of a binomial distribution is 15 and the sum of their squares is 117. Determine the distribution.
View SolutionThe mean and variance of a binomial distribution are 4 and 4/3 respectively, find `P(Xgeq1)dot`
View SolutionA and B are two candidates seeking admission in a college. The probability that A is selected is 0.7 and the probability that exactly one of them is selected is 0.6. Find the probability that B is selected.
View SolutionThe probability of simultaneous occurrence of at least one of two events A and B is p. If the probability that exactly one of A, B occurs is q then prove that `P( A )+P( B )=2-2p+qdot`
View Solution10% of the bulbs produced in a factory are red colour and 2% arered and defective. If one bulb is picked at random, determine the probability of its being defective if it red.
View SolutionThree machinesE1, E2 and E3 in a certain factory producing electric bulbs, produce 50%, 25% and 25% respectively, of the total daily output of electric bulbs. It is known that 4% of the bulbs produced by each of machines E1 and E2 are defective and that 5% of those produced by machine E3are defective. If one bulb is picked up at random from a days production, calculate the probability that it is defective.
View SolutionDetermine variance and standard deviation of the number of heads in three tosses of a coin.
View Solution
EXAMPLES questions and complete solutions for chapter PROBABILITY of ARIHANT MATHS (HINGLISH) of Class 12
All Exercises from MODERN PUBLICATION Class 12 MATHS PROBABILITY
- CHAPTER TEST
- CHECK YOUR UNDERSTANDING
- COMPETITION FILE
- EXAMPLES
- Exercise
- EXERCISE 13 (a) (LATQ)
- EXERCISE 13 (a) (SATQ)
- EXERCISE 13 (b) (LATQ)
- EXERCISE 13 (b) (SATQ)
- EXERCISE 13 (c) (SATQ)
- EXERCISE 13 (d) (LATQ)
- EXERCISE 13 (e) (LATQ)
- EXERCISE 13 (e) (SATQ)
- EXERCISE 13 (f) (LATQ I)
- EXERCISE 13 (f) (LATQ II)
- EXERCISE 13 (f) (SATQ)
- EXERCISE 13 (g) (LATQ)
- EXERCISE 13 (g) (SATQ)
- EXERCISE 13 (h) (LATQ)
- Miscellaneous Exercise on Chapter 13
- MOCK TEST (Answer the following questions)
- MOCK TEST (Fill in the blanks)
- MOCK TEST (Select the correct option)
- MOCK TEST SECTION D
- NCERT-FILE (Exercise 13.1)
- NCERT-FILE (Exercise 13.2)
- NCERT-FILE (Exercise 13.3)
- NCERT-FILE (Exercise 13.4)
- NCERT-FILE (Exercise 13.5)
- Objective Type Question (A. Multiple Choice Questions)
- Objective Type Question (B. Fill in the Blanks)
- Objective Type Question (C. True/False Questions)
- Objective Type Question (D. Very Short Answer Tyep Questions)
- Revision Exercise