Complete Solutions to Practice Exercise 5.1 of chapter ELECTROMAGNETIC INDUCTION AND ALTERNATING CURRENT of Class 12 book with complete answers and questions
| Class 12 | PHYSICS |
| Chapter | ELECTROMAGNETIC INDUCTION AND ALTERNATING CURRENT |
| PHYSICS GALAXY | Practice Exercise 5.1 |
| Number Of Questions | 12 |
| Academic Year | 2023-24 |
A coil of area `500cm^(2)` and having `1000` turns is held perpendicular to a uniform field of `0.4` gauss. The coil is turned through `180^(@)` in `1//10sec` . Calculate the average induced e.m.f.
View SolutionTwo long parallel conducting horizontal rails are connected by a conducting wire at one end. A uniform magnetic field B (directed vertically downwards) exists in the region of space.

A light uniform ring of diameter d which is practically equal to separation between the rails is placed over the rails as shown in Fig. If resistance of ring be `(lambda)` per unit length
The force required to pull the ring with uniform velocity v is
View SolutionA very small circular loop of area `5xx10^(-4)m^(2)` , resistance `2Omega` and negligible inductance is initially coplanar and concentric with a much larger fixed circular loop of radius `0.1m` . A constant current of `1A` is passed in the bigger loop and the smaller loop is rotated with angular velocity `omega rad//sec` about a diameter. Calculate (a) the flux linked with the smaller loop, (b) induced emf (c) induced current in the smaller loop, as a function of time.
View SolutionA flat circular coil having `N` turns (tightly wound `D`) is placed in a time varying magnetic field `B = B_(0) sin omega t`. The outer radius of the coil is `R`. Determine the maximum value of the induced emf in the circuit.
View SolutionTwo long parallel horizontal rails a, a distance d aprt and each having a risistance `lambda` per unit length are joing at one end by a resistance R. A perfectly conduction rod MN of mass m is free to slide along the rails without friction (see figure). There is a uniform magnetic field of induction B normal to the plane of the paper and directed into the paper. A variable force F is applied to the rod MN such that, as the rod moves a constant current flows through R.
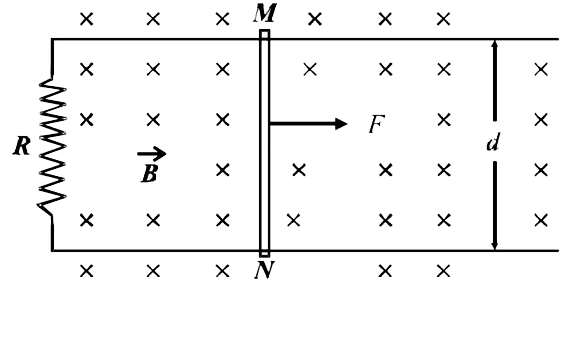
(i) Find the velocity of the rod and the applied force F as function of the distance x of the rod from R.
(ii) What fraction of the work done per second by F is converted into heat?
View SolutionA wire frame of area `3.92xx10^(-4)m^(2)` and resistance `20Omega` is suspended freely from a 0.392m long thread. There is a uniform magnetic field of 0.784 T and the plane of wire-frame is made to oscillate under gravity by displacing it through `2xx10^(-2)m` from its initial position along the direction of magnetic field. The plane of the frame is always along the direction of thread and does not rotate about it . What is the induced EMF in wire-frame us a function of time ? Also find the maximum current in the frame.
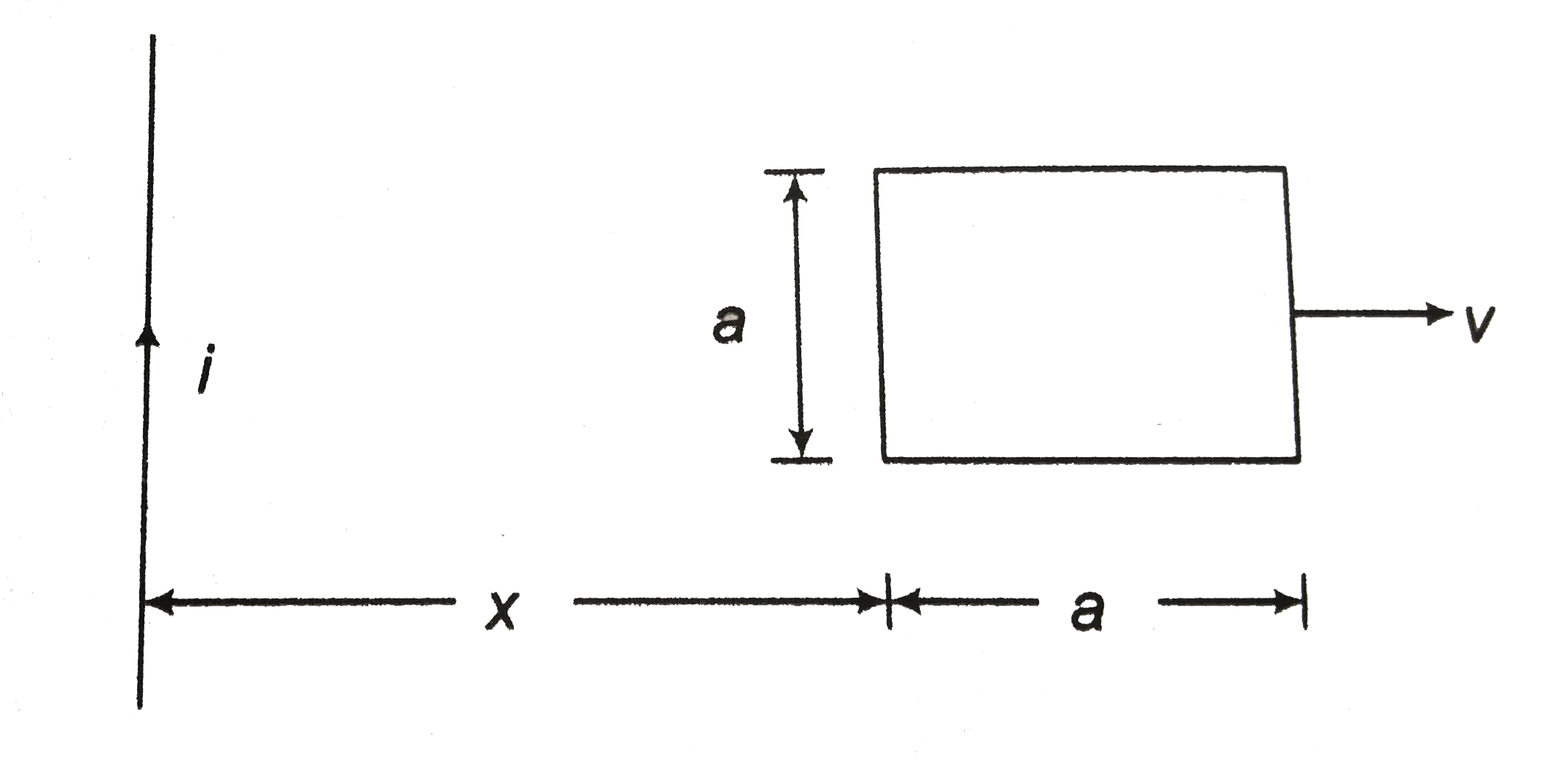
View SolutionA square frame with side a and a long straight wire carrying a current `i` are located in the same plane as m shown in figure. The fram translates to the right with a constant velocity `v`. Find the emf induced in the frame as a function of distance `x`.
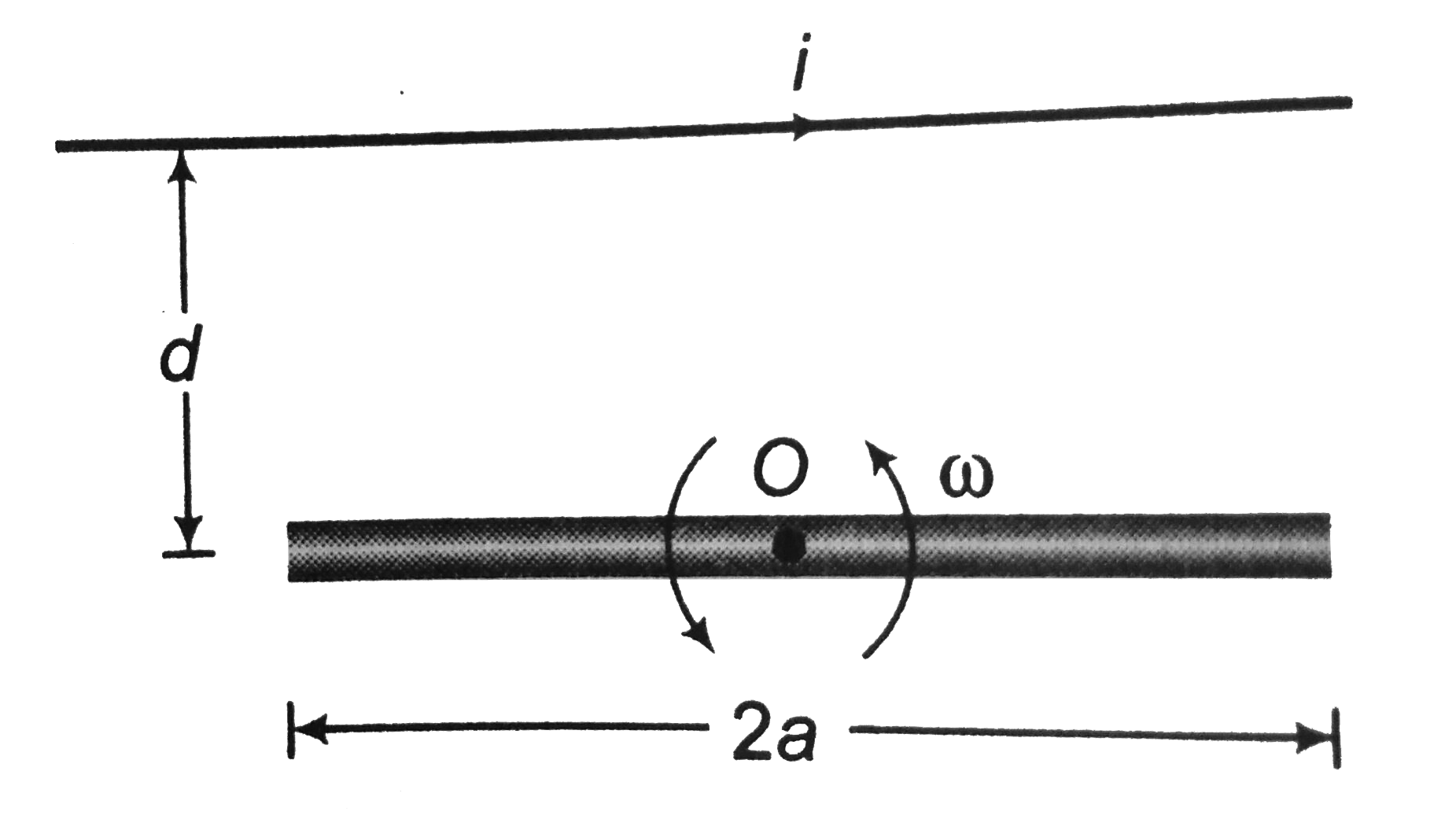
View SolutionA rod of length `2a` is free to rotate in a vertical plane, about a horizontal axis `O` passing through its mid-point. A long straight, horizontal wire is in the same plane and is carrying a constant current i as shown in figure. At initial moment of time, the rod is horizontal and starts to rotate with constant angular velocity `omega`, calculate emf induced in the rod as a function of time.
View SolutionTwo long wires are placed on a pair of parallel rails perpendicular to the wires. The spacing between the rails d is large compared with x, the distance between the wires. Both wires and rails are made of a material of resistivity `rho` per unit length. A magnetic flux density B is applied perpendicular to the rectangle by the wires and rails. One wire is moved along the rails with a uniform speed v while the other is held stationary. Determine how the force on the stationary wire varies with x and show that it vanishes for a value of x approximately equal to
View SolutionA uniform rod AB of mass m and length l is placed over two smooth conducting rails P and Q. If the witch shown as closed at t=0, find the velocity of rod AB as a function of time.
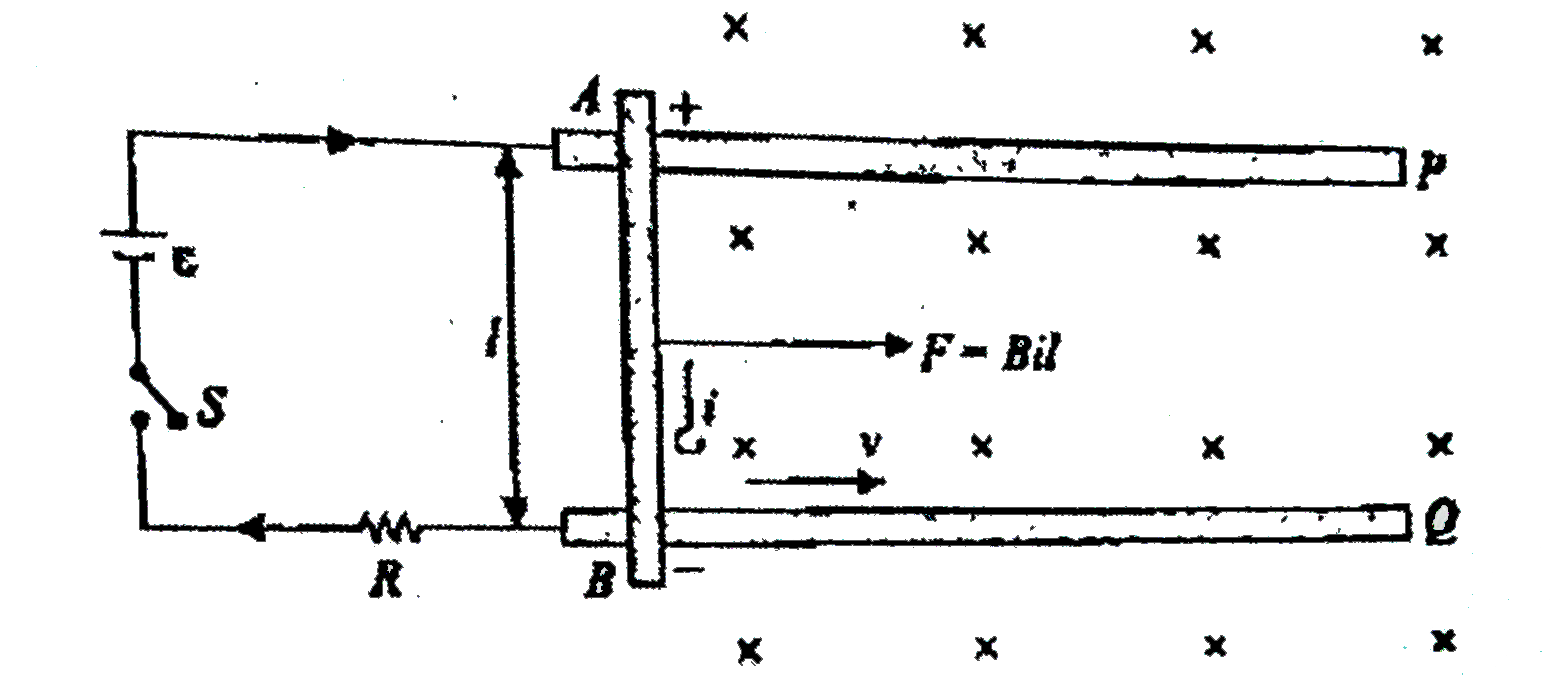
View SolutionIn figure-5.54 a wire ring of radius R is in pure rolling on a surface. Find the EMF induced across the top and bottom points of the ring at any instant.
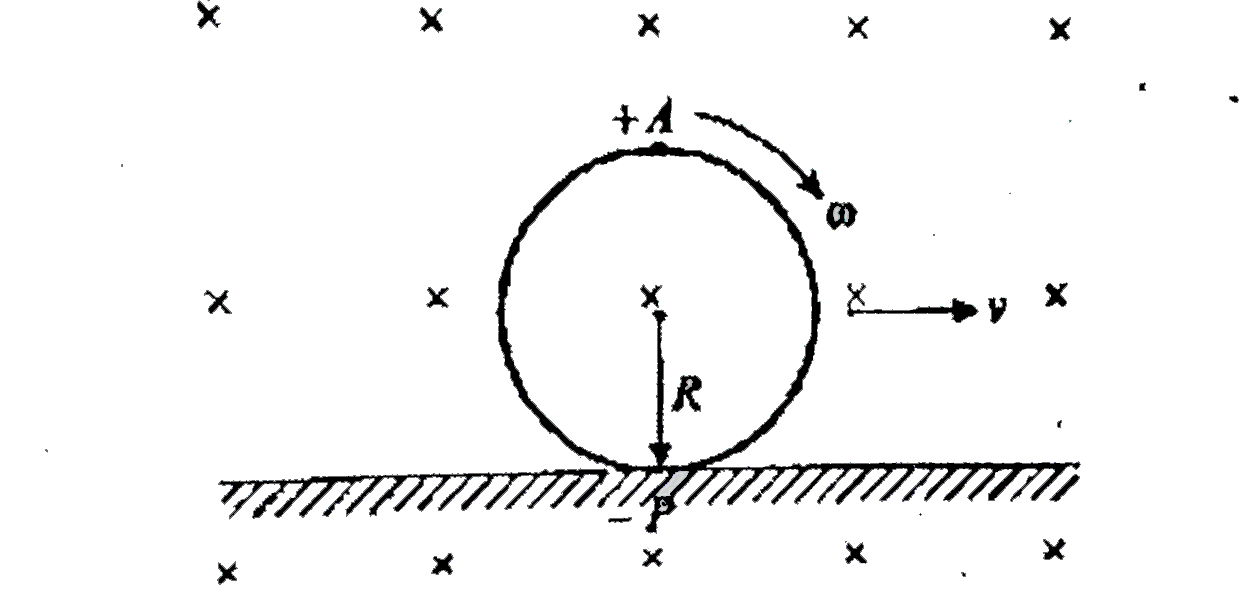
View SolutionFigure-5.55 shows a small circular coil of area A suspended from a point O by a string of length l in a uniform magnetic induction B in a uniform magnetic induction B in horizontal direction. If the coil is set into oscillation like a simple pendulum by displacing it a small angle `0_(0)` as shown, find EMF induced in coil as a function of time. Assume the plane of coil is always in plane of string.
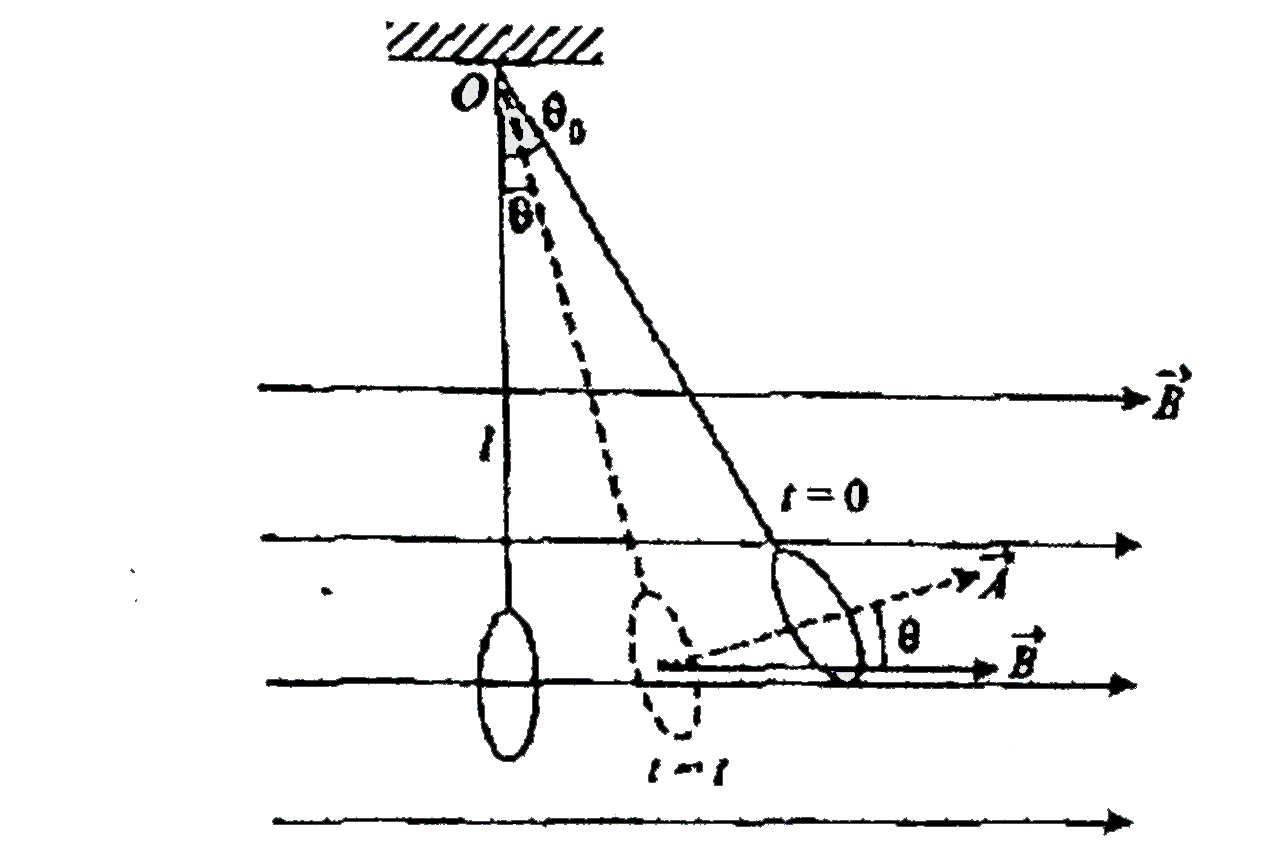
View Solution
Practice Exercise 5.1 questions and complete solutions for chapter ELECTROMAGNETIC INDUCTION AND ALTERNATING CURRENT of GK PUBLICATIONS PHYSICS (HINGLISH) of Class 12