Complete Solutions to Unsolved Numerical Problems of chapter WAVE OPTICS of Class 12 book with complete answers and questions
| Class 12 | PHYSICS |
| Chapter | WAVE OPTICS |
| PHYSICS GALAXY | Unsolved Numerical Problems |
| Number Of Questions | 28 |
| Academic Year | 2023-24 |
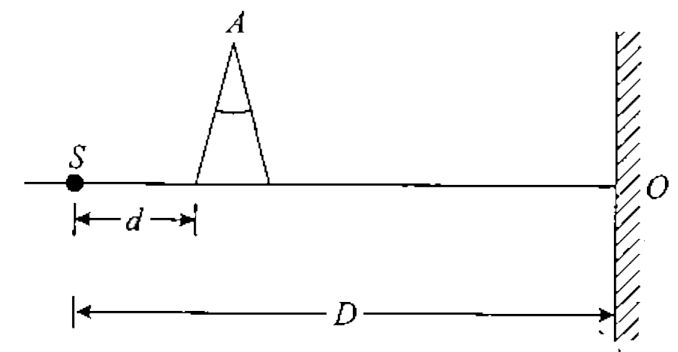
A prism having angle `A^(@)` (which is very small) is placed in front of a point source S at a small distance d. A screen is placed at a large distance D as shown in the figure-6.113. Find the fringe width of interference pattern given that source is emitting light of wavelength `lambda` and refractive index of prism is `mu`.
View SolutionA thin glass plate of thickness t and refractive index `mu` is inserted between screen and one of the slits in a young's experiment. If the intensity at the centre of the screen is I, what was the intensity at the same point prior to the introduction of the sheet.
View SolutionA double slit arrangement produces fringes for light `lambda=5890Å` which are `0.2^@` apart. If the whole arrangement is fully dipped in a liquid of `mu=4/3`, the angular fringes separation is
View SolutionTwo coherent narrow slits emitting light of wavelength `lambda` in the same phase are placed parallel to each other at a small separation of `2lambda`. The light is collected on a screen S which is placed at a distance D(`gtgt lambda`) from the slit `S_1` as shown in figure. Find the finite distance x such that the intensity at P is equal to intensity O.
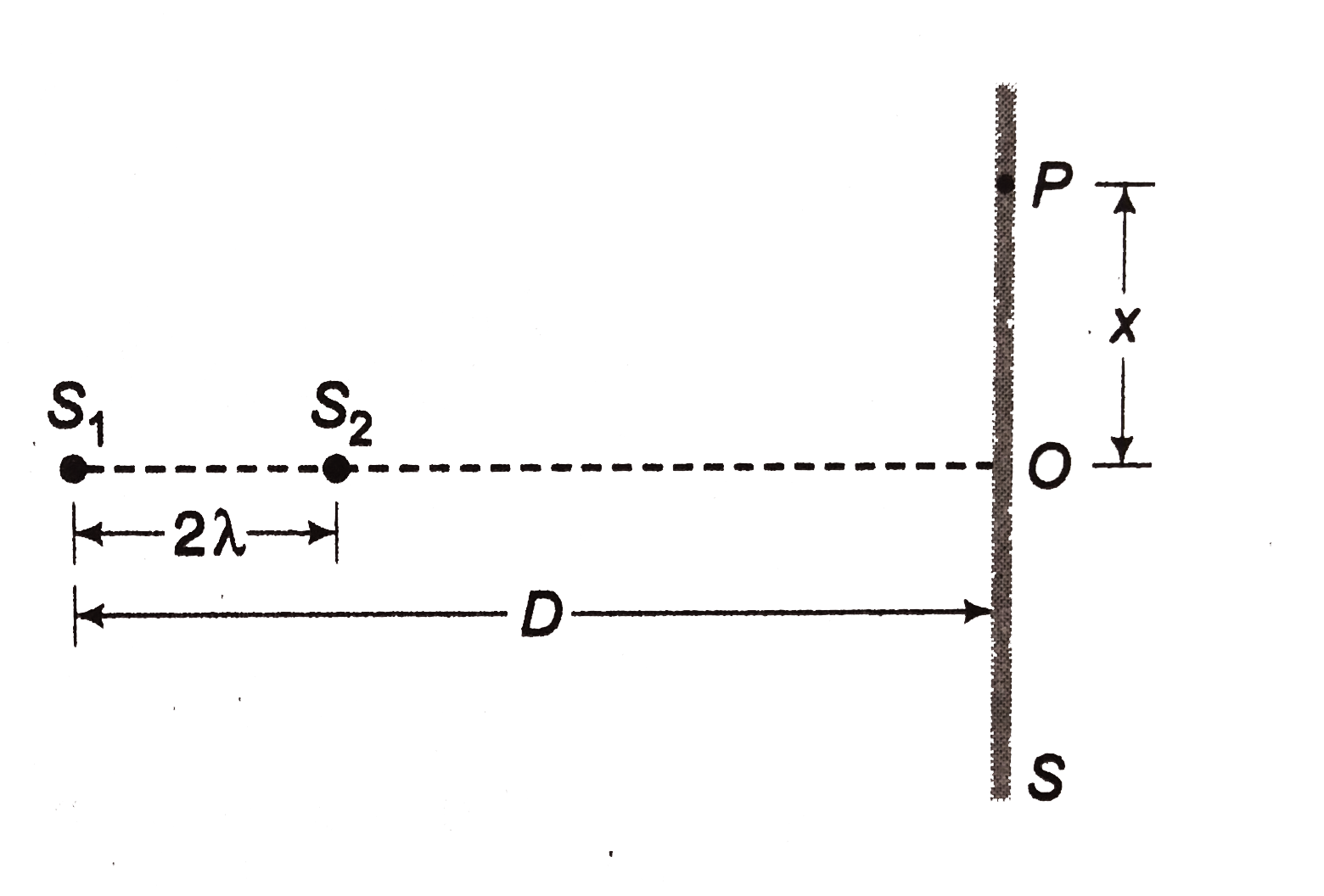 .
.
View SolutionIn Young's double-slit experiment, the slit are 0.5 mm apart and the interference is observed on a screen at a distance of 100 cm from the slits, It is found that the ninth bright fringe is at a distance of 7.5 mm from the second dark fringe from the center of the fringe pattern. The wavelength of the light used is
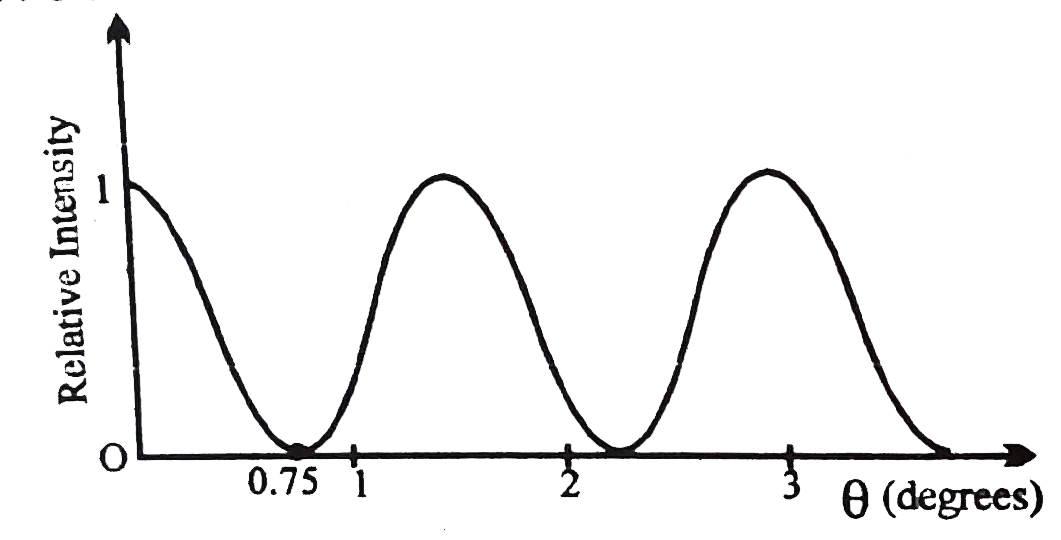
View SolutionLight of wavelength `520nm` passing through a double slit,produced interference pattern of relative intensity versus deflection angle `theta` as shown in the figure.find the separation of between the slits.
View SolutionThe distance between two slits in a YDSE apparatus is 3mm. The distance of the screen from the slits is 1m. Microwaves of wavelength 1 mm are incident on the plane of the slits normally. Find the distance of the first maxima on the screen from the central maxima.
View SolutionOne radio transmitter A operating `60.0MHz`is `10.0`from another similar transmitter `B` that is `180^(@)` out of phase with transmitter`A`.How far must be observer move from transmitter A towards transmitter B along the line connencting A andB to reach the nearest point when the two beam are in phase?
View SolutionThe central fringe of the interference pattern produced by the light of wavelength 6000 Å is found to shift to the position of 4th dark fringe after a glass sheet of refractive index 1.5 is introduced. The thickness of glass sheet would be
View SolutionA broad sources of light of wavelength `680nm` illuminated normally two glass plates.`120nm`long that meet at one end and are separated by a wire`0.048 mm`in diameter at the other end,Find the number of bright fringes formed over the `120nm`distance.
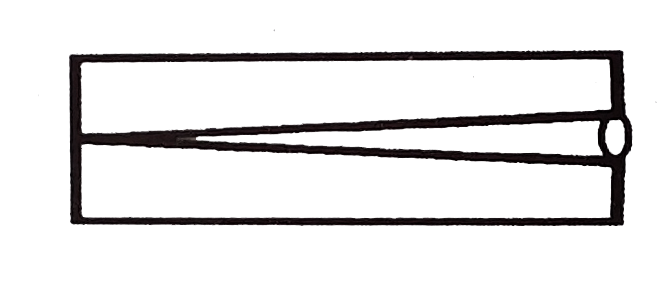
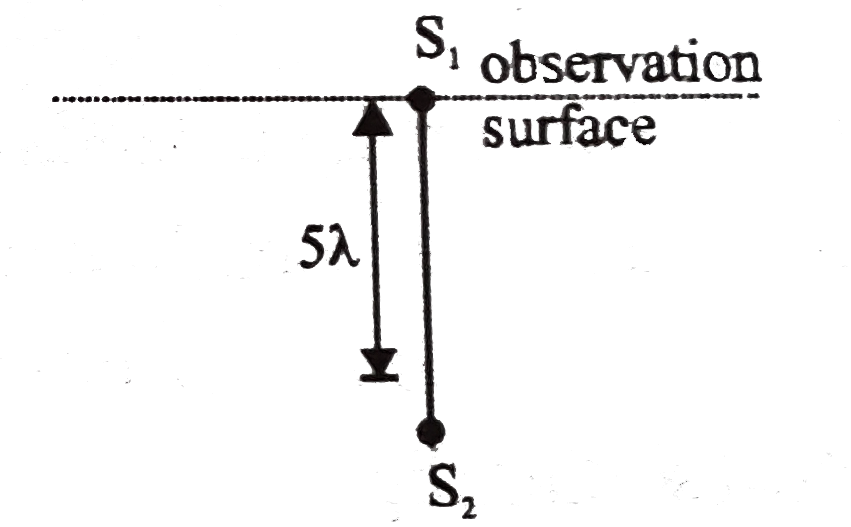
View SolutionTwo microwave coherent point sources emitting waves of wavelenths `lambda`are placed at `5lambda`distance apart.The interference is being observed on a flat non-reflecting surface along a line passing through on sources ,in a direction perpendicular to the line joining the two sources(refer figure)
Considering `lambda`as`4mn` ,calculate the positions of maxima and draw shape of interference pattern,takek initial phase differnce between the two sources to be zero.
View SolutionIn a YDSE two thin transparent sheet are used in front of the slits `S_(1)`&`S_(2)` having `mu_(1)=1.6`and `mu_(2)=1.4` respectively.If both sheets have thickness`t` ,the central maximum is observed at a distance of `5mm`from center`O`.Now the sheets are replaced by another two sheets each of refractive index `(mu_(1)-mu_(2))/(2)` but having thickness `t_(1)&t_(2)=(t_(1)-t_(2))/(2)`.Now central maximum is observed at distance of `8mm` from center`O` on the same side as before.Find the thickness `t_(1)` (in`mu`m) [Given:d=1mm,D=1m]
View SolutionIn a two slit experiment with monochromatic light fringes are obtained on a screen placed at some distance from the sits. If the screen is moved by m `5 xx 10^(-2)m` towards the slits, the change in fringe width is m `3 xx 10^(-5)m` . If separation between the slits is `10^(-3)m` , the wavelength of light used is
View SolutionA source S is kept directly behind the slit `S_(1)` in a double slit apparatus. What will be the phase difference at P, if a liquid of refraction index `mu`is filled : (wavelength of light in aire is `lambda` due to the source, assume `lgtgtd,Dgtgtd`).
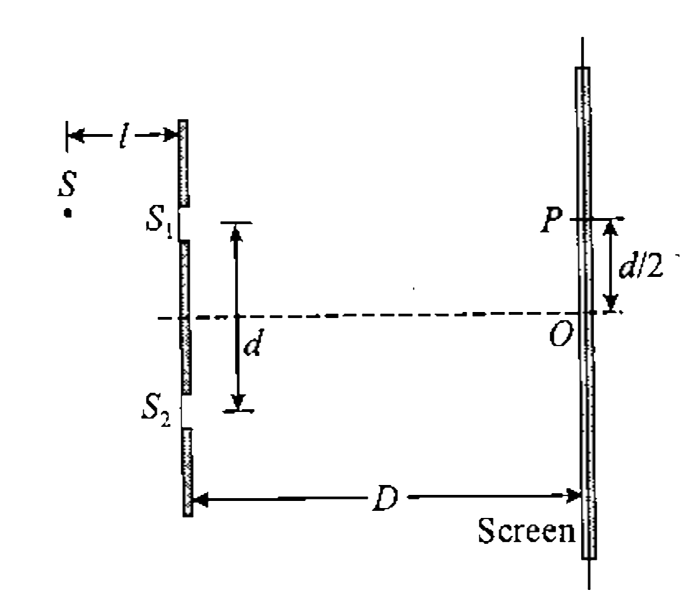
(a) Between the screen and the slits.
(b) Between the slits & the source S. In this case, find the minimum distance between the points on the screen where the intensity is half the maximum intensity on the screen.
View Solution_E01_102_Q01.png)
In a YDSE a parallel beam of light of wavelength 6000Å is incident on slits at angle of incidence `30^(@)`. A and B are two thin transparent films each of refractive index 1.5. thickness of A is `20.4mum`. Light coming through A and B have intensities I and 4I resepctively on the screen. Intensity at point O which is symmetric relative to the slits is 3I. The central maxima is above O.
(i). what is the maximum thickness of B to do so. Assuming thickness of B to be that found in part (i) answer the following parts.
(ii). Find fringe width, maximum intensity and minimum intensity on screen.
(iii). Dinstance of nearest minima from O
(iv) intensity at 5 cm on either side of O.
View SolutionA plastic film with index of refraction 1.80 is put on the surface of a car window to increase the reflectivity and thereby to keep the interior of the car cooler. The widow glass gas index of refraction 1.60.
What minimum thickness is required, if light of wavelength 600 nm in air reflected from the two sides of the film is to interfere constructively?
(b) It is found to be difficult to manufacture and install coatings as thin as calculated in part (a). What is the next greatest thickness for which there will also be constructive interference?
View SolutionTwo coherent monochromatic sources A and B emit light of wavelength `lambda`. The distance beween A and B is `d = 4lambda`.
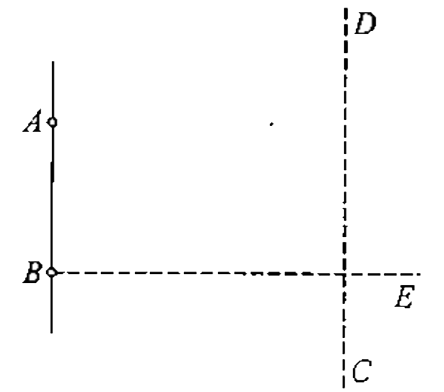
(a) If a light detector is moved along a line CD parallel to AB, what is the maximum number of minima observed ?
(b) If the detector is moved along a line BE perpendicular to AB and passing through B, what is the number of maxima observed ?
View SolutionA beam of light consisting of two wavelength, `6500 Å` and `5200 Å` is used to obtain interference fringes in a Young's double slit experiment. Find the distance of the third fringe on the screen from the central maximum for the wavelength `6500Å`. What is the least distance from the central maximum at whixh the bright fringes due to both wavelengths coincide? The distance between the slits is 2 mm and the distance between the plane of the slits and the screen is 120 cm.
View SolutionIn an experiment using Fresnel biprism, fringes of width `0.185 xx 10^(-3)m` are observed at a distance of 1 m from the slit. Images are of the coherent sources are then produced at the same distane from the slit by placing a convex lens at a distance of 0.3 m form the slit. The images are found to be separated by `0.7 xx 10^(-2)m`. Calculate the wavelength of the light used.
View SolutionTwo identical monochromaticlight sources A & B intensity `10^(-15)W//m^(2)` produce wavelength of light `4000sqrt(3)Å` A glass of thickness 3mm is placed in the path of the ray as shown in figure the glass has a varible refractive index `n=1+sqrt(x)` where x (in mm) is distance of plate from left to right calculate total intensity at focal points F of the lens.
_E01_100_Q01.png)
View SolutionTwo fine slits are placed very close to each other and they are illuminated by a strong beam of light of wavelength `5900Å`. Fringes are obtained at a distance of 0.3 m from the slits. The fringe width is found to be `5.9 xx 10^(-5)m`. Calculate the distance between the slits.
View SolutionBiprism fringes are produced with the help of sodium light `(lambda=5893Å)`. The angle of the biprism is 179.5^(@)` and tis refractive index 1.5. If the distance between the slit and the biprism is 0.4m and the distance between the biprism and the screen is 0.6 m, find the distance between successive bright fringes. Also calculate the maximum width of the slit for which the fringes will still be sharp.
View SolutionA glass plate `1.2 xx 10^(-6)m` thick is placed in the path of one of the interfering beams in a biprism arrangement using monochromatic light of wavelength `6000Å. If the central band shifts by a distance equal to the width of the bands, find the refractive index of glass.
View SolutionA narrow monochromatic beam of light of intensity 1 is incident on a glass plate as shown in figure Another identical glass plate is kept close to the first one and parallel to it. Each glass plate reflects `25%` of the light incident on it and transmits intensities in the interference pattern formed by two beams obtained after one reflection at each plate.
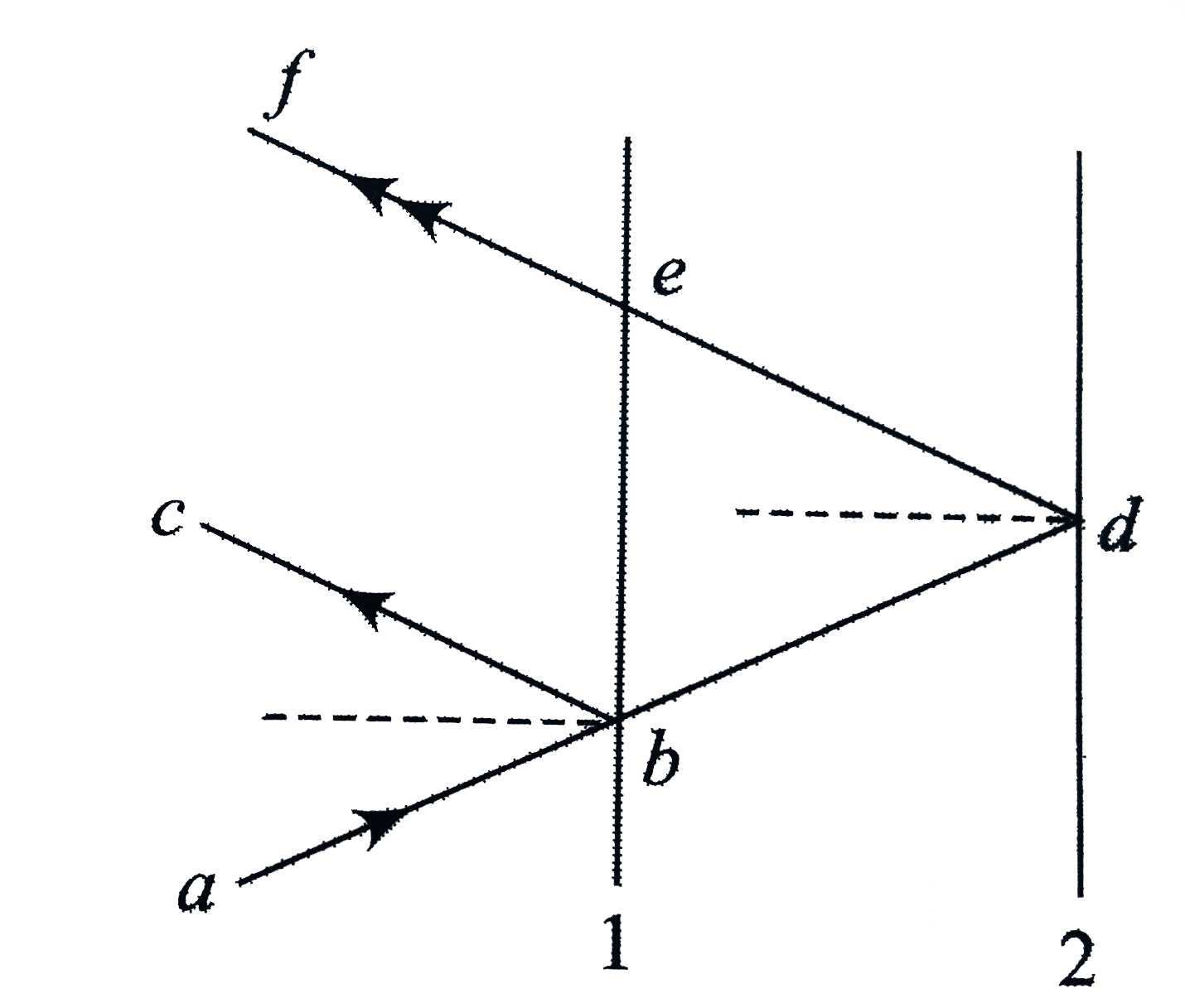
View SolutionA plane light wave of wavelength `lambda = 700nm` falls normally on the base of a biprism made of glass `(mu = 1.560)` width refracting angle `theta = 5^(@)`. A plate is placed behind the biprism and the space between them is filled with a liquid of refractive index `(mu' = 1.500)`. Find the fringe width on a screen behind the biprism.

View SolutionIn a biprism experiment, the angular width of fringes was `theta=2`'. Calculate the separation between the slits if the wavelength of the incident light was `lambda = 700 nm`.
View SolutionIn a biprism experiment, calculate the intensity of lights on the screen at a point `5beta//6` away from the central fringe in terms of the intensity of the central fringe, which is `I_(0)`. Here `beta` is the fringe width.
View SolutionTwo parallel beams of light P and Q are incident normally on a prism and the transmitted rays are brought to focus with the help of a convergent lens as shown in Fig. 2.23. If the intensities of the upper and lower beams immediately after transmission from face AC are 4I and I, respectively, find the resultant intensity at the focus.
View Solution
Unsolved Numerical Problems questions and complete solutions for chapter WAVE OPTICS of GK PUBLICATIONS PHYSICS (HINGLISH) of Class 12