Complete Solutions to EXAMPLES of chapter CHEMICAL KINETICS of Class 12 book with complete answers and questions
| Class 12 | CHEMISTRY |
| Chapter | CHEMICAL KINETICS |
| RC MUKHERJEE | EXAMPLES |
| Number Of Questions | 49 |
| Academic Year | 2023-24 |
v49_newFlow
02:10View SolutionRadioactive decay is a first`-` order process. Radioactive carbon in wood sample decays with a half`-` life of 5770 years. What is the rate constant `(` in years `)` for the decay ? What fraction would remains after 11540 years ?
02:42View SolutionThe first order reaction has `k=1.5xx10^(-6)` per second at `200^(@)C` . If the reaction is allowed to ruf for 10 hours at the same temperature, what percentage of the initial concentration would have changed into the product? What is the half life period of this reaction ?
04:50View SolutionFor a reaction , the concentration of the reactant C varies with time t as shown by the following graph .
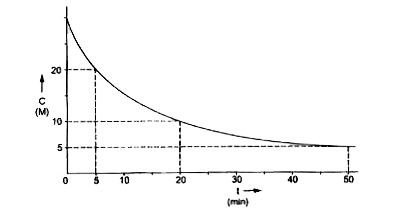
what do you conclude about the order of the reaction ?View SolutionFor a reaction , a graph was plotted between reactant concentration `c` and time as shown in the figure below.
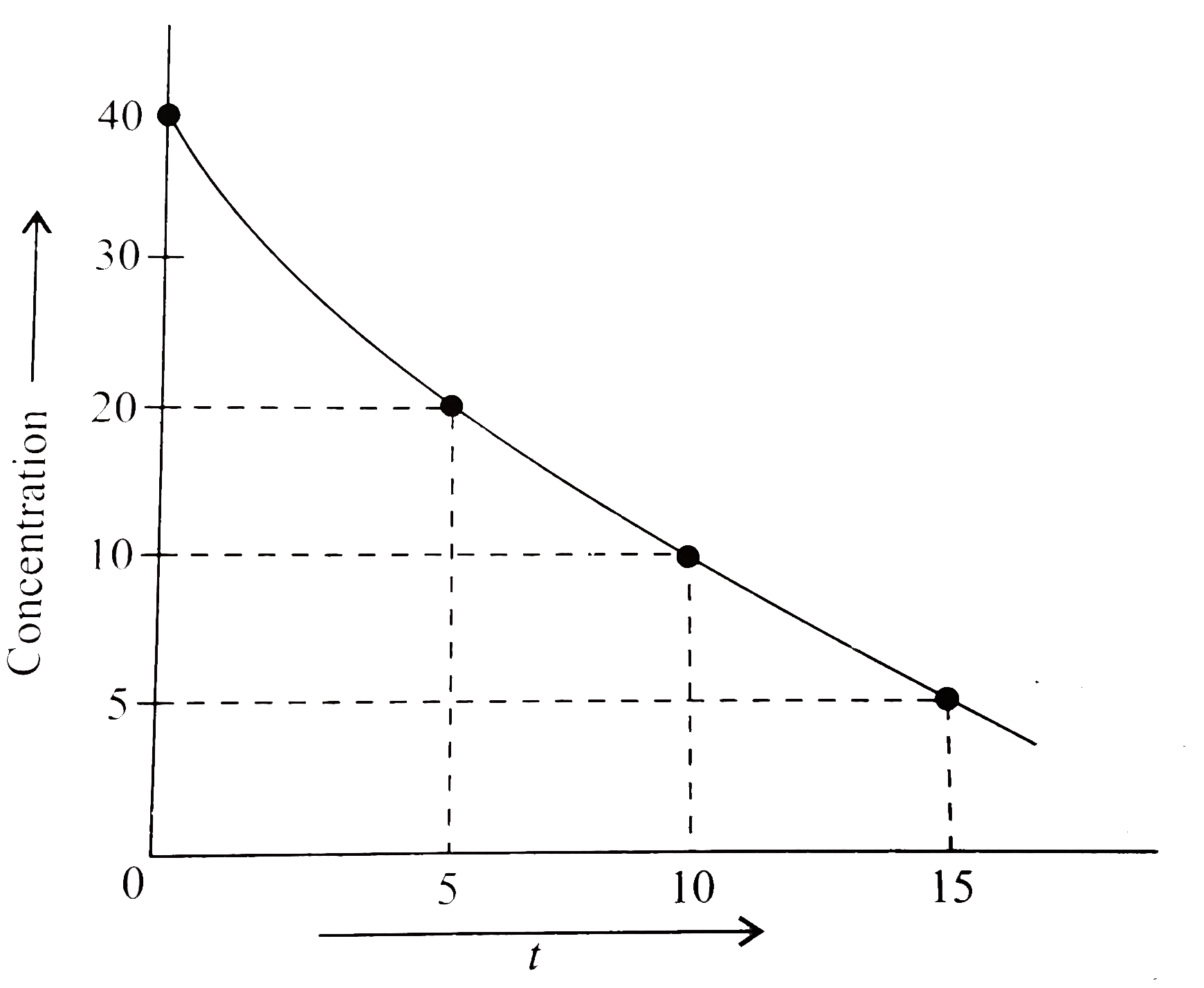
Identify the order of the reaction with respect to the reactant. Can the concentration of the reactant be theoretically zero after infinite time?03:36View SolutionIn a reaction `2N_(2)O_(5) to 4NO_(2)+O_(2)` the rate can be expressed as
(i) `-(d[N_(2)O_(5)])/(dt) =k_(1)[N_(2)O_(5)]`
(ii) `(d[NO_(2)])/(dt) =k-(2)[N_(2)O_(5)]`
(iii) `(d[O_(2)])/(dt) =k_(3)[N_(2)O_(5)]`
How are `k_(1),k_(2) and k_(3) `related ?02:26View SolutionThe reaction `2N_(2)O_(5) to NO_(2)+O_(2)` is forming `NO_(2)` at the rate of `0.0072` mole /L/s at some time .
What is the rate of change of `[O_(2)]` att this time ?01:44View SolutionThe reaction `2N_(2)O_(5) to NO_(2)+O_(2)` is forming `NO_(2)` at the rate of `0.0072` mole /L/s at some time .
What is the rate of change of `[N_(2)O_(5)]` at this time?01:52View SolutionThe reaction `2N_(2)O_(5) to NO_(2)+O_(2)` is forming `NO_(2)` at the rate of `0.0072` mole /L/s at some time .
What is the rate of reaction at this time ?03:47View SolutionAt some temperature the rate constant for the decomposition of HI on a gold surface is `0.08 M s^(-1)`
`HI(g) to H_(2)(g)+I_(2)(g)`
What is the order of the reaction ? How long will it take for the concentration of HI to drop from `1.50 M `to `0.30` M ?03:07View SolutionThe reaction `2N_(2)O_(5)(g) to 2N_(2)O_(4)(g)+O_(2)(g)` obeys the rate law , rate =`k[N_(2)O_(5)]` where `k=0.0084 s^(-1)`
If `2.50` moles of `N_(2)O_(5)` were taken in a `5.0` -litre flask , how many moles of `N_(2)O_(4)` would remain after 60 seconds ?05:23View SolutionThe rate of first order reaction is `0.04 mol L^(-1) s^(-1)` at `10 min` and `0.03 mol L^(-1) s^(-1)` at `20 min` after initiation. Find the half life of the reaction.
04:31View SolutionThe rate law of a chemical reaction
`2NO(g)+O_(2)(g)=2NO_(2(g)`
is given as rate `= k[NO]^(2) [O_(2)]` .How will the rate of the reaction change if the volume of the reaction vessel is reduced to one - fourth of its initial volume ?03:18View SolutionIn a reaction `H_(2)+I_(2) to 2HI` the rate of disappearace of `I_(2)` is found to be `10^(-6)` mole per litre per second . What would be the corresponding rate of appearance of HI?
02:07View SolutionShow that in case of a first order reaction, the time required for `99.9%` of the reaction to take place is about `10` times that the required for half the reaction.
03:22View SolutionFor a reaction at `800^(@)C`
`2NO + 2H_(2) rarr N_(2) + 2H_(2)O`, the following data were obtained:
`|{:(,[NO] xx 10^(-4) (M),[H_(2)] xx 10^(-3)(M),-(1)/(2) (d[NO])/(dt) xx 10^(-4) (mol L^(-1) min^(-1))),(i.,1.5,4.0," " 4.4),(ii.,1.5,2.0," "2.2),(iii.,0.5,2.0," "0.24):}|`
What is the order of this reaction with respect to `NO` and `H_(2)` ? Also calculate the rate constant.05:20View SolutionWhat will be the order of reaction of the type `A to ` Product for which the following data were obtained ?
 02:50View Solution
02:50View SolutionCalculate the order of the reaction `A to ` product from the following data :
 .04:20View Solution
.04:20View SolutionFor a chemical reaction `A+B to ` Product , the order is 1 with respect to each of A and B . Find x and y from the given data .
 03:01View Solution
03:01View SolutionThe following data were obtained at a certain temperature for the decomposition of ammonia in contact with tungsten:
p (m m Hg) 50 100 200
Relative `t_(1//2)` 3.64 1.82 0.91
Find the order of the reaction.04:19View SolutionIn the decomposition of `H_(2)O_(2)` at 300 K , the energy of activation was found to be 18 kcal/mole , while it decreased to 6 kcal/mole when the decomposition was carried out in the presence of a catalyst at 300 K . How many times is the catalysed reaction faster than the uncatalysed one ?
View SolutionThe energy of activation and specific rate constant for a first - order reaction at `25^(@)` C .
`2N_(2)O_(5) to 2N_(2)O_(4) +O_(2)`
`( " in "C Cl_(4)) " " ( " in " C Cl_(4))`
are 100 kJ /mole and `3.46 xx10^(-5) s^(-1)` respectively . Determine the temperature at which the half - life of the reaction is 2 hours .View SolutionIn Arrhenius equation for a certain reaction, the value of A and E_a (activation energy) are 4 x `10^(13) s^(-1)` and 98.6 kJ `mol^(-1)` respectively If the reaction is of first order, then at what temperature will its half life period be 10 minutes? [log 2 88 = 0.4594]
03:24View SolutionTwo reactions proceed at `25^(@)C` at the same rate, the temperature coefficient of the rate of the first reaction is 2 and that of the second reactions 2.5. Find the ratio of rates of these reaction at `95^(@)C`
04:30View SolutionWhat is the energy of activation of a reaction is its rate doubles when the temperature is raised from 290 K to 300 K ?
01:40View SolutionThe activation energy for the reaction ,
`O_(3) (g) + NO(g) to NO_(2)(g) +O_(2)(g)`
is `9.6` kJ/mole .Prepare an activation energy plot if `DeltaH^(@)` for this reaction is 200 kJ/mole . What s the energy of activation for the reverse reaction ?View SolutionThe decomposition of `H_(2)O_(2)` in aqueous solution is a reaction of first order . How can it be justified from the following data :
where x is the volume (mL) of `KMnO_(4)` require to titrate a definite colume of `H_(2)O_(2)` ?View SolutionFrom the following data for the decomposition of diazobenzene chloride , show that the reaction is of first order :
`{:("Time",20,50,70,oo),((min),,,,),("Vol of"N_(2),10,25,33,162),((mL),,,,):}` .View Solution1 mL of methyl acetate was added to 20 mL of `0.5` N HCl and 2 mL of the mixture was withdrawn from time to time during the process of hydorlysis of the ester and titrated with a solution of alkali . The amount of alkali needed for titration at various intervious intervals is given below :
`{:("Time",0,20,119,oo),((min),,,,),("Alkali used",19.24,20.73,26.6 ,42.03),((mL),,,,):}`
Establish that the reaction is of first order .View SolutionThe following data were obtained for the saponification of ethyl acetate using equal concentration of ester and alkali .
`{:("Time",0,4,10,20),((min),,,,),("Vol of acid used",8.04,5.30,3.50,2.22),((mL),,,,):}`
Show that the reaction is of second order .View SolutionThe optical rotation of cane sugar in `0.5` N lactic acid at `25^(@)C` at various time intervals are given below :
`{:("Time",0,1435,11360,oo),((min),,,,),("Rotation",,,,),((.^(@)),34.50^(@),31.10^(@),13.98^(@),-10.77^(@)):}`
Show that the reaction is of first order .View SolutionIn the course of the reaction
`CH_(3) COOCH_(3) (g) to C_(2)H_(4)(g)+H_(2)(g) +CO(g)`
the initial pressure was found to be `0.42` atm while after `6.5` minutes , it was `0.54` atm . If the reaction follows first order kinetics find the rate constant.03:16View SolutionThe second order rate constant for `I^(-) +CIO^(-) to IO^(-) +Cl^(-) " is 0.06 (mol/litre)"^(-1) " M " ClO^(-) ` can you calculate the concentration of `ClO^(-)` after 5 minutes . If no , what additional information is required ?
02:33View SolutionThe rate law for a reaction is Rate = `K [A] [B]^(3//2)`
Can the reaction be an elementary process ? Explain.02:51View SolutionThe decomposition of `N_(2)O_(5)` according to the equation
`2N_(2)O_(5) (g) to 4NO_(2) (g) +O_(2)(g)`
is a first - order reaction . After 30 minutes from the start of the decomposition in a closed vessel , the total pressure developed is found to be `284.5` mmHg and an complete decomposition the total pressure is `584.5` mmHg . Calculate the rate constant of the reaction .04:50View SolutionWhich reaction will have the greater temperature dependence for the rate constant one with a small value E or one large value of E?
02:41View SolutionWhat is the value of the rate constant, predicated by the Arrhenius's equation if `Trarroo` ? It this value phyiscally resonable?
01:19View SolutionBicyclohexane was found to undergo two parallel first order rearrangements . At 730 K , the first order rearrangemen730 K , the first order rate constant for the formation of cyclohexane was measure as `1.26 xx10^(-1)s^(-1)` and for the formation of unethyl cyclopentene the rate constant was `3.8 xx 10^(-5) s^(-1)` . What is the percentage distribution of the rearrangement products ?
03:17View SolutionThe decomoposition of `N_(2)O` into `N_(2)` and O in the presence of argon follows second kinetics with ` k = (5.0 xx 10^(11)) e^(-29000K//T)` Calculate the energy of activation .
02:44View SolutionFor the displacement reaction
`[Co(NH_(3))_(5)Cl]^(2+) +H_(2)O to [ Co (NH_(3))_(5)(H_(2)O)]^(3+) +Cl^(-)`
the rate constant is given by
In` [ k//(min^(-1))] = (11067 K)/T + 31.33`
Evaluate k,E and a for the chemical reaction at `25^(@)C` .03:30View SolutionThe complexation of `Fe^(2+)` with the chelating agent dipyridyl has been studied kinetically in both forward and reverse directions .
`Fe^(2+) +3 dipy to Fe ("dipy")_(3)^(2+)`
Rate (forward) = `(1.45 xx10^(13)) [Fe^(2+)] ["dipy"]^(3)`
and rate (reverse) = `(1.22 xx10^(-4)) [Fe ("dipy")_(3)^(2+)]`
Find the stability constant for the complex .02:33View SolutionThe approach to the following equilibrium was observed kinetically from both directions `Pt Cl_(4)^(2-) +H_(2)O hArr Pt (H_(2)O) Cl_(3)^(-) +Cl^(-) "at " 25^(@)C . ` It was found that ` - (d[PtCl_(4)^(2-)])/(dt) = (3.9 xx10^(-5)) [Pt Cl_(4)^(2-)] - (2.1 xx10^(-3)) [ Pt (H_(2)O) Cl_(3)^(-) ] [Cl^(-)] `
Calculate the equilibrium constant for the complexation of the fourth `Cl^(-)` by Pt(II) .02:35View SolutionFor the reaction `2H_(2) + 2NO rarr N_(2) + 2H_(2)O`, the following mechanism has been suggested :
`2NO ? N_(2)O_(2)` equilibrium constant `K_(1)` (fast)
`N_(2)O_(2) +H_(2) overset(k_(2)) rarr N_(2)O +H_(2)O` (slow)
`N_(2) O +H_(2) overset(k_(3)) rarr N_(2)+H_(2)O` (fast)
Establish the rate law for given reaction.03:13View SolutionThe possible machanism for the reaction
`2NO+ Br_(2) to 2NOBr`
is `NO +Br_(2) to NOBr_(2) ` (fast)
`NOBr_(2) +NO to 2NO Br ` (slow)
Establish the rate law .04:03View SolutionFor the reaction

`k_(1) = 1.78 xx 10^(-3)s^(-1)` and `k_(2) = 5.8 xx 10^(-5)s^(-1)` for the initial concentration of `[Cr(H_(2)O)_(4)Cl_(2)]^(o+)` is `0.0174 mol L^(-)` at `0^(@)C`. Calculate the value of time at which the concentration of `[Cr(H_(2)O)_(5)Cl]^(2+)` is maximum.03:21View SolutionFor the reaction : `A+2B to P ` the rate law is given by rate ` = k [A] [B]^(2)`
From the following data , calculate x and y .
 .View Solution
.View SolutionSome `PH_(3)(g)` is introduced into a flask at `600^(@)C` containing an inert gas . `PH_(3)` proceed to decompose into `P_(4)(g) and H_(2)(g)` and the reaction goes to completion . The total pressure is given below as a function of time . Find the order of the reaction and calculate the rate constant for the reaction :
`4PH_(3) to P_(4) +6H_(2)`
 View Solution
View SolutionThe vapour pressure to two miscible liquids (A) and (B) are 300 and 500 mm of Hg respectively. In a flask 10 mole of (A) is mixed with 12 mole of (B). However, as soon as (B) is added, (A) starts polymerising into a completely insoluble solid. The polymerisation follows first-order kinetics. After 100 minutes, `0.525` mole of a solute is dissolved which arrests the polymerisation completely. The final vapour pressure of the solution is 400 mm of Hg. Estimate the rate constant of the polymerisation reaction. Assume negligible volume change on mixing and polymerisation and ideal behaviour for the final solution.
04:17View SolutionFrom the following reaction scheme , write the rate law for the disappearance of A,B and C .
1 . `A + B overset(k_(1)) C+D`
2 . `C+D overset(k_(2)) to A+B`
3. `B+C overset(k_(3)) E+D`02:30View Solution
EXAMPLES questions and complete solutions for chapter CHEMICAL KINETICS of BHARATI BHAWAN CHEMISTRY (HINGLISH) of Class 12
