Complete Solutions to Problems of chapter CURRENT AND RESISTANCE of Class 12 book with complete answers and questions
| Class 12 | PHYSICS |
| Chapter | CURRENT AND RESISTANCE |
| RESNICK AND HALLIDAY | Problems |
| Number Of Questions | 36 |
| Academic Year | 2023-24 |
A wire 8.00 m long and 6.00 mm in diameter has a resistance of 30.0 `mOmega`. A potential difference of 23.0 V is applied between the ends. (a) What is the current in the wire? (b) What is the magnitude of the current density? (c) Calculate the resistivity of the wire material.
View SolutionIn Fig. 26-18a, a 9.00 V battery is connected to a resistive strip that consists of three sections with the same cross-sectional areas but different conductivities. Figure 26-18h gives the electric potential V(x) versus position x along the strip. The horizontal scale is set by x= 8.00 mm. Section 3 has conductivity `4.00 xx 10^(7) (Omega.m)^(-1)`. What is the conductivity of section (a) 1 and (b) 2 ?
a.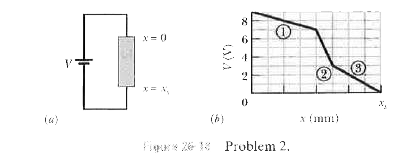
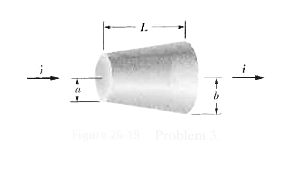
View SolutionIn Fig. 26.19, current is set up through a truncated right circular cone of resistivity `731 Omega.m` lelt radius d= 1.70 mm. right radius b= 2.30 mm, and length L= 3.50 cm. Assume that the current density is uniform across any cross section taken perpendicular to the length. What is the resistance of the conc?
View SolutionKiting during a storm. The legend that Benjamin Franklin flew a kite as a storm approached is only a legend-he was neither stupid nor suicidal. Suppose a kite string of radius 2.00 mm extends directly upward by 1.80 km and is coated with a 0.500 mm layer of water having resistivity `150Omega.m.` If the potential difference between the two ends of the string is 213 MV, what is the current through the water layer? The danger is not this current but the chance that the string draws a lightning strike, which can have a current as large as 500 000 A (way beyond just being lethal).
View SolutionEarth's lower atmosphere contains negative and positive ions that are produced by radioactive elements in the soil and cosmic rays from space. In a certain region, the atmospheric electric field strength is 120 V/m and the field is directed vertically down. This field causes singly charged positive ions, at a density of `640 cm^(-1)` to drift downward and singly charged negative ions, at a density of 550 cm`""^(-3)`. ? to drift upward (Fig. 26-20). The measured conductivity of the air in that region is `2.70 xx 10^(-14)W (Omega.m)^(-1)`. Calculate (a) the magnitude of the current densily and (b) the ion drift speed, assumed to be the same for positive and negative ions.
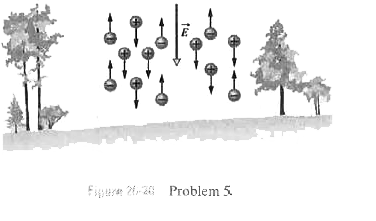
View SolutionA potential difference of 6.00 nV is set up across a 1.50 cm length of copper wire that has a radius of 2.00 mm. How much charge drifts through a cross section in 4.70 ms?
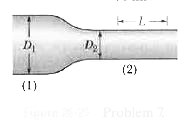
View SolutionFigure 26-21 shows wire section 1 of diameter `D_1= 4.00R` and wire section 2 of diameter `D_2= 1.75R,` connected by a tapered section. The wire is Copper and carries a current. Assume that the current is uniformly distributed across any cross-sectional area through the wire's width. The electric potential change V along the length L= 2.00 m shown in section 2 is `10.0 muV.` The number of charge carners per unit volume is `8.49 xx 10^(28) m^(-3).` What is the drift speed of the conduction electrons in section 1?
View SolutionWhen 230 V is applied across a wire that is 14.1 m long and has a 0.30 mm radius, the magnitude of the current density is `198 xx 10^(8) A//m^(2).` Find the resistivity of the wire.
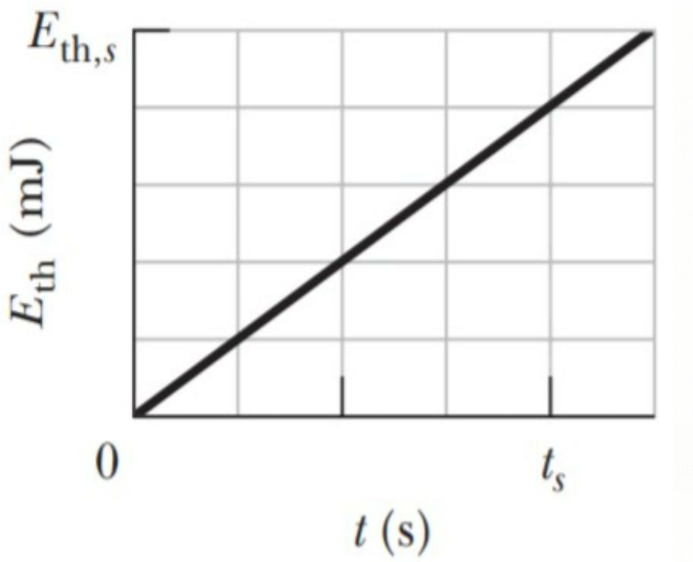
View SolutionIn Fig. 26-22a, a `15Omega` resister is connected to a battery. Figure 26-22b shows the increase of thermal energy `E_(th)` in the resistor as a function of time t. The vertical scale is set by `E_(th.s) = 2.50 mJ,` and the herizontal scale is set by `t_(s)= 4.0s`. What is the electric potential across the battery?
View SolutionNichrome wire consists of a nickel-chromium-iron alloy, is commonly used in heating elements such as on a stove, and has conductivity `2.0 xx 10^(6) (Omega.m)^(-1).` If a Nichrome wire with a cross-sectional area of 2.3 mm`""^(2)?` carries a current of 5.5 A when a 1.4 V potential difference is applied between its ends, what is the wire's length?
View SolutionA heater contains a Nichrome wire `("resistivity "5.0 xx 10^(-7)Omega.m) ` of length 5.85 m, with an end-to-end potential difference of 112 V and with a dissipation power of 4000 W.(a) What is the wire's cross-sectional area? (b) If 100 V is used to obtain the same dissipation rate, what should the length be?
View SolutionAn unknown resistor is connected between the terminals of a 3.00 V battery. Energy is dissipated in the resistor at the rate of 0.707 W. The same resistor is then connected between the terminals of a 12.0 V battery. At what rate is energy now dissipated?
View SolutionIn Fig. 26.23, a battery of potential differenc V= 12 V is connected to a resistive strip of resistance `R=4.0 Omega`. When an electron moves through the strip from one end to the other, (a) in which direction in the figure does the electron move, (b) how much work is done on the electron by the electric field in the strip, and (c) how much energy is transferred to the thermal energy of the strip by the electron?
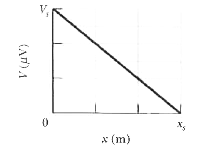
View SolutionFigure 26-24 gives the electric potential V(x) along a copper wire carrying uniform current, from a point of higher potential `V= 12.0 muV` at x= 0 to a point of zero potential at x= 3.00 m. The wire has a 0 radius of 2.20 mm. What is x (m) the current in the wire?.
View SolutionA 890 W radiant heater is constructed to operate at 115 V. (a) What is the current in the heater when the unit is operating? (b) What is the resistance of the heating coil? (c) How much thermal energy is produced in 5.00 h?
View SolutionWire C and wire D are made from different materials and have length `L_C = L_D= 1.0 m.` The resistivity and radius of wire C are `2.0 xx 10^(-6). Omega m` and 1.00 mm, and those of wire D are `1.0 xx 1.0^(-6)Omega. m`. and 0.50 mm. The wires are joined as shown in Fig. 26-25, and a current of 2.0 A is set up in them. What is the electric potential difference between a) points 1 and 2 and (b) points 2 and 3? What is the rate at which energy is dissipated between c) points 1 and 2 and (d) points 2 and 3?

View SolutionA small but measurable current of `1.2 xx 10^(-10) A` exists in a copper wire whose diameter is 3.0 mm. The number of charge carriers per unit volume is `8.49 xx 10^(28)m^(-3) m.` Assuming the current is uniform, calculate the (a) current density and (b) electron drift speed.
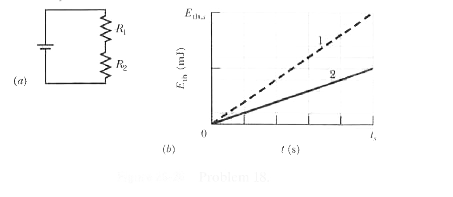
View SolutionThe current through the battery and resistors 1 and 2 in Fig. 26-26a is 1.50 A. Energy is transferred from the current to thermal energy `E_(th)` in both resistors. Curves 1 and 2 in Fig. 26-26b give that thermal energy Ein for resistors 1 and 2, respectively, as a function of time i. The vertical scale is set by `E_(th.s) =40.0mJ` and the horizotal scale is set by `t_(s)=5.00s`. What is the power of the battery?
View SolutionA copper wire of cross-sectional area `2.40 xx 10^(-6)m^(2)` and length 4.00 m has a current of 2.00 A uniformly distributed across that area. (a) What is the magnitude of the electric field along the wire? (b) How much electrical energy is transferred to thermal energy in 30 min?
View SolutionFor a current set up in wire for 28.0 d, a total of `1.36 xx 10^(26)` electrons pass through any cross section across the wire's width at a steady rate. What is the current?
View SolutionThe current-density magnitude in a certain circular wire is `J= (2.75 xx 10^(10) A//m^(4))r^(2)`, where r is the radial distance out to the wire's radius of 3.00 mm. The potential applied to the wire (end to end) is 80.0 V. How much energy is converted to thermal energy in 1.00 h?
View SolutionA charged belt, 50 cm wide, travels at 30 m/s between a source of charge (electrons) and a sphere. The belt carries charge into the sphere at a rate corresponding to 76 A. (a) Compute the surface charge density on the belt. (b) What is the number density (number per unit area) of the electrons on the belt?
View SolutionA wire initially has length `L_0` and resistance `5.00 Omega.` The resistance is to be increased to `45.0 Omega` by stretching the wire. Assuming that the resistivity and density of the material are unaffected by the stretching, find the ratio of the new length to `L_(0)`.
View SolutionA human being can be electrocuted if a current as small as 50 mA passes near the heail. An electrician working with sweaty hands makes good contact with the two conductors he is bolding one in each hand. If his resistance is `2100 Omega.` What might the fatal voltage be?
View SolutionA 120 V potential difference is applied to a space heater that dissipates 1500W during operation. (a) What is the resistance during operation? (b) At what rate do electrons flow through any cross section of the heater element?
View SolutionA certain wire has a resistance R. What is the resistance of a second wire, made of the same material, that is twice as long and has twice the diameter?
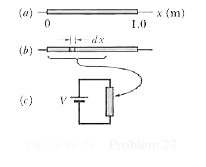
View SolutionFigure 26-27 a shows a rod of resistive material. The resistance per unit length of the rod increases in the positive direction of the x axis. At any position x along the rod, the resistance dR of a narrow (differential) section of width dx is given by dR =5.00x dx, where dR is in ohms and x is in meters. Figure 26-27 shows such a narrow section. You are to slice off a length of the rod between x= 0 and some position x= L and then connect that length to a battery with potential difference V=8.0 V (Fig. 26-27c). You want the current in the length to transfer energy to thermal energy at the rate of 180 W. At what position x =L should you cut the rod?
View SolutionA fuse in an electric circuit is a wire that is designed to melt, and thereby open the circuit, if the current exceeds a predetermined value. Suppose that the material to be used in fise melts whien the current derisity rises to 440 `A/cm^(2)`. What radius of cylindrical wire should be used to make a haal will little current to 6.0A?
View SolutionHow long does it take electrons to get from a car battery to the starting motor? Assume the current is 285 A and the electrons travel through a copper wire with cross-sectional area `0.17 cm^(2)` and length 0.43 m. The number of charge carriers per unit volume is `8.49 xx 10^(28) m^(-3).`
View SolutionWhat is the current in a wire of radius R = 2.67 mm if the magnitude of the current density is given by (a) `J_n= J_0 r//R and (b) J_b=J_0(1-r//R),` in which r is the radial distance and `J_0= 5.50 xx 10^(4) A//m^(2)?` (c) Which function maximizes the current density near the wire's surface?
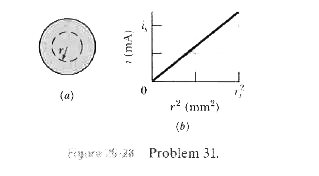
View SolutionA certain cylindrical wire carries current. We draw a circle of radius r around its central axis in Fig. 26-28a to determine the current i within the circle. Figure 26-28b shows current i as a function of `r^2.` The vertical scale is set by `i_s= 4.0 mA,` and the horizontal scale is set by `r_(s)^(2)= 8.0 mm^(2)`. (a) Is the current density uniform? (b) If so, what is its magnitude? (c) What is the current between r = 0 and r=2.0 mm?
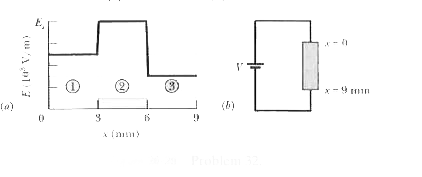
View SolutionFigure 26-29a gives the magnitude E(x) of the electric fields that have been set up by a battery along a resistive rod of length 9.00 mm (Fig. 26-296). The vertical scale is set by `E_(s)=8.00 xx 10^3 V//m`. The rod consists of three sections of the same material but with different radii. (The schematic diagram of Fig. 26.29b does not indicate the differencet radii). The radius of section 3 is 1.70 mm. What is the radius of (a) section 1 and (b) section 2?
View SolutionTwo conductors are made of the same material and have the same length. Conductor A is a solid wire of radius 1.0 mm. Conductor B is a hollow tube of outside radius 2.2 mm and inside radius 1.0 mm. What is the resistance rate `R_A//R_B` measured between their ends?
View SolutionNear Earth the density of protons in the solar wind (a stream of particles from the Sun) can be `4.63cm^(-3)` and their suced can be 391 km/s. (a) Find the current density of these protons. (b) If Earth's magnetic field did not deflect the protons, what total current would Earth receive?
View SolutionAn isolated conducting sphere has a 20 cm radius. One wire carries a current of 1.000 002 0 A into it. Another wire carries a current of 1.000 000 0 A out of it. How long would it take for the sphere to increase in potential by 1000 V?
View SolutionThe magnitude of the current density in a certain lab wire with a circular cross section of radius R=2.50 mm is given by `J= (3.00 xx 10^(8))r^(2)`, with J in amperes per square meter and radial distance rin meters. What is the current through the outer section bounded by r= 0.900R and r=R?
View Solution
Problems questions and complete solutions for chapter CURRENT AND RESISTANCE of RESNICK AND HALLIDAY PHYSICS (HINGLISH) of Class 12