Complete Solutions to PROBLEMS of chapter PHOTONS AND MATTER WAVES of Class 12 book with complete answers and questions
| Class 12 | PHYSICS |
| Chapter | PHOTONS AND MATTER WAVES |
| RESNICK AND HALLIDAY | PROBLEMS |
| Number Of Questions | 50 |
| Academic Year | 2023-24 |
A 100 W sodium lamp is radiating light of wavelength `5890A`, uniformly in all directions,
a. At what rate, photons are emitted from the lamp?
b. At what distance from the lamp, the average flux is 1 photon(`cm^2-s)^-1?`
c. What are the photon flux and photon density at 2m from the lamp?
View SolutionIn an old-fashioned television set, electrons are accelerated through a potential difference of 25.0 kV. What is the de Broglie wavelength of such electrons? (Relativity is not needed.)
View SolutionFind the maximum kinetic energy of electrons ejected from a certain material if the material's work function is 2.3 eV and the frequency of the incident radiation is `2.5 xx 10^(15) Hz`.
View SolutionCalculate the de Broglie wavelength of (a) a 2.00 keV electron, (b) a 2.00 keV photon, and (c) a 2.00 keV neutron.
View SolutionSolar radiations fall on Earth's surface at a rate of `1400 W//m`?. Assuming that the radiation has an average wavelength of 550 nm, how many photos per square meter per second fall on the surface?
View SolutionHow fast must an electron move to have a kinetic energy equal to the photon energy of sodium light at wavelength 500 nm?
View Solution1.5 mW of 400 nm light is directed at a photoelectric cell. If 0.1% of the incident photons produce photoelectrons, find the current in the cell.
View SolutionThe uncertainty in the position of an electron along an x axis is given as 50 pm, which is about equal to the radius of a hydrogen atom. What is the least uncertainty in any simultaneous measurement of the momentum component `P_(x)` of this electron?
View SolutionSuppose the fractional efficiency of a cesium surface (with work function 1.80 eV) is `1.0 xx 10^(-16)`, that is, on average one electron is ejected for every `10^(16)` photons that reach the surface. What would be the current of electrons ejected from such a surface if it were illuminated with 600 nm light from a 3.00 mW laser and all the ejected electrons took part in the charge flow?
View SolutionAn electron and a photon each have a wavelength of 0.25 nm. What is the momentum `("in "kg m//s)` of the (a) electron and (b) photon? What is the energy (in eV) of the (c) electron and (d) photon?
View SolutionThe function `Psi(x)` displayed in Eq. 37-19 can describe a free particle, for which the potential energy is U(x) = 0 in Schrodinger's equation (Eq. 37-11). Assume now that `U(x)=U_(0)= a` constant in that equation. Show that Eq. 37-19 is a solution of Schrodinger's equation, with
`k=(2pi)/(h)sqrt(2m(E-U_(0))`
giving the angular wave number k of the particle.
View SolutionShow that the angular wave number k for a nonrelativistic free particle of mass m can be written as
`k=(2pisqrt(2mk))/(h)`
in which K is the particle's kinetic energy.
View SolutionAn electron with total energy E = 5.1 eV approaches a barrier of height `U_(h) = 6.8 eV` and thickness L = 750 pm. What percentage change in the transmission coefficient T occurs for a `1.0%` change in (a) the barrier height, (b) the barrier thickness, and (c) the kinetic energy of the incident electron?
View SolutionAn ultraviolet lamp emits light of wavelength 400 nm at the rate of 400 W. An infrared lamp emits light of wave- length 700 nm, also at the rate of 400 W. (a) Which lamp emits photons at the greater rate and (b) what is that greater rate?
View SolutionThe smallest dimension (resolving power) that can be resolved by an electron microscope is equal to the de Broglie wavelength of its electrons. What accelerating voltage would be required for the electrons to have the same resolving power as could be obtained using 120 keV gamma rays?
View SolutionThe wavelength of the yellow spectral emission line of sodium is 590 nm. At what kinetic energy would an electron have that wavelength as its de Broglie wavelength?
View SolutionA nonrelativistic particle is moving three times as fast as an electron. The ratio of the de Broglie wavelength of the particle to that of the electron is `1.813 xx 10^(-4)`. By calculating its mass, identify the particle.
View Solution(a) Suppose a beam of 4.5 eV protons strikes a potential energy barrier of height 6.0 eV and thickness 0.70 nm, at a rate equivalent to a current of 1000 A. How long would you have to wait-on average-for one proton to be transmitted? (b) How long would you have to wait if the beam consisted of electrons rather than protons?
View SolutionAn isolated copper sphere of radius 5.0 cm, initially uncharged, is illuminated by ultraviolet light of wave- length 200 nm. What charge will the photoelectric effect induce on the sphere. The work function for copper is 4.70 eV.
View SolutionAn orbiting satellite can become charged by the photo- electric effect when sunlight ejects electrons from its outer surface. Satellites must be designed to minimize such charging because it can ruin the sensitive microelectronics. Suppose a satellite is coated with platinum, a metal with a very large work function `(Phi= 5.32 eV)`. Find the longest wavelength of incident sunlight that can eject an electron from the platinum.
View SolutionThe work function of tungsten is 4.50 eV. Calculate the speed of the fastest electrons ejected from a tungsten sur- face when light whose photon energy is 5.80 eV shines on the surface.
View SolutionA helium-neon laser emits red light at wavelength `lambda`=633 nm in a beam of diameter 3.0 mm and at an energy-emission rate of 5.0 mW. A detector in the beam's path totally absorbs the beam. At what rate per unit area does the detector absorb photons?
View SolutionA light detector (your eye) has an area of `2.00 xx 10^(-6) m^(2)` and absorbs `80%` of the incident light, which is at wavelength 500 nm. The detector faces an isotropic source, 3.00 m from the source. If the detector absorbs photons at the rate of exactly `4.000 s^(-1)`, at what power does the emitter emit light?
View SolutionIf the de Broglie wavelength of a proton is 100 fm, (a) what is the speed of the proton and (b) through what electric potential would the proton have to be accelerated to acquire this speed?
View SolutionWhat is the wavelength of (a) a photon with energy 1.00 eV, (b) an electron with energy 1.00 eV and (c) a photon of energy 1.00 GeV?
View SolutionThe wavelength associated with the cutoff frequency for silver is 325 nm. Find the maximum kinetic energy of electrons ejected from a silver surface by ultraviolet light of wavelength 275 nm.
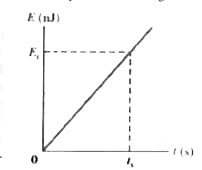
View SolutionA light detector has an absorbing area of `3.00 xx 10^(-6) m^(2)` and absorbs `50%` of the incident light, which is at wavelength 600 nm. The detector faces an isotropic source, 12.0 m from the source. The energy E emitted by the source versus time t is given in Fig. 37-19 Problem 28.
At what rate are photons absorbed by the detector?
View SolutionAt what rate does the Sun emit photons? For simplicity, assume that the Sun's entire emission at the rate of `3.9 xx 10^(26)` W is at the single wavelength of 550 nm.
View SolutionWhat (a) frequency, (b) photon energy, and (c) photon momentum magnitude are associated with x rays having wavelength 35.0 pm?
View SolutionThe beam emerging from a 1.5 W argon laser `(lambda=515 nm)` has a diameter d of 3.5 mm. The beam is focused by a lens system with an effective focal length `f_(L)` of 2.5 mm. The focused beam strikes a totally absorbing screen, where it forms a circular diffraction pattern whose central disk has a radius R given by `1.22f_(L)lambda//d`. It can be shown that `84%` of the incident energy ends up within this central disk. At what rate are photons absorbed by the screen in the central disk of the diffraction pattern?
View SolutionAn electron moves through a region of uniform electric potential of -200 V with a (total) energy of 500 eV. What are its (a) kinetic energy (in electron-volts), (b) momentum, (c) speed, (d) de Broglie wavelength, and (e) angular wave number?
View Solution(a) If the work function for a certain metal is 1.8 e V, what is the stopping potential for electrons ejected from the metal when light of wavelength 400 nm shines on the metal? (b) What is the maximum speed of the ejected electrons?
View SolutionHow fast must an electron move to have a kinetic energy equal to the photon energy of sodium light at wavelength 588 nm?
View SolutionA special kind of light bulb emits monochromatic light of wavelength 630 nm. Electrical energy is supplied to it at the rate of 60 W, and the bulb is `93%` efficient at converting that energy to light energy. How many photons are emitted by the bulb during its lifetime of 730 h?
View SolutionThe stopping potential for electrons emitted from a surface illuminated by light of wavelength 491 nm is 0.710 V. When the incident wavelength is changed to a new value, the stopping potential is 1.43 V. (a) What is this new wave- length? (b) What is the work function for the surface?
View SolutionWhat is the photon energy for yellow light from a highway sodium lamp at a wavelength of 589 nm?
View SolutionYou wish to pick an element for a photocell that will operate via the photoelectric effect with visible light. Which of the following are suitable (work functions are in parentheses): tantalum (4.2 eV), tungsten (4.5 eV), aluminum (4.2 eV), barium (2.5 eV), lithium (2.3 eV)?
View SolutionLight strikes a sodium surface, causing photoelectric emission. The stopping potential for the ejected electrons is 5.0 V, and the work function of sodium is 2.2 eV. What is the wavelength of the incident light?
View SolutionIn a photoelectric experiment using a sodium surface, you find a stopping potential of 1.85 V for a wavelength of 300 nm and a stopping potential of 0.820 V for a wavelength of 400 nm. From these data find (a) a value for the Planck constant, (b) the work function `Phi` for sodium, and (c) the cutoff wavelength `lambda_(0)` for sodium.
View SolutionSingly charged sodium ions are accelerated through a potential difference of 300 V. (a) What is the momentum acquired by such an ion? (b) What is its de Broglie wavelength?
View SolutionA satellite in Earth orbit maintains a panel of solar cells of area `2.90 m^(2)` perpendicular to the direction of the Sun's light rays. The intensity of the light at the panel is `1.39 kW//m^(2)`. (a) At what rate does solar energy arrive at the panel? (b) At what rate are solar photons absorbed by the panel? Assume that the solar radiation is mono- chromatic, with a wavelength of 550 nm, and that all the solar radiation striking the panel is absorbed. (c) How long would it take for a "mole of photons" to be absorbed by the panel?
View SolutionLight of wavelength 200 nm shines on an aluminum surface, 4.20 eV is required to eject an electron. What is the kinetic energy of (a) the fastest and (b) the slowest ejected electrons? (c) What is the stopping potential for this situation? (d) What is the cutoff wavelength for aluminum?
View SolutionAt one time, the metre was defined as 1650763.73 wavelengths of the orange light emitted by a light source containing `Kr^(86)` atoms. What is the corresponding photon energy of this radiation?
View SolutionX rays with a wavelength of 71 pm are directed onto a gold foil and eject tightly bound electrons from the gold atoms. The ejected electrons then move in circular paths of radius r in a region of uniform magnetic field `vecB`. For the fastest of the ejected electrons, the product Br is equal to `1.88 xx 10^(-4)` T.m. Find (a) the maximum kinetic energy of those electrons and (b) the work done in removing them from the gold atoms.
View SolutionWhat are (a) the energy of a photon corresponding to wavelength 1.00 nm, (b) the kinetic energy of an electron with de Broglie wavelength 1.00 nm, (c) the energy of a photon corresponding to wavelength 1.00 fm, and (d) the kinetic energy of an electron with de Broglie wavelength 1.00 fm?
View SolutionFind the (a) maximum kinetic energy of photoelectrons ejected from potassium surface, for which , `phi=2.1 eV`, by photons of wavelengths 2000 Å and 5000 Å. (b) What is the threshold frequency `v_(0)` and (c) the corresponding wavelength?
View SolutionA parellel beam of monochromatic light of wavelength 500 nm is incident normally on a perfectly absorbing surface. The power through any cross section of the beam is 10 W. Find
(a) the number of photons absorbed per second by the surface and
(b) the force exerted by the light beam on the surface.
View SolutionA parallel beam of monochromatic light of wavelength 663 nm is incident on a totally reflecting plane mirror. The angle of incidence is `60^@` and the number of photons striking the mirror per second is `(1.0 xx (10^19))`. Calculate the force exerted by the light beam on the mirror.
View SolutionA beam of white light is incident normally on a plane surface absorbing `50%` of the light and reflecting the rest. If the incident beam carries 10 W of power, find the force exerted by it on the surface.
View SolutionA small laser emits light at power 5.00 mW and wave- length 633 nm. The laser beam is focused (narrowed) until its diameter matches the 1266 nm diameter of a sphere placed in its path. The sphere is perfectly absorbing and has density `5.00 xx 10^(3)' kg/m^(3). What are (a) the beam intensity at the sphere's location, (b) the radiation pressure on the sphere, (c) the magnitude of the corresponding force, and (d) the magnitude of the acceleration that force alone would give the sphere?
View Solution
PROBLEMS questions and complete solutions for chapter PHOTONS AND MATTER WAVES of RESNICK AND HALLIDAY PHYSICS (HINGLISH) of Class 12