Complete Solutions to Problem of chapter ELECTRIC POTENTIAL AND ELECTRIC FLUX of Class 12 book with complete answers and questions
| Class 12 | PHYSICS |
| Chapter | ELECTRIC POTENTIAL AND ELECTRIC FLUX |
| SL ARORA | Problem |
| Number Of Questions | 15 |
| Academic Year | 2023-24 |
The electric potential V at any point x, y, z (all in meters) in space is given by `V=4x^2` volts. The electric field at the point (1m, 0, 2m) is……………..`V//m`.
View SolutionA radioactive source, in the form of a metallic sphere of radius `10^(-2)m` emits `beta-`particles at the rate of `5xx10^(10)` particles per second. The source is electrically insulated. How long will it take for its potential to be raised by `2V`, assuming that `40%` of the emitted `beta-`particles escape the source.
View SolutionAn infinite number of charges each equal to q, are placed along the X-axis at `x = 1, x = 2, x = 4, x = 8`,…….. and so on.
(i) find the electric field at a point `x = 0` due to this set up of charges.
(ii) What will be the electric field if the above setup, the consecutive charges have opposite signs.
View SolutionA charge Q is distributed over two concentric hollow spheres of radii r and `R (gt r)` such that the surface charge densities are equal. Find the potential at the common centre.
View SolutionThree concentric spherical metallic shells A, B and C of radii a, b and c (a lt b ltc) have surface charge densities `sigma`, `-sigma` and `sigma` respectively.
`(i) Find the potential of the three shells A, B and C.
(ii) If the shells A and C are at the same potential, obtain the relation between the radii a, b and c.
View SolutionTwo identical thin ring, each of radius R meters, are coaxially placed a distance R metres apart. If `Q_1` coulomb, and `Q_2` coulomb, are repectively the charges uniformly spread on the two rings, the work done in moving a charge q from the centre of one ring to that of the other is
View SolutionTwo circular loops of radii `0.05` and `0.09 m`, respectively, are put such that their axes coincide and their centers are `0.12 m` apart. A charge of `10^-6 C` is spread uniformly on each loop. Find the potential difference between the centers of the loops.
View SolutionA drop of water of mass `18xx10^-3` g falls away from the bottom of a charged conducting sphere of radius 20 cm, carrying with it a charge of `10^(-9)C` and leaving on the sphere a uniformly distributed charge of `2.5xx10^(-6)C`. What is the speed of the drop after it has fallen 30 cm? `(4piepsi_(0))^(-1)=9xx10^(9)JmC^(-2)`
View SolutionThree charges ` 0.1` coulomb each are placed on the corners of an equilateral triangle of side `1 m`. If the energy is supplied to this system at the rate of ` 1 kW` how much time would be required to move one to the charges on to the midpoint of the line joining the two ?
View SolutionThree point charges `1C, 2C` and `3C` are placed at the corners of an equilaternal triangle of side `1m`. The work required to move these charges to the corners of a smaller equilaternal triangle of side `0.5 m` in two differenct ways as in fig. `(A)` and fig. `(B)` are `W_(a)` and `W_(b)` then:
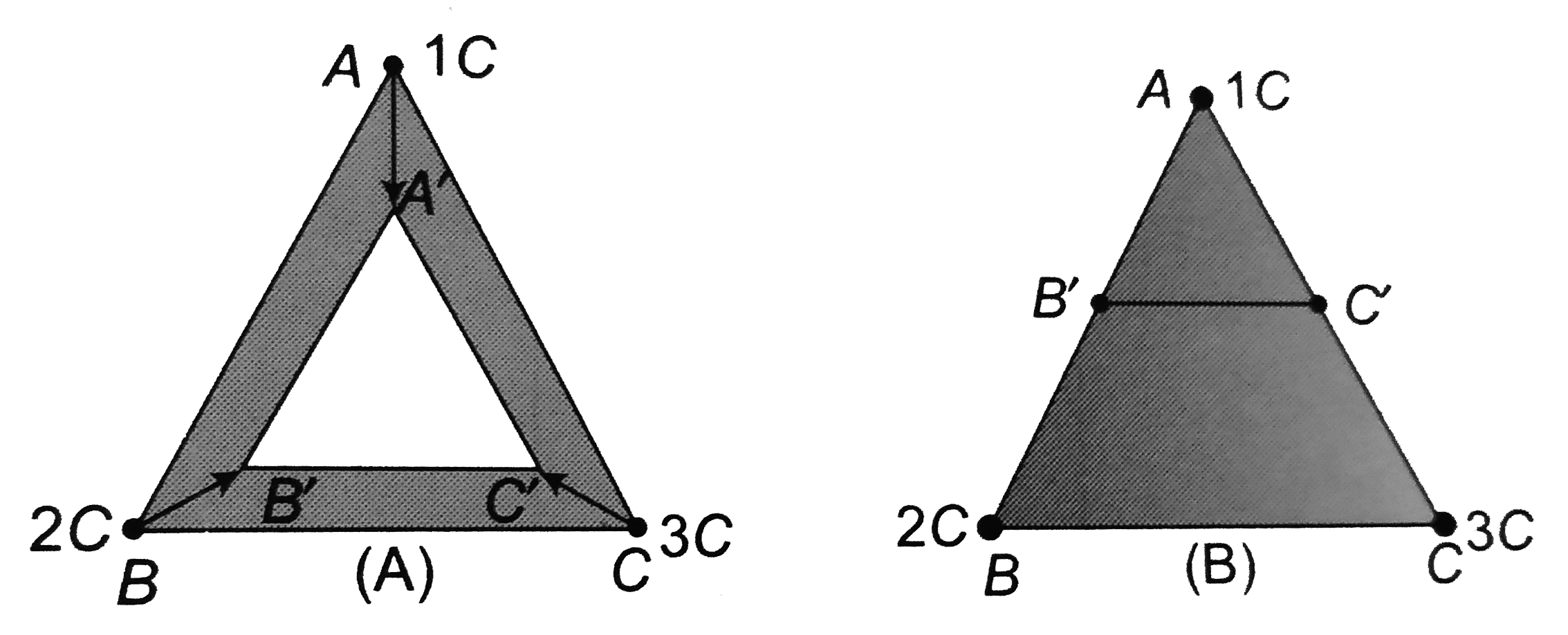
View SolutionThree point charges q, 2q and 8q are to be placed on a
. 9cm long straight line. Find the
. positions where the charges shouldbe placed such that the potential energy
. of this sysrem is minimum. In this situation, what is the
. electric field at the charge q due to the other two charges?
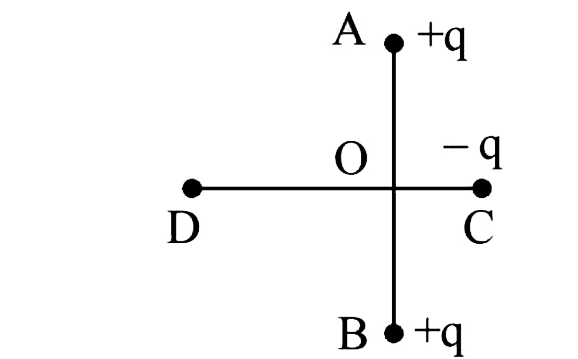
View SolutionTwo fixed, equal, positive charges, each of magnitude `5xx10^-5` coul are located at points A and B separated by a distance of 6m. An equal and opposite charge moves towards them along the line COD, the perpendicular bisector of the line AB.
The moving charge, when it reaches the point C at a distance of 4m from O, has a kinetic energy of 4 joules. Calculate the distance of the farthest point D which the negative charge will reach before returning towards C.
View SolutionThree charges each of value q are placed at the corners of an equilateral triangle. A fourth charge Q is placed at the center of the triangle.
a. Find the net force on charge q.
b. If `Q = -q`, will the charges at the corners move toward the center or fly away from it ?
c. For what value of Q and O will the charges remain stationary ?
d. In situation (c), how much work is done in removing the charges to infinity ?
View Solution(i). A charge of Q coulomb is uniformly distributed over a spherical volume of radius R metre Obtain an expression for the energy of the system.
(ii). What will be the corresponding expression for the energy needed to completely diassemble the planet earth against the gravitational pull amongst its constituent particles? Assume the earth to be a sphere of uniform mass density. calculate the energy, given that the product of the mass and the radius of the earth to be `2.5xx10^(31)kg-m`
View SolutionA proton moves with a speed of `0.75xx10^(5)ms^(-1)` directly towards a free proton originally at rest. Find the distance of closest approach of the two protons. Mass of proton `=1.66xx10^(-27)kg`.
View Solution
Problem questions and complete solutions for chapter ELECTRIC POTENTIAL AND ELECTRIC FLUX of DHANPAT RAI & CO PHYSICS (HINGLISH) of Class 12