Complete Solutions to TEST YOURSELF (MULTIPLE CHOICE QUESTION) of chapter CIRCLES of Class 9 book with complete answers and questions
| Class 9 | MATHS |
| Chapter | CIRCLES |
| CENGAGE | TEST YOURSELF (MULTIPLE CHOICE QUESTION) |
| Number Of Questions | 24 |
| Academic Year | 2023-24 |
How many circles can pass through two fixed points?
View SolutionHow many circles can pass through three non-collinear points?
View SolutionHow many circles can pass through three collinear points?
View SolutionA secant L intersects circle with radius a at two distinct points A and B in such a way that central angle is `60^(@)`. Then length of AB is
View SolutionRatio of circumference and radius of a circle is equal to
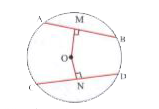
If AB = CD and O is the centre of circle then which of the following can be the values of OM and ON (in cm), respectively?View SolutionIf central angle of an arc is `60^(@)` then the ratio of areas of minor sector and major sector will be
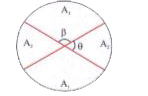
View SolutionIf two diameters of a circle divide it in such a way that the area of one part is 3 times the other, then acute angle between diameters is
 View Solution
View SolutionDistance between two parallel chords of lengths 8 cm and 6 cm is 1 cm. Then radius of circle will be
View Solution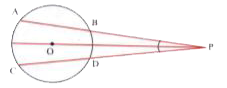
If OP is the angular bisector of angle BPD an O is the centre, then which of the following is correct?View Solution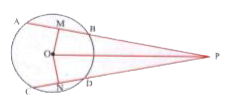
If OM = ON and `angleOMP = angleONP = 90^(@)`, where O is the centre, then which of following is correct?View Solution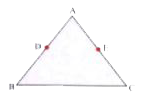
ABC is an isosceles triangle with AB = AC. If AD = AE then choose the correct alternatives.View Solution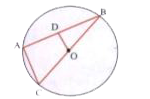
In the above diagram, BC is diameter, O is centre and `OD_|_AB`. If `AC=lambdaOD`, then value of `lambda` isView Solution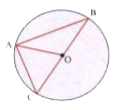
In the above figure, O is the centre and BC = 6 cm. If `angleOBA=45^(@)`, then area `(DeltaOAB)` isView Solution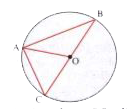
In the above figure, BC is diameter and O is centre. If area `(DeltaOAB)=k xx` area `(DeltaOAC)` then K isView Solution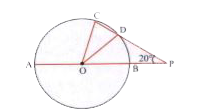
In the above figure, AB is diameter with length 2x. If `angleAPC=20^(@) and OD = DP`, where O is the centre, then length of AC isView SolutionA square of side length 2 cm is circumscribed by a circle, then area of circle is
View Solution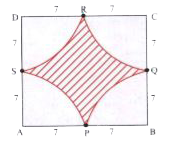
In the above figure, ABCD is a square of side length 14 cm. Area of shaded region will be `(pi=(22)/(7))`View Solution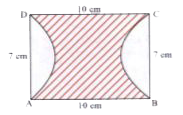
In the above figure, ABC is an equilateral triangle of side length `sqrt(3)cm`. Radius of incircle isView Solution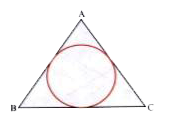
In the above figure, `C_(1), C_(2) and C_(3)` are semicircles of diameters 14 cm, 7 cm and 7 cm, respectively. Area of shaded region is `(pi=(22)/(7))`View Solution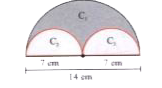
In the above figure `C_1,C_2` and `C_3` are semicircles of diameters 14 cm, 7 cm and 7 cm, respectively. Area of shaded region is `(pi=22/7)`View Solution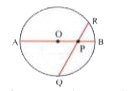
In the above figure, AB is diameter of length 10 cm. If `angleAPQ=45^(@) and QR=8cm` then length of OP isView Solution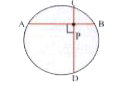
In the above circle two chords AB and CD intersect at `90^(@)`. If AP = 8 cm, PB = 4 cm, PC = 3 cm and PD = 9 cm, then radius of circle isView SolutionIf perimeter of an equilateral triangle is equal to circumference of a circle with radius r. Then height of triangle is
View SolutionIf radius of circle `C_(1)` is 2 times the radius of circle `C_(2)`, then ratio of area `C_(1)` to that of `C_(2)` is
View Solution
TEST YOURSELF (MULTIPLE CHOICE QUESTION) questions and complete solutions for chapter CIRCLES of CENGAGE of Class 9
