Complete Solutions to EXERCISE 13(B) of chapter PYTHAGORAS THEORAM of Class 9 book with complete answers and questions
| Class 9 | MATHS |
| Chapter | PYTHAGORAS THEORAM |
| SELINA PUBLICATION | EXERCISE 13(B) |
| Number Of Questions | 18 |
| Academic Year | 2023-24 |
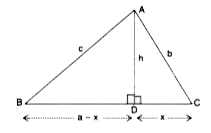
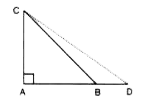
In the figure, given below, AD `_|_` BC.
Prove that : `c^(2)=a^(2)+b^(2)-2ax`.
View SolutionIn equilateral `/_\ABC, AD _|_ BC` and BC=x cm. Find, in terms of x, the length of AD.
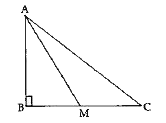
View SolutionABC is a triangle, right angled at B, M is a point on BC. Prove that :
`AM^(2) + BC^(2) = AC^(2) + BM^(2)`
View SolutionM and N are mid- point on sides QR and PQ respectively of `/_\ PQR`, right-anggled at Q. Prove that :
`PM^(2)+RN^(2)= 5 MN^(2)`.
View SolutionM and N are mid point on sides QR and PQ respectively of `/_\ PQR`, right-anggled at Q. Prove that :
`4PM^(2)= 4PQ^(2)+QR^(2)`.
View SolutionM and N are point on sides QR and PQ respectively of `/_\ PQR`, right-anggled at Q. Prove that :
`4PM^(2)= 4PQ^(2)+QR^(2)`.
View SolutionIn Figure, `Pa n dQ` are the midpoints of the sides `C Aa n dC B` respectively of ` A B C` right angled at C. Prove that `4(A Q^2+B P^2)=5A B^2` .
View Solutionabout to only mathematics
View SolutionIn a rectangle ABCD, prove that : `AC^(2)+BD^(2)=AB^(2)+BC^(2)+CD^(2)+DA^(2)`.
View SolutionIn a quadrilateral ABCD, `angleB=90^(@) and angleD=90^(@)`. Prove that :
`" "2AC^(2)-AB^(2)=BC^(2)+CD^(2)+DA^(2)`
View SolutionO is any point inside a rectangle ABCD. Prove that `O B^2+O D^2=O A^2+O C^2`.
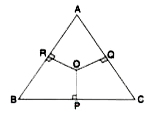
View SolutionIn the following figure, OP, OQ and OR are drawn perpendiculars to the sides BC, CA and AB repectively of triangle ABC. Prove that :
`AR^(2)+BP^(2)+CQ^(2)=AQ^(2)+CP^(2)+BR^(2)`
View SolutionDiagonals of rhombus ABCD intersect each other at point O. Prove that :
`" "OA^(2)+OC^(2)=2AD^(2)-(BD^(2))/2`
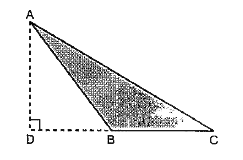
View SolutionIn the figure AB = BC and AD is perpendicular to CD. Prove that :
`" "AC^(2)=2.BC.DC`
View SolutionIn an isosceles triangle ABC, AB = AC and D is a point on BC produced. Prove that :
`AD^(2)=AC^(2)+BD.CD`
View SolutionIn triangle ABC, angle `A =90^(@), CA=AB` and D is a point on AB produced. Prove that:
`DC^(2)-BD^(2)= 2AB.AD`.
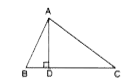
View SolutionIn triangle ABC, AB=AC and BD is perpendicular to AC. Prove that : `BD^(2)-CD^(2)=2CDxxAD`
View SolutionIn the following figure, AD is perpendicular to BC and D divides BC in the ratio 1 : 3.
View Solution
EXERCISE 13(B) questions and complete solutions for chapter PYTHAGORAS THEORAM of ICSE of Class 9