Complete Solutions to EXERCISE 7.3 of chapter TRIANGLES of Class 9 book with complete answers and questions
| Class 9 | MATHS |
| Chapter | TRIANGLES |
| KUMAR PRAKASHAN | EXERCISE 7.3 |
| Number Of Questions | 5 |
| Academic Year | 2023-24 |
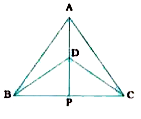
`triangle` ABC and `triangle` DBC are two isosceles triangles on the same base BC and vertices A and D are on the same side of BC (see the given figure). If AD is extended to intersect BC at P, show that, ( i ) `triangle ABD = triangle ACD (ii) triangle ABP = triangle ACP` (iii) AP bisects `angle` A as well as `angle` D. (iv) AP is the perpendicular bisector of BC
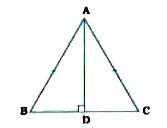
View SolutionAD is an altitude of an isosceles triangle ABC in which AB = AC. Show that: ( i ) AD bisects BC ( ii) AD bisects `angle` A
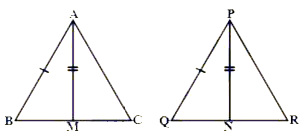
View SolutionTwo sides AB, BC and median AM of one triangle ABC are respectively equal to sides PQ and QR and median PN of `DeltaPQR` (See figure). Show that:
`(i) DeltaABM ~= DeltaPQN`
`(ii) DeltaABC ~= DeltaPQR`
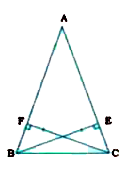
View SolutionBE and CF are two equal altitudes of a triangle ABC. Using RHS congruence rule, prove that the triangle ABC is isosceles.
View SolutionABC is an isosceles triangle with AB = AC. Draw AP `bot` BC to show that `angle B = angle C`
View Solution
EXERCISE 7.3 questions and complete solutions for chapter TRIANGLES of KUMAR PRAKASHAN KENDRA MATHS (GUJRATI ENGLISH) of Class 9