INTRODUCTION TO TRIGONOMETRY for Class 10 MATHS
INTRODUCTION TO TRIGONOMETRY
To bachcho apne triangles ke baare mein to apni pichli classes mein padha hai, particularly right triangle ke baare mein chahe fir wo similarity of triangle ho yaa Pythagoras theorem etc.
Es chapter mein hum triangle ki side and angle ke beech mein relationship smjhenge. Bachcho sabse pehle hum ye dekhte hai ki why we are studying this chapter ? Isko samjhne ke liye chalo apne surroundings se hum kuch live examples lete hai. Manlo aap apne friends ke sath delhi ghumne gye aur Qutub Minar pahuch gye, Ab aapko Qutub Minar ki height nikalni hai to aap kaise nikalenge ?
Chalo ek aur situation consider karte hai manlo apne ek hot air balloon dekha aur jaise hi apne usko dekha aap apni mom ko dikhane ke liye bula ke late ho aur itni der mein balloon position A se B pe aa gya. To kya aap balloon B aur ground ke beech ka altitude nikal sakte hai ?
Esi tarh ki problems ko solve karne ke liye hum trigonometry ka use karte hai. ‘Trigonometry’ word derive hua hai greek words se in which ‘tri’ (meaning three), ‘gon’ (meaning sides) and ‘metron’ (meaning measure). In fact, trigonometry is the study of relationships between the sides and angles of a triangle. Astronomers iska use stars aur planets ke beech ka distance find karne ke liye karte they lekin ab Engineering aur physical sciences mein bahut se technologically advanced methods trigonometric concepts pe based hai.
Hum es chapter mein padhne vale hai about Trigonometric ratios of an acute angle of a right-angled triangle. Proof of their existence (well defined). Values of the trigonometric ratios of 30∘, 45∘ and 60∘. Relationships between the ratios, proof and applications of some simple trigonometric identities.
Trigonometric Ratios
To bachcho jaisa ki triangles chapter mein apne sides ke ratio ke baare mein padha hai vse hi abhi hum ek right angle triangle ki sides ke ratios nikalenge. To chalo ek example consider karte hai, 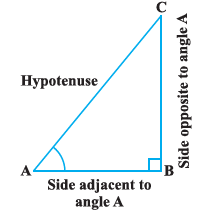
To maan lo humne ye right angle triangle ABC ko consider kiya as shown in figure above.
To dekho yha ∠CAB (or angle A) ek acute angle hai aur side BC ka position note karo w.r.t. ∠A. It faces ∠A. We call it the side opposite to ∠A. Aur jaisa ki hum dekh saktey hai ki BC perpendicular, AC
hypotenuse aur AB base(side adjacent to ∠A) of right angle triangle hai. Humey ye yaad rakhna hai ki agar hum ∠C ko ∠A ki jagh
consider kartey ha to sides ka position change ho jayega.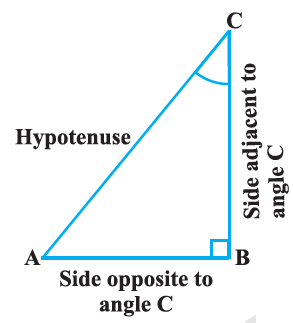
Ab hum kuch aise ratios ko define karenge jisme sides of a right triangle involved hongi. You can see that es triangle mein 3 sides hai AB,BC and AC aur agar hum inke ratio nikale to hume 6 ratios milenge, BCAC ,ABAC ,BCAB ,ACBC ,ACAB ,ABBC. Inhi ratios ko hum trigonometric ratios bolte hai.
The trigonometric ratios of the ∠A in right triangle ABC are defined as follows:
1. sine of ∠A
=side opposite to angle AHypotenuse
=PerpendicularHypotenuse
=BCAC
2. cosine of ∠A
=side adjacent to angle AHypotenuse
=BaseHypotenuse
=ABAC
3. tangent of ∠A
=side opposite to angle Aside adjacent to angle A
=PerpendicularBase
=BCAB
4. cosecant of ∠A
=1sine of∠A
=Hypotenuseside opposite to angle A
=HypotenusePerpendicular
=ACBC
5. secant of ∠A
=1cosine of∠A
= Hypotenuseside adjacent to angle A
=HypotenuseBase
=ACAB
6. cotangent of ∠A
=1tangent of∠A
=side adjacent to angle Aside opposite to angle A
=BasePerpendicular
=ABBC
In ratios ko short mei hum sinA, cosA, tanA, cosecA, secA and cotA kahte hai. And cosecA,secA, aur cotA reciprocals hai sinA,cosA, aur tanA ke respectively.
tanA = sinAcosA and cotA = cosAsinA
So the trigonometric ratios of an acute angle in a right triangle express the relationship between the angle and the length of its sides.
Note : The values of the trigonometric
ratios of an angle do not vary with the lengths of the sides of the triangle, if
the angle remains the same.
Example 1
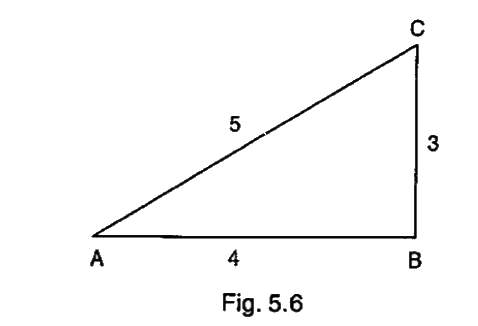
In a ΔABC right-angled at B, if AB = 4, BC = 3, find all the six trigonometric ratios of ∠A.
We have, AB=4 and BC=3.
Ab hum yha per Pythagoras theorem ko apply karte hai to, we have
AC2=AB2+BC2
⇒AC=√AB2+BC2
⇒AC=√42+32=√25=5
When we consider the t-ratios of ∠A, we have
Base = adjacent side to ∠A=AB=4, Perpendicular = opposite side to ∠A=BC=3 and Hypotenuse =AC=5.
∴ sinA=BCAC=35,
cosA=ABAC=45,
tanA=BCAB=34,
cosecA=ACBC=53,
secA=ACAB=54,
and cotA=ABBC=43
Example 2
In ΔPQR, right - angled at Q, PR+QR=25cm and PQ=5cm. Determine the values of sinP, cosP and tanP.
It is given that △PQR is right angled at Q.
Let's say QR is the side opposite to angle P and PQ adjacent to angle
P.
And PR+QR=25cm
So, PR=(25−QR)cm ...(i)
In △PQR, by Pythagoras theorem, we have
PR2=QR2+PQ2
⇒(25−QR)2=QR2+52 (From eq. (i))
⇒625+QR2−50QR=QR2+25
(By using (a−b)2=a2+b2−2ab)
⇒50QR=625−25=600
⇒QR=12cm
Therefore, from (i) PR=(25−12)cm=13cm
Now, sinP=QRPR=1213
cosP=PQPR=513
and tanP=QRPQ=125
Trigonometric Ratios of Some Specific Angles
To bachcho geometry se aap sab already familiar hai so you also know the construction of angles of 0∘, 30∘, 45∘, 60∘ and 90∘. To ab hum inhi angles ki trigonometric ratios ko find out karenge.
Trigonometric Ratios of 45∘
In △ABC, right-angled at B, if one angle is 45∘, then the other angle is also 45∘, i.e., ∠A=∠C=45∘
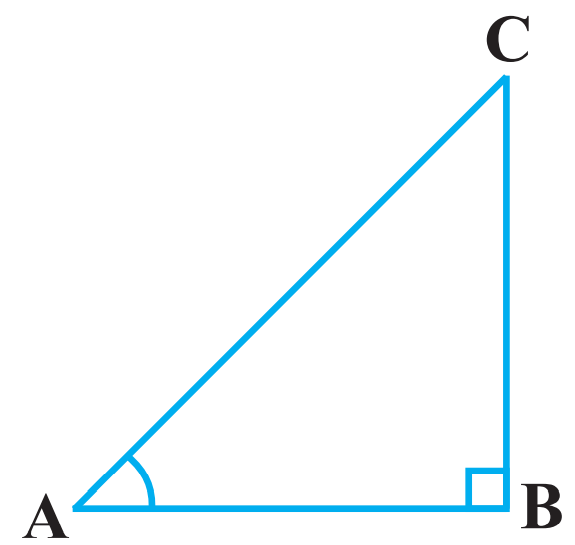
Since we know that, in triangle sides opposite to equal angle are equal, So BC=AB.
Suppose BC=AB=a
Now, by Pythagoras Theorem, AC2=AB2+BC2
=a2+a2=2a2
AC=a√2
Using the definitions of the trigonometric ratios, we have :
sin45∘= side opposite to angle 45∘ hypotenuse =BCAC=aa√2=1√2
cos45∘= side adjacent to angle 45∘ hypotenuse =ABAC=aa√2=1√2
tan45∘= side opposite to angle 45∘ side adjacent to angle 45∘=BCAB=aa=1
cosec45∘=1sin45∘=√2, sec45∘=1cos45∘=√2, cot45∘=1tan45∘=1
Trigonometric Ratios of 30∘ and 60∘
Consider an equilateral triangle ABC. Since each angle in an equilateral triangle is 60∘, therefore, ∠A=∠B=∠C=60∘. Draw the perpendicular AD from A to the side BC
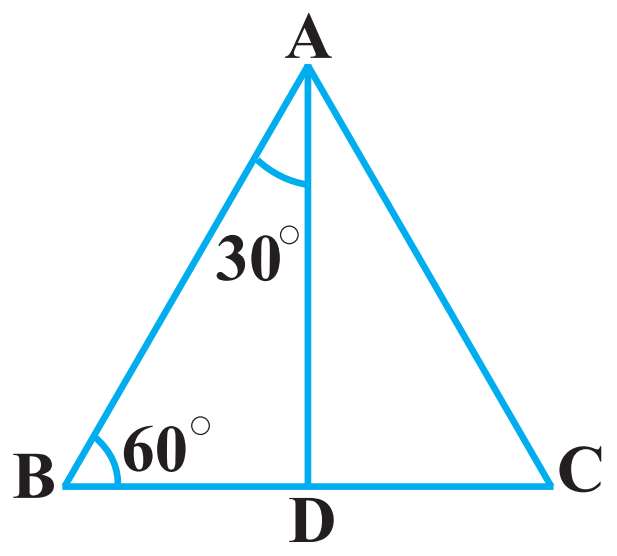
Now in △ABD and △ACD
AD=AD [common]
∠ADB=∠ADC=90∘
AB=AC [side of equilateral △]
Therefore, ΔABD≅ΔACD (By RHS Congruence rule)
Therefore, BD=DC and ∠BAD=∠CAD (CPCT)
So, ∠BAD = 12(∠BAC) = 30∘
Now, △ABD is a right triangle, right-angled at D with ∠BAD=30∘ and ∠ABD=60∘.
Suppose that AB=BC=CA=2a
Then, BD=12(BC)=a
and AD2=AB2−BD2=(2a)2−(a)2=3a2
Therefore, AD=a√3
sin30∘=BDAB=a2a=12
cos30∘=ADAB=a√32a=√32
tan30∘=BDAD=aa√3=1√3
cosec30∘=1sin30∘=2
sec30∘=1cos30∘=2√3
cot30∘=1tan30∘=√3
Similarly,
sin60∘=ADAB=a√32a=√32
cos60∘=12
tan60∘=√3
cosec60∘=2√3
sec60∘=2
cot60∘=1√3
Trigonometric Ratios of 0∘ and 90∘
In the right triangle ABC, ∠A gets smaller and smaller, then length of the side BC decreases to become zero and length of side AC becomes almost the same as AB then ∠A is 0∘.
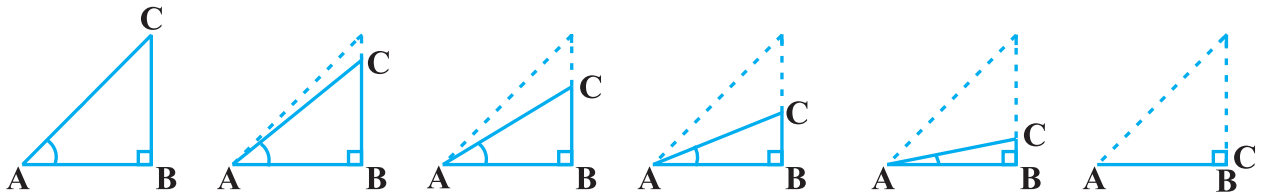
sin0∘=BCAC=BCAB=0
cos0∘=ACAB=ABAB=1
tan0∘=sin0∘cos0∘=0
cot0∘=1tan0∘=not defined
sec0∘=1cos0∘=1
cosec0∘=1sin0∘=not defined
Again ∠A gets larger and larger, then length of the side AB decreases to become zero and length of side AC becomes almost the same as BC then ∠A is 90∘
sin90∘=BCAC=BCBC=1
cos90∘=ABAC=0
tan90∘=sin90∘cos90∘=not defined
cot90∘=1tan90∘=0
sec90∘=1cos90∘=not defined
cosec90∘=1sin90∘=1
Ab in sabhi trigonometric ratios ko hum tabular form mein ek sath likhte hai. 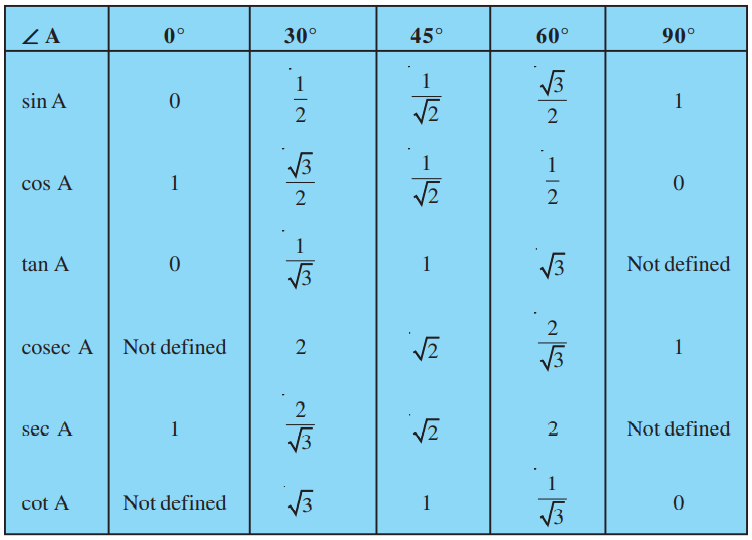
Note : ∠A increases from 0∘ to 90∘, sinA increases from 0 to 1 and cosA decreases from 1 to 0. Similarly, tanA and secA increases and cosecA and cotA decreases.
Example 3
Taking θ=30∘, verify each of the following
(i) sin3θ=3sinθ−4sin3θ
(ii) cos3θ=4cos3θ−3cosθ
When θ=30∘, we have 3θ=90∘.
(i) sin3θ=sin90∘=1
And, 3sinθ−4sin3θ=3sin30∘−4sin330∘
[3×12−4×(12)3]=(32−4×18)
=(32−12)=1.
∴sin3θ=3sinθ−4sin3θ.
(ii) cos3θ=cos90∘=0.
And, 4cos3θ−3cosθ=4cos330∘−3cos30∘
=⎡⎣4×(√32)3−3×√32⎤⎦
=(4×3√38−3√32)
=(3√32−3√32)=0.
∴cos3θ=4cos3θ−3cosθ.
Example 4
If sin(A−B)=12,cos(A+B)=12,
0∘<(A+B)≤90∘, A>B, find A and B.
Since, sin(A−B)=12
therefore, A−B=30∘ →(1)
(because sin ki value 12 kab hoti hai sin30∘ pe to agar hum compare kare to A−B=30∘)
Also, cos(A+B)=12, therefore, A+B=60∘ →(2)
(because cos ki value 12 kab hoti hai cos60∘ pe to agar hum compare kare to A−B=60∘)
Solving (1) and (2), we get : A=45∘ and B=15∘
Example 5
If tan(A+B)=√3 and tan(A−B)=1√3;0∘≤A+B≤90∘ and A≤B,find A and B.
Here, tan(A+B)=√3=tan60∘
So, A+B=60∘→(1)
tan(A−B)=1√3=tan30∘
So, A−B=30∘→(2)
Adding (1) and (2),
2A=90
⇒A=45∘
Putting value of A in (1),
B=60−45=15∘.
Trigonometric Ratios of Complementary Angles
In △ABC, right-angled at B, 
To dekho triangles ke teeno angles ka sum kya hota hai =180∘
∠A+∠B+∠C=180∘
∠A+90∘+∠C=180∘ (Since ∠B is right angle)
∠C=90∘−∠A
To ek baar hum ∠A ke liye trigonometric ratios define karte hai
sinA=BCAC, cosA=ABAC, tanA=BCAB, cosecA=ACBC, secA=ACAB, cotA=ABBC
To agar hum ∠C ke liye trigonometric ratios likhe to hum kya karenge ∠A ko replace kar denge ∠C se since (∠C=90∘−∠A) then we get,
sin(90∘−A)=ABAC=cosA
cos(90∘−A)=BCAC=sinA
tan(90∘−A)=ABBC=cotA
cot(90∘−A)=BCAB=tanA
sec(90∘−A)=ACBC=cosecA
cosec(90∘−A)=ACAB=secA
Therefore sin and cos are complementary function to each other, tan and cot are complementary function to each other and cosec and sec are complementary function to each other.
Example 6
Without using trigonometric tables, evaluate :
(i) cos53∘sin37∘
(ii) tan68∘cot22∘
(iii) sec49∘cosec41∘
(i) cos53∘sin37∘=cos(90∘−37∘)sin37∘=sin37∘sin37∘=1
[becausecos(90∘−θ)=sinθ].
(ii) tan68∘cot22∘=tan(90∘−22∘)cot22∘=cot22∘cot22∘=cot22∘cot22∘=1
[becausetan(90∘−θ)=cotθ]
(iii) sec49∘cosec41∘=sec(90∘−41∘)cosec 41∘=cosec41∘cosec41∘=1
[becausesec(90∘−θ)=cosecθ].
Trigonometric Identities
Bachcho agar apko yaad ho ki, an equation is called an identity when it is true for all values of the variables involved. Jaise ki aapne algebraic identity padhi hai 9th class mein, vse hi koi bhi equation jisme kisi angle ke trigonometric ratios involve ho wo trigonometric identity kahlati hai, if it is true for all values of the angles involved.
In △ABC, right-angled at B, we have:
By Pythagoras theorem
AB2+BC2=AC2 ...(1)
Dividing each term of (1) by AC2, we get
AB2AC2+BC2AC2=AC2AC2
(ABAC)2+(BCAC)2=(ACAC)2
(cosA)2+(sinA)2=1
cos2A+sin2A=1 ...(2)
Now on dividing each term of (2) by cos2A we get
cos2Acos2A+sin2Acos2A=1cos2A
1+tan2A=sec2A ...(3)
Now on dividing each term of (2) by sin2A we get
cos2Asin2A+sin2Asin2A=1sin2A
cot2A+1=cosec2A ...(4)
Result (2), (3), and (4) are important result we can use to simplify our identity and also used to determine the values of other trigonometric ratios in terms of known ratio.
Example 7
Prove that
sinθ−cosθ+1sinθ+cosθ−1=1(secθ−tanθ).
LHS =sinθ−cosθ+1sinθ+cosθ−1
=sinθcosθ−1+1cosθsinθcosθ+1−1cosθ
[ on dividing num. and denom. by cosθ ]
=tanθ−1+secθtanθ+1−secθ=(secθ+tanθ−1)(tanθ−secθ+1)
=(secθ+tanθ)−(sec2θ−tan2θ)(tanθ−secθ+1) [because(1=sec2θ−tan2θ)]
=(secθ+tanθ)[1−(secθ−tanθ)](tanθ−secθ+1)
=(secθ+tanθ)(tanθ−secθ+1)(tanθ−secθ+1)=(secθ+tanθ).
RHS=1(secθ−tanθ)
=1(secθ−tanθ)×(secθ+tanθ)(secθ+tanθ)=(secθ+tanθ)(sec2θ−tan2θ)
=(secθ+tanθ) [because(sec2θ−tan2θ=1)].
Hence, LHS=RHS.
Example 8
Prove that
(i) (1−sin2θ)sec2θ=1
(ii) (1+tan2θ)cos2θ=1
(iii) (1+tan2θ)(1−sinθ)(1+sinθ)=1
(iv) 2cos2θ+2(1+cot2θ)=1
We have
(i) LHS = (1−sin2θ)sec2θ
=cos2θ⋅sec2θ [because(1−sin2θ=cos2θ)]
=cos2θ⋅1cos2θ=1=RHS.
∴ LHS = RHS.
(ii) LHS =(1+tan2θ)cos2θ
=sec2θ⋅cos2θ [because(1+tan2θ=sec2θ)]
=1cos2θ⋅cos2θ=1=RHS.
∴ LHS = RHS.
(iii) LHS = (1+tan2θ)(1−sinθ)(1+sinθ)
=(1+tan2θ)(1−sin2θ)=sec2θ⋅cos2θ
[because(1+tan2θ)=sec2θ,(1−sin2θ)=cos2θ]
=1cos2θ⋅cos2θ [because(sec2θ=1cos2θ)]
=1=RHS.
∴ LHS = RHS.
(iv) LHS = 2cos2θ+2(1+cot2θ)
=2cos2θ+2cosec2θ [because(1+cot2θ=cosec2θ)]
=2cos2θ+2sin2θ [because(1cosec2θ=sin2θ)]
=2(cos2θ+sin2θ)=2×1 [because(cos2θ+sin2θ=1)]
=2=RHS.
∴ LHS = RHS.
Summary
1. In a right triangle ABC, right-angled at B,
sinA= side opposite to angle A hypotenuse
cosA= side adjacent to angle A hypotenuse
tanA= side opposite to angle A side adjacent to angle A
2. cosecA=1sinA;secA=1cosA;tanA=1cotA,tanA=sinAcosA
3. The value of sinA or cosA never exceeds 1, whereas the value of secA or cosecA is always greater than or equal to 1.
4. sin(90∘−A)=cosA
cos(90∘−A)=sinA
tan(90∘−A)=cotA
cot(90∘−A)=tanA
sec(90∘−A)=cosecA
cosec(90∘−A)=secA
5. cos2A+sin2A=1
1+tan2A=sec2A
cot2A+1=cosec2A
Let's Revise
is the study of relationships between the sides and angles of a triangle.
Ratios of the sides of a right triangle with respect to its acute angles.
Suppose karo ki humarey paas ek right angle triangle hai ABC, right angle at B, aur ∠CAB acute angle hai.
AB adjacent side (Base) aur BC opposite side (Perpendicular) hai, ∠A ke liye. To hume trigonometric ratios kuch es tarh se milte hai,
(i) sine of ∠A or sin A=perpendicularhypotenuse=BCAC
(ii) cosine of ∠A or cos A = basehypotenuse =
ABAC
(iii) tangent of ∠A or tan A
= perpendicularbase
=BCAB
(iv) cosecant
of ∠A or cosec A = 1sine of angleA = hypotenuseperpendicular = ACBC
(v) secant of ∠A or sec A = 1cosec of angle A = hypotenusebase =
ACAB
(vi) cotangent of ∠A or cot A =
1tangent of angleA
= baseperpendicular =
ABBC
Similarly, hum ∠C ke liye bhi trigonometric ratios nikal saktey hai lekin angle C ke liye perpendicular AB hoga aur base BC hoga.
- The values of the trigonometric ratios of an angle do not vary with the lengths of the sides of the triangle, if the angle remains the same.
- Trigonometric Ratios of Some Specific Angles:

The value of sin θ and cos θ can never exceed 1. Adjacent side can never be greater than hypotenuse since hypotenuse is the longest side in a right-angled ∆.
- Trigonometric Ratios for Complementary Angles:
If ΔABC is a right-angled triangle, right-angled at B, then ∠A+∠C=90° [∵ ∠A+∠B+∠C=180° angle-sum-property] or ∠C=(90°–∠A). Yha ∠A aur ∠C complementary angles hai aur in relationships ko satisfy kartey hai,
(1) sin C = sin (90° - A) = cos A
(ii) cosec C = cosec (90° – A) = sec A
(iii) cos C = cos (90° – A) = sin A
(iv) sec C = sec (90° – A) = cosec A
(v) tan C = tan (90° – A) = cot A
(vi) cot C = cot (90° – A) = tan A
- Trigonometric Identities:
An equation involving trigonometric ratios of an angle is called a trigonometric identity, if it is true for all values of the angle(s) involved. These are
tanθ=sinθcosθ
cotθ=cosθsinθ
(i) cos2A+sin2A = 1
(ii) 1+tan2A=sec2A (for 0° ≤ A < 90°)
(iii) cot2A+1=cosec2A (for 0° < A ≤ 90º)
