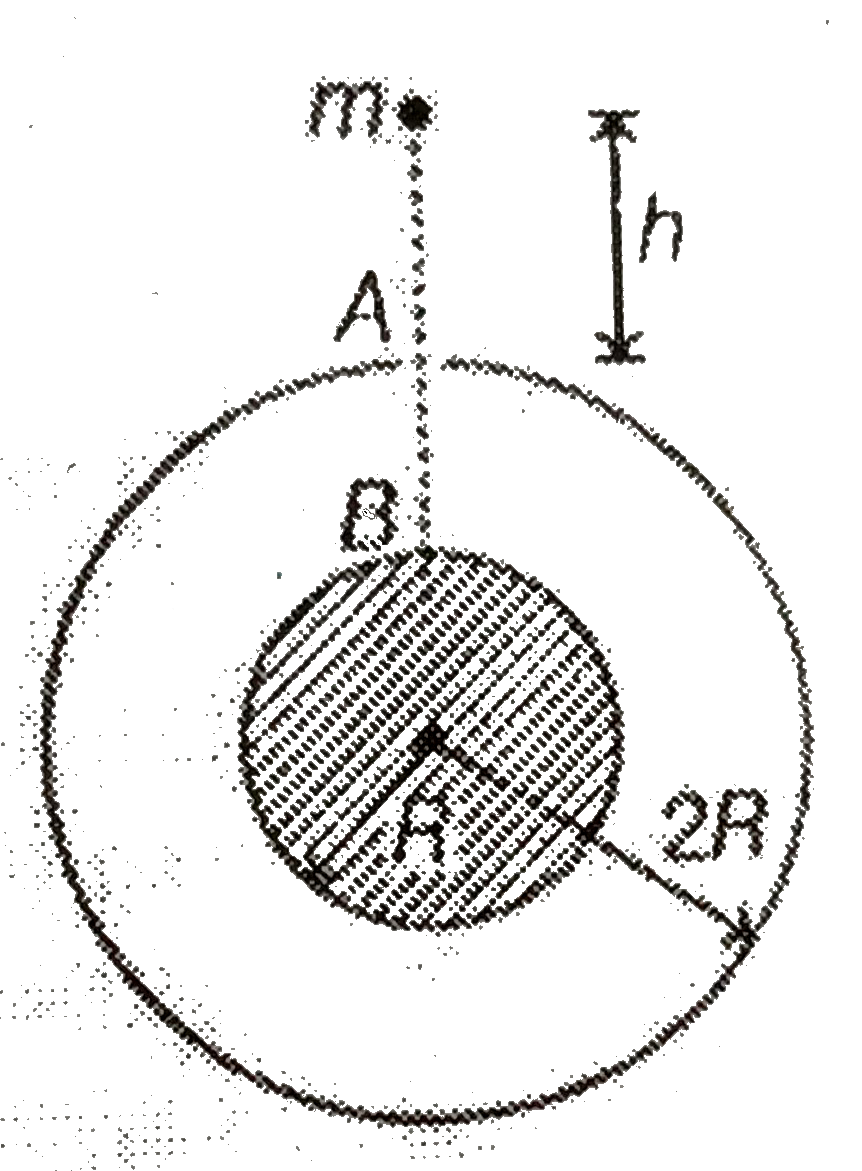D: Matrix Matching Type Questions from DC PANDEY PHYSICS (HINGLISH) GRAVITATION for Class 12
| Class 12 | PHYSICS |
| Chapter | GRAVITATION |
| DC PANDEY PHYSICS (HINGLISH) | D: Matrix Matching Type Questions |
| Medium | English |
| Academic Year | 2023-2024 |
Question 50
Imagine a light planet revolving around a very massive star in a circular orbit of radius R with a period of revolution T. if the gravitational force of attraction between the planet and the star is proportational to R−5/2, then
(a) T2 is proportional to R2
(b) T2 is proportional to R7/2
(c) T2 is proportional to R3/3
(d) T2 is proportional to R3.75.View SolutionQuestion 56
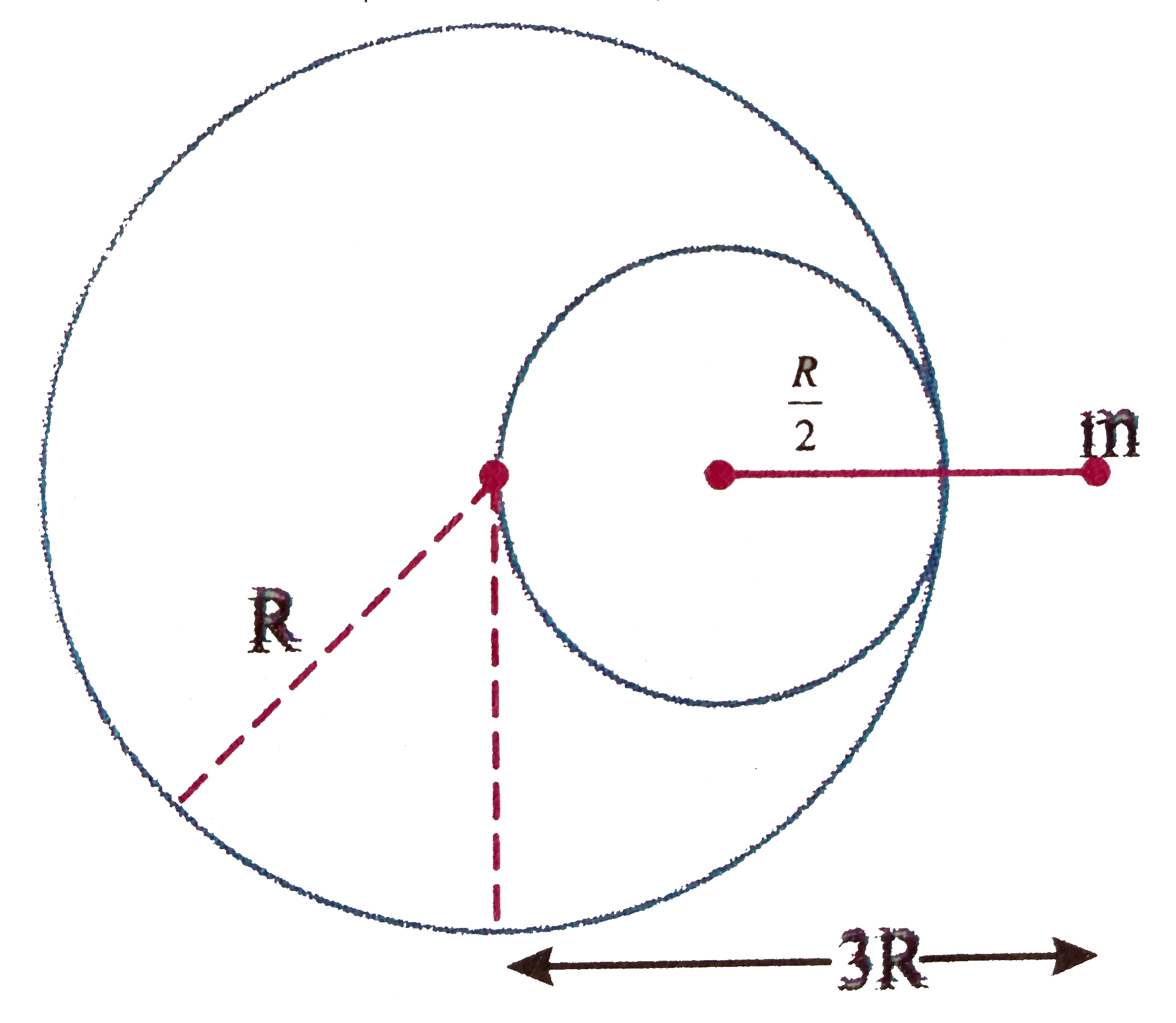
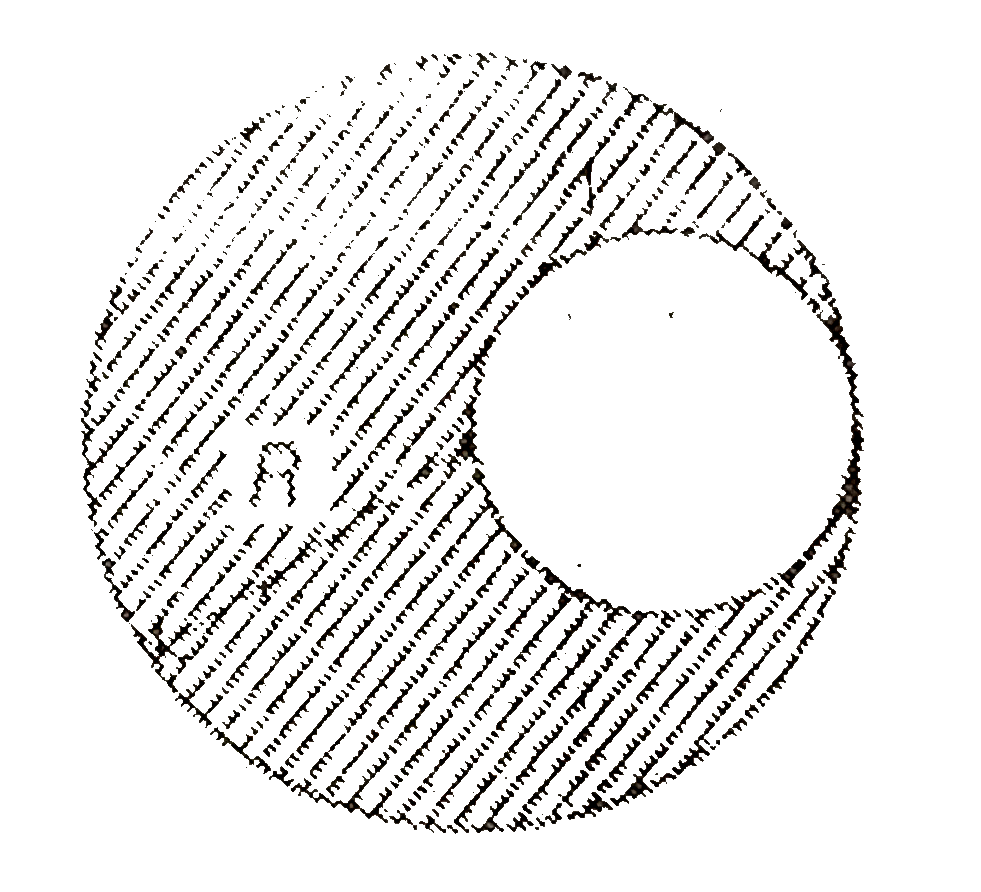
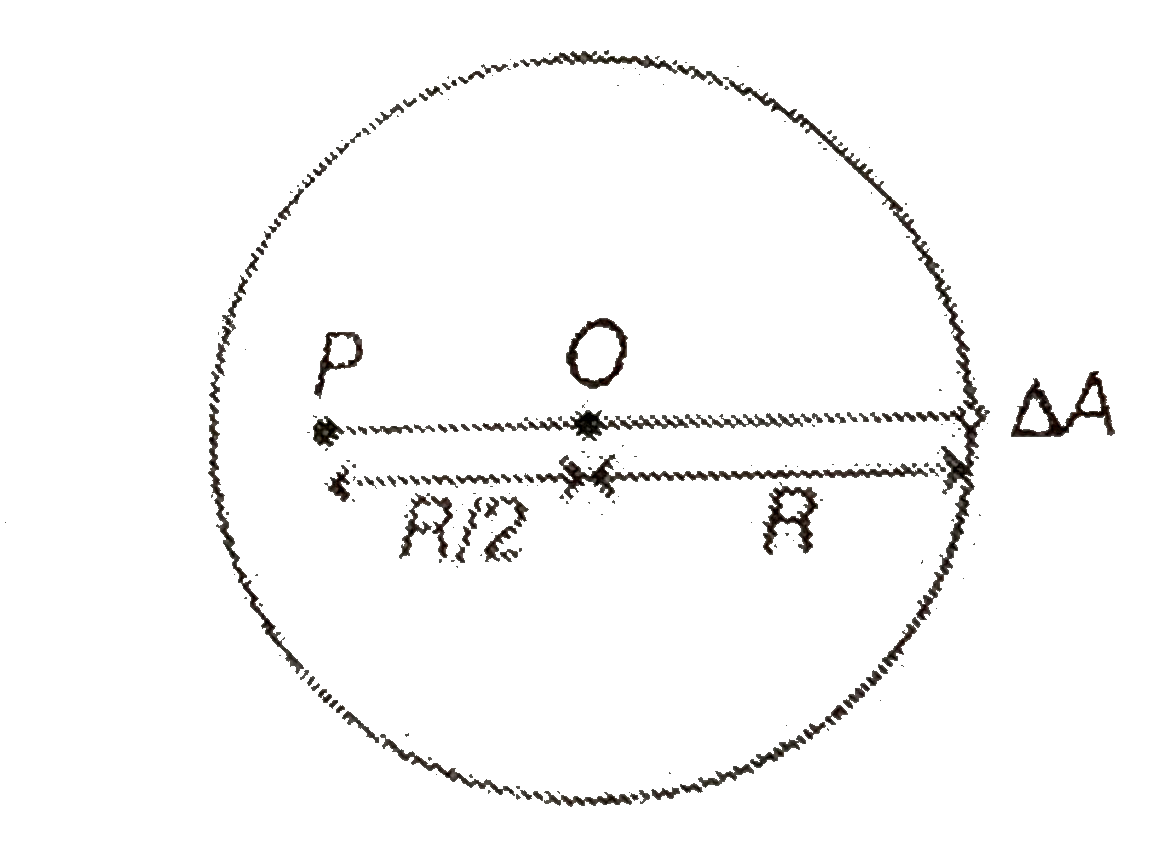
The gravitational force acting on a particle, due to a solid sphere of uniform density and radius R, at a distance of 3R from the centre of the sphere is F1. A spherical hole of radius (R/2) is now made in the sphere as shown in diagram. The sphere with hole now exerts a force F2 on the same particle. ratio of F1 to F2 is
 View Solution
View SolutionQuestion 60
One ring of radius R and mass m and one solid sphere of same mass m and same radius R are placed with their centres on positive x-axis. We are moving from some finite distance on negative x-axis towards positive x-axis plane of the ring is perpendicular to x-axis. How will the net gravitational field vary with distance moved on x-axis We move only up to surface of solid sphere. O is the origin.
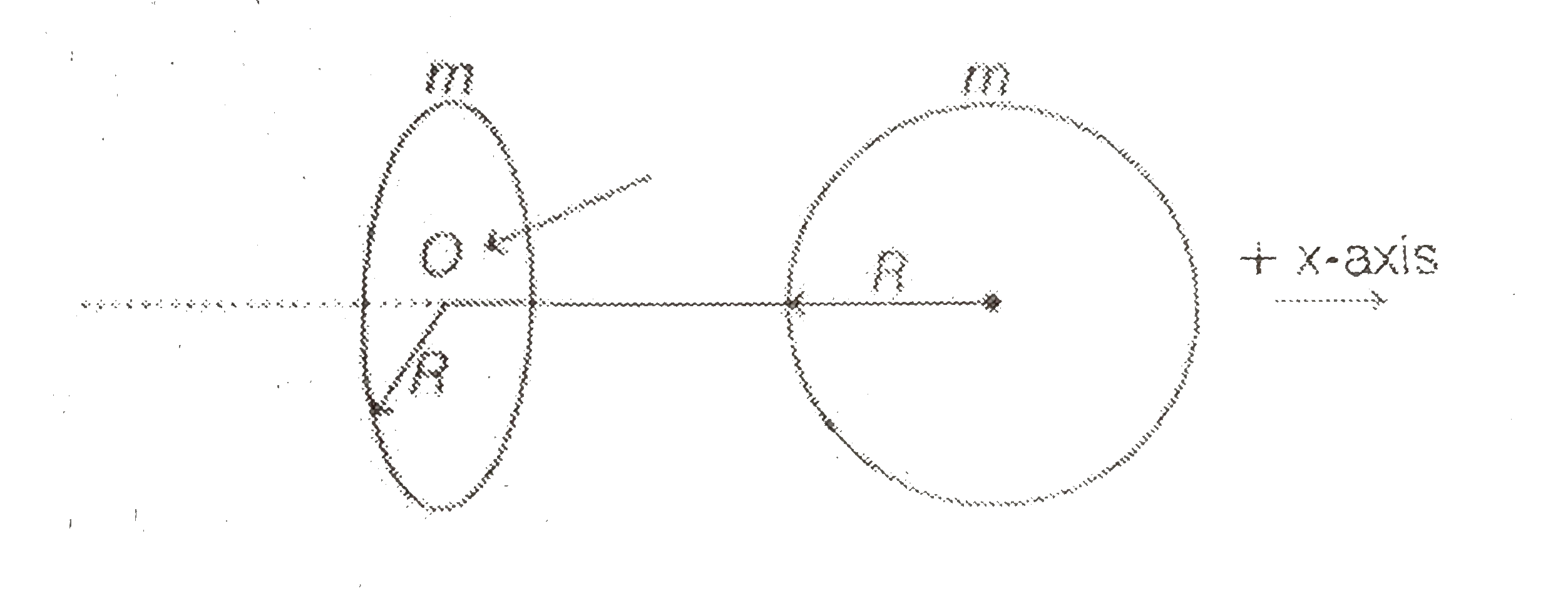 View Solution
View SolutionQuestion 67
A particle is projected from the surface of earth with velocity equal to its escape velocity , at 45∘ with horizontal . What is the angle of its velocity with horizontal at height h = R . (Here horizontal at some point means a line parallel to tangent on earth just below that point . ) View SolutionQuestion 69
The gravitational potential of two homogeneous spherical shells A and B of same surface density at their respective centres are in the ratio 3:4. If the two shells collapse into a single one such that surface charge density remains the same, then the ratio of potential at an internal point of the new shell to shell A is equal to View SolutionQuestion 71
A projectile is launched from the surface of the earth with a very high speed u at an angle θ with vertical . What is its velocity when it is at the farthest distance from the earth surface . Given that the maximum height reached when it is launched vertically from the earth with a velocity v = √GMR View SolutionQuestion 73
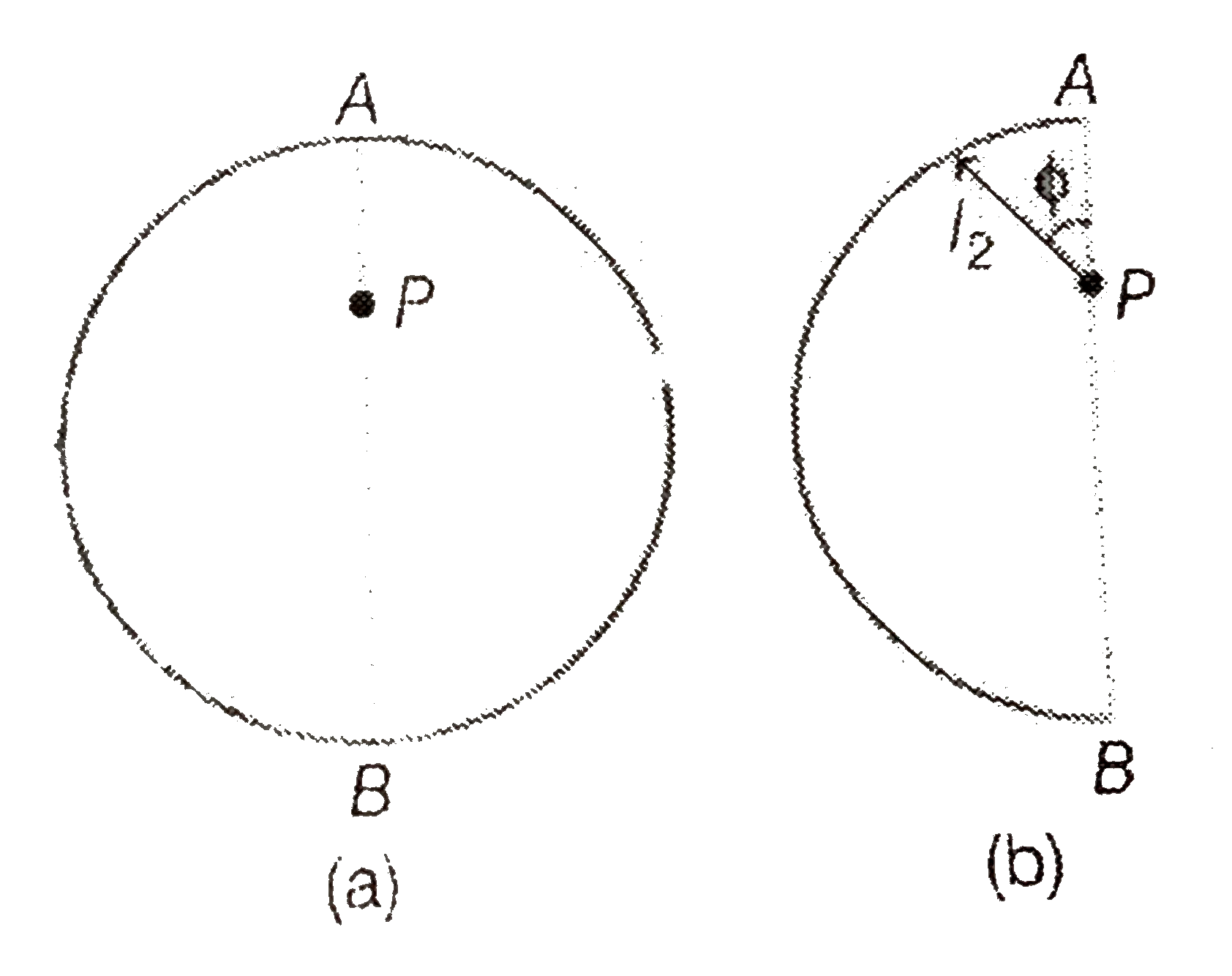
Figure (a) shows a ring of mass m and figure (b) shows half portion of the same ring . Gravitational field at point P in figure (b) is I2 in magnitude and makes an angle ϕ with line . AB. Gravitational field at point P in figure (a) is I1 in magnitude . The ratio of I12I2 is
 View Solution
View SolutionQuestion 76
A solid sphere of mass M and radius R has a spherical cavity of radius R/2 such that the centre of cavity is at a distance R/2 from the centre of the sphere. A point mass m is placed inside the cavity at a distance R/4 from the centre of sphere. The gravitational force on mass m isView SolutionQuestion 78
A binary star system is revolving in a circular path with angular speed 'ω' and mass of the stars are 'm' and '4m' respectively. Both stars stop suddenly, then the speed of havirer star when the separation between the stars when the seperation between the stars becomes half of initial value is :View SolutionQuestion 80
Two smooth tunnels are dug from one side of earth's surface to the other side, one along a diameter are dropped from one end of each of the tunnels. Both time particles oscillate simple harmonically along the tunnels. Let T1 and T2 be the particle along the diameter and along the chord respectively. Then: View SolutionQuestion 90
A tunnel is dug along a chord of the earth at a perpendicular distance R/2 from the earth's centre. The wall of the tunnel may be assumed to be frictionless. A particle is released from one end of the tunnel. The pressing force by the particle on the wall, and the acceleration of the particle vary with x (distance of the particle from the centre) according to View SolutionQuestion 92
A light satellite is initially rotating around a planet in a circular orbit of radius r. Its speed in this circular orbit was u0. It is put in an elliptical orbit by increasing its speed form u0 to vQ ( instantaneously). In the elliptical orbit, the satellite reaches the farthest point P, which is at a distance R from the planet. Satellite's speed at farthest point is vP. At point Q, the speed required by satellite to escape the planet's gravitational pull is vesc
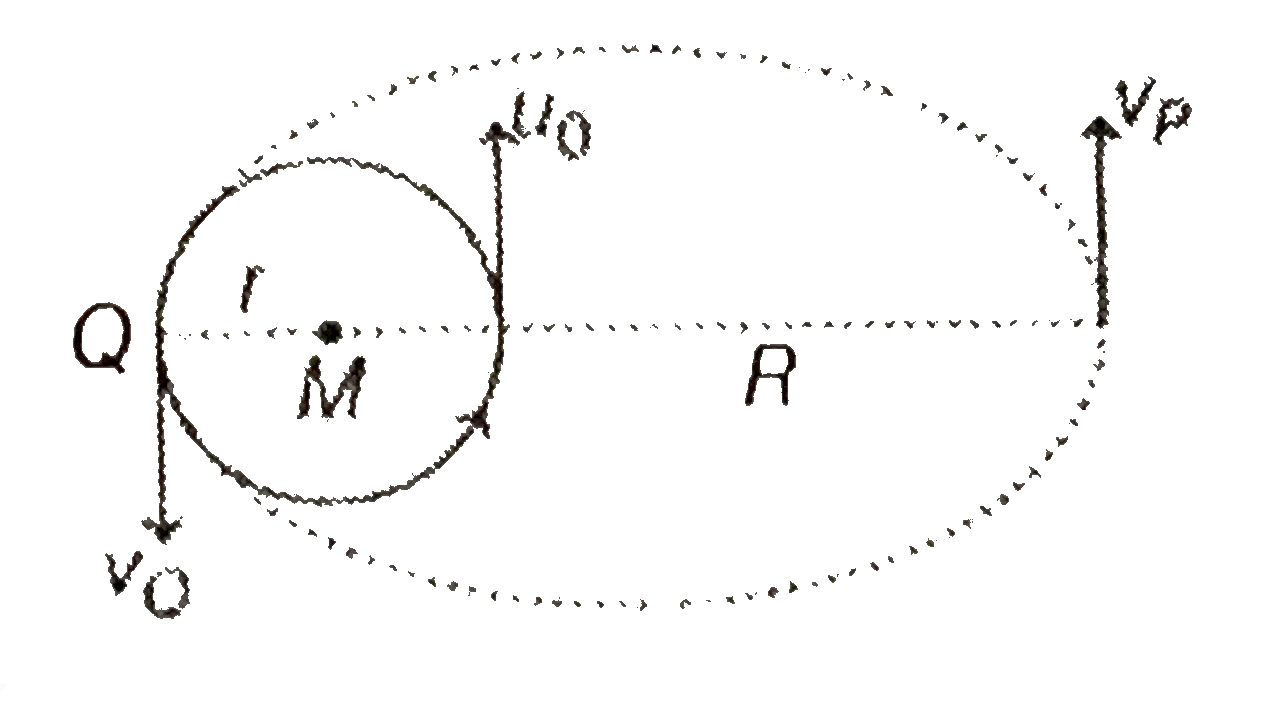 View Solution
View SolutionQuestion 96
When a particle is projected from the surface of earth its mechanical energy and angular momentum about center of earth at all time are constant.
Q. A particle of mass m is projected from the surface of earth with velocity v0 at angle θ with horizontal suppose h be the maximum height of particle from surface of earth and v its speed at that point then v is v0cosθView SolutionQuestion 101
A planet is revolving round the sun is elliptical orbit. Velocity at perigee position ( nearest) is v1 and at apogee position ( farthest) is v2. Both these velocities are perpendicular to the line joining centre of sun and planet. r1 is the minimum distance and r2 the maximum distance.
When the planet is at perigee position, it wants to revolve in a circular orbit by itselff. For this, value of GView SolutionQuestion 102
A planet is revolving round the sun is elliptical orbit. Velocity at perigee position ( nearest) is v1 and at apogee position ( farthest) is v2. Both these velocities are perpendicular to the line joining centre of sun and planet. r1 is the minimum distance and r2 the maximum distance.
At apogee position suppose speed of planet is slightly decreased from v2, then what will happen to minimum distance r1 and maximum distance r2 in the subsequent motion.View SolutionQuestion 106
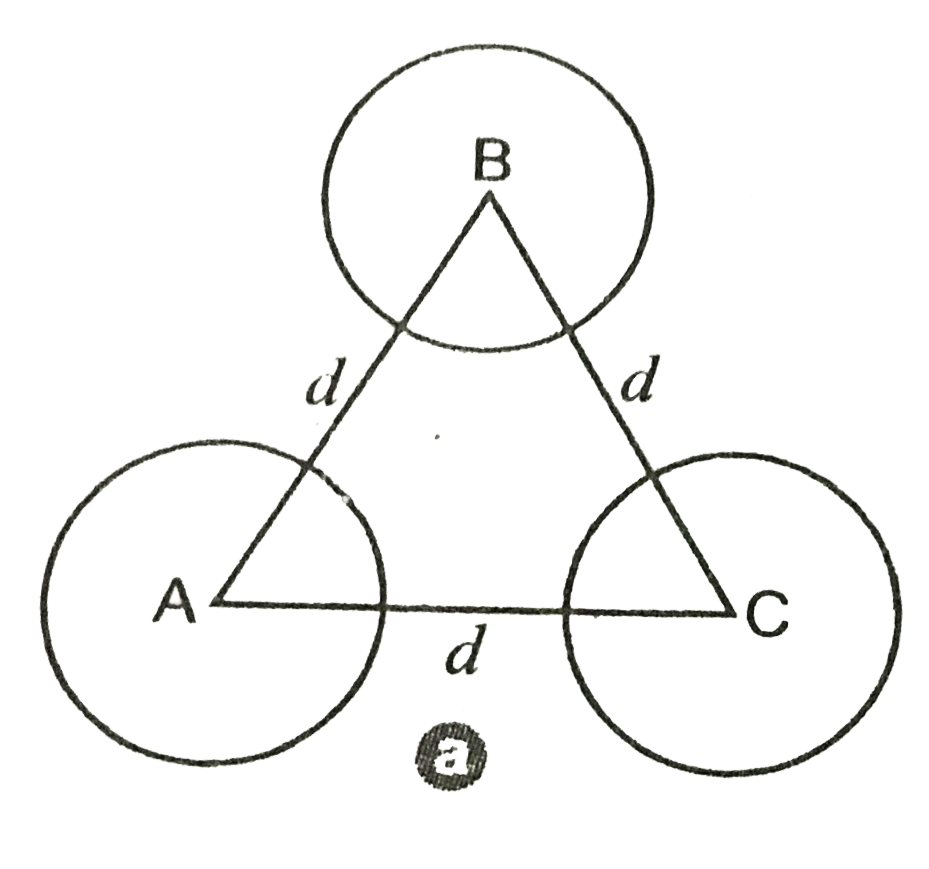
Three equal masses each of mass 'm' are placed at the three-corner of an equilateral triangle of side 'a'
In the above system, if two particles are kept fixed and third particle is released, then speed of the particle when it reaches to the mid point of the side connectiong other two masses isView SolutionQuestion 107
An artificial satellite is moving in circular orbit around the earth with speed equal to half the magnitude of escape velocity from the surface of earth. R is the radius of earth and g is acceleration due to gravity at the surface of earth (R=6400km)
Then the distance of satelite from the surface of earth is .View SolutionQuestion 108
An artificial satellite is moving in a circular orbit around the earth with a speed equal to half the magnitude of escape velocity from the surface of earth. R is the radius of earth and g is acceleration due to gravity at the surface of earth. (R=6400 km).
The time period of revolution of satellite in the given orbit is View SolutionQuestion 109
An artificial satellite is moving in a circular orbit around the earth with a speed equal to half the magnitude of escape velocity from the earth.
(i) Determine the height of the satellite above the earth's surface.
(ii) If the satellite is stopped suddenly in its orbit and allowed to fall freely onto the earth, find the speed with which it hits the surface of the earth.View SolutionQuestion 110
A pair of stars rotates about their centre of mass One of the stars has a mass M and the other has mass m such that M=2m The distance between the centres of the stars is d (d being large compared to the size of either star) .
The period of rotation of the stars about their common centre of mass (in time of d ,m ,G) is .View SolutionQuestion 111
A pair of stars rotates about their centre of mass One of the stars has a mass M and the other has mass m such that M=2m The distance between the centres of the stars is d (d being large compared to the size of either star) .
The ratio of the angular momentum of the two stars about their common centre of mass (Lm/Lm) is .View SolutionQuestion 112
A pair of stars rotates about their centre of mass One of the stars has a mass M and the other has mass m such that M=2m The distance between the centres of the stars is d (d being large compared to the size of either star) .
The ratio of kinetic energies of the two stars (Km/KM) is .View SolutionQuestion 114
Consider a spherical planet of radius R . Its density varies with the distance of its centre t as ρ=A−Br, where A and B are positive constants. Now answer the following questions.
For B=0 , pressure due to gravitation force at a distance r(<R) from the centre of the planet is View SolutionQuestion 116
A particle is projected from the surface of earth of mass M and radius R with speed v. Suppose it travels a distance x(<<R) when its speed becomes v to v/2 and y(<<R) when speed changes from v/2 to 0. Similarly, the corresponding times are suppose t1andt2. Then
Column-IColumn-II(A)x/y(p)=1(B)t1/t2(r)>1(r)<1View SolutionQuestion 118
In elliptical orbit of a planet, as the planet moves from apogee position to perigee position to perigee position, match the following columns
Column-IColumn-II(A)Speed of planet(p)Remains same(B)Distance of planet from centre of sun(q)Decreases(C)Potential energy(r)Increase(D)Angular momentum about centre of sun(s)Cannot sayView SolutionQuestion 120
Let V and E denote the gravitational potential and gravitational field at a point. Then, match the following columns
Column-IColumn-II(A)E=0,V=0(p)At centre of spherical shell(B)E≠0,V=0(q)At centre of solid sphere(C)V≠0,E=0(r)At centre of circular ring(D)V≠0,E≠0(s)At cetre of two point masses of equal magnitude(t)NoneView SolutionQuestion 121
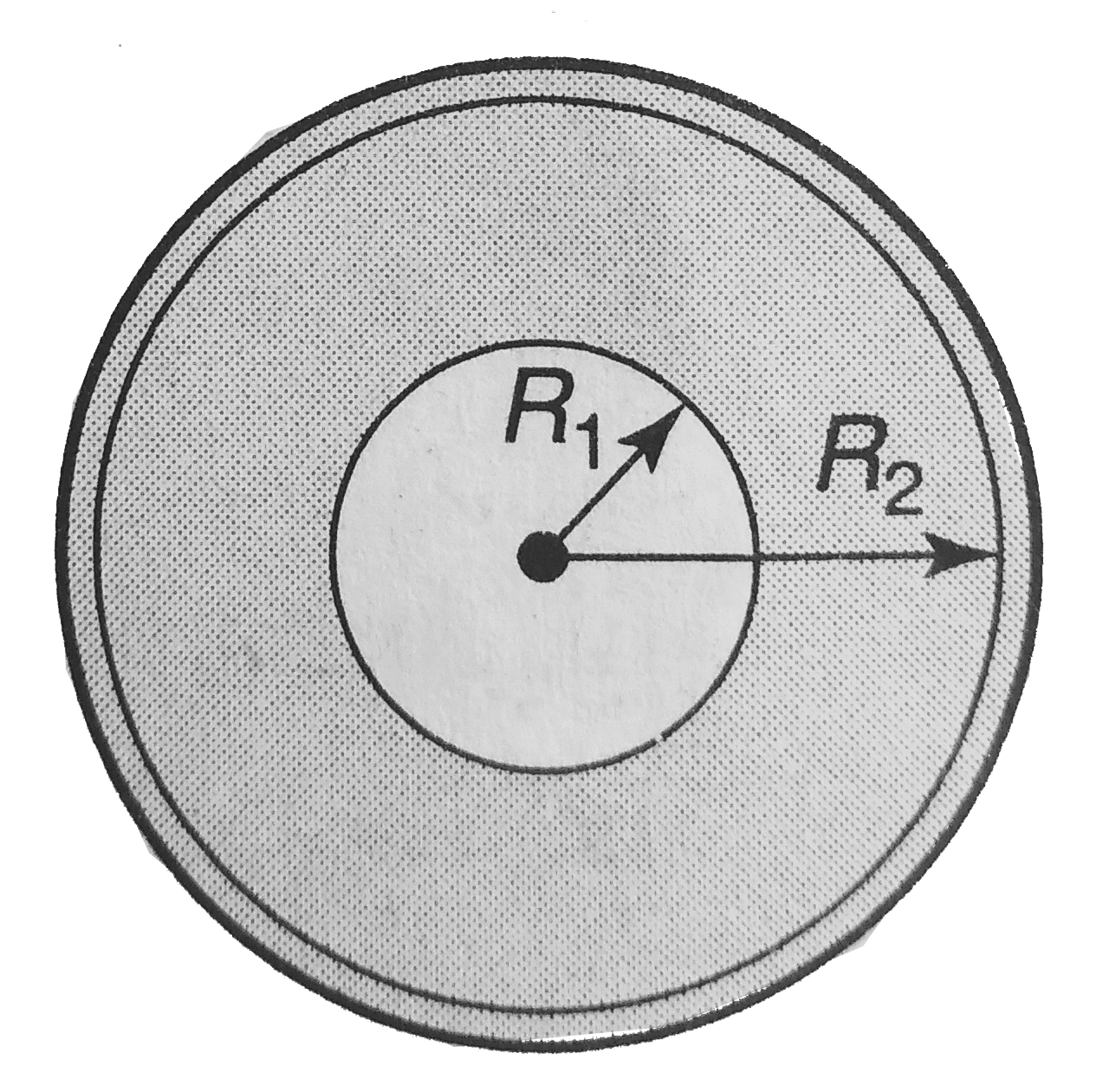
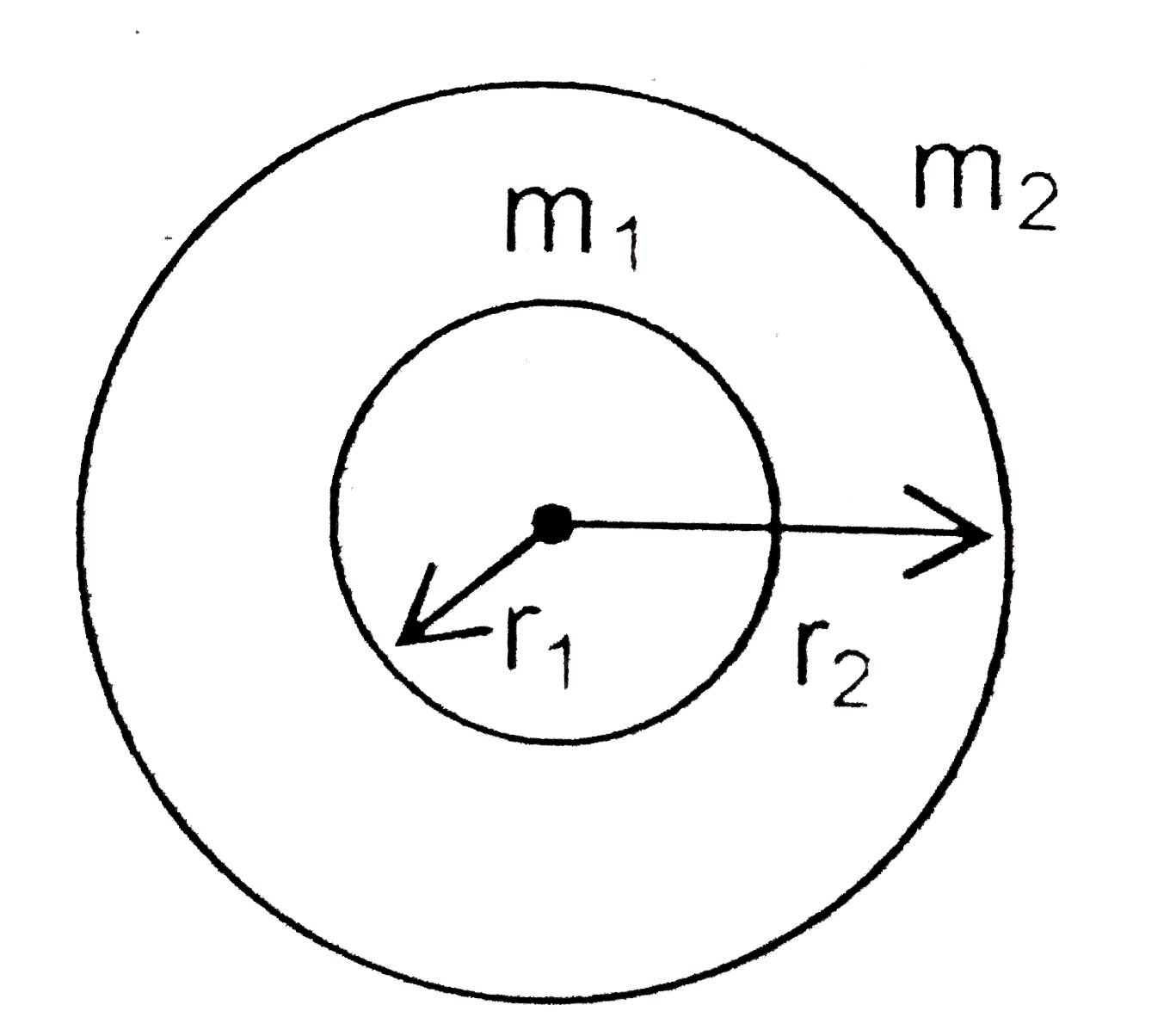
Two concentric spherical shells are as shown in figure. Match the following columns
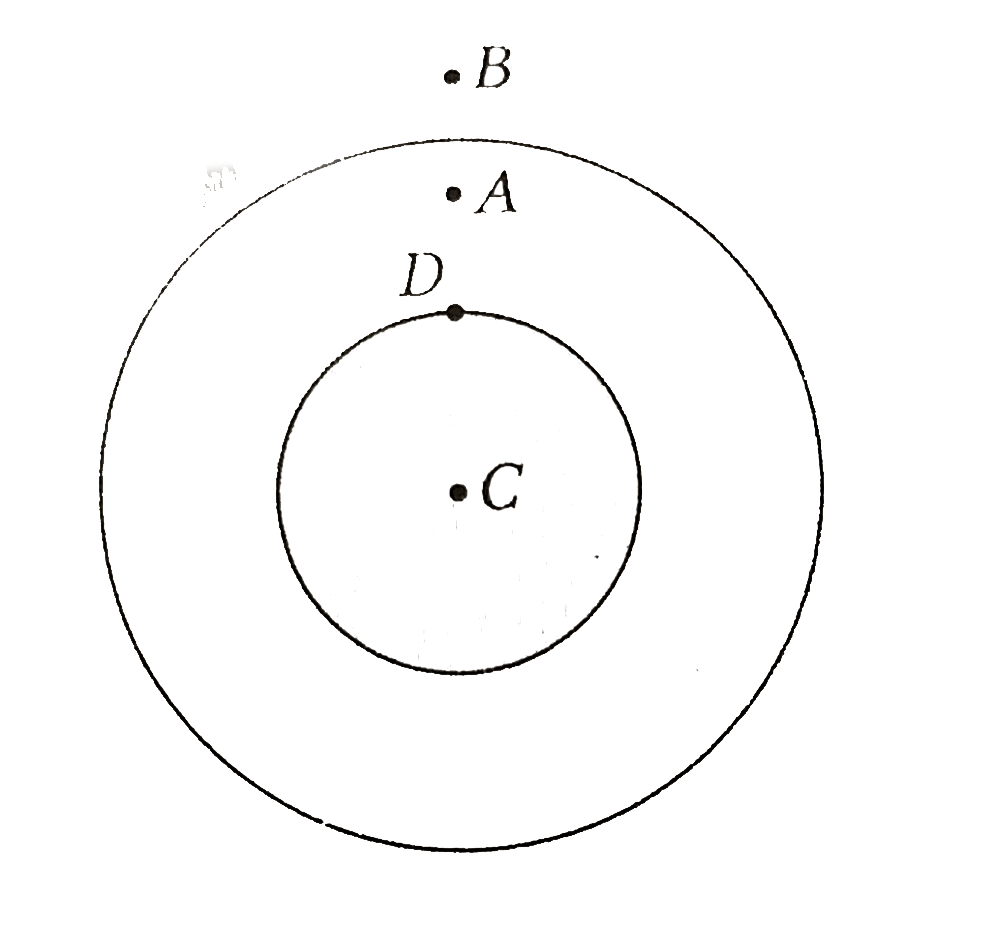
Column-IColumn-II(A)Potential at A(p)greater than B(B)Gravitational field at A(q)less than B(C)As one moves from C to D(r)Potential remains constant(D)As one moves from D to A(s)Gravitational field decreases(t)NoneView Solution


_E01_035_Q01.png)
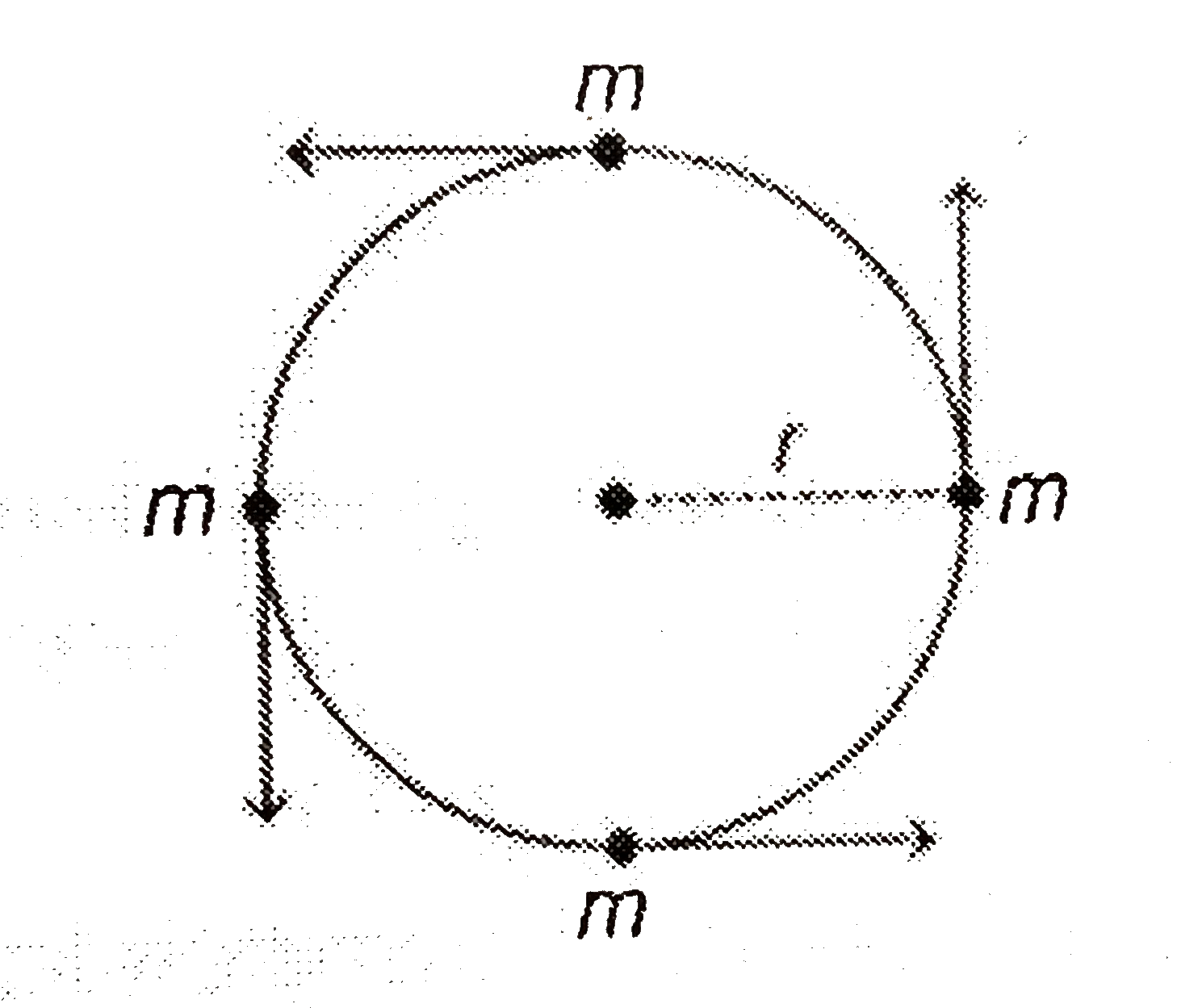


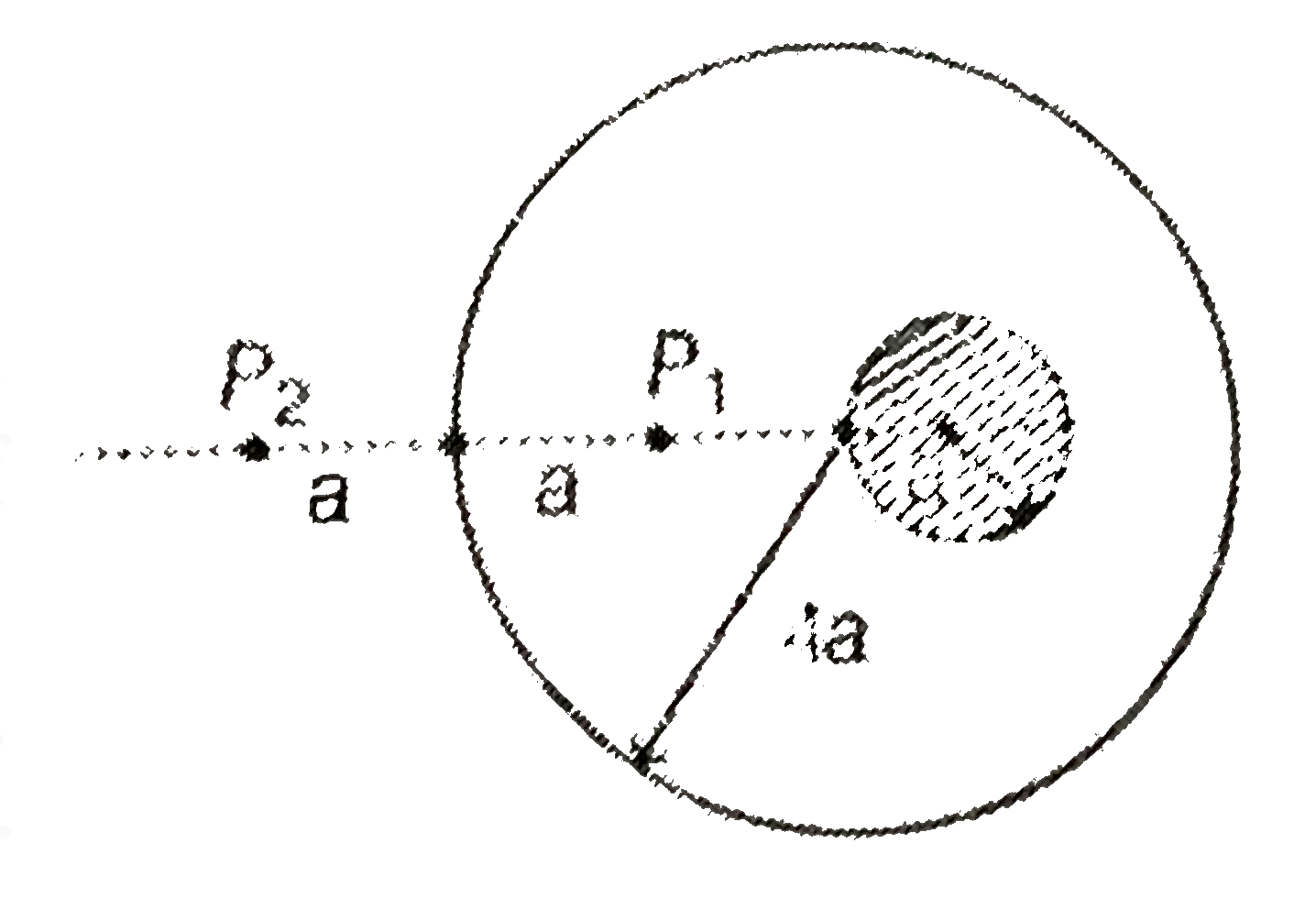
_S01_036_Q01.png)