PROBLEMS from RESNICK AND HALLIDAY PHYSICS (HINGLISH) FLUIDS for Class 12
| Class 12 | PHYSICS |
| Chapter | FLUIDS |
| RESNICK AND HALLIDAY PHYSICS (HINGLISH) | PROBLEMS |
| Medium | English |
| Academic Year | 2023-2024 |
Question 1
Giraffe bending to drink. In a giraffe with its head 1.8 m above its heart, and its heart 2.0 m above its feet, the (hydrostatic) gauge pressure in the blood at its heart is 250 torr. Assume that the giraffe stands upright and the blood density is 1.06×103kg/m3 . In torr (or mm Hg), find the (gauge) blood pressure (a) at the brain (the pressure is enough to perfuse the brain with blood, to keep the giraffe from fainting) and (b) at the feet (the pressure must be countered by tight-fitting skin acting like a pressure stocking). (c) If the giraffe were to lower its head to drink from a pond without splaying its legs and moving slowly, what would be the increase in the blood pressure in the brain? (Such action would probably be lethal.)View SolutionQuestion 2
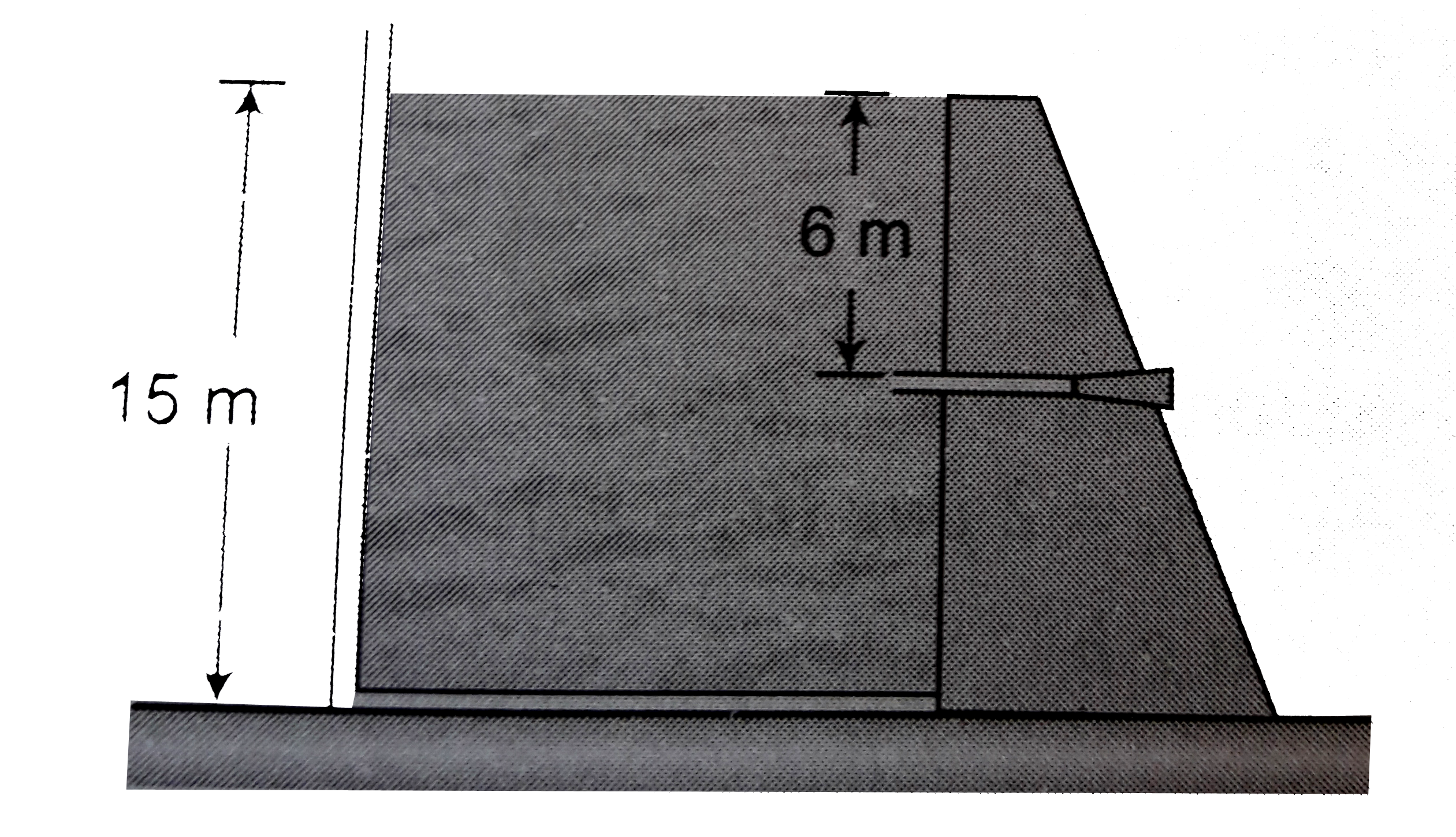
The fresh water behind a reservoir dam is 15m deep. A horizontal pipe 4.0cm in diameter passes through the dam 6.0m below the water surface as shown in the figure. A plug secures the pipe opening :
(a) Find the friction force between the plug and the pipe wall.
(b) The plug is removed. What volume of water flows out of the pipe in 3.0 hour ? Assume that reservoir is large.
 .View Solution
.View SolutionQuestion 3
In Fig ., the fresh water stands at depth D = 30.0 m behind the vertical upstream face of a dam of width W = 250 m. Find (a) the net horizontal force on the dam from the gauge pressure of the water and (b) the net torque due to that force about a horizontal line through O parallel to the (long) width of the dam. This torque tends to rotate the dam around that line, which would cause the dam to fail. (c) Find the moment arm of the torque.
 View Solution
View SolutionQuestion 4
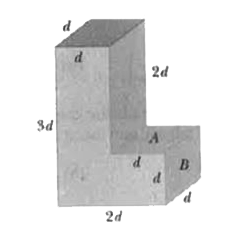
The volume of air space in the passenger compartment of an 1800 kg car is 5.00 m3 . The volume of the motor and front wheels is 0.710m3, and the volume of the rear wheels, gas tank, and trunk is 0.800m3, water cannot enter these two regions. The car rolls into a lake. (a) At first, no water enters the passenger compartment. How much of the car, in cubic meters, is below the water surface with the car floating (Fig. 14-45)? (b) As water slowly enters, the car sinks. How many cubic meters of water are in the car as it disappears below the water surface? (The car, with a heavy load in the trunk, remains horizontal.)
 View Solution
View SolutionQuestion 5
The maximum depth dmax that a diver can snorkel is set by the density of the water and the fact that human lungs can function against a maximum pressure difference between inside and outside the chest cavity) of 0.050 atm. What is the difference in dmax for fresh water and the water of the Dead Sea (the saltiest natural water in the world, with a density of 1.5x103kg/m3)?View SolutionQuestion 6
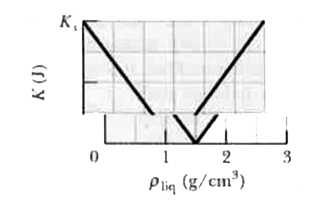
A small solid ball is released from rest while fully sub merged in a liquid and then its kinetic energy is measured when it has moved 2.0 cm in the liquid. Figure 14.46 gives the results after many liquids are used: The kinetic energy K is plotted versus the liquid density ρliq and Ks = 2.4 J sets the scale on the vertical axis. What are (a) the density and (b) the volume of the ball?
 View Solution
View SolutionQuestion 7
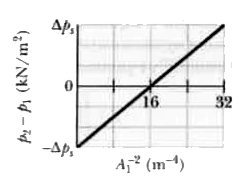
Fresh water flows horizontally from pipe section 1 of cross-sectional area A, into pipe section 2 of cross-sectional area A2 Figure gives a plot of the pressure difference p2−p1 versus the inverse area squared A−21 that would be expected for a volume flow rate of a certain value if the water flow were laminar under all circumstances. The scale on the vertical axis is set by Δps=600kN/m2. For the conditions of the figure, what are the values of (a) A2 and (b) the volume flow rate?
 View Solution
View SolutionQuestion 8
In Fig. water flows steadily from the left pipe section (radius r1 = 2.00R), through the middle section (radius R), and into the right section (radius r3 = 3.00R). The speed of the water in the middle section is 0.620 m/s. What is the net work done on 0.700m3 of the water as it moves from the left section to the right section?
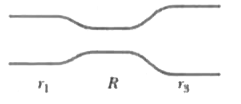 View Solution
View SolutionQuestion 10
A liquid of density 900 kg/m flows through a horizontal pipe that has a cross-sectional area of 1.90×10−2m2 in region A and a cross-sectional area of 9.50×10−2m2 in region B. The pressure difference between the two regions is 720×103 Pa. What are (a) the volume flow rate and (b) the mass flow rate? View SolutionQuestion 11
The plastic tube in Fig. has a cross-sectional area of 5.00 cm2. The tube is filled with water until the short arm (of length d = 0.800 m) is full. Then the short arm is figure sealed and more water is gradually poured into the long arm. When the total height of water in the long arm reaches 2.80 m, the seal is on the verging of popping. What force is then on the seal?
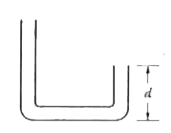 View Solution
View SolutionQuestion 12
When researchers find a reasonably complete fossil of a dinosaur, they can determine the mass and weight of the living dinosaur with a scale model sculpted from plastic and based on the dimensions of the fossil bones. The scale of the model is 1/20, that is, lengths are 1/20 actual length, areas are (1/20)2 actual areas, and volumes are (1/20)3 actual volumes. First, the model is suspended from One arm of a balance and weights are added to the other arm until equilibrium is reached. Then the model is fully submerged in water and enough weights are removed from the second arm to reestablish equilibrium (Fig. ). For a model of a particular T. rex fossil 791.10 g had to be removed to a reestablish equilibrium . what was the volume of (a) the model and (b) the actual T . rex ? (c) if the density of T.rex was approximately the density of water , what was its mass ?
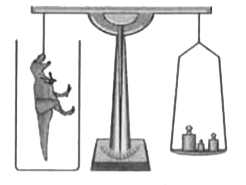 View Solution
View SolutionQuestion 15
A 3.00 kg object is released from rest while fully sub merged in a liquid. The liquid displaced by the submerged object has a mass of 5.00 kg. How far and in what direction does the object move in 0.200 s, assuming that it moves freely and that the drag force on it from the liquid is negligible?View SolutionQuestion 16
Blood pressure in Argentinosaurus. (a) If this long-necked, gigantic sauropod had a head height of 18 m and a heart height of 8.0 m, what (hydrostatic) gauge pressure in its blood was required at the heart such that the blood pressure at the brain was 80 torr (just enough to perfuse the brain with blood)? Assume the blood had a density of 1.06×10kg/m3. (b) What was the blood pressure (in torr or mm Hg) at the feet?View SolutionQuestion 17
A boat floating in fresh water displaces water weighing 50.1 KN. (a) What is the weight of the water this boat displaces when floating in salt water of density 1.10×103kg/m3 ? (b) What is the difference between the volume of fresh water displaced and the volume of salt water displaced? View SolutionQuestion 18
Snorkeling by humans and elephants. When a person snorkels, the lungs are connected directly to the atmosphere through the snorkel tube and thus are at atmospheric pressure. In atmospheres, what is the difference Δp between this internal air pressure and the water pressure against the body if the length of the snorkel tube is (a) 20 cm (standard situation) and (b) 3.5 m (probably lethal situation)? In the latter, the pressure difference causes blood vessels on the walls of the lungs to rupture, releasing blood into the lungs. As depicted in Fig. 14-52, an elephant can safely snorkel through its trunk while swimming with its lungs 3.5 m below the water surface because the membrane around its lungs contains connective tissue that holds and protects the blood vessels, preventing rupturing.
 View Solution
View SolutionQuestion 22
A piston of cross-sectional area a is used in a hydraulic press to exert a small force of magnitude f on the enclosed liquid. A connecting pipe leads to o a larger piston of cross sectional area A (Fig. 14-53). (a) What force magnitude F will the larger piston sustain without moving? (b) If the piston diameters are 3.50 cm and 60.0 cm, what force magnitude on the large piston will balance a 20.0 N force on the small piston?
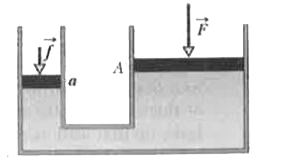 View Solution
View SolutionQuestion 25
Figure shows an iron ball suspended by thread of negligible mass from an upright cylinder that floats partially submerged in water. The cylinder has a height of 6.00 cm, a face area of 12.0cm2 on the top and bottom, and a density of 0.25g/cm2 , and 1.00 cm of its height is above the water surface. What is the radius of the iron ball?
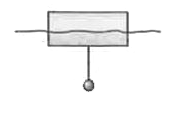 View Solution
View SolutionQuestion 26
A flotation device is in the shape of a right cylinder, with a height of 0.650 m and a face area of 4.00m2 on top and bottom, and its density is 0.300 times that of fresh water. It is initially held fully submerged in fresh water, with its top face at the water surface. Then it is allowed to ascend gradually until it begins to float. How much work does the buoyant force do on the device during the ascent?View SolutionQuestion 27
Suppose that two tanks, 1 and 2, each with a large opening at the top, contain different liquids. A small hole is made in the side of each tank at the same depth h below the liquid surface, but the hole in tank 1 has half the cross-sectional area of the hole in tank 2. (a) What is the ratio ρ1/ρ2 of the densities of the liquids if the mass flow rate is the same for the two holes? (b) What is the ratio Rv1/Rv2 of the volume flow rates from the two tanks? (c) At one instant, the liquid in tank 1 is 16.0 cm above the hole. If the tanks are to have equal volume flow rates, what height above the hole must the liquid in tank 2 be just then?View SolutionQuestion 28
In Fig. a spring of spring constant 3.75×104 N/m Container is between a rigid beam Spring and the output piston of a hydraulic lever. An empty container with negligible mass sits on the input piston. The input piston has area Ai and the output piston has area 18.0Ai Initially the spring is at its rest length. How many kilograms of sand must be (slowly) poured into the container to compress the spring by 5.00 cm?
 View Solution
View SolutionQuestion 29
Two identical cylindrical vessels with their bases at the same level each contain a liquid of density 1.30×103kg/m3. The area of each base is 4.25cm2 , but in one vessel the liquid height is 0.854 m and in the other it is 1.560 m. Find the work done by the gravitational force in equalizing the levels when the two vessels are connectedView SolutionQuestion 30
Figure shows two sections of an old pipe system that runs through a hill, with distances dA=dB=40 m and D=110 m. On each side of the hill, the pipe radius is 2.00 cm. However, the radius of the pipe inside the hill is no longer known. To determine it, hydraulic engineers first establish that water flows through the left and right sections at 2.50 m/s. Then they release a dye in the water at point A and find that it takes 88.8 s to reach point B. What is the average radius of the pipe within the hill?
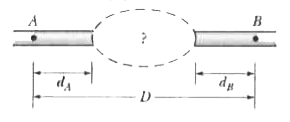 View Solution
View SolutionQuestion 33
Canal effect. Figure shows an anchored barge that extends across a canal by distance d = 30 m and into the water by distance b = 12 m. The canal has a width D = 55 m, a water depth H = 14 m, and a uniform water-flow speed vi=1.2 m/s. Assume that the flow around the barge is uniform. As the water . passes the bow, the water level undergoes a dramatic dip known as the canal effect. If the dip has depth h = 0.80 m, what is the water speed alongside the boat through the vertical cross sections at (a) point a and (b) point b? The erosion due to the speed increase is a common concern to hydraulic engineers.
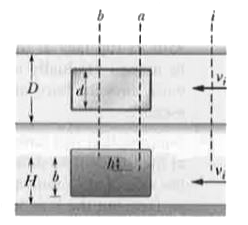 View Solution
View SolutionQuestion 35
The intake in Figure, Reservoir has cross-sectional area of 0.740m2 and water flows in at 0.400 m/s. At the outlet, the cross-sectional area is smaller than at the intake and the water flows out at 9.50 m/s. The pressure difference between intake and outlet is 3.00 MPa. What is vertical distance D?
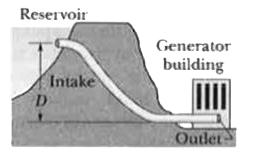 View Solution
View SolutionQuestion 36
A cylindrical tank with a large diameter is filled with water to a depth D =0.30 m. A hole of cross-sectional area A = 6.2 cm2 in the bottom of the tank allows water to drain out. (a) What is the drainage rate in cubic meters per second? (b) At what distance below the bottom of the tank is the cross-sectional area of the stream equal to one half the area of the hole? View SolutionQuestion 37
You inflate the front tires on your car to 32 psi. Later, you measure your blood pressure, obtaining a reading of 135/70, the readings being in mm Hg. In metric countries (which is to say, most of the world), these pressures are customarily reported in kilopascals (kPa). In kilopascals, what are (a) your tire pressure and (b) your blood pressure?View SolutionQuestion 39
The water flowing through a 1.9 cm (inside diameter) pipe flows out through three 1.5 cm pipes. (a) If the flow rates in the three smaller pipes are 26, 21, and 16 L/min, what is the flow rate in the 1.9 cm pipe? (b) What is the ratio of the speed in the 1.9 cm pipe to that in the pipe carrying 26 L/min?View SolutionQuestion 41
Figure shows a stream of water flowing through a hole at depth h = 12 cm in a tank holding water to height H = 40 cm. (a) At what distance x does the stream strike the floor? (b) At what depth should a second hole be made to give the same value of x? (c) At what depth should a hole be made to maximize x?
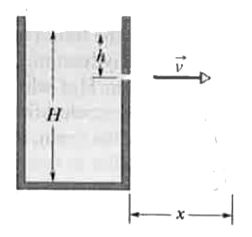 View Solution
View SolutionQuestion 43
A pitot tube Fig. is used to determine the air speed of an airplane. It consists of an outer tube with a number of small holes B (four are shown that allow air into the tube, that tube is connected to one arm of a U-tube. The other arm of the U-tube is connected to hole A at the front end of the device, which points in the direction the plane is headed. At A the air becomes stagnant so that vA = 0. At B, however, the speed of the air presumably equals the airspeed v of the plane. (a) Use Bernoulli's equation to show that
v=√2ρghρair
where ρ is the density of the liquid in the U-tube and his the difference in the liquid levels in that tube. (b) Suppose that the tube contains alcohol and the level difference h is 20.0 cm. What is the plane's speed relative to the air? The density of the air is 1.03kg/m3 and that of alcohol is 810 kg/m3
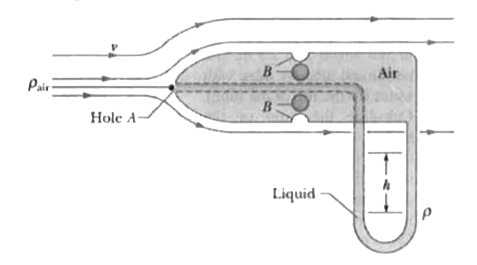 View Solution
View SolutionQuestion 45
Models of torpedoes are sometimes tested in a horizontal pipe of flowing water, much as a wind tunnel is used to test model airplanes. Consider a circular pipe of internal diameter 25.0 cm and a torpedo model aligned along the long axis of the pipe. The model has a 6,00 cm diameter and is to be tested with water flowing past it at 2.00 m/s. (a) With what speed must the water flow in the part of the pipe that is unconstricted by the model? (b) What will the pressure difference be between the constricted and unconstricted parts of the pipe? View SolutionQuestion 46
In one observation, the column in a mercury barometer (as is shown in Fig. ) has a measured height h of 740.35 mm. The temperature is −5.0∘ C, at which temperature the density of mercury ρ is 1.3608×104kg/m3 . The free-fall acceleration g at the site of the barometer is 9.7828 m/s2. What is the atmospheric pressure at thal site in pascals and in torr (which is the common unit for barometer readings)? View SolutionQuestion 47
What fraction of the volume of an iceberg (density 917kg/m3 ) would be visible if the iceberg floats (A) in the ocean (salt water density 1024kg/m3) and (B) in a river (fresh water density 1000 kg/m3 )?(when salt water freezes to form ice, the salt is excluded. So an iceberg could provide fresh water to a community) View SolutionQuestion 49
At a depth of 10.9 km, the Challenger Deep in the Marianas Trench of the Pacific Ocean is the deepest site in any ocean. Yet, in 1960, Donald Walsh and Jacques Piccard reached the Challenger Deep in the bathyscaph Trieste. Assuming that seawater has a uniform density of 1024 kg/m3, approximate the hydrostatic pressure (in atmospheres) that the Trieste had to withstand. (Even a slight defect in the Trieste structure would have been disastrous.) View SolutionQuestion 50
A water pipe having a 2.5 cm inside diameter carries water into the basement of a house at a speed of 0.90 m/s and a pressure of 190 kPa. If the pipe tapers to 1.2 cm and rises to the second floor 7.6 m above the input point, what are the (a) speed and (b) water pressure at the second floor ? View SolutionQuestion 52
In Fig. water flows through a horizontal pipe and then out into the atmosphere at a speed v1 = 23.0 m/s. The diameters of the left and right sections of the pipe are 5.00 cm and 3.00 сm. (a) What volume of water flows into the atmosphere during a 20.0 min period? In the left section of the pipe, what are (b) the speed v2 and (c) the gauge pressure?
 View Solution
View SolutionQuestion 53
Three liquids that will not mix are poured into a cylindrical container. The volumes and densities of the liquids are 1.50 L, 2.6 g/cm3: 0.75 L, 1.0 g/cm3 , and 0.60 L, 0.80 g/cm3. What is the force on the bottom of the container due to these liquids? One liter = 1 L = 1000 cm3 (Ignore the contribution due to the atmosphere.)View SolutionQuestion 54
Consider the venturi tube of Sample Problem 14.21 and Fig.without the manometer. Let A equal 5a. Suppose the pressure p1 at A is 3.0 atm. Compute the values of (a) the speed V at A and (b) the speed vat a that make the pressure p2 , at a equal to zero. (c) Compute the corresponding volume flow rate if the diameter at A is 5.0 cm. The phenomenon that occurs at a when p, falls to nearly zero is known as cavitation. The water vaporizes into small bubbles. View SolutionQuestion 55
In 1654 Otto von Guericke, inventor of the air pump, gave a demonstration before the noble-men of the Holy Roman Empire in which two teams of eight horses could not pull apart two evacuated brass hemispheres. (a) Assuming the hemispheres have (strong) thin walls, so that R in Fig. 14-63 may be considered both the inside and outside radius, show that the force →F required to pull apart the hemispheres has magnitude F=πR2Δp, where Δp is the difference between the pressures outside and inside the sphere,
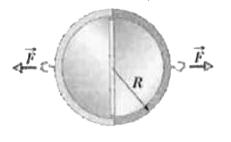
(b) Taking R as 40 cm, the inside pressure as 0.10 atm, and the outside pressure as 1.00 atm, find the force magnitude the teams of horses would have had to exert to pull apart the hemispheres. (c) Explain why one team of horses could have proved the point just as well if the hemispheres were attached to a sturdy wall. View SolutionQuestion 58
Water is moving with a speed of 5.0 m/s through a pipe with a cross-sectional area of 4.0cm2. The water gradually descends 12 m as the pipe cross-sectional area increases to 8.0cm2 (a) What is the speed at the lower level? (b) If the pressure at the upper level is 1.5×105 Pa, what is the pressure at the lower level?View SolutionQuestion 59
Crew members attempt to escape from a damaged submarine 100 m below the surface. What force must be applied to a pop-out hatch, which is 1.2 m by 0.60 m, to push it out at that depth? Assume that the density of the ocean water is 1024 kg/m3 and the internal air pressure is at 1.00 atm.View SolutionQuestion 60
A very simplified schematic of the rain drainage system for a home is shown in Fig. Rain falling on the slanted roof runs off into gutters around the roof edge, it then drains through downspouts (only one is shown) into a main drainage pipe M below the basement, which carries the water to an even larger pipe below the street. In Fig. a floor drain in the basement is also connected to drainage pipe M. Suppose the following apply:
(1) the downspouts have height h1 = 11 m,
(2) the floor drain has height h2 = 1.2 m,
(3) pipe M has radius 2.0 cm,
(4) the house has side width w = 40 m and front length L = 70 m,
(5) all the water striking the roof goes through pipe M,
(6) the initial speed of the water in a downspout is negligible, and
(7) the wind speed is negligible (the rain falls vertically).
At what rainfall rate, in centimeters per hour, will water from pipe M reach the height of the floor drain and threaten to flood the basement?
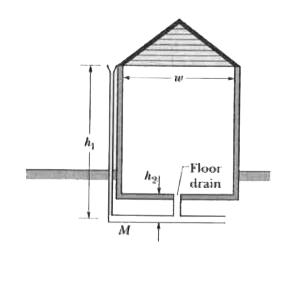 View Solution
View SolutionQuestion 63
Suppose that you release a small ball from rest at a depth of 0.400 m below the surface in a pool of water. If the density of the ball is 0.450 that of water and if the drag force on the ball from the water is negligible, how high above the water surface will the ball shoot as it emerges from the water? (Neglect any transfer of energy to the splashing and waves produced by the emerging ball.) View SolutionQuestion 64
In Fig. an open tube of length L = 2.3 m and crosssectional area A = 9.2 cm2 is fixed to the top of a cylindrical barrel of diameter D = 1.2 m and height H = 2.3 m. The barrel and tube are filled with water to the top of the tube). Calculate the ratio of the hydrostatic force on the bottom of the barrel to the gravitational force on the water contained in the barrel. Why is that ratio not equal to 1.0? (You need not consider the atmospheric pressure.)
 View Solution
View SolutionQuestion 65
In Fig. a cube of edge length L=0.500 m and mass 450 kg is suspended by a rope in an open tank of liquid of density 1030 kg/m3. Find (a) the magnitude of the total downward force on the top of the cube from the liquid and the atmosphere, assuming atmospheric pressure is 1.00 atm, (b) the magnitude of the total upward force on the bottom of the cube, and (c) the tension in the rope. (d) Calculate the magnitude of the buoyant force on the cube using Archimedes' principle. What relation exists among all these quantities?
 View Solution
View SolutionQuestion 66
The bends during flight. Anyone who scuba dives is advised not to fly within the next 24 h because the air mixture for diving can introduce nitrogen to the bloodstream. Without allowing the nitrogen to come out of solution slowly, any sudden air-pressure reduction (such as during airplane ascent) can result in the nitrogen forming bubbles in the blood, creating the bends, which can be painful and even fatal. Military special operation forces are especially at risk. What is the change in pressure on such a special-op soldier who must scuba dive at a depth of 25 m in seawater one day and parachute at an altitude of 8.1 km the next day? Assume that the average air density within the altitude range is 0.87 kg/m3. View SolutionQuestion 67
If Fig. 14-67a , a rectangular block is gradually pushed facedown into a liquid . The block has height d, on the bottom and top the face area is A = 8.00cm2 . Figure gives the apparent weight Wapp of the block as a function of the depth h of its lower face . The scale on the vertical axis is set by Wa=0.20 N . What is the density of the liquid ?
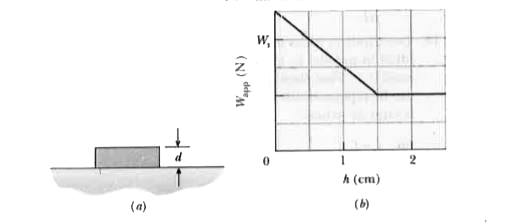 View Solution
View Solution
All Exercises from RESNICK AND HALLIDAY PHYSICS (HINGLISH) Class 12 PHYSICS FLUIDS
- CHECKPOINT
- PROBLEMS
- PRACTICE QUESTIONS (MORE THAN ONE CORRECT CHOICE TYPE )
- PRACTICE QUESTIONS (INTEGER TYPE )
- PRACTICE QUESTIONS (LINKED COMPREHENSION )
- PRACTICE QUESTIONS (MATRIX - MATCH )
- PRACTICE QUESTIONS (SINGLE CORRECT CHOICE TYPE)
- SAMPLE PROBLEM 14.01
- SAMPLE PROBLEM 14.02
- SAMPLE PROBLEM 14.03
- SAMPLE PROBLEM 14.04
- SAMPLE PROBLEM 14.05
- SAMPLE PROBLEM 14.06
- SAMPLE PROBLEM 14.07
- SAMPLE PROBLEM 14.08
- SAMPLE PROBLEM 14.09
- SAMPLE PROBLEM 14.10
- SAMPLE PROBLEM 14.11
- SAMPLE PROBLEM 14.12
- SAMPLE PROBLEM 14.13
- SAMPLE PROBLEM 14.14
- SAMPLE PROBLEM 14.15
- SAMPLE PROBLEM 14.16
- SAMPLE PROBLEM 14.17
- SAMPLE PROBLEM 14.18
- SAMPLE PROBLEM 14.19
- SAMPLE PROBLEM 14.20
- SAMPLE PROBLEM 14.21
- SAMPLE PROBLEM 14.22
- SAMPLE PROBLEM 14.23
- SAMPLE PROBLEM 14.24
- SAMPLE PROBLEM 14.25
- SAMPLE PROBLEM 14.26
- SAMPLE PROBLEM 14.27
- SAMPLE PROBLEM 14.28
- SAMPLE PROBLEM 14.29