Let C be a circle with centre ‘O’ and HK is the chord of contact of pair of the tangents from point A. OA intersects the circle C at P and Q and B is the midpoint of HK then Statement-I: AB is the harmonic mean of AP and AQ.Statement-II: AK is the Geometric mean of AB and AO and OA is the arithmetic mean of AP and AQA Statement-I is true statement-II is true and statement-II is correct explanation for statement-I. B Statement-I is true statement-II is true and statement-II is NOT the correct explanation for statement-I. C Statement-I is true statement-II is false. D Statement-I is false statement-II is true
Correct option C Statement-I is true statement-II is false. Explanation:
Related Solutions
AP and AQ are two tangents drawn from an external point A to a circle with centre O, P and Q are points of contact. If PR is a diameter, prove that
View Solutionकेंद्र O वाले वृत्त में, AB जीवा है जिसकी लम्बाई 10 cm है। बिंदु A और B पर खींची गई स्पर्शरेखएं, वृत्त के बाहर बिंदु P पर प्रतिच्छेदित करती है। यदि OP = 2 OA है, तो AP की लम्बाई (cm में) ज्ञात करे।
View Solutionमाना एक वृत्त है तथा 0 मूल बिन्दु है। माना OAB एक रेखा है जो वृत्त को A तथा B बिन्दुओं पर प्रतिच्छेद करती है। जीवा AB पर एक बिन्दु P लिया गया है। निम्न स्थितियों में P का बिन्दुपथ ज्ञात कीजियेः
(i) यदि OA तथा OB का समान्तर माध्य OP है।
(ii) यदि OA तथा OB का गुणोत्तर माध्य OP है।
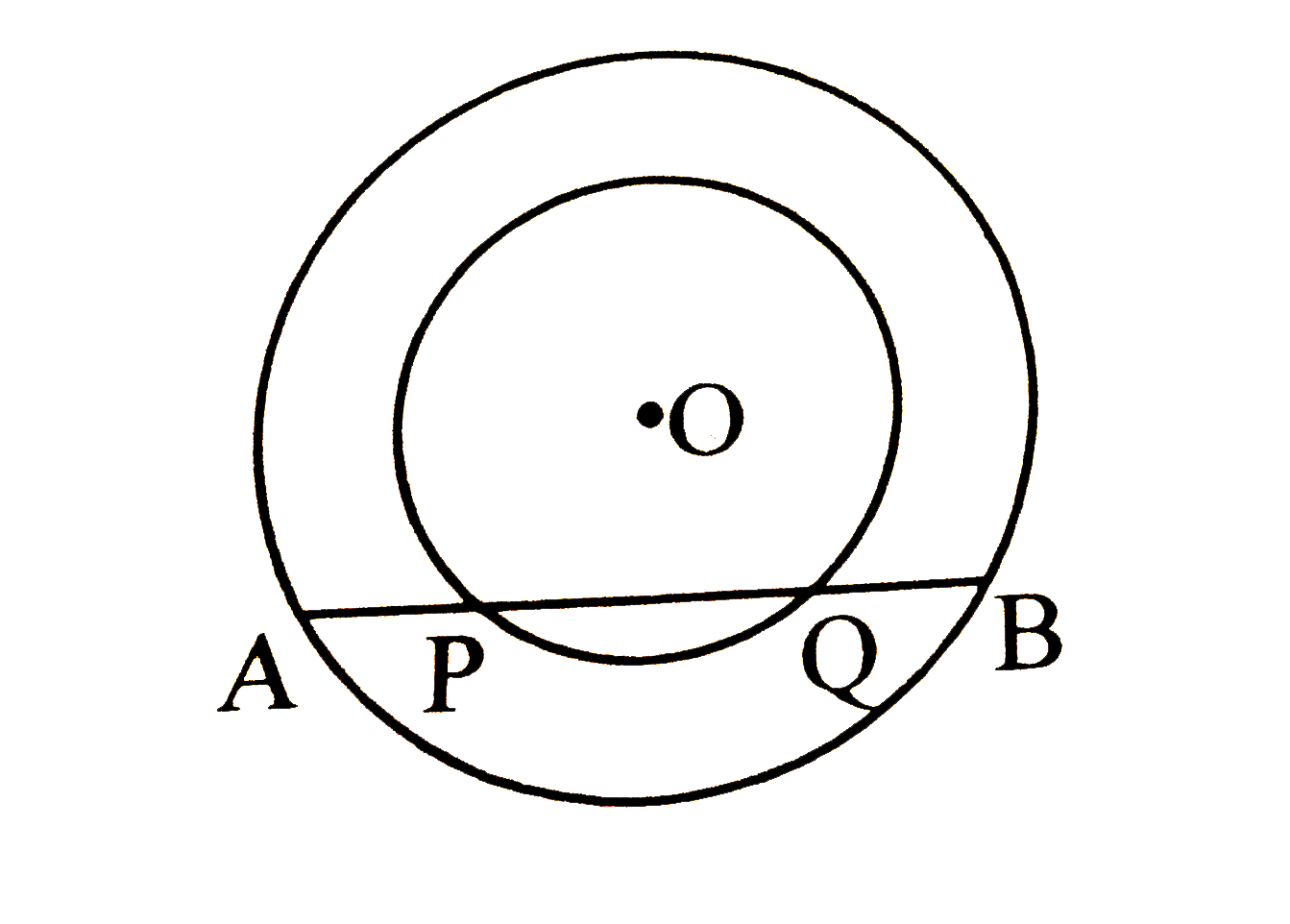
(iii) यदि OA तथा OB का हरात्मक माध्य OP है।View SolutionIn the given figure, centre of two circles is O. Chord AB of bigger circle intersects the smaller circle in points P and Q . Show that AP = BQ.
 View Solution
View SolutionIn the given figure, centre of two circles is O. Chord AB of bigger
circle intersects the smaller circle in points P and Q .
Show that AP = BQ.
 View Solution
View SolutionThe figure has two circles with O center. The chord AB of the large circle intersects the small circle at points P and Q. So learn: AP = BQ
View SolutionIn circle with centre if is chord and is point on such that cm and cm then is
View SolutionO AP and AQ are two tangents to the central circle. If the radius of the circle is 9 cm and OA = 15 cm AP+AQ = ?
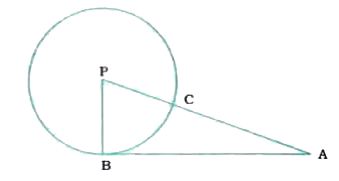
View SolutionAB is a tangent to a cricle with centre P and B is the point of contact. PA intersects the circle at C. If AB=15cm and AC=9cm, find the radius of the circle.
 View Solution
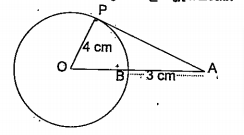
View Solution4 സെന്റിമീറ്റര് ആരമുള്ള ഒരു വൃത്തത്തില് A യില് നിന്നുള്ള തൊടുവരയാണ് AP. AB യുടെ നീളം 3 സെന്റിമീറ്ററാണ്. OA യുടെ നീളം എത്ര? AP യുടെ നീളം ഏത്രയാണ്?
 View Solution
View SolutionThe tangent AB touches a -circle with centre O, at the point P. If the radius of the circle is 5cm,OB =10 cm and OB = AB then find AP.
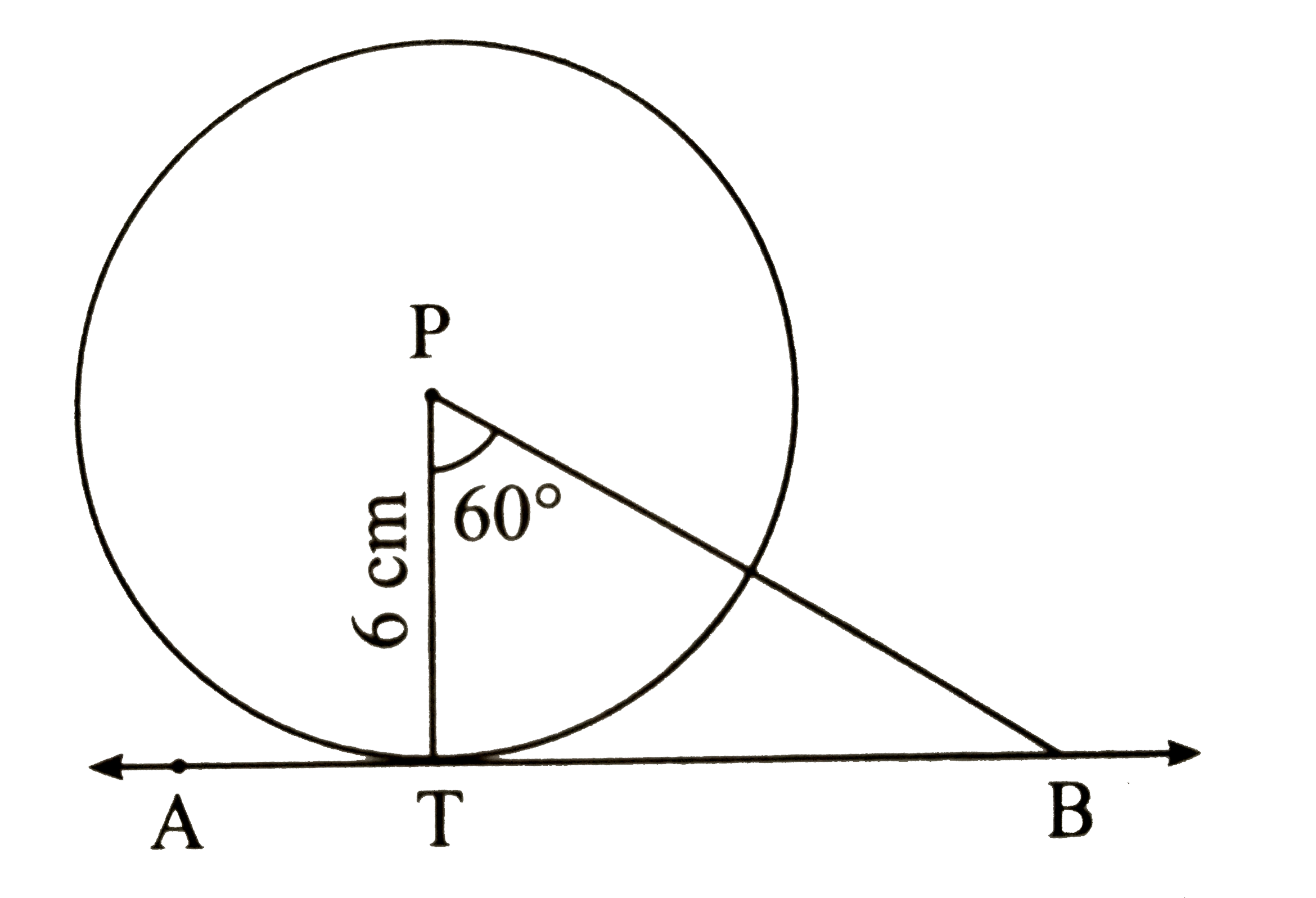
View SolutionIn the figure, point P is the centre of the circle and line AB is the tangent to the circle at the point T. The radius of the circle is 6 cm . Find PB, if
 View Solution
View SolutionAB and AC are tangents to a circle with centre O. A is the external point of the circle. The line AO intersect the chord BC at D. The measure of the is
View SolutionTwo variable chords AB and BC of a circle are such that . M and N are the midpoints of AB and BC, respectively, such that the line joining MN intersects the circles at P and Q, where P is closer to AB and O is the center of the circle.
The locus of the points of intersection of tangents at A and C isView SolutionTwo variable chords AB and BC of a circle are such that . M and N are the midpoints of AB and BC, respectively, such that the line joining MN intersects the circles at P and Q, where P is closer to AB and O is the center of the circle.
The locus of the points of intersection of tangents at A and C isView SolutionTwo variable chords AB and BC of a circle are such that . M and N are the midpoints of AB and BC, respectively, such that the line joining MN intersects the circles at P and Q, where P is closer to AB and O is the center of the circle.
The locus of the points of intersection of tangents at A and C isView SolutionTwo variable chords AB and BC of a circle are such that . M and N are the midpoints of AB and BC, respectively, such that the line joining MN intersects the circles at P and Q, where P is closer to AB and O is the center of the circle.
The locus of the points of intersection of tangents at A and C isView SolutionTwo variable chords AB and BC of a circle are such that . M and N are the midpoints of AB and BC, respectively, such that the line joining MN intersects the circles at P and Q, where P is closer to AB and O is the center of the circle.
The locus of the points of intersection of tangents at A and C isView SolutionAP and AQ are tangent drawn from a ponit A to a circle with centre O and radius 9cm. If OA = 15 cm. then AP + AQ = …….. Cm.
View SolutionIf ‘a', is the Arithmetic mean of b and c, 'b', is the Geometric mean of c and a, then prove that 'c' is the Harmonic mean of a and b.
View Solution
