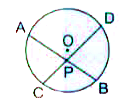
In the given figure, centre of two circles is O. Chord AB of bigger circle intersects the smaller circle in points P and Q . Show that AP = BQ.
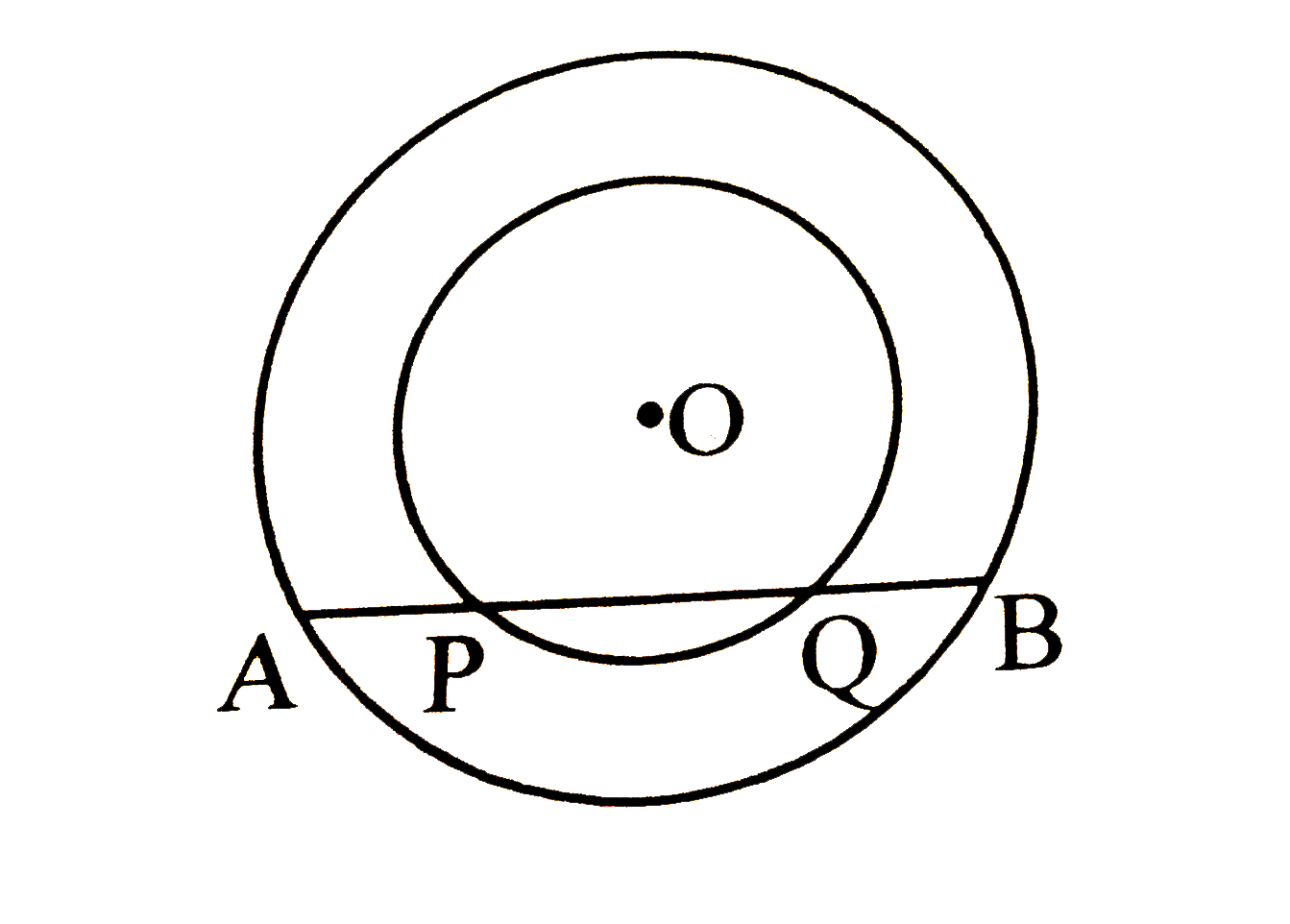
Step by step video & image solution for In the given figure, centre of two circles is O. Chord AB of bigger circle intersects the smaller circle in points P and Q . Show that AP = BQ. by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.
Similar Questions
In the given figure, O is the centre of the circle.

Name all chords of the circle.View SolutionIn the adjoining figure,O is the centre of two concentric circles. The chord AB of larger circle intersects the smaller circle at C and D.
(i) Find AC:BD.
(ii) If AC=2cm, then find the length of BD.
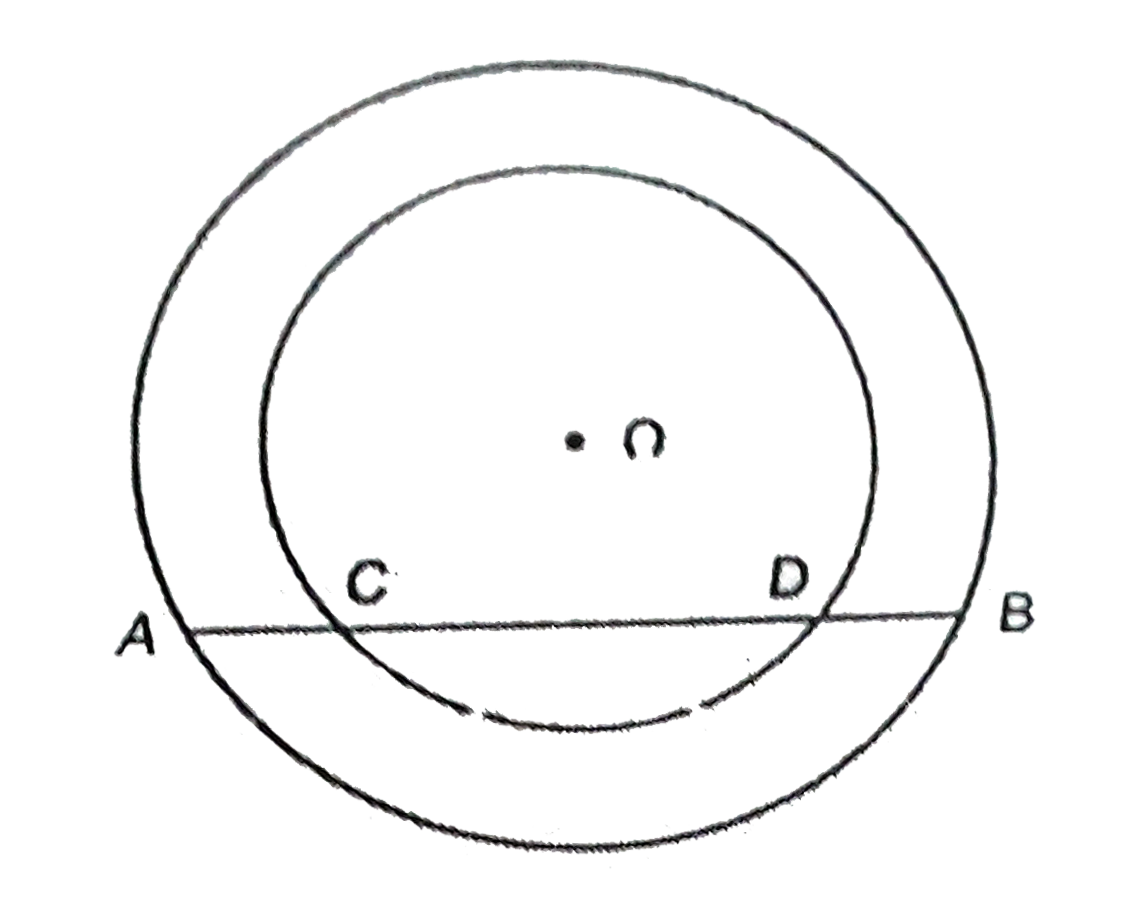 View Solution
View SolutionIn the figure, two circles touch internally at point P. chord AB of the larger circle intersects the smaller circle in C and D. Prove ∠CPA≅∠DPB.
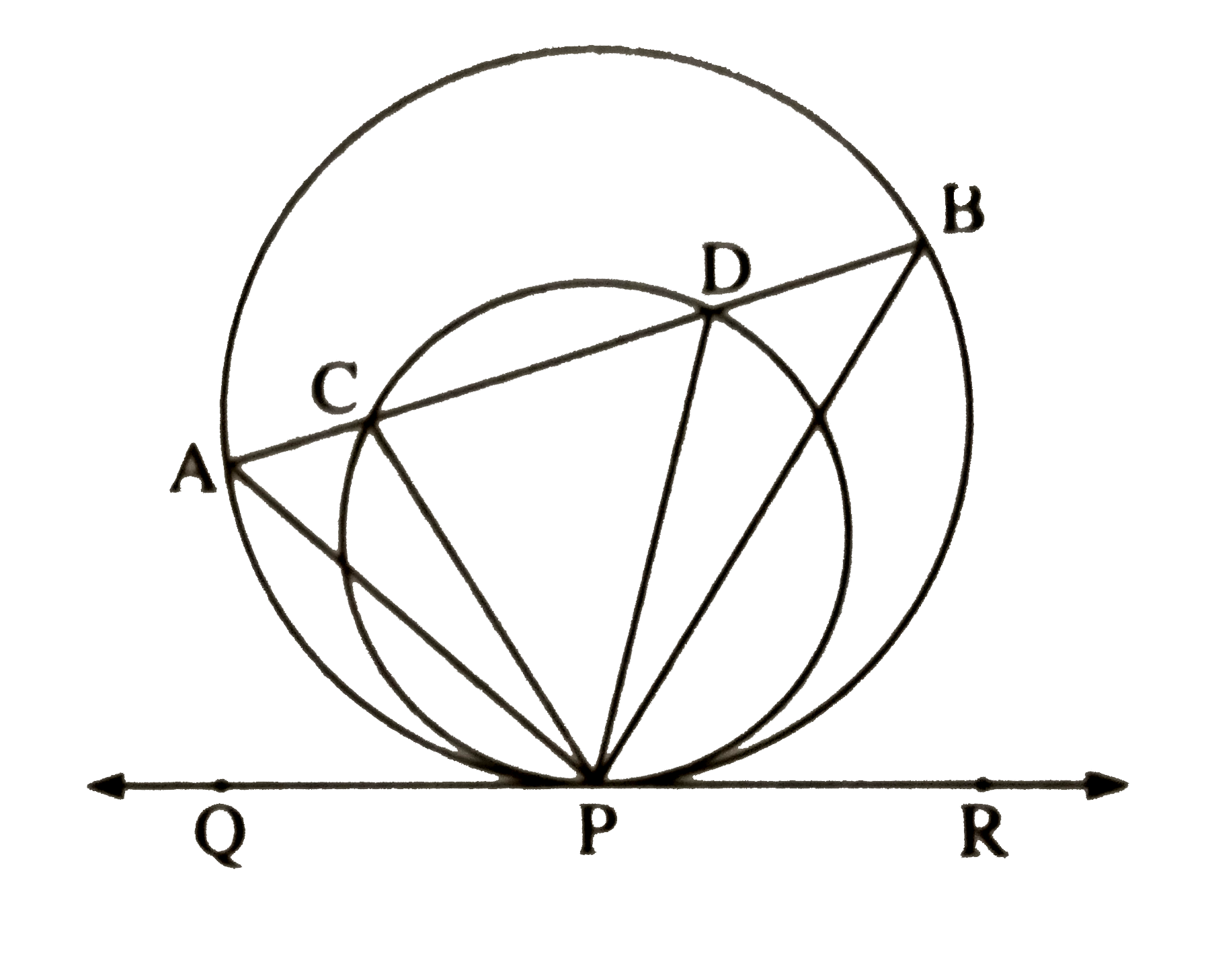 View Solution
View SolutionIn the given figure, O is the centre of the circle.

Name a chord, which is not the diameter of the circle.View SolutionIn given figure, O is the centre of the circle.
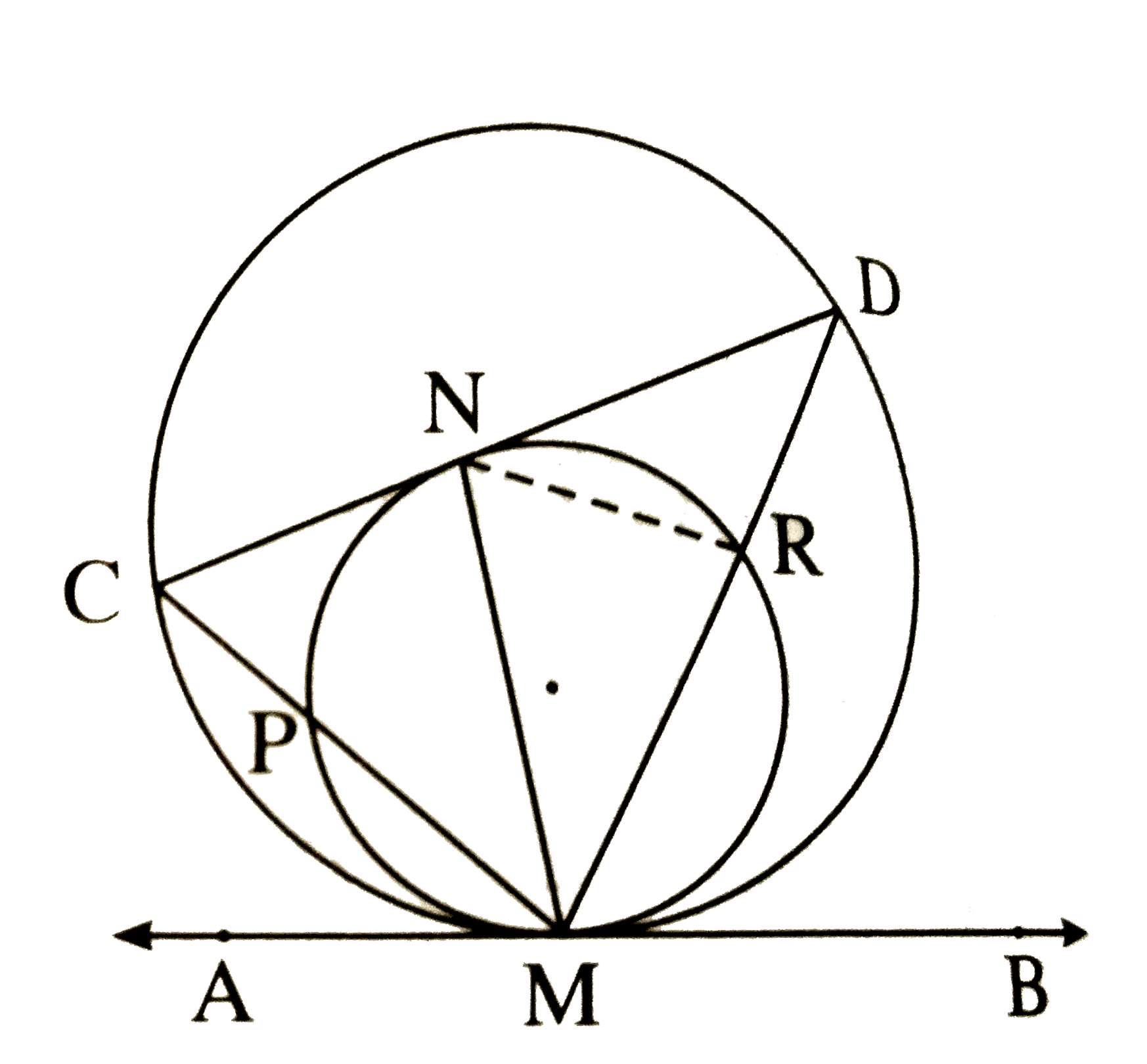
A chord, which is not the diameter of the circle isView SolutionLet M be a point of contact of two internally touching circles. Let line AMB be their common tangent. The chord CD of the bigger circle touches the smaller circle at the point N. Chord CM and chord DM of the bigger circler intersect the smaller circle at the points P and R respectively.
Prove that ∠CMN≅∠DMN.
Construction : Draw seg NR.
 View Solution
View SolutionIn the given figure, O is the centre of the circle. Its two chords AB and CD intersect each other at the point p within the circle. If AB=15cm,PB=9cm, then find the length of PD.
 View Solution
View SolutionIn the figure , two concentric circles of radii a&b(a>b) are given.The chord AB of larger circle touches the smaller circle at C .The length AB is :
View SolutionIn the given figure, O is the centre of the circle. Its two chords AB and CD intersect each other at the point P within the circle. If AB = 18 cm, PB = 8 cm, CP = 5 cm, then find the length of CD.
 View Solution
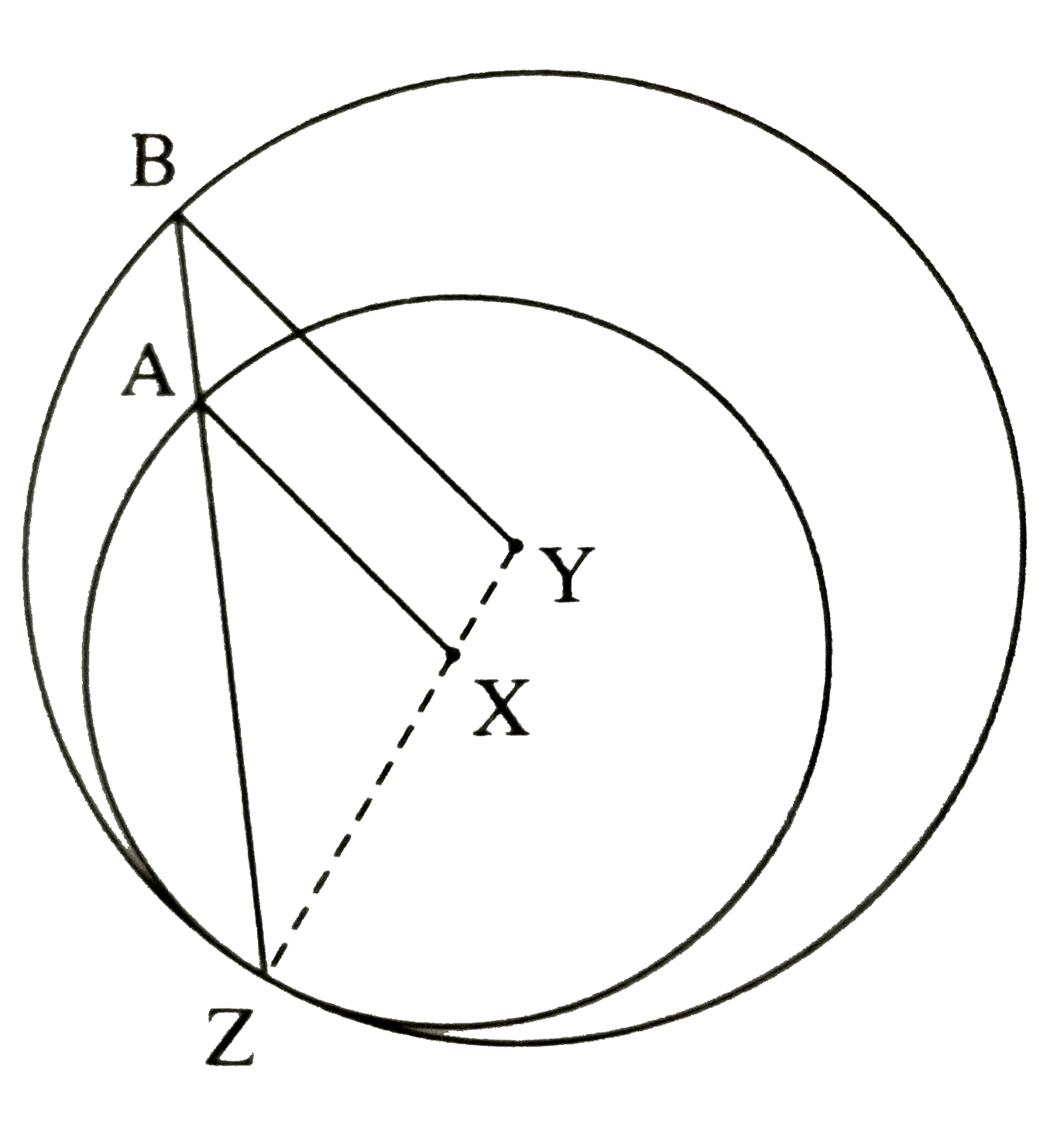
View SolutionIn the figure, circles with centres X and Y touch internally at point Z. Seg BZ is a chord of bigger circle and it intersects smaller circle at point A. Prove that, seg AX || seg BY.
 View Solution
View Solution

