Two blocks of masses m1 and m2 are connected by a spring of stiffness k.Another block of mass m1 sliding at V0 with out friction hits the set up elastically as shown in the figure.Plot elastic energy of the spring as a function of time t after the collision.
Due to one dimensional elastic collision of identical bodies velocity is transferred totally to m1.Let x1 and x2 be positions of m1 and m2 at a time t. Then extension in the spring with natural length = lx = x2 – x1 – l ...1The equation of motion of m1 is writen asFrom theseSolving these Fromi and ii
Related Solutions
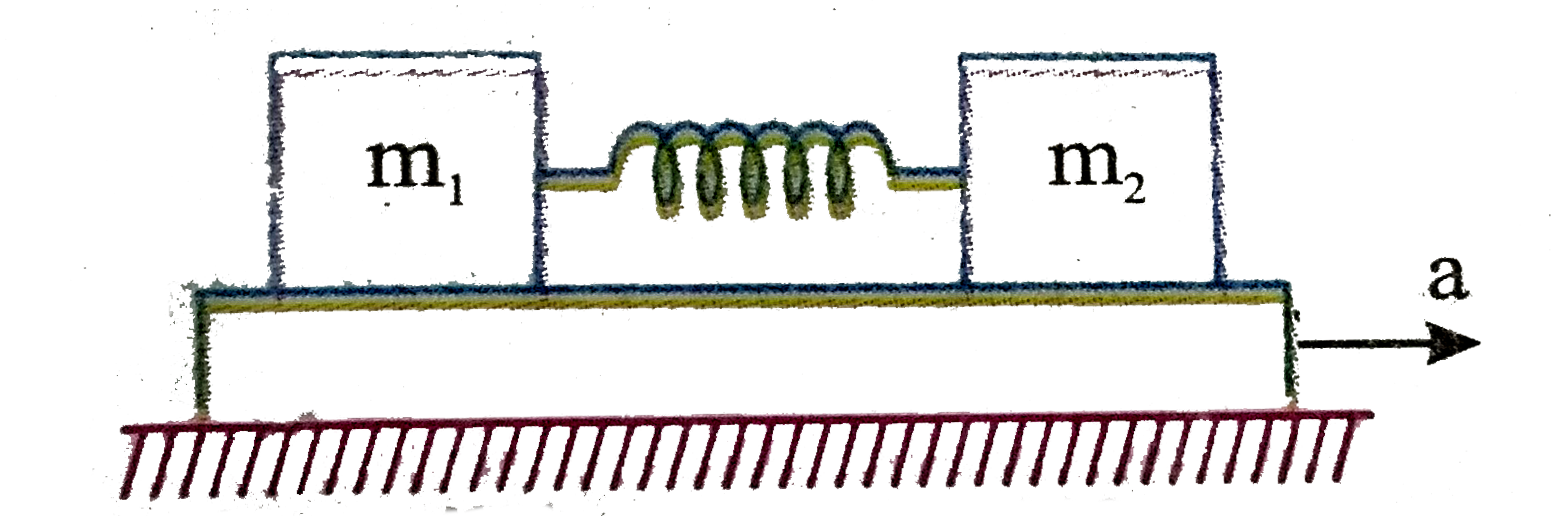
Two block of masses m1 and m2 are connected with a massless unstretched spring and placed over a plank moving with an acceleration 'a' as shown in figure. The coefficient of friction between the blocks and platform is mu
View SolutionTwo block of masses and are connected with a massless unstretched spring and placed over a plank moving with an acceleration as shown in figure. The coefficient of friction between the blocks and platform is
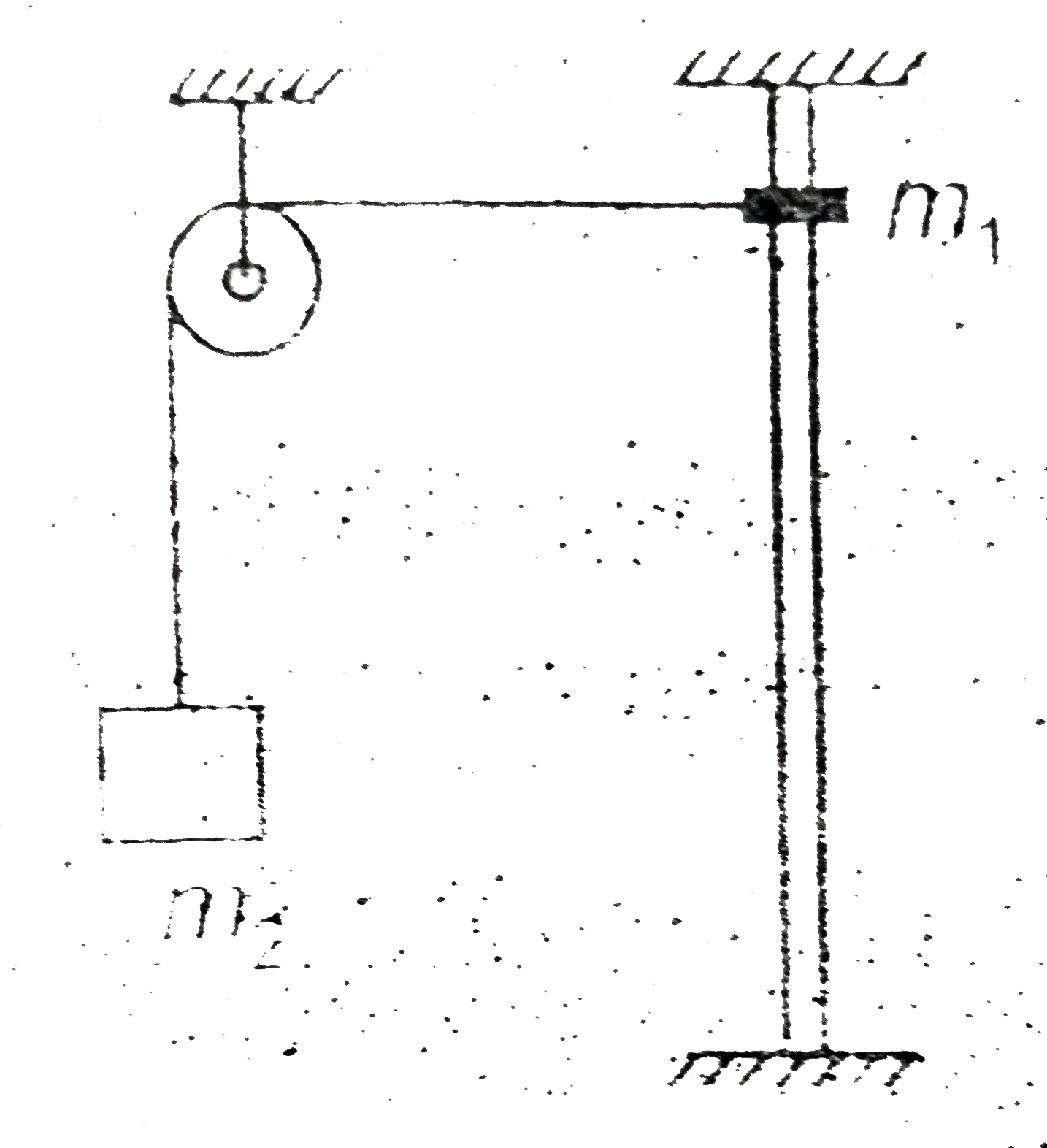
View SolutionA smooth ring of mass connected with a hanging block of mass by a long inextensible string, is released from rest. The ring moves vertically along a fixed rigid rod as shown in figure. Describe the motion of the ring if
(A)
(B)
(C )
View SolutionTwo masses m1 and m2 are suspended together by a massless spring of constant k. When the masses are in equilibrium, m1 is removed without disturbing the system. The amplitude of oscillations is

View SolutionTwo masses m1 and m2 are suspended together by a massless spring of constant k. When the masses are in equilibrium, m1 is removed without disturbing the system. The amplitude of oscillations is

View SolutionA block of mass attached to a spring of stiffness is lying on a frictionless floor as shown. The block is moved to compress the spring by and released. If the collision with the wall is elastic then find the time period of oscillations.
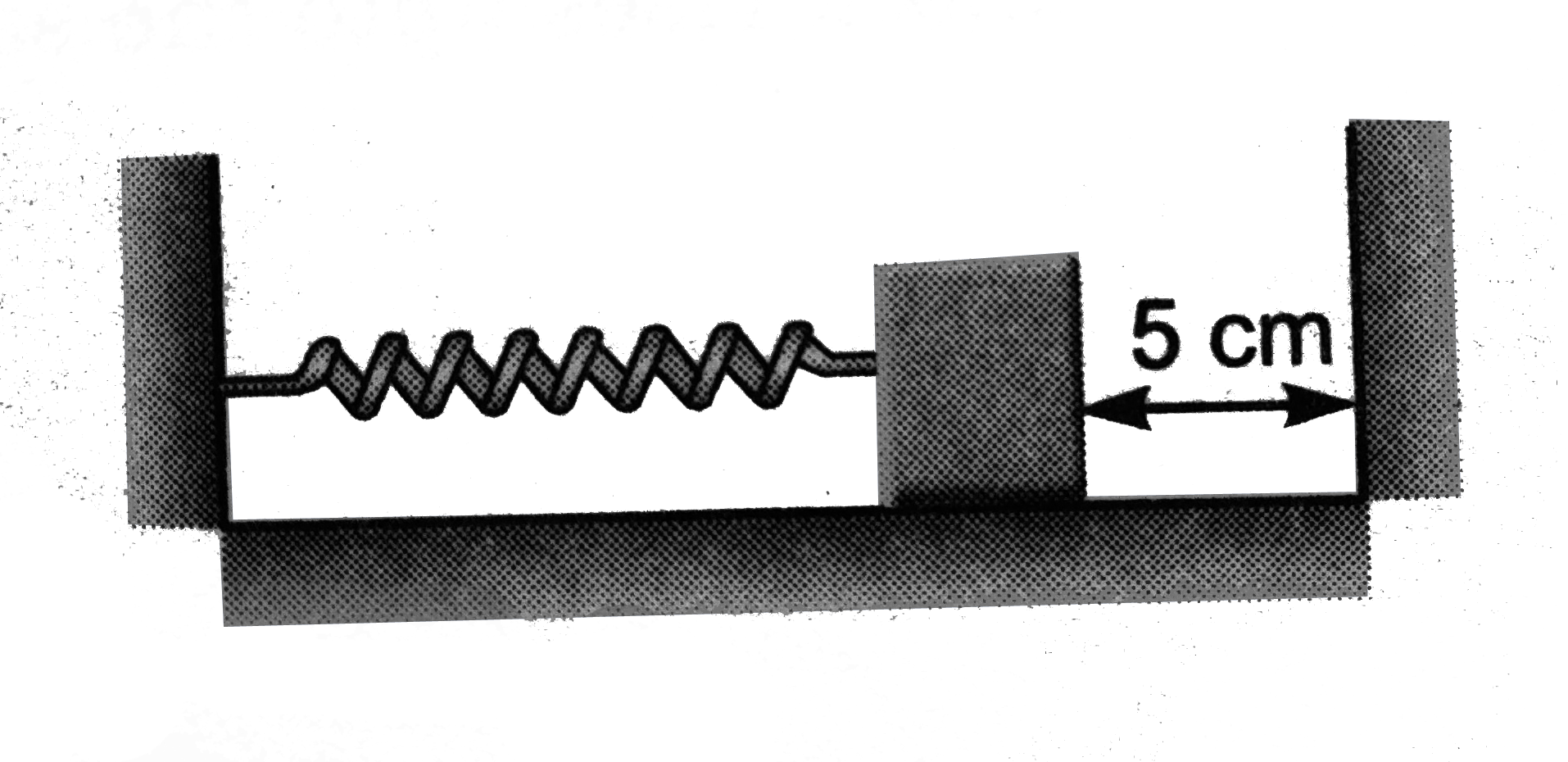
View SolutionA spring-block system is resting on a frictionless floor as shown in the figure. The spring constant is and the mass of the block is 2.0 kg. Ignore the mass of the spring. Initially the spring is in an unstretched condition. Another block of mass 1.0 kg moving with a speed of collides elastically with the first block. The collision is such that the 2.0 kg block does not hit the wall. The distance, in metres, between the two blocks when the spring returns to its unstretched position for the first time after the collision is
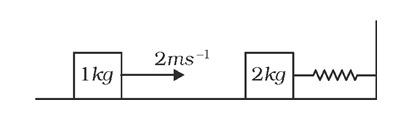
View SolutionA spring-block system is resting on a frictionless floor as shown in the figure. The spring constant is 2.0Nm^(-1) and the mass of the block is 2.0 kg. Ignore the mass of the spring. Initially the spring is in an unstretched condition. Another block of mass 1.0 kg moving with a speed of 2.0ms^(-1) collides elastically with the first block. The collision is such that the 2.0 kg block does not hit the wall. The distance, in metres, between the two blocks when the spring returns to its unstretched position for the first time after the collision is
View SolutionA spring-block system is resting on a frictionless floor as shown in the figure. The spring constant is 2.0 N m^(–1) and the mass of the block is 2.0 kg. Ignore the mass of the spring. Initially the spring is in an unstretched condition. Another block of mass 1.0 kg moving with a speed of 2.0 m s^(–1) collides elastically with the first block. The collision is such that the 2.0 kg block does not hit the wall. The distance, in metres, between the two blocks when the spring returns to its unstretched position for the first time after the collision is _________.
View SolutionTwo spherical balls of masses m1 and m2 collide as shown in figure. After collision mass m1 moves with velocity u/3 in a direction perpendicular to original direction. The angle at which mass m2 will move after collision is
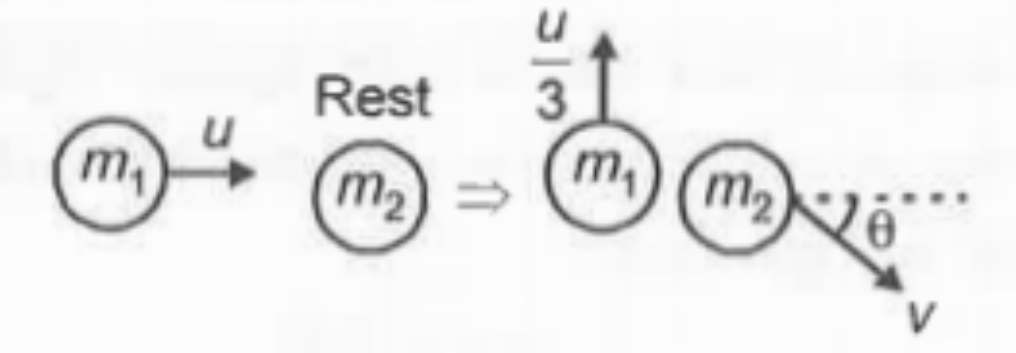
View SolutionIn the figure, the block of mass m, attached to the spring of stiffness k is in correct with the completely elastic wall, and the compression in the spring is e. The spring is compressed further by e by displacing the block towards left and is then released. If the collision between the block and the wall is completely eleastic then the time period of oscillation of the block will be
View SolutionIn the figure, the block of mass m, attached to the spring of stiffness k is in correct with the completely elastic wall, and the compression in the spring is e. The spring is compressed further by e by displacing the block towards left and is then released. If the collision between the block and the wall is completely eleastic then the time period of oscillation of the block will be

View SolutionTwo blocks of Masses M and 2M are connected by a spring of stiffness k .The coefficient of friction between the blocks and the surface is mu Find the minimum constant force F to be applied to 2m in order to slide the mass M .
View SolutionA small block of mass m is attached to free end of a spring of force cosntant k , whose other end is fixed to a rigid support . The block is lying on a frictionless horizontal plane and it starts movi0ng away from fixed end of the spring a\t t= 0 with intial velocity u When the speed of the block is it collides with a fixed heavy mass elastically. After the collision with a fixed heavy mass elastically . Aftere the collision the time at which the block passes through its equilibrium position for the first time is close to
View SolutionTwo masses m1 and m2 are connected by light string, which passes over the top of a smooth plane inclined at 30º to the horizontal, so that one mass rests on the plane and the other hangs vertically as shown in fig. It is found that m1, hanging vertically can draw m2 up the full length of the plane in half the time in which m2 hanging vertically draws m1 up. Find m1/m2 . Assume pulley to be smooth-

View SolutionFor the shown situation, if collision between block and is perfectly elastic, then find the maximum energy (in joules) stored in the spring.
'(##CEN_KSR_PHY_JEE_C08_E01_031_Q20##)'
View SolutionFor shown situation, if collision between block A and B is perfectly elastic, then find the maximum energy stored in spring in joules.
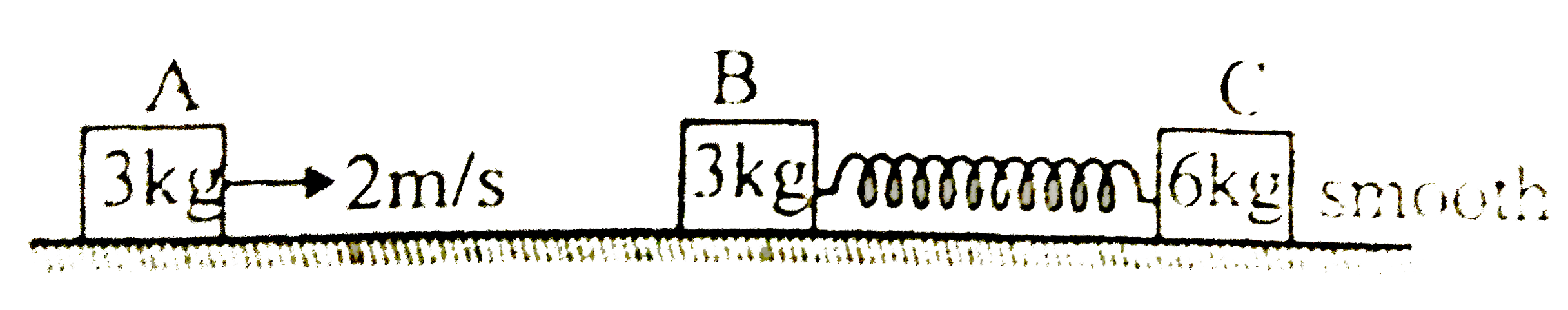
View SolutionFor shown situation, if collision between block A and B is perfectly elastic, then find the maximum energy stored in spring in joules.
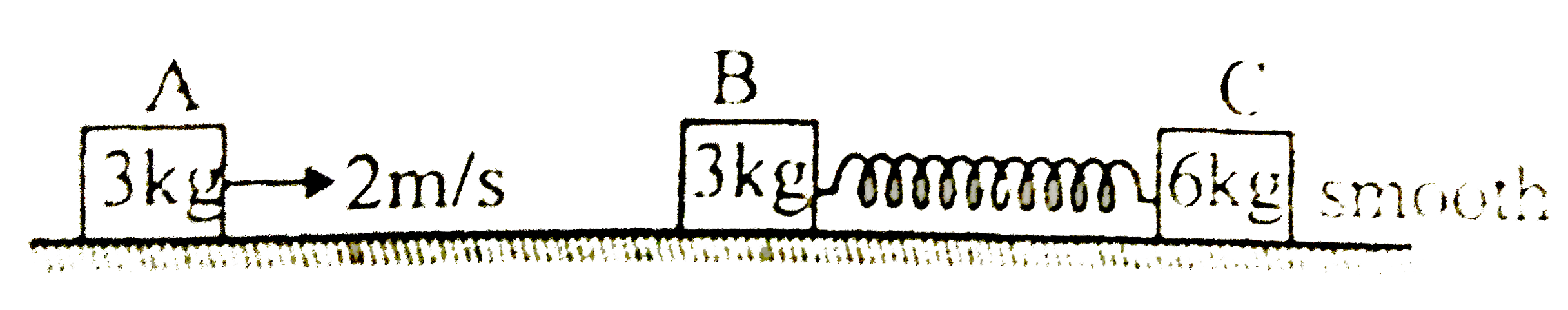
View SolutionFor shown situation, if collision between block A and B is perfectly elastic, then find the maximum energy stored in spring in joules.
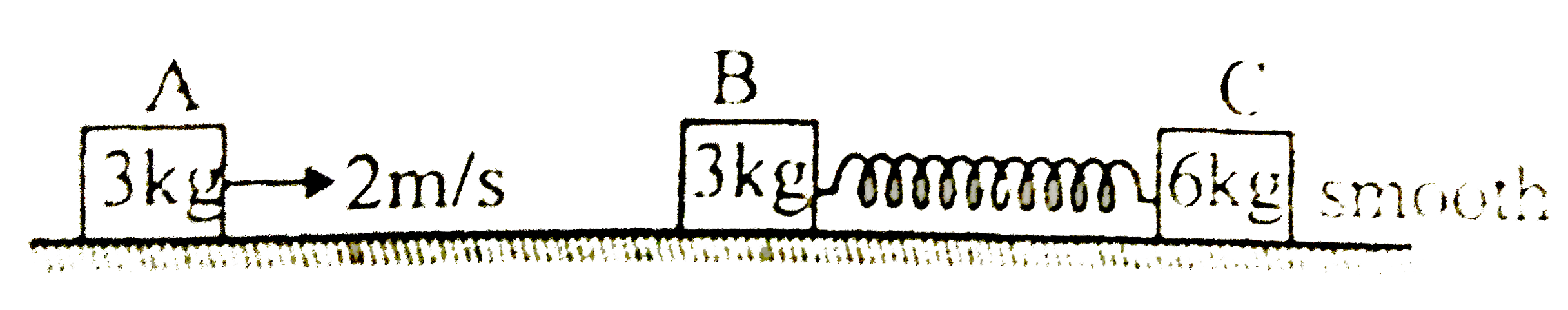
View SolutionThe figure shows two blocks of mass m each, which are connected by an ideal elastic spring and then placed on a frictionless floor. As shown, if the blocks are v_0 And 2v_0 The velocity is given, then the maximum extension in the spring is:
View Solution