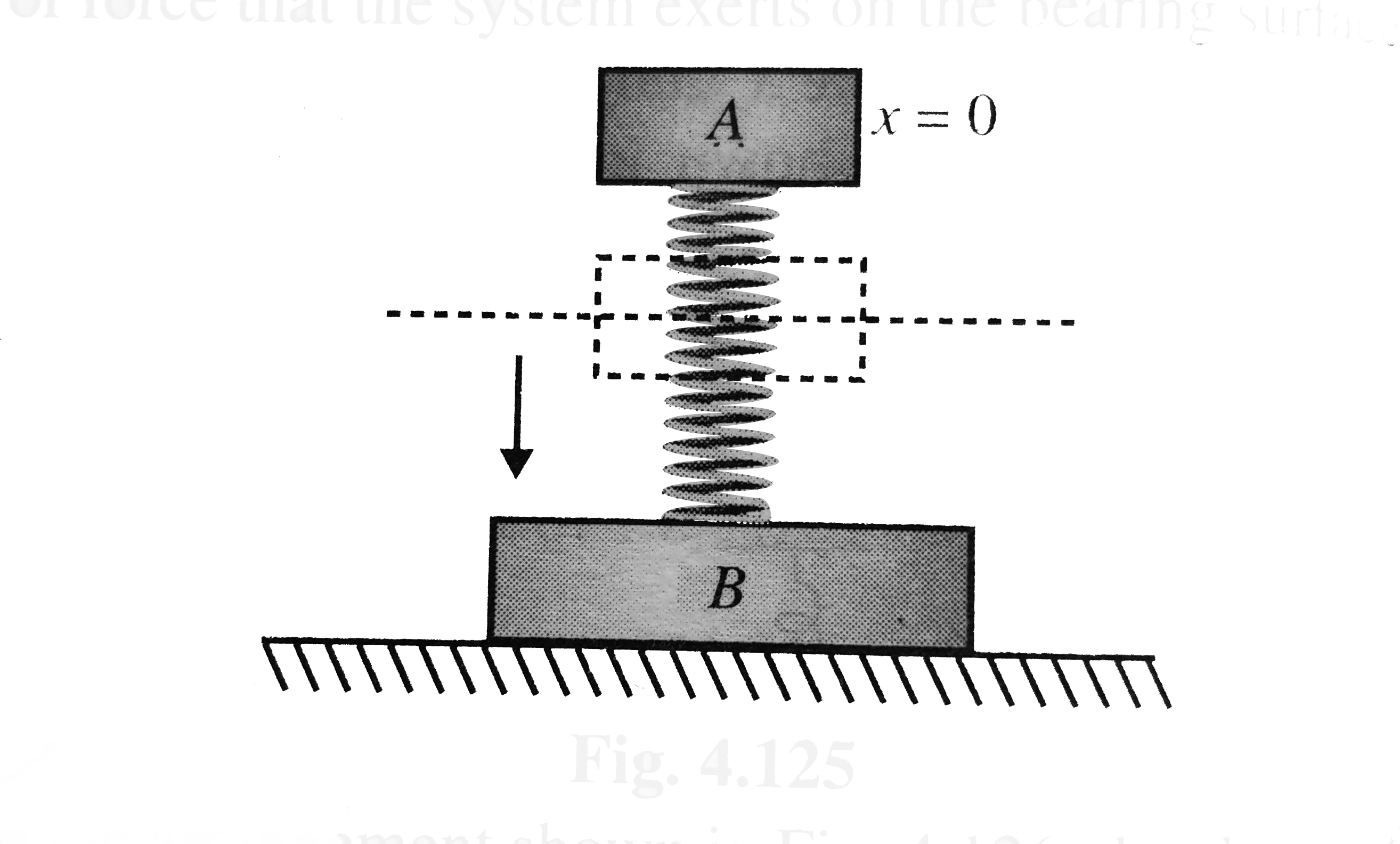
A body A of mass m1 = 1.00kg and a body B of mass m2 = 4.10kg are interconnected by a spring as shown in Fig. 4.9. The body A performs free vertical harmonic oscillations with amplitude a = 1.6cm and frequency ω = 25s-1. Neglecting the mass of the spring find the maximum and minimum values of force that this system exerts on the bearing surface.
While the body A is at its upper extreme position the spring is obviously elongated by the amountIf we indicate y-axis in vertically downward direction Newtons second law of motion in projection form i.e Fy = mwy for body A gives:Because at any extreme position the magnitude of acceleration of an oscillating body equals ω2a and is restoring in nature.If N be the normal force exerted by the floor on the body B while the body A is at its upper extreme position from Newtons second law for body BWhen the body A is at its lower extreme position the spring is compresed by the distanceFrom Newtons second law in projection form i.e. Fy = mwy for body A at this state:In this case if N be the normal force exerted by the floor on the body B from Newtons second lawFrom Newtons third law the magnitude of sought forces are N and N respectively.
Related Solutions
A body A mass m_(1) = 1 kg and body B of mass m_(2) = 4.1 kg . The body A perform free vertical harmonic oscillations with the amplitude 1.6 cm and frequency 25 Hz . Neglecting the mass of the spring , find the maximum and m inimum value of force that the system exerts on the hearing surface.
View SolutionA body A mass and body B of mass . The body A perform free vertical harmonic oscillations with the amplitude and frequency . Neglecting the mass of the spring , find the maximum and m inimum value of force that the system exerts on the hearing surface.
 View Solution
View SolutionA body of mass and body of mass are interconnected by a massless spring as shown. The body performs free vertical harmonic oscillations with the amplitude and frequency . The maximum value of such that body does not leave the surface is
 View Solution
View SolutionA body A of mass m_(1)=1kg and a body B of mass m_(2)=4kg are attached to the ends of a spring. The body A performs vertical simple harmonic oscillations of amplitude a=1.6 cm and angular frequency omeg=25rads . Neglecting the mass of the sppring determine the maximum and minimum values of force the system exerts on the surface on which in rests. ["Take g"=10 m//s^(2)]
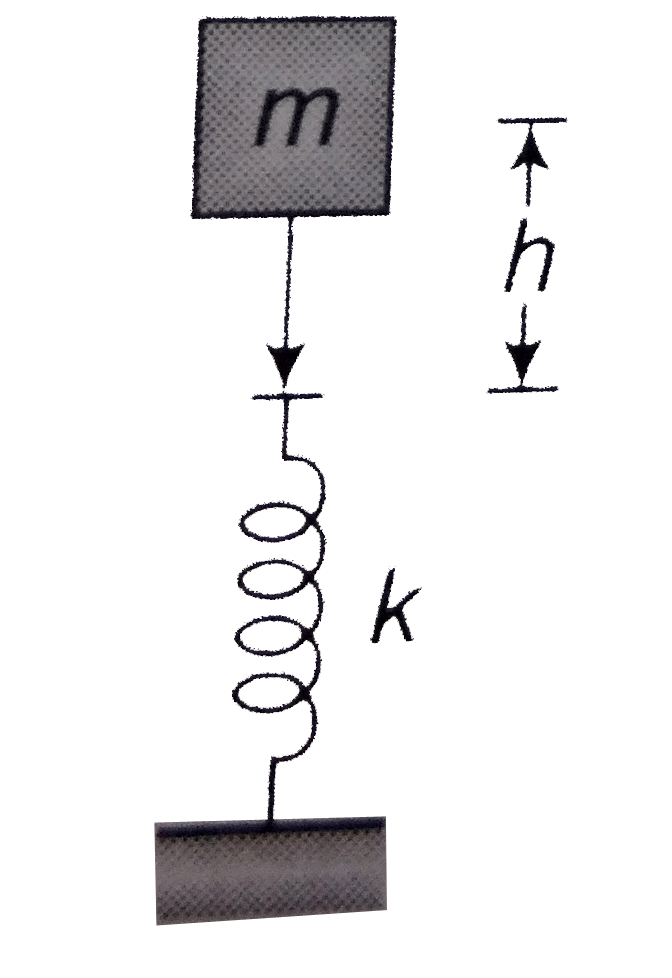
View SolutionA body of mass m falls from a height h onto the pan of a spring balance. The masses of the pan and spring are negligible. The force constant of the spring is k. The body sticks to the pan and oscillates simple harmonically. The amplitude of oscillation is
 View Solution
View SolutionTwo masses m1 and m2 are suspended together by a massless spring of constant k. When the masses are in equilibrium, m1 is removed without disturbing the system. The amplitude of oscillations is
 View Solution
View SolutionTwo masses m1 and m2 are suspended together by a massless spring of constant k. When the masses are in equilibrium, m1 is removed without disturbing the system. The amplitude of oscillations is
 View Solution
View SolutionA body of mass m falls from a height h on to the pan of a spring balance. The masses of the pan and spring are negligible. The spring constant of the spring is k. Having stuck to the pan the body starts performing harmonic oscillations in the vertical direction. Find the amplitude and energy of oscillation.
View SolutionAn M mass is connected to the horizontal spring of the body of the spring, the force of the spring is the constant k, one end of the spring is connected to a cooling base as shown. The body oscillates with T periodic and A amplitude on a frictionless surface. When the body is in equilibrium, another body whose mass is m is placed on it. What is the new dimension of oscillations then?
View Solutionएक क्षैतिज स्प्रिंग - पिंड निकाय जिसका द्रव्यमान M है सरल आवर्त गति करता है। जब पिंड अपनी माध्य स्थिति से गुजरता है तो उस पर m द्रव्यमान का एक पिंड रख दिया जाता है। नये आयाम तथा दोलन की आवृत्ति ज्ञात कीजिए।
View Solutionनगण्य द्रव्यमान के स्प्रिंग से m द्रव्यमान को ऊर्ध्वाधर लटका दिया जाता है। द्रव्यमान को ऊपर नीचे - नीचे दोलन कराने पर निकाय की आवृति n है। यदि उस स्प्रिंग से 4m द्रव्यमान लटकाया जाए तो निकाय की दोलन आवृत्ति होगी -
View SolutionA body of mass m was suspended by a non-stretched spring, and then set free without push. The stiffness of the spring is k. Neglecting the mass of the spring, find: the maximum and minimum tensions of the spring in the process of motion.
View Solutionकिसी स्प्रिंग पर लटका एक पिण्ड स्प्रिंग पर 24.5 न्यूटन का बल लगाता है। पिण्ड का द्रव्यमान बताइये। ()
View SolutionA body of mass 1kg is suspended from a spring of negligible mass. Another body of mass 500g moving vertically upwards hits the suspended body with a velocity of and gets embedded in it. If the frequency of oscillation of the system of the two bodies after collision is , the amplitude of the motion and the spring constant are respectively,
View SolutionTwo spring of spring constants are connected in series. One end of the combination is supported rigidly and the other to a body of mass 2 kg. Neglecting the masses of the springs, calculate the frequency of oscillation of the system.
View Solutionm द्रव्यमान का एक पिण्ड एक स्प्रिंग से बँधा हुआ है और दोलन करता है। यदि स्प्रिंग का बल नियतांक K हो तो पिण्ड का दोलनकाल होगा
View SolutionFind the period of small vertical oscillations of a body with mass m in the system illustrated in figure. The stiffness values of the springs are x_(1) and x_(2) , their masses are negligible.
View SolutionA block of mass m is suspended from a spring and executes vertical SHM of time period T as shown in Fig. The amplitude of the SHM is A and spring is never in compressed state during the oscillation. The magnitude of minimum force exerted by spring on the block is
View SolutionA block of mass m is suspended from a spring and executes vertical SHM of time period T as shown in Fig. The amplitude of the SHM is A and spring is never in compressed state during the oscillation. The magnitude of minimum force exerted by spring on the block is
View Solutionनगण्य द्रव्यमान कि स्प्रिंग से जुड़ा 2 किग्रा द्रव्यमान का पिण्ड सरल आवर्त गति कर रहा है । गति का आयाम 0.2 मीटर तथा आवर्तकाल 3.14 सेकण्ड है । पिण्ड का अधिकतम त्वरण तथा स्प्रिंग द्वारा आरोपित अधिकतम बल का मान ज्ञात कीजिए । स्प्रिंग का बल नियतांक (k ) क्या है ?
View Solution
