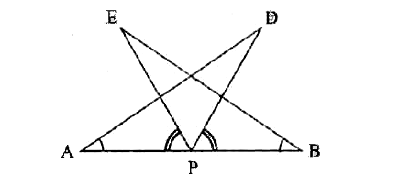
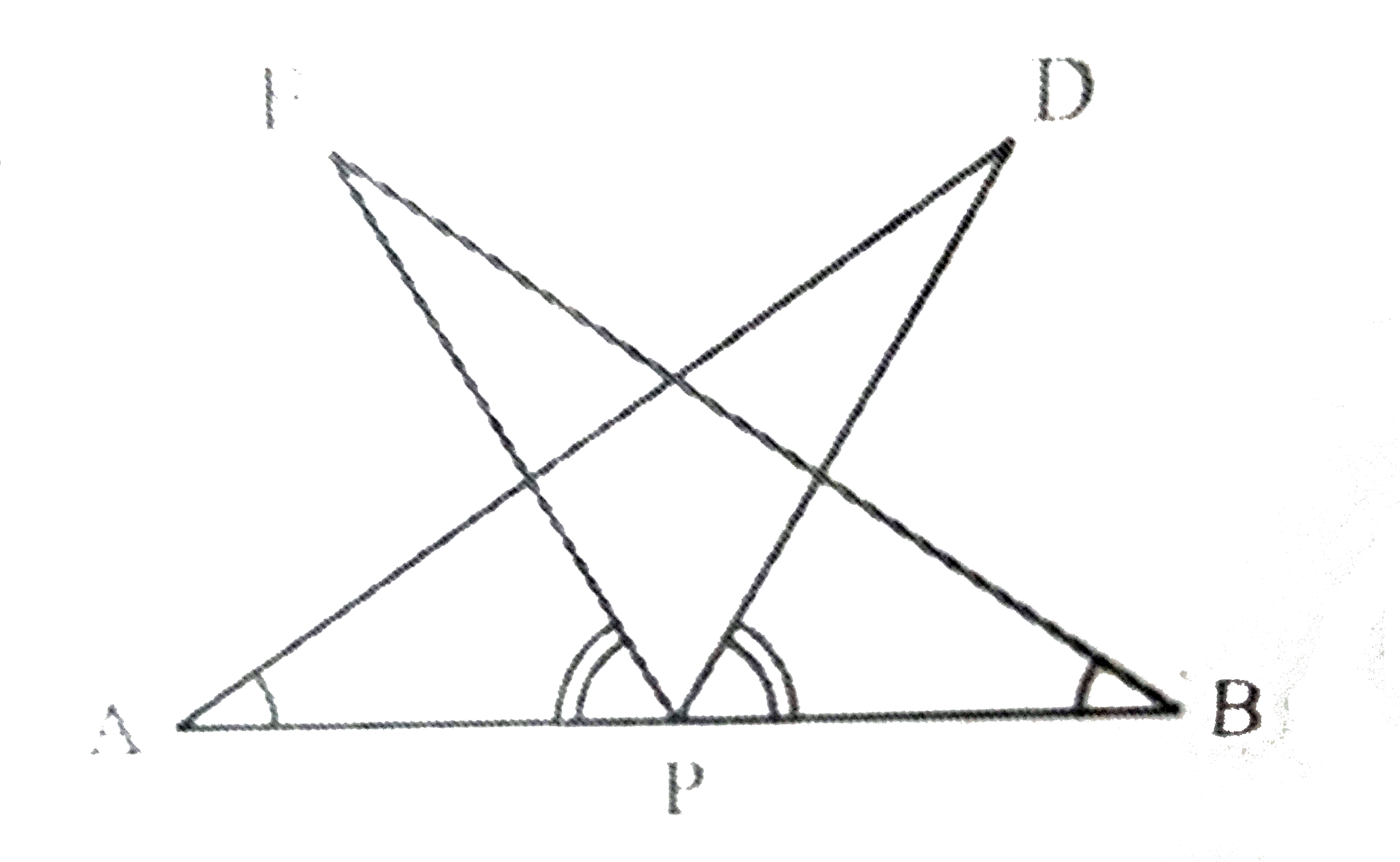
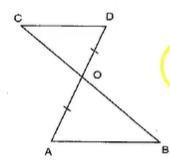
AB is a line segment and P is its mid-point. D and E are points on the same side of AB such that ∠BAD = ∠ABE and ∠EPA = ∠DPB See the given figure. Show that i ΔDAP ≅ ΔEBPii AD = BE
It is given that ∠EPA = ∠DPB∠EPA + ∠DPE = ∠DPB + ∠DPE∠DPA = ∠EPBIn ΔDAP and ΔEBP ∠DAP = ∠EBP GivenAP = BP P is mid-point of AB∠DPA = ∠EPB From above∴ ΔDAP ≅ ΔEBP ASA congruence ruleAD = BE By CPCT
Related Solutions
AB is line segment and P is its mid point. D and E are points on the same side of AB such that BAD = ABE and EPA = DPB show that
(i)
(ii) AD = BE
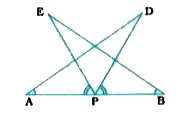
View SolutionAB is a line segment and P is its midpoint. D and E are points on the same side of AB such that (see the given figure). Show that:
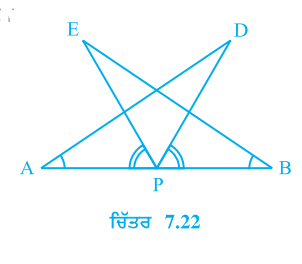
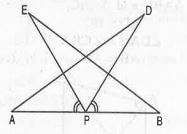
View SolutionAB ਇੱਕ ਰੇਖਾ ਖੰਡ ਹੈ ਅਤੇ P ਇਸਦਾ ਮੱਧ ਬਿੰਦੂ ਹੈ। D ਅਤੇ E ਰੇਖਾਖੰਡ AB ਦੇ ਇਕੋ ਪਾਸੇ ਦੋ ਬਿੰਦੂ ਇਸ ਤਰ੍ਹਾਂ ਹਨ ਕਿ ਅਤੇ (ਵੇਖੋ ਚਿੱਤਰ 7.22 ) ਸਿੱਧ ਕਰੋ(i) (ii) AD = BE
View SolutionAB is a line segment and P is its mid-point. D and E are points on the same side of AB such that angleBAD = angleABE and angleEPA = angleDPB (see figure). Show that AD=BE
View SolutionAB एक रेखाखण्ड है और P इसका लम्ब-बिन्दु है | D और E रेखाखण्ड AB के एक ही ओर स्थित दो बिन्दु इस प्रकार है कि -= और -= है | ( देखिए आकृति ) दर्शाइए कि-
(i) ~-=
(ii) AD -= BE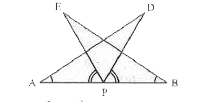
View SolutionAB is a line segment D and P is its mid-point. D and E are points on the same side of AB such that angleBAD = angleABE and angleEPA = angleDPB (see figure). Show that DeltaDAP~=DeltaEBP
View SolutionAB एक रेखाखंड है और P इसका मध्य-बिंदु है। D और E रेखाखंड AB के एक ही ओर स्थित दो बिंदु इस प्रकार हैं कि और है। दर्शाइए कि
(i)
(ii)
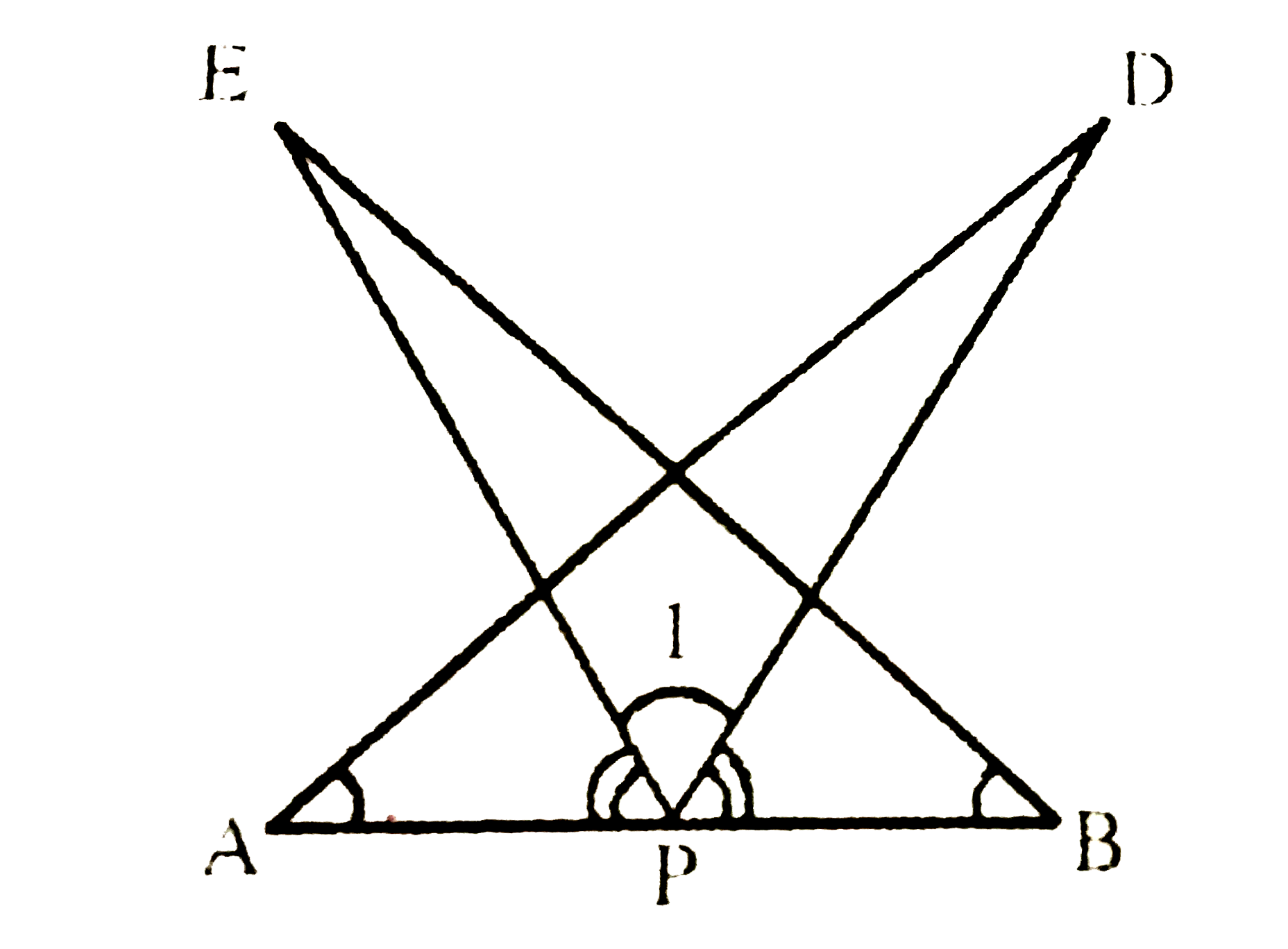
View SolutionAB is a line segment and P is its midpoint. D and E are points on the same side of AB such that and . Show that AD=BE.
View SolutionAB एक रेखाखंड है और P इसका मध्य बिंदु है | D और E रेखाखंड AB के एक ही ओर स्थित दो बिंदु इस प्रकार हैं कि और है | (देखिए चित्र) | दर्शाइए कि
(i)
(ii) AD = BE
View SolutionAB is a line and P is its midpoint. D and E are two points on the same side of line segment AB such that . Prove that
View SolutionLet AB be a line and P be its midpoint. To become angleBAD = angleABE And angleEPA = angleDPB Thus the points D and E are on the same side of AB (see Figure). (i) triangleDAP cong triangleEBP (ii) Show that AD = BE.
View SolutionIn given Fig.
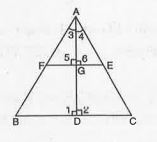 , is isosceles with AB = AC. D, E, F are the mid-points of sides BC, AC and AB respectively. Show that the line segment AD is perpendicular to the line segment EF and is bisected by it.
, is isosceles with AB = AC. D, E, F are the mid-points of sides BC, AC and AB respectively. Show that the line segment AD is perpendicular to the line segment EF and is bisected by it.
View SolutionIn given Fig.
 ,
,
is isosceles with AB = AC. D, E, F are the mid-points of sides BC, AC and AB respectively. Show that the line segment AD is perpendicular to the line segment EF and is bisected by it.
View SolutionAB एक रेखाखण्ड है तथा बिन्दु P और Q इस रेखाखण्ड AB के विपरीत और इस प्रकार स्थित है कि इनमे से प्रत्येक A और B से समदूरस्थ है ( देखिए आक्रति ) दर्शाइए कि रेखा PQ रेखाखण्ड AB का लम्ब समद्धिभाजक है |
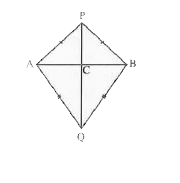
View SolutionAB is a line segment. P and Q are points on opposite sides of AB such that each of them is equidistant from the points A and B (see the given figure). Show that the line PQ is the perpendicular bisector of AB.
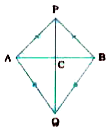
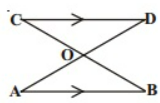
View SolutionIn the given figure and is the mid-point of a line segment .Show that is also the mid- point of any line segment , having its end points on and , respectively.
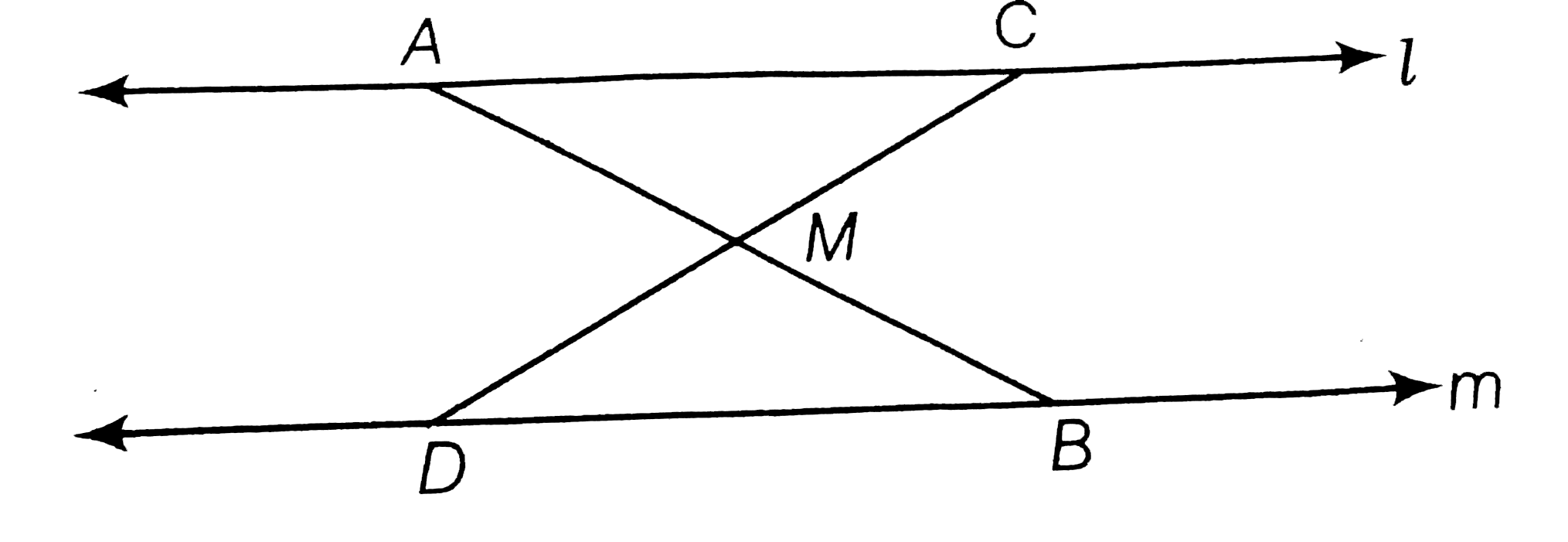
View SolutionIn Figure, line segments is parallel to another line segment is the mid-point of Show that:
View SolutionIn Figure, line segments is parallel to another line segment is the mid-point of Show that: is also the mid-point of
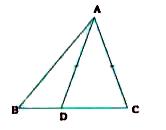
View SolutionD is a point on side BC of ABC such that AD = AC (see the given figure). Show that AB AD.
View SolutionIn give figur and M is the mid-point of a line segment AB .Show that M is also the mid- point of any line segment CD,having its end points on l and m,respectively.

View Solution